AP Physics Workbook 6.B Simple Harmonic Motion and Energy Review

Mr.S ClassRoom

14 Apr 202019:37

EducationalLearning

32 Likes 10 Comments

TLDRThe video script discusses the principles of simple harmonic motion and energy conservation in a scenario where a cart attached to a spring moves along an incline. It explains how the total mechanical energy remains constant, transitioning between kinetic and potential forms, with a focus on the spring's potential energy increasing quadratically with displacement and the system's energy distribution at various points throughout the motion.

Takeaways

📚 The scenario involves a cart of mass M attached to an ideal spring on a smooth angle track, with the cart initially at rest at Point E.
🌐 The system requires work to be done to stretch or compress the spring, which stores potential energy in the form of elastic potential energy.
🔄 The total energy (E) in the system is the sum of kinetic energy (KE) and potential energy (PE), with the conservation of energy law stating that the initial total energy equals the final total energy.
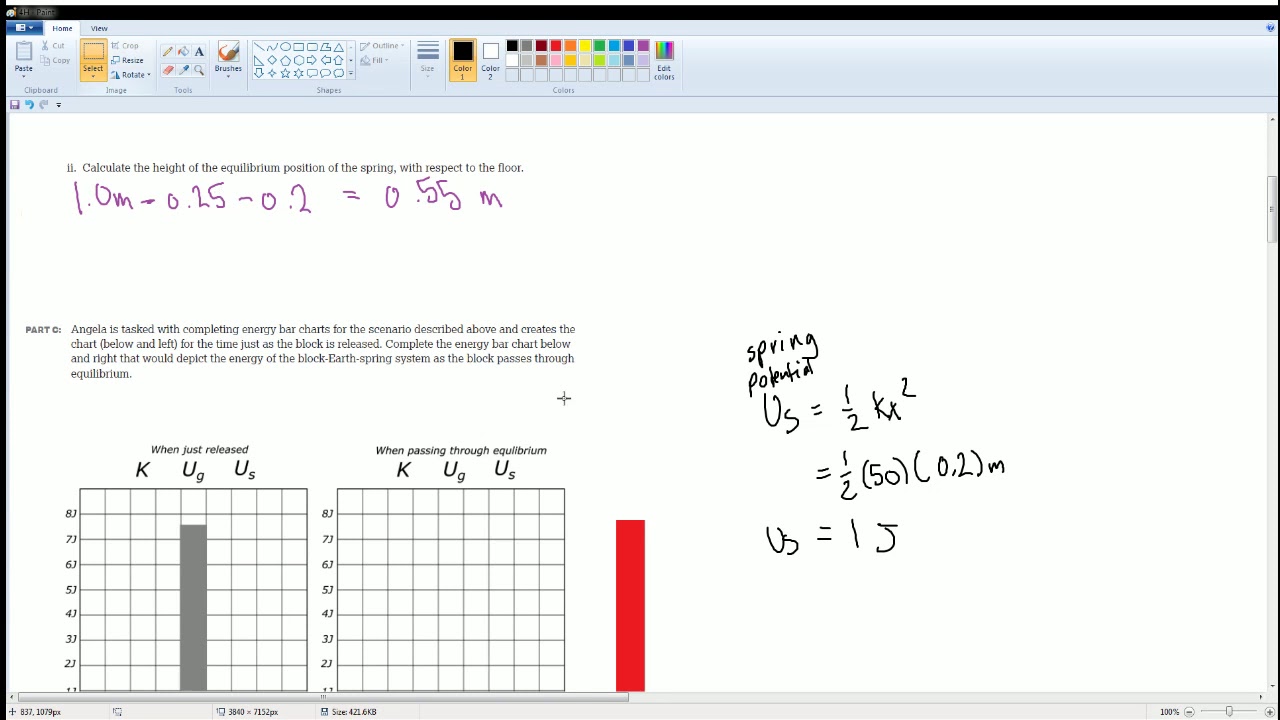
📈 The elastic potential energy is given by the formula 1/2 KX^2, where K is the spring constant and X is the displacement from the equilibrium position.
🚀 Kinetic energy is given by the formula 1/2 MV^2, where M is the mass and V is the velocity of the cart.
🔄 At Point A, the cart has maximum potential energy (all spring potential) and no kinetic energy, as it is at maximum displacement from equilibrium.
🔄 At Point E, the cart is at equilibrium with no kinetic or spring potential energy, as it is at the natural length of the spring and not moving.
🔄 As the cart moves from Point A to E, it gains gravitational potential energy due to the incline, and the system's energy transitions from spring potential to kinetic and then back to gravitational potential.
🔄 The energy bar chart does not depend on the direction of the cart's movement (left or right), as the potential and kinetic energies are squared, making the direction irrelevant.
🔄 The total energy at each labeled point should remain constant due to the conservation of energy, assuming no external forces are acting on the system.
📊 The spring potential energy increases quadratically as the cart moves from E to A because it is proportional to the square of the displacement (X^2).

Q & A

What is simple harmonic motion?
-Simple harmonic motion is a type of periodic motion where an object moves back and forth along a straight line or a curve, experiencing a restoring force that is directly proportional to the displacement from its equilibrium position and acts in the opposite direction of the displacement.
What is the natural length of a spring?
-The natural length of a spring is the length at which the spring rests without being stretched or compressed, when no external forces are acting upon it.
How is elastic potential energy calculated in a spring?
-The elastic potential energy stored in a spring is given by the formula EPE = (1/2) * k * x^2, where k is the spring constant and x is the displacement from the equilibrium position.
What is the relationship between kinetic energy and potential energy in simple harmonic motion?
-In simple harmonic motion, the total mechanical energy (the sum of kinetic and potential energy) remains constant. The kinetic energy and potential energy convert into each other as the object moves through its cycle, but their total value remains unchanged.
What is the significance of the conservation of energy principle in the context of the spring-cart scenario?
-The conservation of energy principle ensures that the total energy in the system (spring, cart, and Earth) remains constant throughout the motion. This means that the sum of gravitational potential energy, kinetic energy, and elastic potential energy at any point in the motion must equal the total initial energy.
Why does the cart have no kinetic energy at point A in the scenario?
-At point A, the cart is at its maximum displacement from the equilibrium position. It has stopped momentarily before reversing direction, so its velocity is zero, and hence, it has no kinetic energy at that instant.
How does the gravitational potential energy of the cart change as it moves from point B to point C?
-As the cart moves from point B to point C, it gains height on the inclined plane, which increases its gravitational potential energy. The increase in height results in an increase in gravitational potential energy, given by the formula U = mgh, where m is the mass of the cart, g is the acceleration due to gravity, and h is the height above the reference point.
What is the velocity of the cart at point E?
-At point E, the cart is at its equilibrium position. It has no velocity because it momentarily comes to rest before reversing direction and continuing its oscillation.
How does the spring potential energy change as the cart moves from point E to point A?
-As the cart moves from point E to point A, it stretches the spring from its natural length to its maximum displacement. This causes the spring potential energy to increase quadratically, following the formula EPE = (1/2) * k * x^2, where x is the displacement from the equilibrium position.
Why is the total energy the same at each labeled point in the energy bar chart?
-The total energy is the same at each labeled point because of the conservation of energy principle. In a closed system with no external forces, the total mechanical energy remains constant throughout the motion. This means that the sum of gravitational potential energy, kinetic energy, and spring potential energy at any point in the motion must equal the total initial energy.
How does the direction of the cart's movement affect the energy bar chart?
-The direction of the cart's movement does not affect the total energy values in the energy bar chart. The energies are scalar quantities, and the direction of motion does not change their magnitude. For instance, the kinetic energy and spring potential energy are squared in the formulas, which makes them positive regardless of the direction of displacement or velocity.

Outlines

00:00

📚 Introduction to Simple Harmonic Motion and Energy Concepts

This paragraph introduces the topic of Simple Harmonic Motion (SHM) and energy review in the context of AP Physics. It sets up a scenario involving a cart attached to a spring on an incline track. The cart is initially at rest at Point E and is pulled to a position A before being released. The discussion focuses on the energy transformations within the system, emphasizing the need to understand the work done and potential energy stored in the spring. The elastic potential energy is introduced as 1/2 KX^2, where K is the spring constant and X is the displacement from equilibrium. The total energy E is the sum of kinetic and potential energy, with the conservation of energy playing a crucial role in the analysis.

05:06

📈 Analysis of Energy Transformations at Various Points

This paragraph delves into the energy transformations as the cart moves from Point A to Point E and back. It explains the transition from potential energy to kinetic energy and vice versa during the oscillation of the spring. The narrative highlights the conservation of energy, emphasizing that the total energy remains constant throughout the motion. The paragraph also discusses the gravitational potential energy and its relationship with the height of the cart on the incline. It points out that the system never fully converts to kinetic energy due to the presence of gravitational potential energy from the incline. The conservation of mechanical energy is reiterated, with a focus on the energies at Points A, B, C, D, and E.

10:08

🤔 Thought Process for Filling the Bar Chart in Part A

This paragraph discusses the thought process required to fill in the bar chart for Part A of the problem. It emphasizes the conservation of energy, stating that the total energy in each point must be the same. The narrative explains the energy distribution at Point 2, where the cart has maximum spring potential energy and begins to move up the incline, gaining gravitational potential energy. It clarifies that the system never fully converts into kinetic energy due to the incline. The paragraph also addresses the energies at Points C and D, noting the presence of both kinetic and gravitational potential energy as the cart moves towards Point E, where it has all gravitational potential energy and no kinetic or spring potential energy.

15:12

🔄 Dependence of Energy Bar Chart on the Cart's Motion

This paragraph explores whether the energy bar chart depends on the cart's direction of motion (left or right) or its position on the track. It concludes that the energy distribution does not depend on the direction of motion, as the kinetic energy and spring potential are squared, eliminating the direction aspect. The paragraph also explains that the energy change is the same whether the object is moving left or right, emphasizing the mathematical and physical consistency of the energy transformations within the system.

💡 Conservation of Energy and Its Implications for the System

This paragraph reinforces the principle of conservation of energy and its implications for the cart-spring system. It explains that in a closed system with no external forces, the total mechanical energy remains constant. The discussion includes the interplay between the cart, spring, and the Earth, highlighting that the Earth provides gravitational potential energy. The paragraph clarifies that if the Earth were removed from the system, the energy distribution would be different, as the cart-spring system alone does not account for gravitational potential energy. The narrative also corrects any potential misunderstandings regarding the energy bar chart, emphasizing the need for consistency with the conservation of energy principle.

📊 Gravitational and Spring Potential Energy Changes

This paragraph examines the changes in gravitational and spring potential energy as the cart moves from Point A to E and back. It explains that the gravitational potential energy should increase uniformly between each labeled point as the cart moves up the incline. The spring potential energy is described as increasing quadratically between each point due to its proportionality to X^2. The paragraph clarifies that the potential energy quadruples with a doubling of the displacement X. The summary provides a clear understanding of how energy is conserved and transformed within the cart-spring system during its motion.

Mindmap

Keywords

💡Simple Harmonic Motion

Simple Harmonic Motion (SHM) is a type of periodic motion where an object moves back and forth along a straight line or a curve, experiencing a restoring force that is directly proportional to the displacement from its equilibrium position. In the video, the cart attached to a spring moving along an incline exemplifies SHM, where the spring's force pulls the cart back towards its equilibrium point.

💡Potential Energy

Potential energy is the stored energy an object has due to its position or condition. In the context of the video, elastic potential energy is associated with the energy stored in a spring when it is stretched or compressed. The video explains that this energy is given by the formula 1/2 KX^2, where K is the spring constant and X is the displacement from the equilibrium position.

💡Kinetic Energy

Kinetic energy is the energy of motion an object possesses. It depends on the object's mass and velocity. In the video, as the cart moves from point 'a' towards the equilibrium point 'E', its potential energy is converted into kinetic energy, which is represented by the formula 1/2 MV^2, where M is the mass of the cart and V is its velocity.

💡Spring Constant

The spring constant, denoted as K, is a measure of a spring's stiffness. It indicates the amount of force needed to extend or compress the spring by a certain distance. In the video, the spring constant is crucial for calculating the potential energy stored in the spring, as it relates to how much energy is stored per unit of displacement from the equilibrium position.

💡Conservation of Energy

The law of conservation of energy states that the total amount of energy in a closed system remains constant, though it may change from one form to another. In the video, this principle is used to explain how the sum of kinetic and potential energies at any point in the cart's motion remains the same, reflecting the conservation of energy in the system.

💡Equilibrium Position

The equilibrium position is the position where the net force acting on an object is zero, and thus the object is either at rest or moving at a constant velocity. In the context of the video, the equilibrium position is point 'E', where the cart is at the natural length of the spring without any stretch or compression, and it has no kinetic or potential energy associated with the spring.

💡Gravitational Potential Energy

Gravitational potential energy is the energy an object has due to its elevated position in a gravitational field. It is dependent on the object's mass, the height above a reference point, and the acceleration due to gravity. In the video, as the cart moves up the incline, it gains height and thus gains gravitational potential energy.

💡Work

In physics, work is a measure of energy transfer that occurs when an object is moved by an external force along a displacement. The work done on the system is equal to the force applied times the displacement. In the video, work is done on the spring when the cart is pulled to a position 'a', storing energy in the spring as potential energy.

💡Amplitude

Amplitude in the context of oscillations refers to the maximum displacement of an object from its equilibrium position. It is a measure of the extent of the motion in SHM. In the video, the amplitude is represented by 'a', the maximum distance the cart is pulled from the equilibrium position before it is released to oscillate.

💡Incline

An incline is a surface that is sloped, not perpendicular to the horizontal plane. In the video, the cart moves along an incline, which affects the gravitational potential energy of the system as the cart's height changes during its motion.

💡Frictionless Surface

A frictionless surface is an idealized surface where there is no friction, meaning no resistance to motion. In the video, the cart moves on a smooth, frictionless incline, which allows for the idealized analysis of the energy transformations without the energy loss due to friction.

Highlights

The scenario involves a cart of mass M attached to an ideal spring, moving on a smooth angle track.

The cart is pulled to a position A and then released, moving towards point E where it reverses direction.

Work must be done to stretch or compress the spring, storing potential energy in the process.

The elastic potential energy is given as one-half KX squared, where K is the spring constant.

The total energy E is the sum of kinetic and potential energy.

The law of conservation of energy includes both gravitational and spring potential energies.

At the equilibrium point x equals 0, the cart has no kinetic energy and its potential energy is at a minimum.

At position A, the cart is fully compressed and has all spring potential energy.

As the cart moves from A to E, it gains gravitational potential energy due to the incline.

The system never fully converts into kinetic energy due to the presence of gravitational potential energy.

At point E, the cart is at equilibrium with no kinetic or spring potential energy.

The conservation of energy principle states that the total mechanical energy of the system is conserved.

The energy bar chart must show that the total energy at each labeled point is the same.

The energy change does not depend on whether the cart is moving left or right, only on the magnitude of displacement and velocity.

The gravitational potential energy increases linearly as the cart moves from A to E due to the incline.

The spring potential energy increases quadratically as the cart moves from E to A.

The potential energy is directly proportional to the square of the displacement from equilibrium.

The conservation of energy is a fundamental principle in physics that applies to the cart-spring system.

The energy bar chart should accurately represent the conservation of energy and the transitions between potential and kinetic energy.

Transcripts

Browse More Related Video

2023 AP Physics 1 Free Response #1

'AP Physics 2023 Exam Solutions|Q1. PART C ( ii ) | Complete Step-by-Step Answers and Explanations"

AP Physics Workbook 6.I Changing Mass and Period of a Mass Spring SYstem

AP Physics Workbook 4.H Potential Energy and Choice of Zero

AP Physics 8-5 Potential Energy Curves

Open & Closed Systems in Energy - IB Physics