Integrating Exponential Functions By Substitution - Antiderivatives - Calculus

The Organic Chemistry Tutor
21 Feb 201711:16
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a comprehensive guide on integrating exponential functions. It begins with basic integration of e^(3x) and e^(2x), then progresses to more complex examples involving u-substitution and integration by substitution. The script demonstrates the technique of dividing complex integrals into simpler parts, handling fractions with exponentials in the numerator and denominator, and emphasizes the importance of understanding the properties of exponential functions and their derivatives. The final examples showcase the application of these methods to solve a variety of integral problems, reinforcing the concepts taught throughout the video.

Takeaways
  • 📚 The antiderivative of e^(3x) is simply e^(3x) + C, highlighting the property of exponential functions with constant bases.
  • 🔄 For the function e^(2x), the antiderivative is (e^(2x))/2 + C, demonstrating the division by the derivative of the exponent.
  • 🌟 When integrating e^(-5x), the technique involves using substitution with u = -5x, leading to the result -(e^(-5x))/5 + C.
  • 🧠 The concept of substitution is applied to x^4e^x by setting u = x^4, resulting in the antiderivative (1/4)e^u + C.
  • 🤔 For the function xe^(sqrt(1-e^x)), the substitution with u = 1 - e^x simplifies the integration process, leading to the antiderivative -(2/3)(1 - e^x)^(3/2) + C.
  • 📈 The integration of (e^x + e^-x)/(e^x - e^-x) is achieved by setting u = e^x - e^-x, and the result is the natural logarithm of the absolute value of u plus C.
  • 🌠 In the case of (e^(1/x^2))/(x^3), the substitution with u = 1/x^2 yields the antiderivative -(1/2)e^(1/u) + C.
  • 🔧 The script illustrates the method of splitting complex fractions, such as (e^(3x) + 4e^x + 5)/e^x, into simpler parts for easier integration.
  • 📊 The antiderivative of each term in the split fraction is found by simplifying the exponents and applying basic integration rules.
  • 🎓 The script emphasizes the importance of understanding the properties of exponential functions and the application of substitution and simplification techniques in integration.
Q & A

  • What is the antiderivative of e^(3x) dx?

    -The antiderivative of e^(3x) dx is simply e^(3x) + C, where C is the constant of integration.

  • How is the antiderivative of e^(2x) dx found?

    -The antiderivative of e^(2x) dx is calculated by dividing e^(2x) by its derivative, which is 2. So, the antiderivative is (1/2)e^(2x) + C.

  • What substitution technique is used to integrate e^(-5x) dx?

    -To integrate e^(-5x) dx, we use u-substitution. We let u = -5x, then du = -5 dx, and solving for dx gives us dx = du/(-5). After substituting these into the integral, we get the antiderivative as (-1/5)e^(-5x) + C.

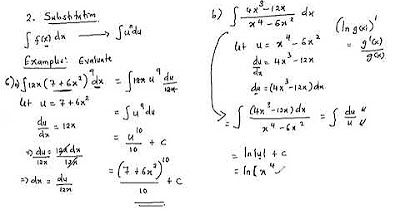
  • How does the u-substitution method work for integrating e^(x^4) dx?

    -For integrating e^(x^4) dx, we set u = x^4, which means du = 4x^3 dx. Solving for dx gives us dx = du/(4x^3). Substituting these into the integral and simplifying, we find the antiderivative to be (1/4)e^(x^4) + C.

  • What is the first step in integrating a function with u-substitution?

    -The first step in u-substitution is to choose an appropriate u that simplifies the integral. This usually involves setting u equal to a term or a combination of terms in the integrand that can be easily differentiated.

  • How do you handle a fraction with an exponential function in the numerator and denominator when integrating?

    -When integrating a fraction with an exponential function in both the numerator and denominator, you can first simplify the fraction by canceling out common terms. Then, you can integrate the simplified expression using u-substitution or other appropriate methods.

  • What is the role of the constant of integration (C) in an antiderivative?

    -The constant of integration (C) is added to the antiderivative to account for the infinite number of possible antiderivatives that exist for a given function. It represents the arbitrary constant that can be present in the solution to the differential equation.

  • How does the derivative of a function affect the process of integration?

    -The derivative of a function is crucial in the process of integration because it is the inverse operation. When integrating, you often need to find the derivative of the integrand to apply u-substitution or other integration techniques effectively.

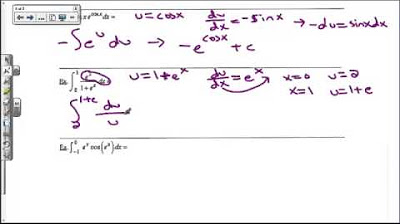
  • What is the antiderivative of e^(x) times the square root of (1 - e^x) dx?

    -To find the antiderivative of e^(x) times the square root of (1 - e^x) dx, we first let u = 1 - e^x, then du = -e^x dx. Solving for dx gives us dx = -du/e^x. The antiderivative is then (-2/3)(1 - e^x)^(3/2) + C.

  • How do you integrate a function that has a term in the numerator and the same term in the denominator?

    -When a function has a term in both the numerator and the denominator, you can often simplify the expression by canceling out the common terms. Then, you can integrate the simplified expression using the appropriate integration techniques.

  • What is the antiderivative of e^(-x)/x^3 dx?

    -To find the antiderivative of e^(-x)/x^3 dx, we first let u = 1/x^2. The derivative of u with respect to x is du = -2/x^3 dx. Solving for dx gives us dx = du/(-2x^2). Substituting these into the integral, we get the antiderivative as (-1/2)e^(-x)/x^2 + C.

  • What is the final answer for the integral of e^(x)/(x^3) dx?

    -The final answer for the integral of e^(x)/(x^3) dx is (-1/2)e^(x)/x^2 + C, after substituting back u = 1/x^2 and simplifying the expression.

Outlines
00:00
📚 Integration of Exponential Functions

This paragraph delves into the process of integrating exponential functions. It begins with the antiderivative of e^(3x) and explains that the answer is simply e^(3x) + C. The video then tackles the integration of e^(2x) by dividing it by its derivative, 2. A key concept highlighted is that this technique works only for division by constants. The paragraph further explores the integration of e^(-5x) using u-substitution, demonstrating the steps to arrive at the final answer of -1/5 * e^(-5x) + C. The technique of u-substitution is also applied to integrate x^4 * e^(x^4), resulting in 1/4 * e^(x^4) + C. The paragraph concludes with the integration of a more complex function, e^(x) * √(1 - e^x), and explains the process of substitution and simplification to obtain the final answer.

05:01
🧠 Advanced Integration Techniques

The second paragraph focuses on advanced integration techniques, starting with the integration of a function involving x and e^(x). The video explains the use of substitution, setting u as 1 - e^x, and solving for dx in terms of du. The final answer is presented as -2/3 * (1 - e^x)^(3/2) + C. The paragraph then moves on to the integration of a complex fraction involving e^(x) and e^(-x). Through the process of substitution and simplification, the video derives the natural logarithm of the absolute value of (e^(x) - e^(-x)) + C. The paragraph concludes with the integration of e^(1/x^2) / x^3, using substitution to find the antiderivative, which is -1/2 * e^(1/x^2) + C.

10:04
🔢 Fractional Exponential Integration

This paragraph addresses the integration of a fraction where the numerator is a sum of exponential terms and the denominator is e^x. The video script outlines the strategy of splitting the fraction into three separate fractions, each easier to integrate. The paragraph explains the process of simplifying each term by subtracting exponents and integrating term by term. The final answers for each term are combined to provide the overall solution, which includes e^(2x)/2, 4x, and -5e^(-x)/1 + C, showcasing the methodical approach to dealing with fractional exponential functions.

Mindmap
Keywords
💡Antiderivative
The antiderivative, also known as the indefinite integral, is a fundamental concept in calculus that represents a function whose derivative is given by a specified function. In the context of the video, finding the antiderivative of various exponential functions is the primary focus, such as determining the antiderivative of e^(3x) or e^(-5x). The process involves using different techniques, including direct integration and substitution methods, to find the integrand that, when differentiated, yields the original function.
💡Exponential Functions
Exponential functions are mathematical functions of the form f(x) = a^x, where 'a' is a constant and 'x' is the independent variable. These functions are crucial in many areas of mathematics and science due to their properties, such as continuous growth or decay. In the video, several exponential functions are integrated, like e^(2x) and e^(-5x), showcasing how their antiderivative can be found using calculus techniques.
💡Integration
Integration is the reverse process of differentiation in calculus. It is used to find the original function whose derivative is given, or to calculate the accumulated quantity under a curve. The video focuses on integrating exponential functions, using various methods such as direct integration and substitution with variables like u, to find the antiderivative.
💡Substitution
Substitution is a technique used in calculus to simplify complex integrals by replacing a part of the integrand with a new variable. This makes the integration process easier, especially when dealing with complicated functions. In the video, substitution is used to integrate functions like e^(-5x) by setting u as a negative 5x, which simplifies the integral and allows for easier computation of the antiderivative.
💡Derivative
The derivative of a function is a measure of how the function changes with respect to its independent variable. It is a fundamental concept in calculus and is used to analyze the rate of change, slope of a curve, and optimize functions. In the context of the video, derivatives are used to find the antiderivative of exponential functions, as the process often involves dividing by the derivative of a variable.
💡Constant
In mathematics, a constant is a value that does not change. In the context of the video, constants are used in the integration process, such as the constant 'C' that is added to the antiderivative to account for the arbitrary constant in indefinite integration. Constants are also important when dividing exponential functions by their derivatives, as seen when dividing e^(2x) by the derivative of 2x.
💡Integration by Substitution
Integration by substitution is a method used to evaluate integrals by transforming the original integral into a simpler one through the introduction of a new variable. This technique is particularly useful for integrals that are products of the function being integrated and its derivative. The video demonstrates this method by setting up u in terms of the original variable 'x' and then solving for 'dx' in terms of 'du', which simplifies the integration process.
💡Chain Rule
The chain rule is a fundamental calculus technique used to find the derivative of a composite function. It states that the derivative of a function composed of two or more functions is the product of the derivative of the outer function and the derivative of the inner function. Although not explicitly mentioned in the video, the concept is implicitly used when integrating functions like e^(-5x) by substituting 'u' and then applying the chain rule to find 'du' in terms of 'dx'.
💡U-Substitution
U-substitution, also known as variable substitution, is a technique used in integration to simplify the integrand by replacing it with a new variable (u) that is more manageable to integrate. This method is particularly useful for integrals that involve the product of the function and its derivative. In the video, u-substitution is employed to integrate complex exponential functions, such as e^(-5x) and e^(x^4), by setting u to expressions involving 'x' and then solving for 'dx' in terms of 'du'.
💡Integration Techniques
Integration techniques are methods used in calculus to evaluate integrals. These include direct integration, substitution, integration by parts, and trigonometric substitution, among others. The video focuses on direct integration and u-substitution as the primary techniques for integrating exponential functions. Understanding these techniques is crucial for solving more complex integrals and is a key theme of the video.
💡Arbitrary Constant
The arbitrary constant, often denoted as 'C', is added to the antiderivative of a function to account for the infinite number of possible antiderivatives that exist for a given derivative. Since differentiation is an injective process (different derivatives yield different originals), integration must account for the fact that adding a constant to an antiderivative does not change its derivative. In the video, 'C' is consistently added to the end of each antiderivative found, reflecting the principle that there are infinitely many functions that can be differentiated to yield the same derivative.
Highlights

The antiderivative of e^(3x) dx is simply e^(3x) + C.

For the function e^(2x), the antiderivative is e^(2x)/2 + C, using the property of dividing by the derivative of the exponent.

The antiderivative of e^(-5x) is found to be the same as the previous example, e^(-5x)/-5 + C.

U-substitution is used to prove the antiderivative of e^(-5x) by setting u as -5x and solving for du/dx.

For the integral of x^4 * e^(x^4) dx, substitution with u as x^4 simplifies the integration process.

The antiderivative of x^4 * e^(x^4) is 1/4 * e^(x^4) + C after substitution and simplification.

Integration by substitution is applied to the function involving e^(x) * sqrt(1 - e^x) dx.

The antiderivative of e^(x) * sqrt(1 - e^x) is -2/3 * (1 - e^x)^(3/2) + C after solving for du/dx and integrating.

For the complex function (e^x + e^(-x)) / (e^x - e^(-x)), the integration involves setting u as e^x - e^(-x) to simplify the expression.

The natural log function arises in the antiderivative of the complex function, resulting in ln(u) + C.

The antiderivative of e^(1/x^2) / x^3 dx is found by setting u as 1/x^2 and solving for dx, resulting in -1/2 * e^(1/x^2) + C.

The technique of splitting the fraction into smaller parts is used to integrate (e^(3x) + 4e^x + 5) / (e^x) dx.

Integration of the split fraction reveals the antiderivative to be 2x/2 - 1/x - 5/x + C.

The video demonstrates the use of exponent rules and substitution methods for integrating exponential functions.

The antiderivative of e^x involving different techniques showcases the flexibility in solving integrals.

The video provides a comprehensive guide on integrating exponential functions with various methods.

Transcripts

Browse More Related Video

Integrating Exponential Functions

4.6 - Integration Techniques - Integration by Parts

Integration using substitution method

Integration By Parts

Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems

Integral of a Product

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsCalculusIntegrationExponential FunctionsTutorialEducationProblem SolvingSubstitution MethodOnline Learning