Indefinite Integral of Exponential Functions | Calculus
TLDRThe video script presents a comprehensive guide on finding the indefinite integral of exponential functions. It begins by reviewing the basics of integration, emphasizing the use of u-substitution for non-linear functions. The video demonstrates the process through various examples, including integrals of the form a to the u, where a is a constant and u is a variable expression. It then tackles the main problem of integrating 4x times 5 to the x squared, using u-substitution with a detailed explanation of each step. The script also includes a method to verify the correctness of the solution by differentiating the antiderivative. The video concludes with an additional practice example, reinforcing the concept and encouraging further exploration of integration techniques.
Takeaways
- 📚 The indefinite integral of a function can be found using basic integration techniques and the u-substitution method.
- 🔍 When integrating a constant raised to a variable, remember that the derivative of that constant to the variable is the constant times the variable's derivative times the natural log of the constant.
- 🌟 For exponential functions, the integral can be found by separating the constant base and the variable exponent, and applying the formula for integration involving natural logs.
- 📈 The u-substitution method is particularly useful when the exponent is a linear function, allowing for straightforward application of the integration formula.
- 🧩 To verify the correctness of an indefinite integral, differentiate the result with respect to the variable and compare it to the original function.
- 🔧 When applying u-substitution, replace the variable with the u variable in the integrand and solve for the differential (du) in terms of the original variable's differential (dx).
- 🌐 For more complex exponential functions, such as those with sums or products in the exponent, identify the correct u variable and its derivative to apply the u-substitution method effectively.
- 📊 Practice is essential for mastering integration techniques; solving additional examples helps solidify understanding and application of the concepts.
- 🔗 The video provides additional resources in the description section for further learning on integration topics.
- 🎓 The process of integration involves recognizing patterns and applying the appropriate formulas, which can be reinforced through repeated practice and problem-solving.
Q & A
What is the indefinite integral of 5 raised to the x dx?
-The indefinite integral of 5 raised to the x dx is 5^x divided by the derivative of x (which is 1) times the natural log of 5, plus the constant of integration, so the answer is 5^x * ln(5) + C.
How does the formula for the integral of a to the u work?
-The integral of a to the u du is equal to a to the u divided by u prime times ln(a), where u prime is the derivative of u with respect to x. This formula is applicable when u is a linear function.
What is the indefinite integral of 3 raised to the 7x?
-The indefinite integral of 3 raised to the 7x is 3^(7x) divided by the derivative of 7x (which is 7) times the natural log of 3, plus the constant of integration, so the answer is 3^(7x) * ln(3)/7 + C.
How do you find the indefinite integral of 9 raised to the (4x + 5)?
-The indefinite integral of 9 raised to the (4x + 5) is 9^(4x + 5) divided by the derivative of (4x + 5) (which is 4) times the natural log of 9, plus the constant of integration, so the answer is 9^(4x + 5) * ln(9)/4 + C.
What is the u substitution method used for in integration?
-The u substitution method is used in integration to simplify the integrand by replacing a part of the expression with a new variable u, making the integration easier to perform.
How do you verify if the indefinite integral of an expression is correct?
-To verify if the indefinite integral is correct, you can differentiate the result with respect to x and check if you get the original expression. The derivative of a constant is zero, which helps in simplifying the verification process.
What is the indefinite integral of 4x times 5 raised to the x squared dx?
-The indefinite integral of 4x times 5 raised to the x squared dx is 2 times 5 raised to the x squared divided by the natural log of 5, plus the constant of integration, so the answer is 2*5^(x^2) * ln(5) + C.
How do you use the u substitution method to solve the integral of 9 x squared times 8 raised to the x cubed dx?
-To solve the integral of 9 x squared times 8 raised to the x cubed dx using u substitution, let u equal to x cubed, so du is 3x squared dx. Then replace x cubed with u and dx with du/3x squared. The indefinite integral becomes 3 times the integral of 8 raised to u du. Finally, replace u with x cubed to get the result, which is 3*8^(x^3) * ln(8)/3 + C.
What is the importance of the natural log in integration involving exponential functions?
-The natural log is important in integration involving exponential functions because it helps in simplifying the expression when integrating a constant raised to a variable. It is used in the formula for the integral of a to the u, where the natural log of the base is multiplied by the derivative of u.
What is the role of the constant of integration (C) in indefinite integrals?
-The constant of integration (C) is added to the indefinite integral to account for the arbitrary constant that arises from the indefinite nature of the integration process. It represents the constant that can be added to any antiderivative without changing the derivative of the function.
How does the derivative of a constant raised to a variable affect the integration process?
-The derivative of a constant raised to a variable is equal to the constant to the variable times the variable prime (the derivative of the variable) times the natural log of the constant. This relationship is crucial in integration by parts and substitution methods, where it helps to simplify the integrand and find the antiderivative.
Outlines
📚 Introduction to Indefinite Integrals of Exponential Functions
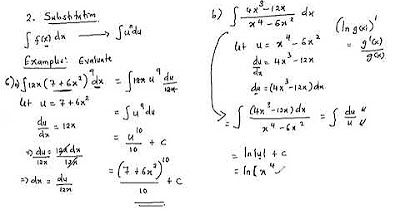
This paragraph introduces the concept of finding the indefinite integral of exponential functions. It begins with the problem of finding the indefinite integral of 4x times 5 raised to the power of x squared. The video emphasizes the importance of understanding the basics before tackling this problem. It starts with a simpler example, the indefinite integral of 5 raised to the power of x, and explains the process of using the power rule for integration. The concept of u-substitution is introduced, and the formula for integrating a constant raised to a variable is derived. The paragraph also provides examples to illustrate the process, such as finding the indefinite integral of 3 raised to the power of 7x and 9 raised to the power of 4x plus 5. The explanation includes identifying the correct u and u prime for each example and applying the integration formula accordingly.
🧠 Solving the Original Problem and Verification
The paragraph focuses on solving the original problem of finding the indefinite integral of 4x times 5 raised to the power of x squared. It explains the process of u-substitution by setting u equal to x squared and finding its derivative. The integral is then expressed in terms of u, and the formula for integrating a power function is applied. The paragraph also covers the verification of the solution by differentiating the antiderivative to obtain the original function. The verification process confirms that the derived antiderivative matches the original integrand, thus validating the solution. Additionally, the paragraph provides another example of integrating 9x squared times 8 raised to the power of x cubed, further demonstrating the u-substitution method and its application to more complex exponential functions.
🔗 Additional Resources and Conclusion
In this final paragraph, the video concludes by directing viewers to additional resources for learning about integration. The speaker encourages the audience to check the description section below the video for links to helpful materials. The video wraps up with a reminder to subscribe to the channel for more content on related topics. This paragraph serves as a closing segment, summarizing the content covered in the video and providing guidance on where to find further information.
Mindmap
Keywords
💡indefinite integral
💡exponential function
💡u-substitution
💡derivative
💡constant
💡natural log
💡constant of integration
💡integration techniques
💡antiderivative
💡differentiation
💡integration by substitution
Highlights
The video discusses finding the indefinite integral of 4x times 5 raised to the power of x squared.
Before solving the main problem, the video covers the basics of finding the indefinite integral of 5 raised to the power of x.
The derivative of a constant raised to a variable is explained as the constant to the variable times the derivative of the variable.
The integral of a to the u is equal to a to the u divided by u prime times the natural log of the constant, plus the constant of integration.
The video demonstrates how to find the indefinite integral of 3 raised to the power of 7x using the explained formula.
The indefinite integral of 9 raised to the power of 4x plus 5 is calculated by identifying a, u, and u prime.
The video explains the use of u substitution for solving integrals of exponential functions.
The integral of 4x times 5 raised to the power of x squared is found by substituting x squared with u and 2x with du.
The antiderivative of 5 raised to the power of u is calculated using the natural log of the base 5.
The final answer for the main problem is 2 times 5 raised to the power of x squared divided by the natural log of 5 plus a constant.
The correctness of the answer is verified by differentiating the antiderivative and matching it with the original function.
Another example is provided to practice finding the integral of 9x squared times 8 raised to the power of x cubed.
The video concludes by summarizing the method for finding the antiderivative of exponential functions using u substitution.
Additional resources for learning integration are mentioned in the video description.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: