AP Physics Workbook 4.H Potential Energy and Choice of Zero

Mr.S ClassRoom
3 May 202011:54
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses a physics problem involving a spring and a block. It explains the forces acting on the block, the use of Hooke's law to calculate the displacement of the spring, and the concept of potential and kinetic energy. The video also addresses the impact of changing the zero point for gravitational potential energy and demonstrates through an interactive example that the total mechanical energy remains constant, regardless of the chosen reference point for potential energy. The problem is solved with detailed calculations, resulting in the block's motion and energy distribution at equilibrium.

Takeaways
  • 📚 The lesson focuses on understanding work, energy, potential energy, and the concept of choosing a reference point for zero potential energy.
  • 🌟 The scenario involves a spring with an unstretched length (L_0) of 0.25 meters and a spring constant (K) of 250 N/m, hung vertically with a 1 kg block attached.
  • 🔍 In Part A, forces are identified: gravitational force pulling the block down and the spring's restorative force, which is described by Hooke's Law.
  • ⚖️ Newton's second law is applied to find the stretch in the spring when the block is in equilibrium: F_spring = -mg, leading to x = mg/K.
  • 📐 The displacement of the spring from its natural length is calculated to be 0.2 meters, using the given values for gravity and the spring constant.
  • 📊 The height of the equilibrium position is determined to be 0.55 meters above the floor, considering the unstretched length of the spring.
  • 💡 The energy chart is completed, showing 1 Joule of spring potential energy, 1 Joule of kinetic energy, and 5.5 Joules of gravitational potential energy at the equilibrium position.
  • 🌐 Dominique's assertion is highlighted: the height above the ground doesn't affect the energy calculations, only the changes in energy matter.
  • 🔄 The interactive demonstration illustrates that the mechanical energy of the system (spring potential energy + kinetic energy) remains constant regardless of the choice of the zero potential energy reference point.
  • 🔽 Moving the ground closer to the spring decreases the total mechanical energy but does not affect the individual values of spring potential energy and kinetic energy.
  • 🎯 The final chart and interactive reaffirm that the motion of the system and the values of kinetic and spring potential energy remain unchanged, regardless of the positioning of the gravitational potential energy reference point.
Q & A

  • What is the unstretched length (L naught) of the spring in the given scenario?

    -The unstretched length (L naught) of the spring is 0.25 meters.

  • What is the spring constant (K) for the spring in the physics problem?

    -The spring constant (K) is 250 Newton meters per unit length.

  • What is the mass of the block attached to the spring?

    -The mass of the block is 1 kilogram.

  • What are the forces acting on the block when it is in equilibrium?

    -The forces acting on the block in equilibrium are the force of gravity pulling it down and the restorative force from the spring, which is described by Hooke's law.

  • How can we calculate the displacement of the spring when the block is in equilibrium?

    -We can calculate the displacement of the spring using Newton's second law, setting the net force to zero and solving for x, which gives us x = (mg / K). With the given values, the displacement is 0.2 meters.

  • What is the height of the equilibrium position of the block with respect to the floor?

    -The height of the equilibrium position is the total length (1 meter) minus the unstretched length of the spring (0.25 meters) and the displacement (0.2 meters), resulting in 0.55 meters.

  • How does the spring potential energy (U_spring) change as the block moves from its highest to its lowest point?

    -The spring potential energy changes from 0 Joules at the highest point (no displacement) to 1 Joule at the lowest point (maximum displacement of 0.2 meters), following the formula (1/2)KX^2.

  • What is the total mechanical energy of the block-spring system at the equilibrium position?

    -At the equilibrium position, the total mechanical energy is the sum of the spring potential energy, kinetic energy, and gravitational potential energy, which is 1 Joule (spring potential) + 1 Joule (kinetic) + 5.5 Joules (gravitational potential).

  • Does changing the height above the ground affect the spring potential energy and kinetic energy of the system?

    -No, changing the height above the ground does not affect the spring potential energy and kinetic energy of the system. These energies are independent of the zero position of the gravitational potential energy.

  • What can we conclude from the interactive demonstration about the effect of the ground position on the system's energy?

    -The interactive demonstration shows that the system's spring potential energy and kinetic energy remain constant regardless of the ground position. Only the total mechanical energy changes when the ground position is altered.

  • How does the concept of mechanical energy conservation apply to the block-spring system?

    -The concept of mechanical energy conservation states that the total mechanical energy (sum of kinetic, potential, and spring energies) remains constant in the absence of non-conservative forces. In the block-spring system, this means that as the block oscillates, the energy transitions between kinetic, potential, and spring forms, but the total mechanical energy is conserved.

Outlines
00:00
📚 Introduction to Work, Energy, and Springs

This paragraph introduces the concepts of work and energy in the context of a physics workbook, focusing on potential energy and the behavior of a spring. It describes a scenario where a 1-kilogram block is attached to a vertically hung spring with a spring constant of 250 N/m. The spring is unstretched at a length of 0.25 meters. The paragraph details the forces acting on the block, including gravity and the restorative force from the spring, as described by Hooke's Law. It explains how to calculate the displacement of the spring (x) when the block is in equilibrium, using Newton's second law. The calculation results in a displacement of 0.2 meters. The height of the equilibrium position is then determined to be 0.55 meters. The paragraph also discusses the energy chart that needs to be completed, indicating the total energy at different points in the system's motion.

05:02
🔋 Calculation of Spring and Gravitational Potential Energy

This paragraph delves into the calculation of spring potential energy and gravitational potential energy. It explains how to determine the spring potential energy using the formula (1/2)KX^2, where K is the spring constant and X is the displacement. The calculation yields 1 Joule of spring potential energy. The paragraph then addresses the distribution of energy in the system, with 1 Joule attributed to kinetic energy and 5.5 Joules to gravitational potential energy. It emphasizes that the height above the ground does not affect the change in energy within the system. The concept is further illustrated with an interactive example, showing that the mechanical energy of the system remains constant despite changes in the position of the ground. The interactive concludes by reinforcing that the spring potential energy and kinetic energy remain unaffected by the choice of the zero point for gravitational potential energy.

10:02
🌐 Interactive Explanation and Conclusion

The final paragraph uses an interactive to visually explain the concepts discussed earlier. It shows a mass on a spring at rest, with no potential or kinetic energy due to the absence of displacement and velocity. As the mass is stretched and released, it gains spring potential and kinetic energy. The interactive demonstrates the conservation of mechanical energy as the mass oscillates between potential and kinetic forms. The total mechanical energy remains constant, even when the position of the ground (and thus the zero point for gravitational potential energy) is changed. The paragraph concludes by reiterating that the motion of the system is unaffected by the choice of the equilibrium height for gravitational potential energy, as the values of kinetic and spring potential energy remain constant.

Mindmap
Keywords
💡Work and Energy
Work and energy are fundamental concepts in physics. Work is defined as the force applied to an object times the distance moved in the direction of the force, and it is a measure of energy transfer. In the context of the video, work is done by the spring's force and gravity on the block, which leads to the change in energy of the system. Energy, on the other hand, is the capacity to do work and exists in various forms such as kinetic, potential, and mechanical energy. The video discusses the transformation of these energy forms as the block oscillates due to the spring's motion.
💡Hooke's Law
Hooke's Law is a principle in physics that describes the force exerted by a spring. It states that the force (F) exerted by a spring is proportional to the displacement (x) from its equilibrium position, with the proportionality constant being the spring constant (k). In the video, Hooke's Law is used to calculate the restorative force of the spring and to determine the displacement of the spring when the block is in equilibrium with the force of gravity.
💡Newton's Second Law
Newton's Second Law of Motion states that the force (F) acting on an object is equal to the mass (m) of the object times its acceleration (a). This law is fundamental in understanding the dynamics of objects and is used in the video to analyze the forces acting on the block and to determine the conditions for equilibrium.
💡Potential Energy
Potential energy is the stored energy an object has due to its position or condition. In the context of the video, potential energy is associated with the spring (spring potential energy) and the height of the block above a reference level (gravitational potential energy). The video explains how potential energy changes as the block moves up and down the spring, and how it is related to the spring's displacement and the height of the block above the floor.
💡Kinetic Energy
Kinetic energy is the energy an object possesses due to its motion. It is defined as (1/2)mv^2, where m is the mass of the object and v is its velocity. In the video, kinetic energy is discussed in relation to the block's motion as it oscillates on the spring, transitioning from potential energy to kinetic energy and vice versa.
💡Mechanical Energy
Mechanical energy is the sum of kinetic and potential energies in a system. It is a conserved quantity in an isolated system, meaning the total mechanical energy remains constant if no external forces are acting on it. The video emphasizes the conservation of mechanical energy as the block oscillates on the spring, with the transformation between kinetic, spring potential, and gravitational potential energy.
💡Equilibrium
Equilibrium in physics refers to a state where all forces acting on an object are balanced, resulting in no net force and thus no acceleration. In the video, equilibrium is discussed in relation to the block hanging from the spring, where the gravitational force is balanced by the spring's restorative force.
💡Displacement
Displacement in the context of waves and vibrations refers to the change in position of a point mass from its equilibrium position. In the video, displacement is used to describe the stretch or compression of the spring as the block moves up or down, which is essential for calculating the spring potential energy.
💡Spring Constant
The spring constant, denoted by k, is a measure of the stiffness of a spring. It is defined as the force needed to stretch or compress the spring by one unit of length. In the video, the spring constant is used in Hooke's Law to determine the force exerted by the spring and in the calculation of potential energy.
💡Acceleration
Acceleration is the rate of change of velocity of an object with time. It is a vector quantity that describes how quickly an object speeds up, slows down, or changes direction. In the context of the video, acceleration is used in Newton's Second Law to analyze the dynamics of the block as it moves under the influence of gravity and the spring's force.
💡Conservation of Energy
The principle of conservation of energy states that energy cannot be created or destroyed, only transformed from one form to another. In the video, this principle is illustrated by the block-spring system, where mechanical energy is conserved, and energy transforms between kinetic, potential, and spring potential forms without a loss or gain in total mechanical energy.
Highlights

Introduction to the AP Physics workbook focusing on work and energy, specifically point H.

Explanation of the scenario with a spring of unstretched length L naught and spring constant K.

Description of the setup with a 1-kilogram block attached to the spring in a vertically hung configuration.

Labeling of forces in Part A, including gravity and the normal force according to Hooke's law.

Application of Newton's second law to find the string stretch in equilibrium.

Calculation of the displacement of the spring, resulting in 0.2 meters.

Determination of the height of the equilibrium position with respect to the floor, calculated as 0.55 meters.

Completion of the energy chart based on the system's release and passage through equilibrium.

Calculation of spring potential energy using the formula (1/2)KX^2, resulting in 1 Joule.

Discussion on the distribution of energy into kinetic, spring potential, and gravitational potential.

Explanation by Dominique that the height above the ground doesn't affect the energy changes in the system.

Interactive demonstration showing the oscillation of the system between spring potential and kinetic energy.

Observation that the positioning of the ground does not change the spring's potential or kinetic energy.

Final chart illustrating 1 Joule of kinetic energy and 1 Joule of spring potential energy, unaffected by the zero point of gravitational potential.

Conclusion that the motion of the system remains the same regardless of the zero location of the gravitational potential energy.

Transcripts

Browse More Related Video

2015 AP Physics 1 free response 3a

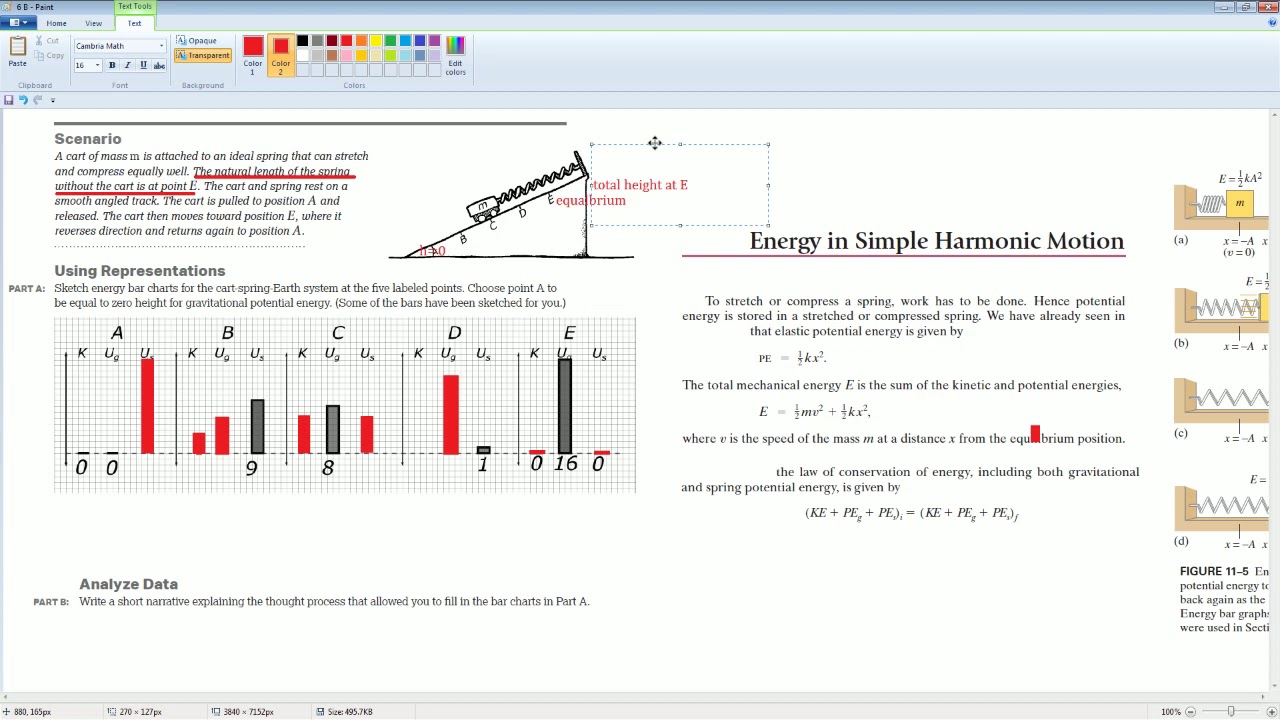
AP Physics Workbook 6.B Simple Harmonic Motion and Energy Review

2023 AP Physics 1 Free Response #1

'AP Physics 2023 Exam Solutions|Q1. PART C ( ii ) | Complete Step-by-Step Answers and Explanations"

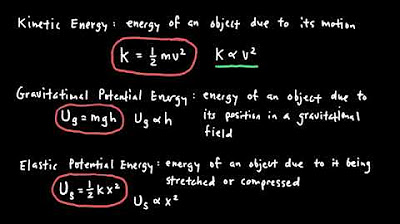
AP Physics 1 Energy Review

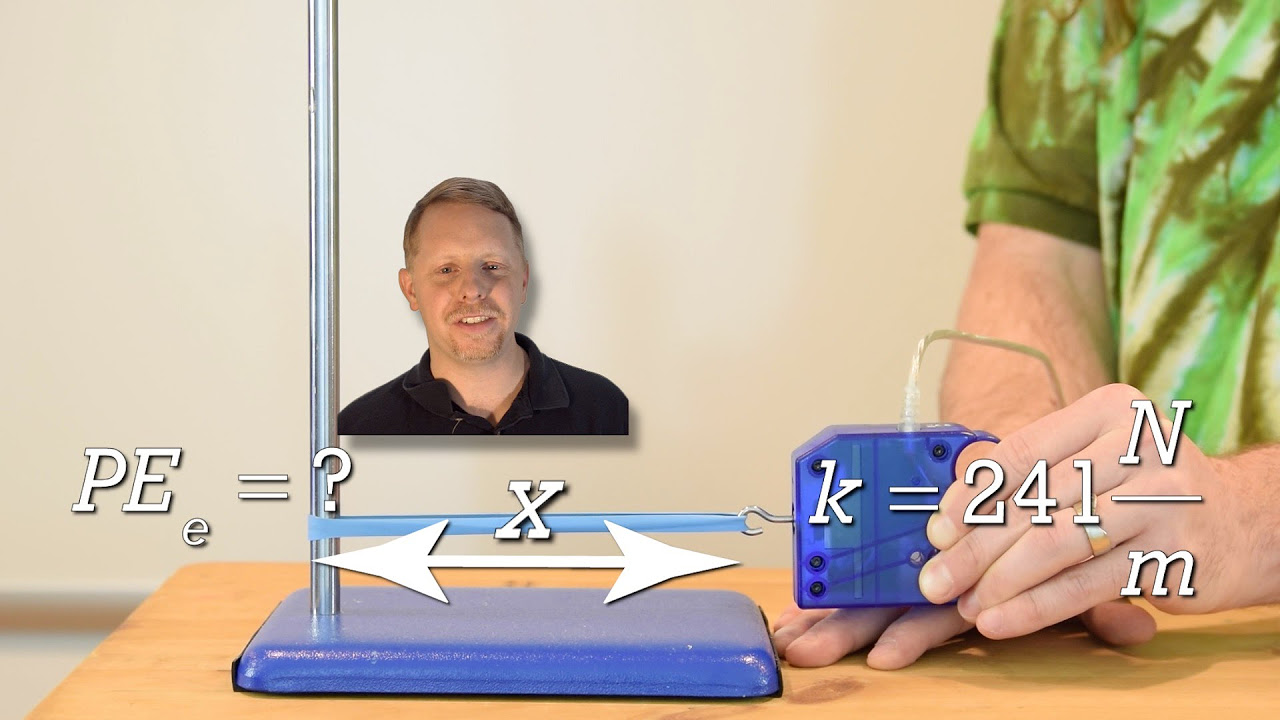
(previous version) Introduction to Elastic Potential Energy with Examples

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics ConceptsSpring-Mass SystemWork and EnergyHooke's LawMechanical EquilibriumInteractive SimulationAP PhysicsEducational ContentConceptual UnderstandingEnergy Conservation