Limits at infinity of quotients (Part 1) | Limits and continuity | AP Calculus AB | Khan Academy
TLDRThe video script discusses the limit of a function as x approaches infinity. It explains that by focusing on the highest degree terms in the numerator and denominator, one can determine the behavior of the function for very large values of x. The example given, f(x) = (4x^5 - 3x^2 + 3) / (6x^5 - 100x^2 - 10), simplifies to a horizontal asymptote at y = 2/3 for both positive and negative infinity. The script emphasizes the importance of identifying dominant terms to understand the function's behavior at extreme values.
Takeaways
- 📚 The function f(x) is given by a complex rational expression involving high-degree terms of x.
- 🌟 To find the limit of f(x) as x approaches infinity, one can either plug in large values of x or reason through the behavior of the expression's numerator and denominator.
- 🔍 When reasoning, focus on the highest degree terms in the numerator and denominator, as they will dominate the behavior of the function for very large values of x.
- 💡 As x becomes very large, the term 4x to the fifth in the numerator and 6x to the fifth in the denominator become the most significant.
- 📈 The limit of f(x) as x approaches infinity can be approximated by simplifying the dominant terms to x to the fifth divided by x to the fifth, which simplifies to 2/3.
- 📊 A graph from Wolfram Alpha confirms that the function f(x) approaches a horizontal asymptote at y equals 2/3 as x gets larger.
- 🔄 The logic for large positive x values also applies to large negative x values, leading to the same horizontal asymptote of 2/3 as x approaches negative infinity.
- 🤔 The process of determining the limit involves identifying which terms will dominate as x becomes very large or very small.
- 🌐 The horizontal asymptote is a visual representation of the limit of the function as x approaches infinity or negative infinity, which can be observed in the graph.
- 🎯 This analysis is a demonstration of how to approach limits in complex functions by focusing on the leading terms and their behavior at extreme values.
Q & A
What is the given function f(x) in the script?
-The given function f(x) is 4x to the fifth minus 3x squared, plus 3, all of that over 6x to the fifth minus 100x squared minus 10.
What is the limit of f(x) as x approaches infinity?
-The limit of f(x) as x approaches infinity is 2/3.
How does the script suggest to find the limit of f(x) as x approaches infinity?
-The script suggests two methods: plugging in larger and larger numbers for x to see if it approaches a value, or reasoning through the behavior of the numerator and denominator as x gets very large.
Why does the term 4x to the fifth become more significant as x gets very large?
-The term 4x to the fifth becomes more significant because when you raise a number to a higher power, it grows significantly faster than squaring it or any lower power.
What is the highest degree term in the denominator of f(x)?
-The highest degree term in the denominator is 6x to the fifth.
How does the term 6x to the fifth affect the limit of f(x) as x approaches infinity?
-The term 6x to the fifth grows very fast as x increases, and it dominates the other terms in the denominator. When simplified, x to the fifth divided by x to the fifth equals 1, leading to the limit being 2/3.
What does the script imply about the behavior of f(x) as x approaches negative infinity?
-The script implies that as x approaches negative infinity, f(x) behaves similarly to when x approaches positive infinity, and the limit is also 2/3.
What is a horizontal asymptote in the context of the given function?
-A horizontal asymptote is a horizontal line that the graph of the function approaches as x gets very large or very small. In this case, the horizontal asymptote is at y equals 2/3.
How does the graph from Wolfram Alpha support the conclusions drawn in the script?
-The graph from Wolfram Alpha shows that as x gets larger or smaller, f(x) approaches the value of 2/3, confirming the horizontal asymptote and the limits as x approaches infinity or negative infinity.
What is the key takeaway from the script for solving limits of rational functions?
-The key takeaway is to focus on the dominant terms in the numerator and denominator when x is very large or very small, as these terms will dictate the behavior and the limit of the function.
How does the script illustrate the concept of dominant terms in a polynomial?
-The script illustrates that when dealing with large values of x, the terms with the highest powers (like 4x to the fifth and 6x to the fifth) become dominant and dictate the function's behavior and its limit as x approaches infinity or negative infinity.
Outlines
📈 Understanding Limits at Infinity
The script explores calculating the limit of a complex function, f(x) = (4x^5 - 3x^2 + 3)/(6x^5 - 100x^2 - 10), as x approaches infinity. The narrative starts by suggesting two methods to find the limit: direct substitution of large values for x or a more theoretical approach focused on the function's behavior at large x values. It highlights that for large x, the dominant terms in both the numerator and the denominator are those with the highest power of x, in this case, x^5. This dominance renders other terms insignificant, leading to a simplification of the function to (4x^5)/(6x^5), which further simplifies to 2/3. Thus, the script concludes that as x approaches both positive and negative infinity, the function approaches a limit of 2/3, indicating a horizontal asymptote at y = 2/3. This theoretical deduction is validated by observing the function's graph, which confirms the limit and the presence of the horizontal asymptote at y = 2/3, for both positive and negative values of x. The discussion encapsulates the significance of dominant terms in determining the behavior of functions at the extremes of their domain.
Mindmap
Keywords
💡limit
💡asymptote
💡numerator
💡denominator
💡degree
💡Wolfram Alpha
💡graph
💡dominant terms
💡cancel out
💡reasoning
Highlights
The function f(x) is given as 4x to the fifth minus 3x squared, plus 3, over 6x to the fifth minus 100x squared minus 10.
The limit of f(x) as x approaches infinity is being investigated.
One approach to find the limit is by plugging in larger numbers for x and observing the behavior.
Another method is to reason through the behavior of the numerator and denominator as x becomes very large.
As x becomes very large, the term 4x to the fifth in the numerator becomes significantly more important.
Similarly, the highest degree term 6x to the fifth in the denominator grows much faster than other terms.
The function f(x) simplifies to 4x to the fifth over 6x to the fifth for large x, which further simplifies to 2/3.
The limit of f(x) as x approaches infinity is 2/3, indicating a horizontal asymptote at y equals 2/3.
Wolfram Alpha graph confirms that f(x) approaches 2/3 as x becomes very large in both positive and negative directions.
The horizontal asymptote at y equals 2/3 is observed from both the top and bottom approaches to negative and positive infinity.
The dominant terms in the function are 4x to the fifth and 6x to the fifth when x is very large or negative.
The limit of f(x) as x approaches negative infinity also equals 2/3, following the same logic as for positive infinity.
The graph from Wolfram Alpha illustrates a clear horizontal asymptote at y equals 2/3 for the function f(x).
When analyzing limits, it's crucial to focus on the terms that will dominate as x becomes very large or negative.
The concept of horizontal asymptotes is crucial in understanding the behavior of functions at infinity.
This analysis demonstrates the importance of identifying and focusing on the leading terms of a function for large values of x.
Transcripts
Browse More Related Video

Limits at infinity of quotients (Part 2) | Limits and continuity | AP Calculus AB | Khan Academy
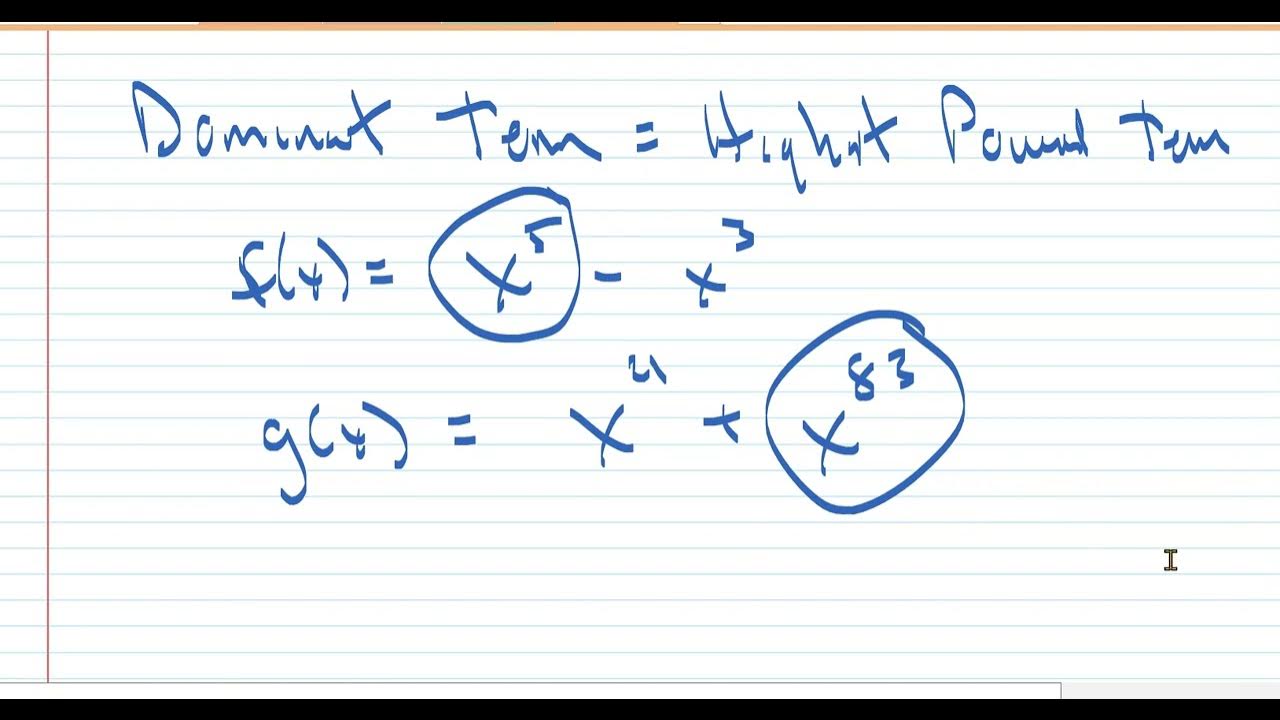
Calculus:Limits of Rational Functions

Infinite Limit vs Limits at Infinity of a Composite Function
Limits "at" infinity

Finding a Horizontal Asymptote of a Rational Function (Precalculus - College Algebra 40)
Where a function is not differentiable | Taking derivatives | Differential Calculus | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: