Where a function is not differentiable | Taking derivatives | Differential Calculus | Khan Academy

Khan Academy
22 Aug 201303:35
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of differentiability in the context of a function f(x). It visually analyzes the function's behavior, identifying points at x = -2 and x = 3 where the function's slope changes abruptly, indicating non-differentiability. The explanation emphasizes the importance of the limit of the secant line's slope from both left and right sides to determine differentiability, highlighting the function's transition from a negative to a positive slope at x = -2 and from a decreasing to a flat line at x = 3.

Takeaways
  • 📚 The function f is defined for all real numbers and the discussion revolves around its differentiability.
  • 🔍 The presenter visualizes the derivative, f'(x), to understand where f(x) is not differentiable.
  • 📉 On the interval from x = -8 to x = -1.5, the slope of f(x) appears to be consistently negative 2, indicating a constant derivative.
  • 🔄 At x = -2, there's a significant change in the slope of f(x), transitioning from negative to positive, suggesting a non-differentiability point.
  • 🆚 The slope of the tangent line at x = -2 is estimated to be around 3.5, which is a stark contrast to the left-hand derivative.
  • 📊 The slope decreases gradually from x = -2 until x = 3, implying a continuous but non-constant rate of change.
  • 🔺 At x = 3, the slope of f(x) becomes zero, marking another point of non-differentiability.
  • 🚫 The points x = -2 and x = 3 are identified as the locations where the derivative 'jumps', indicating non-differentiability.
  • 🤔 The concept of a derivative as a limit of the secant line's slope is discussed, highlighting the inconsistency at the points of non-differentiability.
  • ↔️ The left and right-hand limits of the derivative do not match at x = -2 and x = 3, confirming the points of non-differentiability.
  • 📐 The script emphasizes the importance of the slope's behavior in determining the differentiability of a function.
Q & A

  • What is the main topic discussed in the transcript?

    -The main topic discussed in the transcript is the concept of differentiability of a function f at various points x, and the identification of points where the function is not differentiable.

  • How does the speaker attempt to visualize the derivative of the function f(x)?

    -The speaker attempts to visualize the derivative of the function f(x) by estimating the slope of the function over different intervals and drawing the derivative in a purple color.

  • What is the estimated slope of the function f(x) between x = -8 and x = 1/2?

    -The estimated slope of the function f(x) between x = -8 and x = 1/2 is consistently negative 2.

  • At what point does the speaker observe a change in the slope of the function f(x)?

    -The speaker observes a change in the slope of the function f(x) right as it crosses x = -2.

  • What is the estimated slope of the tangent line at x = -2?

    -The estimated slope of the tangent line at x = -2 is around 3 and 1/2.

  • How does the slope of the function change as x approaches 2?

    -As x approaches 2, the slope of the function continues to get lower at a constant rate.

  • At which point does the slope of the function become zero?

    -The slope of the function becomes zero right as x crosses 3.

  • What does the speaker conclude about the differentiability of f(x) at x = -2?

    -The speaker concludes that f(x) is not differentiable at x = -2 because the slope jumps and the limit of the secant line's slope from the left and right are not the same.

  • What is the reason for the function f(x) not being differentiable at x = 3?

    -The function f(x) is not differentiable at x = 3 because the slope approaches negative 1 from the left and is zero from the right, indicating different limits for the secant line's slope.

  • What is the general condition for a function to be differentiable at a point x?

    -A function is differentiable at a point x if the limit of the secant line's slope as it approaches the point from both the left and right sides exists and is the same.

  • How does the speaker describe the process of finding the slope of the tangent line?

    -The speaker describes the process of finding the slope of the tangent line as taking the limit of the slope of the secant line between that point and some other point on the curve.

Outlines
00:00
📉 Discontinuities in the Derivative of a Function

The script discusses the concept of differentiability of a function 'f' across the real numbers. It visually analyzes the function's derivative, denoted as 'f prime of x', to identify points of non-differentiability. The function's slope is observed to be consistently negative 2 between x = -8 and x = -2.5, with a derivative that would be a straight line with this constant slope. However, at x = -2, the slope transitions from negative to positive, and the derivative changes from a line to a curve, with an estimated slope of 3.5 at the point of transition. The slope decreases continuously until x = 2, where it becomes lower until x = 3, where the slope suddenly drops to 0. The function's derivative is seen to jump at x = -2 and x = 3, indicating points of non-differentiability. The left and right limits of the secant line's slope do not match at these points, suggesting that the function does not have a well-defined derivative at x = -2 and x = 3.

Mindmap
Keywords
💡Differentiable
Differentiable refers to a property of a function where it has a derivative at a certain point. In the context of the video, the function f(x) is discussed in terms of its differentiability at various points. The script describes how the function appears to have a constant slope of negative 2 in an interval, suggesting it is differentiable there, but then notes points where the slope jumps, indicating non-differentiability.
💡Derivative
The derivative of a function at a given point is the slope of the tangent line to the function at that point. In the script, the derivative is visualized as a separate function f'(x), which is used to analyze the differentiability of f(x). The derivative is key to understanding the function's behavior and where it may not be differentiable.
💡Slope
Slope is a measure of the steepness of a line, and in calculus, it is used to describe the rate of change of a function. The script discusses the slope of the function f(x) and its derivative f'(x), noting changes in slope that indicate points of non-differentiability, such as at x = -2 and x = 3.
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. In the script, the tangent line is used to estimate the derivative at a point, and changes in the slope of the tangent line are discussed as the function transitions from differentiable to non-differentiable at certain points.
💡Secant Line
A secant line is a straight line that intersects a curve at two or more points. The script mentions the secant line in the context of taking the limit of its slope as it approaches a point from the left and right to determine the derivative. The difference in these limits at certain points indicates non-differentiability.
💡Limit
In calculus, a limit is the value that a function or sequence approaches as the input approaches some value. The script discusses the limit of the secant line's slope as it approaches from the left and right sides of a point, which is crucial for determining the existence of a derivative and thus differentiability.
💡Constant Rate
A constant rate implies that the change in a function's value is consistent over an interval. The script describes how the slope of the function f(x) appears to decrease at a constant rate over an interval, suggesting a consistent rate of change that is indicative of differentiability.
💡Jump
A jump in the context of the script refers to a sudden change in the slope of the function, which indicates a point of non-differentiability. The function f(x) is said to have 'jumps' in its slope at x = -2 and x = 3, where the derivative does not exist.
💡Interval
An interval is a continuous range of values on the real number line. The script refers to intervals over which the function f(x) has a consistent slope, such as between x = -8 and x = 1/2, and intervals where the slope changes abruptly, indicating points of non-differentiability.
💡Estimate
An estimate is an approximate value or assessment of something. In the script, the speaker uses estimation to determine the slope of the tangent line at various points, which helps in identifying the points where the function is not differentiable.
💡Visualize
To visualize means to form a mental image or picture of something. The script describes the process of visualizing the derivative f'(x) to understand the function's behavior and identify points of non-differentiability, which is a key part of the analysis presented in the video.
Highlights

The function f is defined for all real numbers, and the task is to determine where it is not differentiable.

Visualization of f'(x) is attempted to understand where the function may not be differentiable.

The slope of f(x) appears to be consistently negative 2 between x = -8 and x = 1/2.

The derivative f'(x) is drawn as a constant negative 2 line in the given interval.

A change in slope occurs at x = -2, transitioning from negative to positive.

The slope of the tangent line at x = -2 is estimated to be around 3 and 1/2.

The slope decreases continuously until x = 2, suggesting a non-linear change in the derivative.

The slope of the function continues to decrease until x = 3, indicating a consistent rate of change.

At x = 3, the slope becomes 0, indicating a potential point of non-differentiability.

The function's slope jumps at certain points, suggesting undefined derivatives at these points.

f(x) is not differentiable at x = -2 due to the absence of a defined slope.

The left and right limits of the secant line slope differ at x = -2, indicating non-differentiability.

A similar situation occurs at x = 3, with the slope approaching negative 1 from the left and being 0 from the right.

The derivative jumps at x = 3, indicating another point of non-differentiability.

The concept of the limit of the secant line slope is used to determine differentiability.

The importance of matching left and right limits for the existence of a derivative is emphasized.

Transcripts

Browse More Related Video

Calculus - Lesson 9 | When does the Derivative Not Exist? | Don't Memorise

Differentiability at a point: algebraic (function isn't differentiable) | Khan Academy

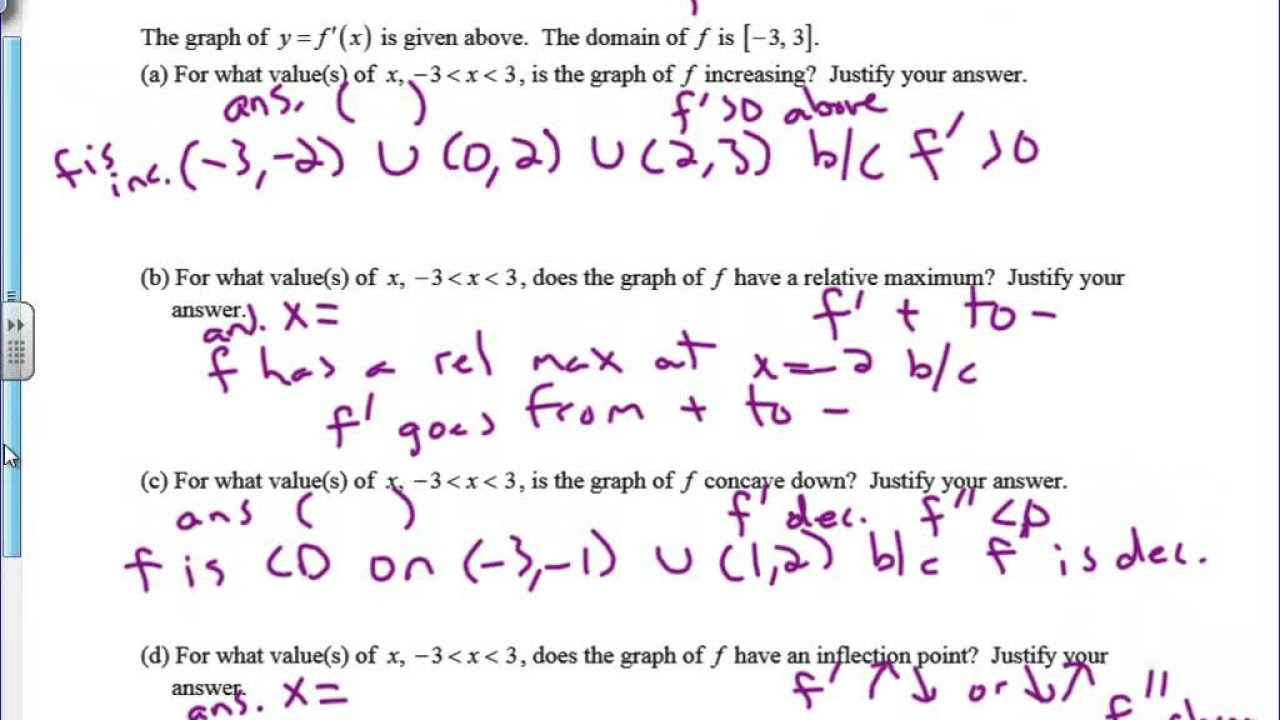
Graphs of f, f prime, and f double prime

Limits at infinity of quotients (Part 1) | Limits and continuity | AP Calculus AB | Khan Academy

Infinite Limit vs Limits at Infinity of a Composite Function

Sketching Derivatives From Parent Functions - f f' f'' Graphs - f(x), Calculus

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusDifferentiabilityMath AnalysisDerivativeSlopeTangent LineLimitsMathematicsGraph AnalysisCritical Points