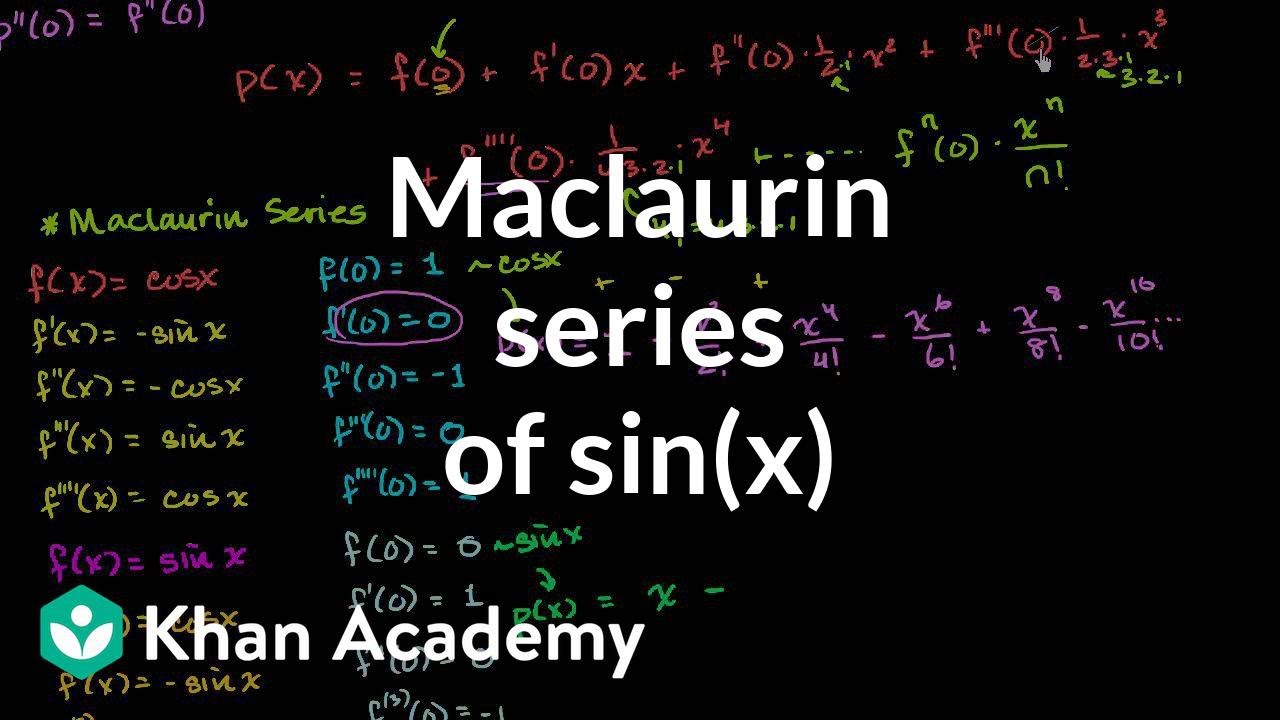Maclaurin series of cos(x) | Series | AP Calculus BC | Khan Academy

Khan Academy
17 May 201105:37
EducationalLearning
32 Likes 10 Comments

TLDRIn the video, the concept of Maclaurin series is introduced as a special case of Taylor series, focusing on approximating functions around x=0. The video demonstrates the derivation of the Maclaurin series for the cosine function, highlighting the pattern of alternating signs and the relationship between derivatives at zero. This series provides a polynomial approximation of the cosine function, showcasing the interconnectedness of mathematical concepts and their potential for profound insights.

Takeaways
  • ๐Ÿ“š The Maclaurin series is a special case of the Taylor series, used for approximating functions around x=0.
  • ๐ŸŒŸ The Taylor series offers more flexibility, allowing for an arbitrary point x to approximate the function.
  • ๐Ÿ” The focus of the discussion is on Maclaurin series due to its simplicity and its ability to lead to profound mathematical insights.
  • ๐Ÿ“ˆ The Maclaurin series of cos(x) is derived by taking successive derivatives and evaluating them at x=0.
  • ๐ŸŒ€ Derivatives of cos(x) cycle through -sin(x), cos(x), and their signs alternate in a predictable pattern.
  • ๐Ÿงฎ The pattern of derivatives at x=0 shows a repeating sequence of 1, 0, -1, 0, 1, and this repeats for higher order derivatives.
  • ๐Ÿ‘Œ The Maclaurin series for cos(x) is a polynomial approximation that captures the function's behavior near x=0.
  • ๐Ÿ”ข The series is structured with a sign pattern of (+1)^n, where n is the order of the term, and each term is divided by the corresponding factorial.
  • ๐Ÿ” The polynomial representation of cos(x) using its Maclaurin series is a simple expression of a complex trigonometric function.
  • ๐ŸŒ The interconnectedness of mathematical concepts is highlighted, suggesting deeper connections in future discussions.
  • ๐Ÿš€ The exploration of Maclaurin and Taylor series is just the beginning of understanding the vast network of mathematical relationships.
Q & A

  • What is the main difference between a Maclaurin series and a Taylor series?

    -A Maclaurin series is a special case of a Taylor series where the function is approximated around x equals 0, while a Taylor series can approximate the function around an arbitrary value of x.

  • Why is the Maclaurin series named after Maclaurin?

    -The Maclaurin series is named after the Scottish mathematician Colin Maclaurin, who made significant contributions to the development of the series in the 18th century.

  • What function was used in the script to demonstrate the Maclaurin series?

    -The cosine function, f(x) = cos(x), was used to illustrate the Maclaurin series in the script.

  • What is the first derivative of cos(x)?

    -The first derivative of cos(x) is -sin(x).

  • What pattern can be observed in the derivatives of the cosine function?

    -The pattern observed in the derivatives of the cosine function is a cycle of sine and cosine functions alternating in sign, starting with cosine, then sine, negative cosine, and so on.

  • What is the value of f(0) for the cosine function?

    -The value of f(0) for the cosine function is 1, as cos(0) equals 1ๆ— ่ฎบๆ˜ฏไปฅๅผงๅบฆ่ฟ˜ๆ˜ฏๅบฆๆ•ฐ่กก้‡ใ€‚

  • What is the second derivative of cos(x) evaluated at x equals 0?

    -The second derivative of cos(x) evaluated at x equals 0 is -1, as the first derivative is -sin(x) and its derivative is -cos(x).

  • How does the sign pattern of the coefficients in the Maclaurin series for cosine function work?

    -The sign pattern of the coefficients in the Maclaurin series for the cosine function alternates between positive and negative, starting with a positive sign for the constant term, followed by a negative sign for the x^2 term, then positive for the x^4 term, and so on.

  • What is the significance of factorial in the Maclaurin series?

    -The factorial is used in the denominator of each term in the Maclaurin series to indicate the number of possible permutations of the terms when expanding the series. It helps in determining the weight of each term as the power of x increases.

  • How does the Maclaurin series representation of cosine function show the interconnectedness of mathematics?

    -The Maclaurin series representation of the cosine function demonstrates the interconnectedness of mathematics by showing how a complex trigonometric function can be expressed as a simple polynomial. This reveals the underlying relationships and structures within mathematical concepts.

  • What does the script suggest about the future exploration of these mathematical concepts?

    -The script suggests that future exploration of these mathematical concepts will uncover even more profound connections and insights, indicating that the understanding of these series and their applications is just the beginning.

Outlines
00:00
๐Ÿ“š Introduction to Maclaurin Series and Derivatives

This paragraph introduces the concept of the Maclaurin series, explaining it as a special case of the Taylor series centered around x=0. It contrasts the Maclaurin series with the Taylor series, highlighting the flexibility of the latter in choosing an arbitrary point of approximation. The focus is on the Maclaurin series for its simplicity and its potential to lead to profound mathematical insights. The paragraph delves into the process of deriving the Maclaurin series for the cosine function, emphasizing the importance of calculating successive derivatives and evaluating them at x=0. A pattern in the signs and powers of the derivatives is observed, which forms the basis of the Maclaurin series for cos(x).

05:03
๐ŸŒ Pattern and Application of Maclaurin Series for Cosine Function

This paragraph continues the discussion on the Maclaurin series for the cosine function, detailing the pattern of alternating signs and the progression in the powers of x. It explains how the derivatives of cos(x) at x=0 contribute to the construction of the polynomial representation. The paragraph highlights the simplicity and elegance of the pattern, which reflects the interconnectedness of mathematical concepts. It concludes by suggesting that future discussions will reveal even deeper connections and implications of these mathematical series.

Mindmap
Keywords
๐Ÿ’กMaclaurin series
The Maclaurin series is a mathematical concept used to approximate functions around x equals zero. It is a special case of the Taylor series, which can approximate functions around any chosen point. In the context of the video, the Maclaurin series is used to derive a polynomial representation of the cosine function, demonstrating the interconnectedness of mathematical concepts.
๐Ÿ’กTaylor series
The Taylor series is a mathematical representation that allows functions to be expressed as infinite sums of terms calculated from the values of the function's derivatives at a single point. Unlike the Maclaurin series, which is specific to approximation around x=0, the Taylor series can be applied around any given point. The video mentions the Taylor series as a broader concept from which the Maclaurin series is derived.
๐Ÿ’กDerivatives
Derivatives in calculus represent the rate of change of a function with respect to its variable. They are fundamental to understanding the behavior of functions, such as slopes of curves or tangents at points. In the video, derivatives of the cosine function are calculated repeatedly to establish the pattern needed for the Maclaurin series approximation.
๐Ÿ’กApproximation
In mathematics, approximation involves creating a simpler model or expression that closely resembles a more complex real-world situation or mathematical function. The video focuses on using the Maclaurin series to approximate the cosine function around x=0, which simplifies the function into a polynomial form that can be more easily manipulated and understood.
๐Ÿ’กTrigonometric functions
Trigonometric functions, such as sine, cosine, and tangent, are mathematical functions that relate angles to real numbers. They are widely used in various fields, including mathematics, physics, and engineering, to model periodic phenomena. In the video, the cosine function is used as an example to illustrate the process of approximating trigonometric functions using the Maclaurin series.
๐Ÿ’กPolynomial
A polynomial is an algebraic expression consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. Polynomials are used to model and approximate various types of functions, as demonstrated in the video where the cosine function is approximated by a polynomial as part of its Maclaurin series.
๐Ÿ’กFactorials
A factorial is a mathematical function that multiplies a given non-negative integer by all positive integers less than it. It is denoted by the symbol '!' and is used in various mathematical contexts, including the calculation of coefficients in series expansions like the Maclaurin and Taylor series. In the video, factorials are used to determine the coefficients for the terms in the Maclaurin series of the cosine function.
๐Ÿ’กPatterns
In mathematics, a pattern refers to a regular sequence or arrangement of numbers, shapes, or expressions that repeats over time or across space. Identifying patterns is crucial for understanding and predicting mathematical behavior. The video highlights the alternating sign pattern in the Maclaurin series for the cosine function, which is a key aspect of its representation.
๐Ÿ’กConnectedness
Connectedness in mathematics refers to the relationships and interdependencies between different concepts, theorems, or structures. The video emphasizes the connectedness of math by showing how the Maclaurin series, a specific case of the Taylor series, can be used to approximate and understand complex functions like the cosine.
๐Ÿ’กInterchangeable
In the context of the video, 'interchangeable' refers to the idea that different mathematical representations or methods can be used to describe the same function or phenomenon. The Maclaurin series provides an alternative way to represent and understand the behavior of functions, such as the cosine, which can also be described using its original trigonometric form.
Highlights

Maclaurin series is a special case of Taylor series, focused on approximating functions around x=0.

Taylor series allows for an arbitrary x value to approximate the function, unlike Maclaurin series which is centered at x=0.

The Maclaurin series of cosine function is discussed, highlighting its simplicity and profound mathematical implications.

Derivatives of cos(x) are taken to understand the pattern and application of Maclaurin series.

The first derivative of cos(x) is -sin(x), showing the periodic nature of trigonometric functions.

Derivatives of sin(x) revert back to cos(x), illustrating the interconnection of trigonometric functions.

The pattern of derivatives at x=0 reveals a cycle of one, zero, negative one, zero, one, etc., which is crucial for Maclaurin series construction.

The Maclaurin representation of cos(x) is derived, showcasing a polynomial approximation of the trigonometric function.

The polynomial approximation includes terms like 1x^4/4!, demonstrating the pattern of even powers of x and alternating signs.

The alternating signs in the Maclaurin series for cos(x) indicate a positive, negative, positive, and so on pattern.

The Maclaurin series captures the essence of trigonometric functions, revealing the interconnectedness of mathematical concepts.

The discussion emphasizes the potential for more profound connections in mathematics beyond the scope of the current video.

The process of deriving the Maclaurin series for cos(x) is a step-by-step approach, emphasizing the methodical nature of mathematical analysis.

The video content serves as an educational tool, breaking down complex concepts like Maclaurin series into understandable components.

The practical application of Maclaurin series in approximating functions is highlighted, showing its relevance in mathematical analysis.

The video concludes with a teaser for future content, promising deeper insights into the interconnected nature of mathematical concepts.

Transcripts

Browse More Related Video

Maclaurin series of sin(x) | Series | AP Calculus BC | Khan Academy

Polynomial approximation of functions (part 4)

AP Calculus BC Lesson 10.14 Part 1

Taylor Polynomials

AP Calculus BC Lesson 10.11 Part 1

Taylor & Maclaurin polynomials intro (part 1) | Series | AP Calculus BC | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsMaclaurin SeriesTrigonometryCosine FunctionSeries ApproximationDerivativesPolynomial RepresentationEducationalInterconnected ConceptsMathematical Patterns