Separable Equations with Initial Values (Differential Equations 13)
TLDRThis video tutorial delves into solving initial value problems using the separation of variables technique for differential equations. The instructor emphasizes the strategic use of initial values, demonstrating how plugging them in at different stages can simplify the process. Through a series of examples, the video illustrates how to transform general solutions into specific particular solutions by carefully timing the insertion of initial conditions. The approach is tailored to the complexity of the equation, with guidance on when to plug in initial values to avoid unnecessary work or complications.
Takeaways
- 📚 The video focuses on solving differential equations using the technique of separation of variables, specifically initial value problems.
- 🔍 Importance is given to the strategic use of plugging in initial values to solve for the arbitrary constant, with the decision based on the complexity of the equation and the potential for simplifying the process.
- 🌟 The video provides examples to illustrate the process, emphasizing that sometimes it's beneficial to plug in initial values early, especially when dealing with exponential functions, and other times it's better to wait until a general solution is found.
- 📈 The process involves grouping variables (Y and dy on one side, X on the other), integrating both sides, and then solving for the arbitrary constant using the initial value condition.
- 🧮 Examples include a range of scenarios such as dealing with exponential functions (e^x), tangent functions (tan^-1), and other trigonometric functions.
- 🔢 The video script includes a detailed walkthrough of solving a differential equation where the solution involves both exponential and logarithmic functions, highlighting the steps and considerations in the process.
- 🛠️ The technique of separating variables is applied to various equations, demonstrating how to handle different types of differential equations and the importance of recognizing when to plug in initial values for the most efficient solution path.
- 📊 The video emphasizes the practical application of the technique, suggesting that the approach to plugging in initial values can significantly affect the ease and efficiency of solving the equations.
- 🎓 The script serves as a review and clarification of the separation of variables technique, aiming to reinforce understanding and provide strategies for tackling initial value problems in differential equations.
- 🌐 The video is part of a series that aims to teach and review integral calculus concepts, with a focus on the application of these concepts to real-world problems.
- 📖 The video concludes with a preview of the next topic, which involves applying the separation of variables technique to solve real-life problems involving differential equations.
Q & A
What is the main technique discussed in the video?
-The main technique discussed in the video is solving differential equations using the method of separation of variables.
What are separable equations?
-Separable equations are a type of differential equation that can be written in the form of dy/dx = g(x)h(y), where the variables can be separated and integrated separately.
Why is it sometimes beneficial to plug in the initial value early in the process?
-It is sometimes beneficial to plug in the initial value early in the process, especially when dealing with exponents, as it can simplify the equation and make solving for the arbitrary constant easier.
How does the presence of an exponential function affect when to plug in the initial value?
-If the exponential function can be simplified into a larger expression that includes the arbitrary constant, it's often easier to plug in the initial value after wrapping up the constant into a larger expression. Otherwise, it might be better to plug it in sooner.
What is the significance of the arbitrary constant in the general solution?
-The arbitrary constant represents the fact that there are infinitely many solutions to a differential equation, and it is determined by the initial conditions or boundary values of the specific problem.
How does the video demonstrate the process of solving a differential equation with an initial value?
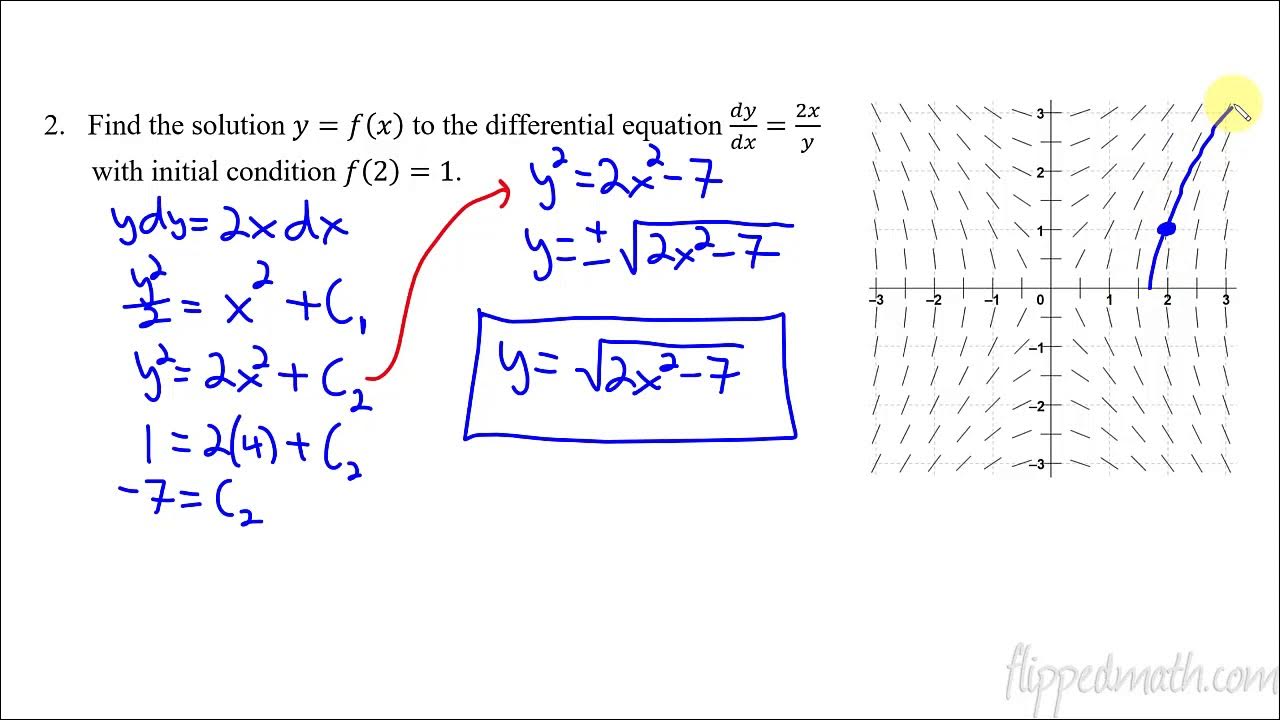
-The video demonstrates the process by first separating the variables, integrating both sides, and then solving for the arbitrary constant using the given initial value to find the particular solution that passes through the specified point.
What is the role of integration in solving separable equations?
-Integration is used to undo the effect of differentiation represented by the dy/dx term. By integrating both sides of the separated equation, we can find the function y(x) that satisfies the original differential equation.
How does the video address the challenge of solving differential equations with complex expressions?
-The video suggests strategies for dealing with complex expressions, such as factoring out common terms, using integration tables, and deciding when to plug in the initial value based on the form of the equation and the potential for simplifying the process.
What is the purpose of the examples provided in the video?
-The examples are provided to illustrate the process of solving separable equations with initial values, showing how to apply the technique in different scenarios and how to make decisions about when to plug in the initial value for the best results.
What advice does the video give for students solving these types of problems?
-The video advises students to be flexible in deciding when to plug in the initial value, based on whether it will simplify the process or make it more complicated. It also encourages students to practice and develop their problem-solving skills.
Outlines
📚 Introduction to Solving Differential Equations with Separation of Variables
The video begins with an introduction to solving differential equations using the separation of variables technique. The focus is on initial value problems and the importance of plugging in the initial value at the right time to simplify the process. The video aims to review integrals and the concept of converting a general solution with an arbitrary constant into a particular solution by using the initial value.
🔍 Exploring the Timing of Initial Value Plug-In
The paragraph discusses the strategic timing of when to plug in the initial value during the process of solving differential equations. It highlights that the decision depends on the nature of the problem and the potential for simplifying the equation. The speaker provides examples to illustrate the benefits of early or delayed plug-in of initial values, emphasizing the case-by-case approach.
📈 Working with Exponential Functions and Initial Values
This section delves into the specifics of handling exponential functions within differential equations. The speaker explains the process of integrating and dealing with exponential expressions, emphasizing the importance of grouping and simplifying before plugging in the initial value. The paragraph also covers the technique of combining smaller constants into a larger one to simplify the equation further.
🌟 Advanced Techniques for Solving Complex Differential Equations
The speaker presents advanced techniques for solving complex differential equations, including factoring and dealing with multiple terms. The paragraph emphasizes the importance of recognizing when to factor and when to separate variables for easier integration. The speaker also provides hints for tackling challenging problems and encourages viewers to practice on their own.
🧠 Factoring and Integrating in Separable Equations
This part of the video focuses on the importance of factoring in the process of solving separable equations. The speaker demonstrates how to group terms and factor out common factors to simplify the equation before integrating. The paragraph also discusses the integration of fractions and the separation of terms to make the process more manageable.
📊 Applying Initial Values to Complex Equations
The speaker continues to explore the application of initial values to complex equations, emphasizing the need to understand when to plug in the initial value to avoid unnecessary complications. The paragraph covers the handling of exponential functions and logarithms, and how to simplify the equation before integrating to find the particular solution.
🎓 Summary of Techniques for Solving Differential Equations
The video concludes with a summary of the techniques discussed for solving differential equations with separation of variables. The speaker reiterates the importance of understanding when to plug in the initial value and how to approach complex equations. The paragraph encourages viewers to apply these techniques to real-life problems and to continue practicing to master the process.
Mindmap
Keywords
💡Separation of Variables
💡Initial Value Problem
💡Arbitrary Constant
💡Integration
💡Exponential Functions
💡Logarithmic Functions
💡Tangent Function
💡Particular Solution
💡Factoring
💡Integration Tables
Highlights
The video focuses on solving differential equations using the technique of separation of variables.
Initial value problems are discussed, emphasizing the importance of plugging in the initial value to solve for the arbitrary constant.
The video provides a method for deciding when to plug in the initial value, depending on the problem's complexity and the presence of exponents.
An example is given where the differential equation is dy/dx = y * e^x, and the process of solving it is demonstrated.
The concept of grouping variables and separating them to simplify the equation is explained.
The video illustrates how to handle exponential functions in the context of differential equations.
A detailed walkthrough of solving a differential equation involving natural logarithms and exponentials is provided.
The video emphasizes the importance of understanding when to plug in the initial value to avoid unnecessary work.
An example with a differential equation involving the tangent function is solved, demonstrating the application of initial values.
The process of integrating both sides of a differential equation is explained, with a focus on simplifying the equation.
The video discusses the use of integration tables to simplify the process of solving differential equations.
A strategy for solving differential equations with complex expressions is presented, highlighting the benefits of factoring and simplifying before plugging in the initial value.
The video concludes with a summary of the key points, reinforcing the importance of knowing when to plug in the initial value based on the problem's specifics.
Three additional examples are provided for the viewer to practice solving differential equations with initial values.
The video encourages viewers to pause and attempt the examples on their own, promoting active learning.
Hints are given for the additional examples to guide viewers in their problem-solving process.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: