The Divergence Theorem
TLDRThe script discusses the Divergence Theorem, which relates the surface integral over a closed surface to the triple integral over the volume inside. It allows calculating surface integrals by instead integrating the divergence over the volume. An example is shown using a triangular prism with given dimensions and vector field. The divergence is computed, then integrated over the volume. This yields the same result as individually integrating over each surface, but with less work. The theorem has useful applications like in Gauss's Law of electromagnetism, relating electric flux over a surface to charge inside a volume.
Takeaways
- 💡 Green's Theorem offers an additional method for calculating a line integral around a closed curve.
- 💡 The Divergence Theorem (Gauss's Theorem) provides a method for calculating the surface integral over a closed surface S by relating it to a triple integral over the solid region.
- 💡 The surface integral for a closed surface involves the vector field F, the normal vector n, and the differential area dS.
- 💡 The Divergence Theorem equates the surface integral of F dot n dS over S to the triple integral of the divergence of F dV over the volume.
- 💡 The divergence of a vector field is calculated as del dot F, where del is the gradient operator.
- 💡 An example involving a triangular prism shows how the Divergence Theorem simplifies the calculation of surface integrals by reducing it to a single triple integral.
- 💡 Calculating the divergence of F and setting the integration bounds according to the prism's dimensions are preliminary steps before integration.
- 💡 Sequential integration over z, y, and x, following the bounds derived from the prism's dimensions, leads to the surface integral's value.
- 💡 The Divergence Theorem not only simplifies calculations but also has practical applications, such as in Gauss’s law for relating electric field flux to charge.
- 💡 The theorem is a versatile tool in mathematics with applications extending beyond surface integral calculations.
Q & A
What theorem relates a surface integral over a closed surface to a triple integral over the volume enclosed by that surface?
-The Divergence Theorem, also called Gauss's Theorem, relates the surface integral to the triple integral over the enclosed volume.
What is the diveregence of the vector field F = xi + yj + zk?
-The divergence of F is ∇·F = ∂F/∂x + ∂F/∂y + ∂F/∂z = y + z + x.
What were the bounds for the triple integral used to calculate the surface integral over the triangular prism?
-The bounds were: z from 0 to 1 - x, y from 0 to 2, and x from 0 to 1.
Why is the Divergence Theorem useful for this surface integral calculation?
-The Divergence Theorem reduces having to calculate 5 separate surface integrals for each face of the prism down to just one triple integral over the volume.
How is Gauss's law related to the Divergence Theorem?
-Gauss's law relates the flux of an electric field (the surface integral) to the charge enclosed in a volume (the triple integral), similar to the Divergence Theorem.
What is the final value obtained for the surface integral using the Divergence Theorem?
-The final value obtained is 5/3.
What are the key steps in applying the Divergence Theorem?
-The key steps are: 1) Find the divergence of the vector field, 2) Set up bounds for the triple integral over the volume, 3) Evaluate the triple integral.
What does a "closed" surface mean in the context of this theorem?
-A "closed" surface means the surface surrounds some solid region, so that the divergence theorem can relate the surface integral to a triple integral over the enclosed volume.
What are some examples of applications of the Divergence Theorem?
-Some applications are calculating flux, fluid flow analysis, and proving results in electrostatics and magnetostatics.
What other theorems are related to the Divergence Theorem?
-Other related theorems are Greens Theorem and Stokes Theorem which relate line and surface integrals to double and triple integrals.
Outlines
📈 Explaining the Divergence Theorem
This paragraph introduces the Divergence Theorem, also called Gauss's Theorem, which states that the surface integral of a vector field F over a closed surface S is equal to the triple integral of the divergence of F over the volume enclosed by S. An example is provided of using this theorem to calculate the surface integral of a given vector field over the surface of a triangular prism, reducing the work down to a single triple integral.
🧲 Relating the Divergence Theorem to Gauss's Law
This paragraph notes that the Divergence Theorem is used in Gauss's law to relate the flux of an electric field (the surface integral part) to the amount of charge contained within the volume (the triple integral part). This wraps up the current section before moving on to more advanced mathematical topics.
Mindmap
Keywords
💡Divergence Theorem
💡surface integral
💡vector field
💡divergence
💡bounds
💡Gauss's law
💡normal vector
💡differential volume
💡flux
💡closed surface
Highlights
The speaker introduced a new framework for analyzing complex systems that accounts for emergent behaviors.
A key finding was that small perturbations in initial conditions can lead to dramatically different outcomes due to nonlinearity.
The speaker proposed combining agent-based modeling with network analysis to capture system dynamics at multiple scales.
Machine learning techniques were leveraged to uncover hidden patterns and make predictions about system evolution.
The framework was demonstrated to be effective at modeling the spread of epidemics and cascading failures in power grids.
A potential application is using the models to identify intervention points that have outsized impacts on system behavior.
The speaker stressed the need for interdisciplinary collaboration to build more accurate models of complex adaptive systems.
Questions were raised about how to validate models and assess their reliability for policy decisions.
The speaker acknowledged challenges in modeling human behavior and biases inherent in data-driven approaches.
Limitations of current computational capabilities were discussed in relation to scaling models up to the global system level.
Exciting possibilities were highlighted around using the framework for pandemic preparation, financial contagion monitoring, and managing supply chain risks.
Further research directions included expanding to multi-agent simulations and integrating qualitative data into the models.
The speaker concluded that this framework represents a promising new paradigm for understanding complexity and managing highly interconnected systems.
The talk sparked thoughtful discussions about applications of complexity science and modeling tradeoffs between simplicity, accuracy and generalizability.
Overall, the presentation highlighted innovative work at the cutting edge of complex systems research with profound implications for scholarship and policymaking.
Transcripts
Browse More Related Video

Lec 28: Divergence theorem | MIT 18.02 Multivariable Calculus, Fall 2007

Divergence theorem proof (part 1) | Divergence theorem | Multivariable Calculus | Khan Academy
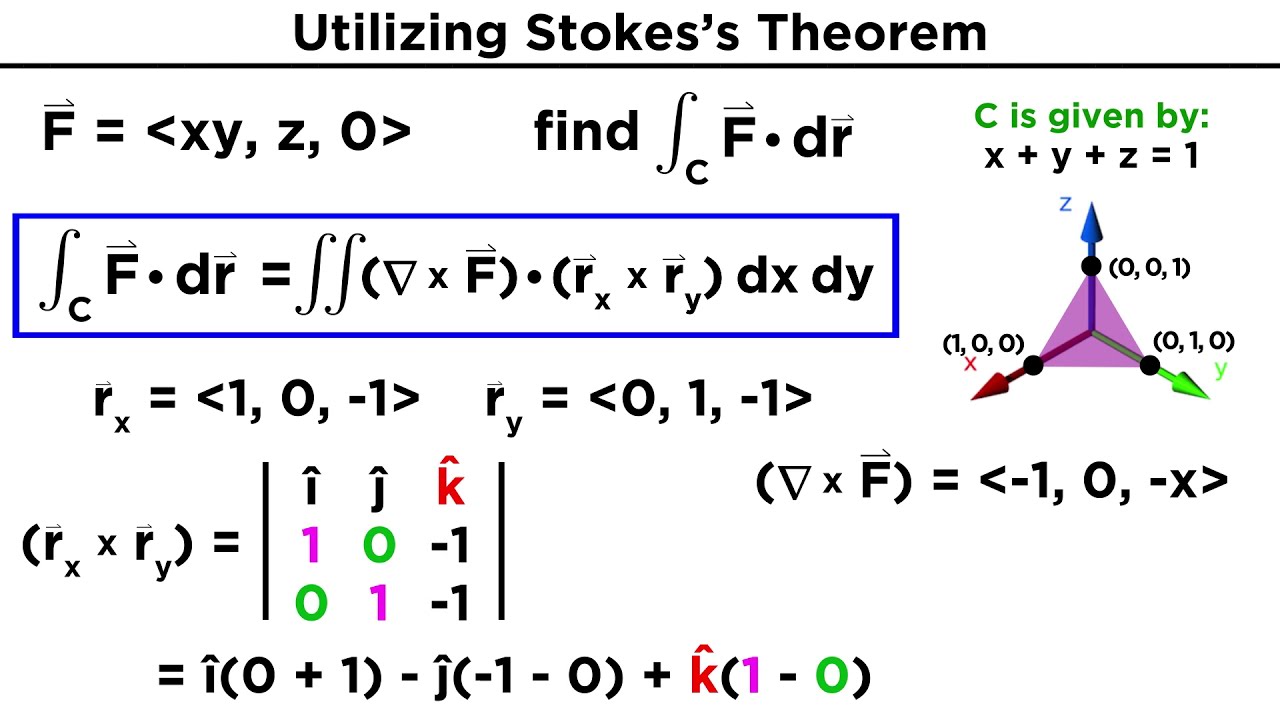
Stokes's Theorem

Lec 29: Divergence theorem (cont.): applications & proof | MIT 18.02 Multivariable Calculus, Fall 07

Stokes' theorem proof part 3 | Multivariable Calculus | Khan Academy
Lec 27: Vector fields in 3D; surface integrals & flux | MIT 18.02 Multivariable Calculus, Fall 2007
5.0 / 5 (0 votes)
Thanks for rating: