Angular Momentum
TLDRIn this insightful video, Mr. Andersen explores the concept of angular momentum, emphasizing its significance in physics, particularly for rotating objects. He explains the vector nature of angular momentum and its relationship with mass, velocity, and the radius from the center of rotation. The video delves into calculating angular momentum for both point objects and extended objects, using simple formulas and examples. It also discusses the conservation of angular momentum, the role of torque, and how changes in torque over time affect angular momentum, illustrated with engaging examples and animations.
Takeaways
- 🌀 Angular momentum is a property of rotating or spinning objects, similar to linear momentum for objects in translational motion.
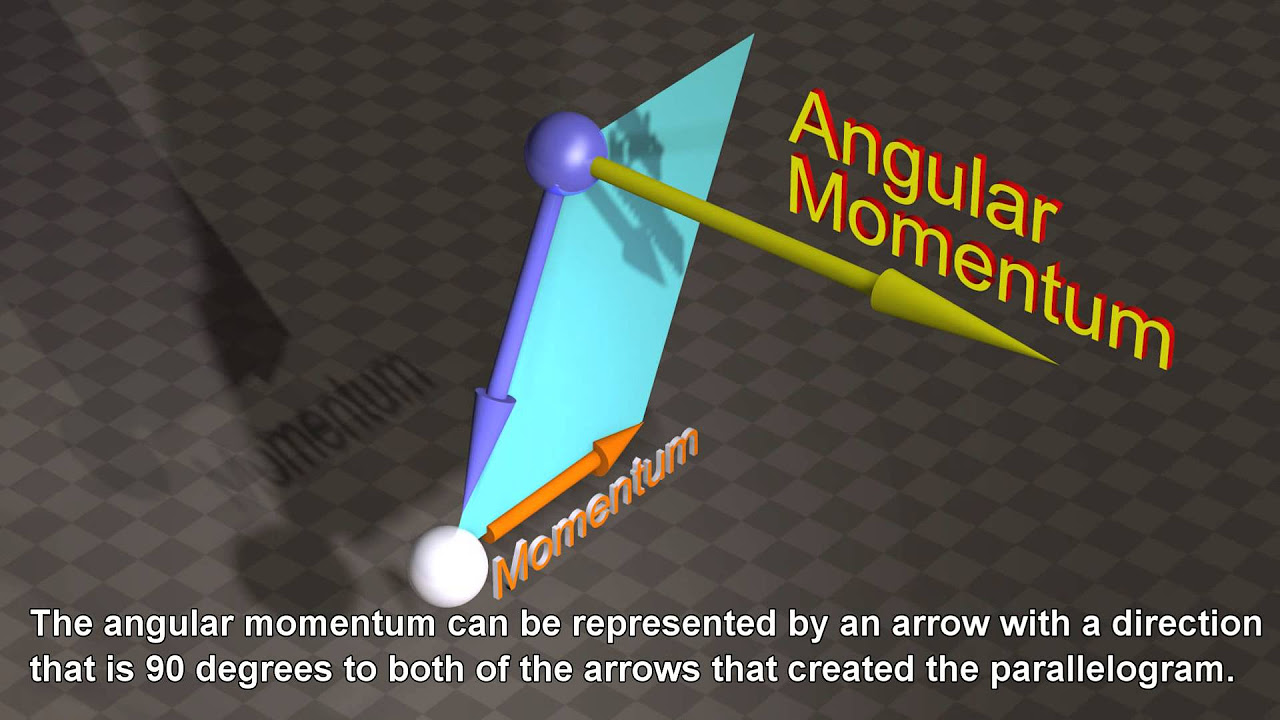
- 🔄 Angular momentum is a vector quantity, which means it has both magnitude and direction, and can be represented as the product of mass, velocity, and the radius from the center of rotation.
- 🚲 The concept is illustrated with the example of a bicycle staying upright due to the angular momentum of its wheels resisting changes, showing how it aids in balance.
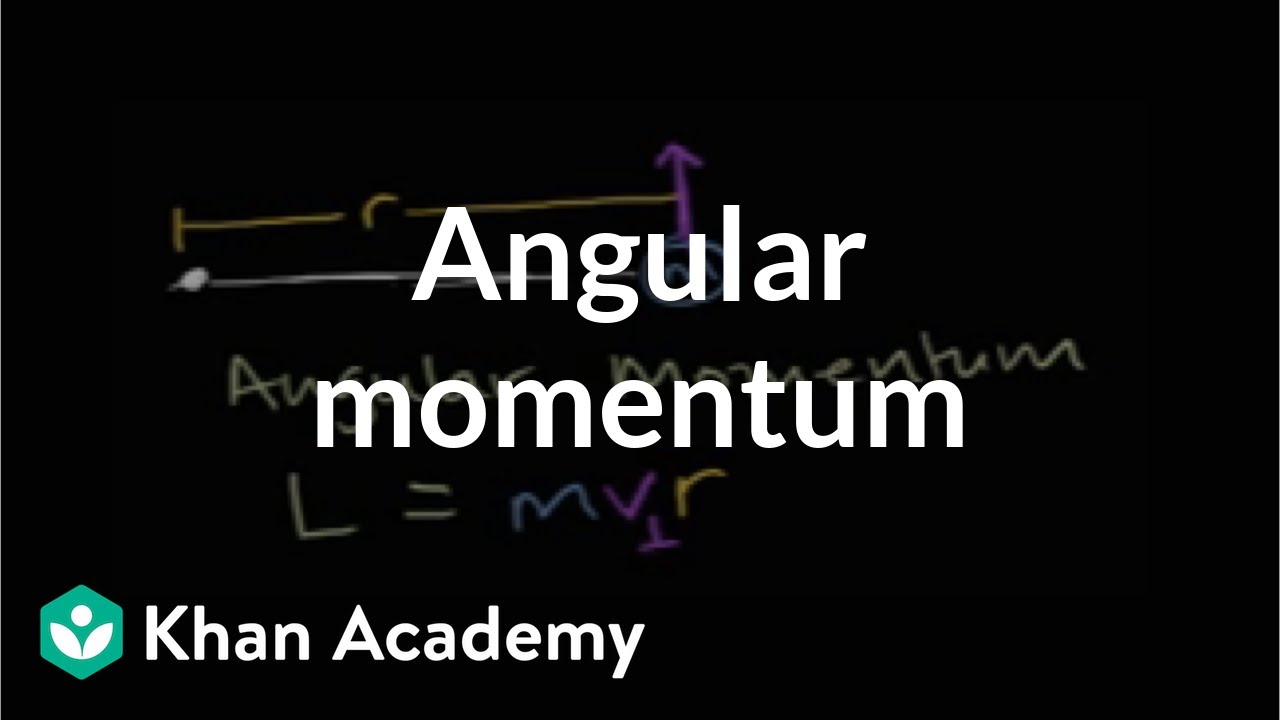
- 📐 The formula for angular momentum of a point object is L = r * (mass * velocity), where r is the distance from the center of rotation to the object.
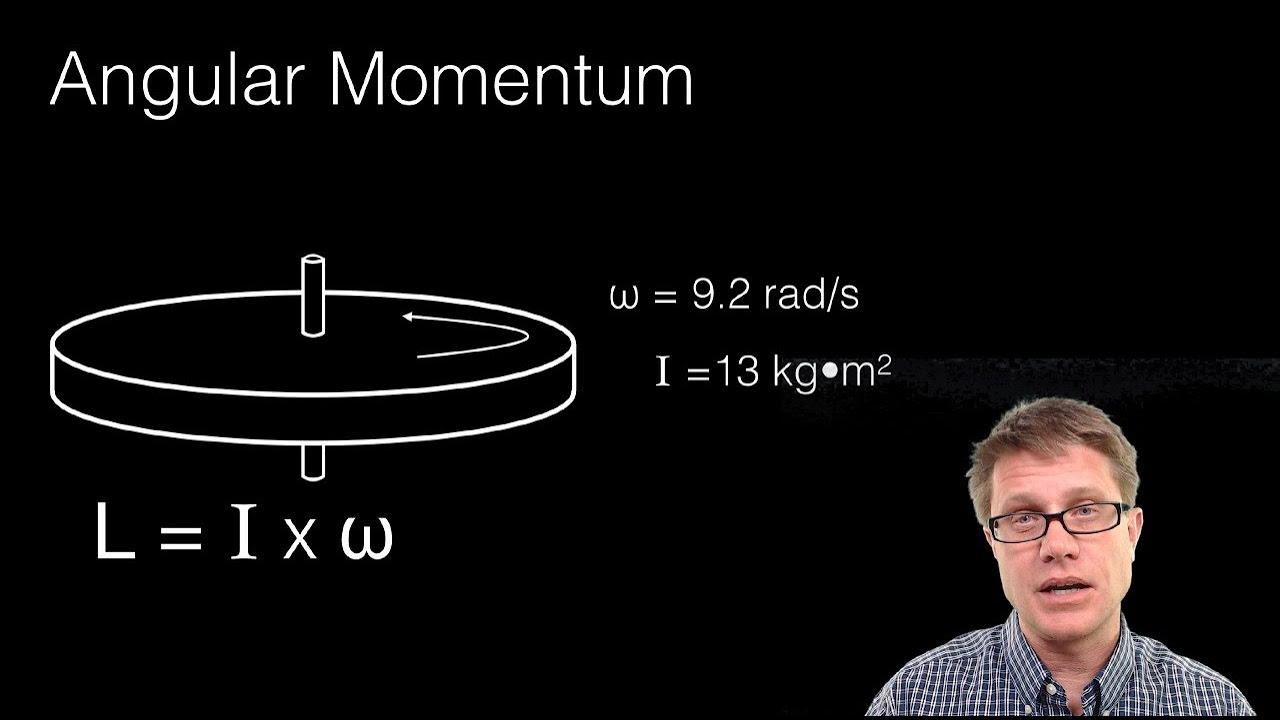
- 📐 For an extended object, angular momentum is calculated as L = I * ω, where I is the rotational inertia and ω is the angular velocity.
- 🧭 The direction of angular momentum is determined using the right-hand rule, with the thumb pointing in the direction of rotation and the fingers indicating the direction of angular momentum.
- 🔄 Conservation of angular momentum is a key principle, meaning that the total angular momentum of an isolated system remains constant unless acted upon by an external torque.
- 💥 Changes in angular momentum are related to the impulse (force applied over time), with the net torque times the change in time giving the change in angular momentum.
- 📊 In a physics lab, angular momentum can be measured and studied using devices like turntables and photo gates to determine rotational inertia and angular velocity.
- 🤹♂️ Collisions between rotating objects result in the transfer of angular momentum, with the total angular momentum of the system being conserved before and after the collision.
- 🚀 Practical applications of angular momentum include modeling systems with rotating components, such as turntables with rockets or wheels, and understanding how forces and torques affect their rotation.
Q & A
What is the main difference between momentum and angular momentum?
-Momentum is the product of an object's mass and its velocity, while angular momentum is related to rotating or spinning objects and involves the object's moment of inertia and angular velocity.
How does angular momentum help a bicycle remain upright?
-As a bicycle moves, its wheels gain angular momentum which resists changes, making it easier for the rider to maintain balance and keep the bicycle upright.
What is the vector nature of angular momentum?
-Angular momentum is a vector quantity, which means it has both magnitude and direction, and it acts in a specific direction determined by the right-hand rule.
How is the angular momentum of a point object calculated?
-The angular momentum of a point object is calculated using the formula L = r * p, where L is the angular momentum, r is the radius (distance from the center to the object), and p is the linear momentum (mass times velocity).
What is the role of rotational inertia in calculating the angular momentum of an extended object?
-Rotational inertia (I) is a measure of how difficult it is to change the angular momentum of an object. For an extended object, the angular momentum is calculated as L = I * ω, where ω is the angular velocity.
How does the right-hand rule determine the direction of angular momentum?
-The right-hand rule states that if you curl the fingers of your right hand in the direction of the rotation, your thumb will point in the direction of the angular momentum.
What is the relationship between impulse and change in angular momentum?
-Just as impulse (force applied over time) equals the change in linear momentum, the change in angular momentum is equal to the torque (rotational force) times the change in time.
How can angular momentum be transferred between objects?
-Angular momentum can be transferred between objects when they collide or interact in a way that conserves the total angular momentum, causing a redistribution of angular velocities.
What is a practical way to measure angular momentum in a physics lab?
-A turntable can be used to measure angular momentum by applying a known torque and measuring the resulting angular velocity, or by using a photo gate to measure the speed of rotation.
How does a change in torque affect the angular momentum of an object?
-A change in torque over a given period of time will change the angular momentum of an object, similar to how impulse affects linear momentum in translational motion.
Can you explain the concept of conservation of angular momentum in collisions?
-In collisions, the total angular momentum is conserved. This means that the sum of the angular momenta of the colliding objects before the collision is equal to the sum after the collision, assuming no external torques act on the system.
Outlines
🚴 Introduction to Angular Momentum
This paragraph introduces the concept of angular momentum, contrasting it with linear momentum. Angular momentum is associated with rotating or spinning objects, such as a bicycle wheel, which resists changes and helps maintain balance. It is a vector quantity with a specific direction, which can be determined using the right-hand rule. The paragraph explains how to calculate angular momentum for a point object (an object accelerating around a point) using the formula L = r * (mass * velocity). It also touches on the conservation of angular momentum and its application in future videos, mentioning how angular momentum can be transferred as the angle of force application changes.
🔧 Calculating and Measuring Angular Momentum
This paragraph delves into the calculation of angular momentum for both point and extended objects. For point objects, the formula involves the radial distance (r) and linear momentum. The paragraph provides a worked example with given values for mass, velocity, and radius, resulting in a vector value for angular momentum. For extended objects, the angular momentum is calculated using the rotational inertia (I) and angular velocity. The direction of the angular momentum vector is again determined using the right-hand rule. Practical methods for measuring angular momentum in a physics lab are discussed, such as using a turntable and photo gate to measure rotational inertia and velocity. The concept of angular momentum conservation during collisions is also introduced.
Mindmap
Keywords
💡Angular Momentum
💡Momentum
💡Vector
💡Rotational Inertia
💡Angular Velocity
💡Torque
💡Conservation of Angular Momentum
💡Right Hand Rule
💡Impulse
💡Collisions
💡Turntable
Highlights
Angular momentum is a concept in physics that describes the rotational motion of objects.
Momentum is the product of an object's mass and its velocity.
Angular momentum helps in understanding why a bicycle remains upright when in motion.
Angular momentum is a vector quantity, meaning it has both magnitude and direction.
The formula for angular momentum of a point object is L = r * p, where r is the radius and p is the linear momentum.
For an extended object, angular momentum is calculated as L = I * ω, where I is the rotational inertia and ω is the angular velocity.
The direction of angular momentum is determined using the right-hand rule.
A gyroscope demonstrates the conservation of angular momentum, maintaining its spinning plane despite external forces like gravity.
Angular momentum can be transferred from one object to another, as seen in collisions.
The conservation of angular momentum is a fundamental principle in physics, applicable in various scenarios including celestial bodies and spinning objects.
The change in angular momentum is equal to the torque applied over a period of time.
In a physics lab, a turntable can be used to demonstrate and measure angular momentum.
Photo gates can be attached to a turntable to measure angular velocity in radians per second.
Collisions between objects can be analyzed using the conservation of angular momentum principle.
The application of torque can change the angular momentum of an object, as seen when forces are applied perpendicular to the lever arm.
The concept of angular momentum is crucial in AP Physics and is applicable to both point objects and extended objects.
Understanding torque and its impact on angular momentum is essential for predicting the behavior of objects in motion.
The video provides practical examples and demonstrations to help grasp the concept of angular momentum and its applications.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: