Angular Momentum of a System
TLDRIn this enlightening video, Mr. Andersen explores the concept of angular momentum in physics, using the spinning top and Earth's precession as examples. He explains how angular momentum is calculated for both point objects and extended objects, and how it's direction is determined using the right-hand rule. The video delves into the stability of a helicopter due to its tail rotor and the dynamics of a gyroscope, demonstrating the principles with engaging experiments that challenge intuition and reinforce the importance of understanding angular momentum in a system.
Takeaways
- 🌀 Angular momentum is a property of a rotating system and can be determined by the right-hand rule.
- 🎯 When a top spins, it gains angular momentum and may exhibit precession due to external forces like gravity.
- 🌍 The Earth's rotation causes a slow wobble, or precession, over a 26,000-year cycle due to gravitational forces from the sun, moon, and other planets.
- 🚁 A helicopter's main rotor generates angular momentum, and a tail rotor is used to counteract torque and maintain stability.
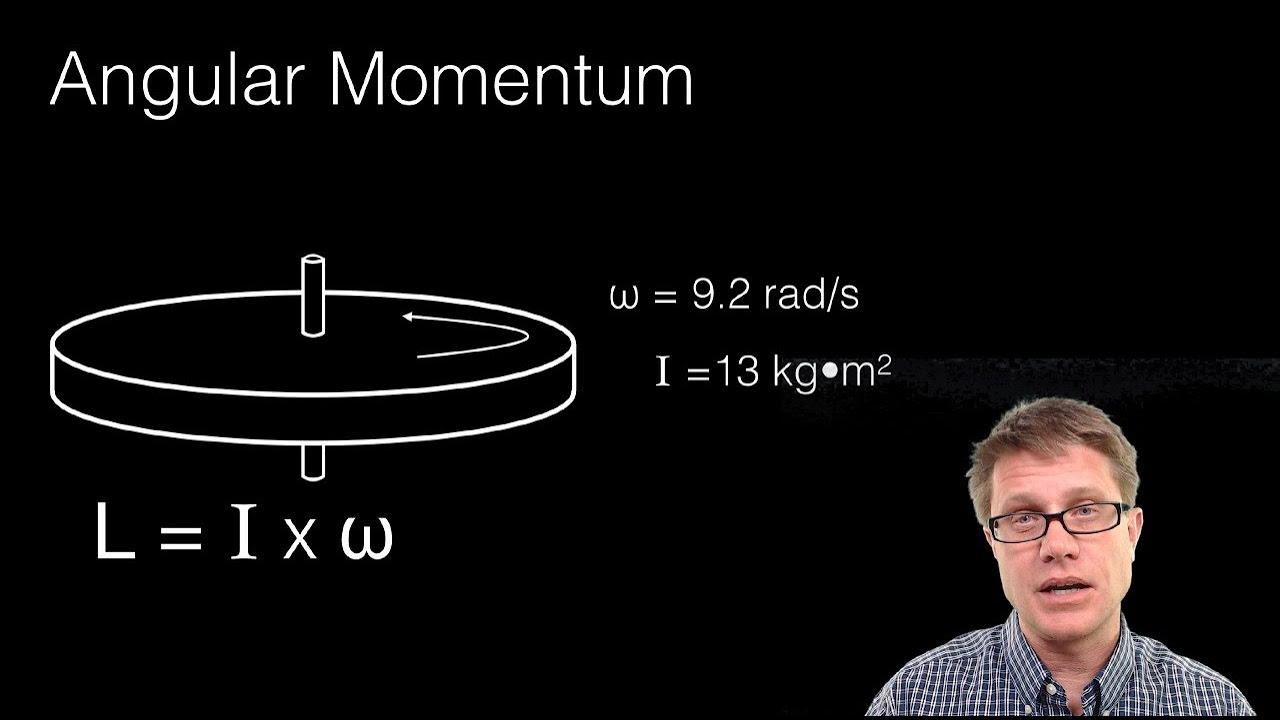
- 🔄 For a point object, angular momentum is calculated by multiplying the radius (distance from the center) by the object's momentum (mass times velocity).
- 🌗 The direction of angular momentum for an extended object is found by multiplying its rotational inertia (mass distribution) by its angular velocity.
- 🔧 The right-hand rule helps determine the direction of angular momentum: curl fingers in the direction of rotation, and the thumb points in the direction of momentum.
- 💫 A gyroscope is a useful tool for studying angular momentum as it demonstrates how external forces affect its direction and stability.
- 🤔 The behavior of a gyroscope under force can seem counterintuitive unless the right-hand rule and the system's total angular momentum are considered.
- 📈 The angular momentum of a system is the sum of the angular momenta of all objects within that system, which can include both point objects and extended objects.
Q & A
What is the main topic of the video?
-The main topic of the video is the concept of angular momentum in a system, particularly focusing on rotation and precession.
How can you determine the direction of angular momentum using the right-hand rule?
-Using the right-hand rule, you can determine the direction of angular momentum by pointing your finger in the direction of rotation, and your thumb will point in the direction of the angular momentum.
What is the phenomenon observed in a spinning top as it begins to wobble?
-The phenomenon observed in a spinning top as it begins to wobble is called precession in physics.
How does the Earth experience precession?
-The Earth experiences precession as it rotates, slowly wobbling due to the gravitational forces exerted by other celestial bodies like the Sun, the Moon, and other planets.
How is angular momentum calculated for a point object?
-For a point object, angular momentum is calculated by multiplying the radius (distance from the center to the object) by the momentum of the object (mass times its linear velocity).
What is the role of a tail rotor in a helicopter?
-The tail rotor in a helicopter applies additional angular momentum to counteract the torque created by the main rotor, helping to keep the helicopter stable.
How does the angular momentum of an extended object, like a spinning gyroscope, differ from that of a point object?
-For an extended object, angular momentum is calculated by multiplying its rotational inertia (a product of its mass distribution and its moment of inertia) by its angular velocity.
What is the significance of a gyroscope in studying angular momentum?
-A gyroscope is significant in studying angular momentum because it demonstrates how the angular momentum of a system is determined by the combined angular momentum of all objects within the system, and how external forces can affect its direction.
How does the right-hand rule help in predicting the behavior of a gyroscope when a force is applied?
-The right-hand rule helps predict the behavior of a gyroscope by determining the direction of the resulting angular momentum when a force is applied, which in turn indicates the direction the gyroscope will move.
What happens to the direction of angular momentum when multiple forces are applied to a system?
-When multiple forces are applied to a system, the overall angular momentum is the vector sum of the angular momenta of all the individual objects within the system.
Outlines
🌀 Introduction to Angular Momentum and Precession
This paragraph introduces the concept of angular momentum in a system, specifically focusing on a spinning top. It explains that as the top spins, it gains angular momentum, the direction of which can be determined using the right-hand rule. The paragraph then discusses the phenomenon of precession, exemplified by the wobbling of a spinning top, which eventually stops due to the interplay of different angular momenta within the system. The example of Earth's precession over a 26,000-year cycle is used to illustrate this concept further, highlighting how celestial bodies like the Sun and Moon contribute to this effect. The paragraph emphasizes that the angular momentum of a system is the sum of the angular momenta of all objects within it, and provides the formula for calculating angular momentum for both point objects and extended objects, such as a helicopter blade.
🚁 Understanding Angular Momentum with Gyroscopes
This paragraph delves into the practical application of angular momentum principles using the example of a gyroscope. It explains how a gyroscope, with its significant rotational inertia, can be used to study the effects of adding or changing angular momentum within a system. The paragraph describes an experiment where forces are applied to a spinning gyroscope, resulting in non-intuitive movements that can be predicted using the right-hand rule. The gyroscope serves as a model to analyze how multiple objects within a system contribute to its overall angular momentum, and the paragraph encourages the viewer to apply the right-hand rule to predict the gyroscope's behavior, reinforcing the concept of system-wide angular momentum.
Mindmap
Keywords
💡Angular Momentum
💡Precession
💡Right Hand Rule
💡Torque
💡Rotational Inertia
💡Helicopter
💡Gyroscope
💡Conservation of Angular Momentum
💡System
💡Force
Highlights
Angular momentum is a key concept in physics, particularly in the study of rotational motion.
When a top starts to rotate, it gains angular momentum, and its direction can be determined using the right-hand rule.
As a spinning top picks up speed, it begins to wobble, a phenomenon known as precession in physics.
The Earth also experiences precession, which takes approximately 26,000 years to complete one cycle, due to gravitational forces from the sun, moon, and other planets.
The angular momentum of a system is the sum of the angular momenta of all the objects within that system.
For a point object, angular momentum can be calculated by multiplying the radius and the momentum of the object.
The momentum of an object is its mass times its linear velocity.
For an extended object, angular momentum is found by multiplying its rotational inertia by its angular velocity.
Helicopters use a tail rotor to counteract the torque produced by the main rotor and maintain stability.
The direction of angular momentum for an extended object can be determined by using the right-hand rule and considering the object's rotation.
Gyroscopes are excellent tools for studying angular momentum and its effects on systems.
MIT has a video demonstrating the principles of angular momentum using a gyroscope, where applying a force results in a non-intuitive change in direction.
The right-hand rule is crucial for predicting the direction of angular momentum in a system.
Understanding angular momentum is essential for grasping the behavior of rotating and precessing objects.
The video provides practical examples and demonstrations to help viewers understand the concept of angular momentum.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: