Momentum and Angular Momentum of the Universe
TLDRThe video script explores fundamental physical principles of the universe, focusing on the conservation of energy, electric charge, momentum, and angular momentum. It explains how the total momentum of a system remains zero when two particles repel or attract each other, with the heavier particle moving slower to maintain equal momentum in opposite directions. The script also delves into the concept of angular momentum, which is conserved around any chosen point and can be transferred between objects. The parallelogram formed by the distance and momentum vectors represents the strength of angular momentum. As objects rotate, their angular momentum vectors point in opposite directions, potentially canceling each other out. The video emphasizes the importance of these principles in understanding the dynamics of particle interactions and the behavior of rotating objects. It concludes by inviting viewers to learn more about torque, levers, and the universal law of rotation through additional content on the channel.
Takeaways
- 🌌 **Conservation of Energy**: The total amount of energy in the Universe remains constant.
- ⚡ **Conservation of Electric Charge**: The total electric charge in the Universe is always constant.
- 🚀 **Conservation of Momentum**: The total momentum in the Universe is conserved and remains zero unless acted upon by an external force.
- 🔄 **Velocity and Momentum**: An object's momentum is the product of its mass and velocity.
- 🤲 **Opposite Momentums**: When two particles repel, they develop equal and opposite momentums, which cancel each other out.
- 🏃 **Mass and Velocity Relationship**: A particle with a larger mass will move slower but can have the same momentum as a lighter particle if their velocities differ appropriately.
- 💥 **Momentum Transfer**: During a collision, momentum can be transferred from one object to another, conserving the total momentum.
- 📏 **Multidimensional Momentum**: Objects can possess momentum in multiple directions, with the total momentum in each dimension being conserved separately.
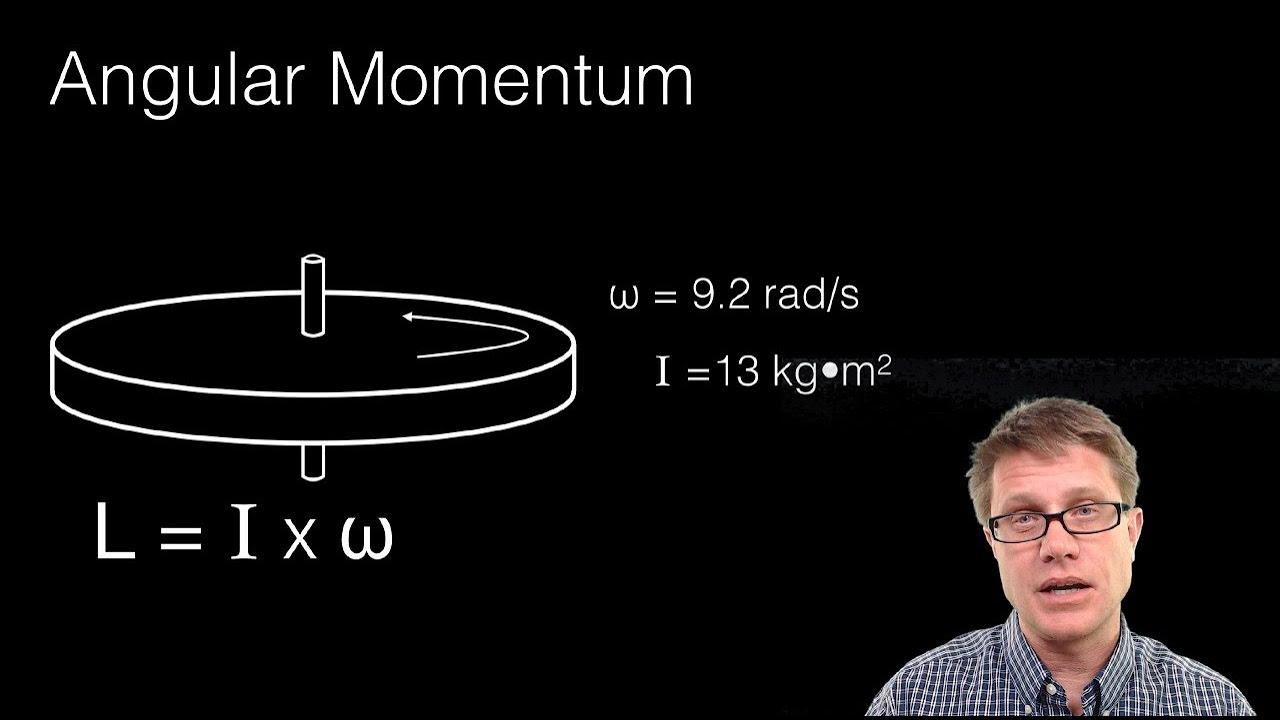
- 📐 **Conservation of Angular Momentum**: The angular momentum around a point is conserved, represented by the area of a parallelogram formed by the position and momentum vectors.
- 🔀 **Direction of Angular Momentum**: The direction of angular momentum is perpendicular to the plane formed by the position and momentum vectors.
- ↔️ **Opposite Rotations**: Particles rotating around a point in opposite directions have angular momenta in opposite directions, which can cancel each other out.
- 🔍 **Constant Angular Momentum**: For any chosen point in the Universe, the total angular momentum around that point remains constant over time.
- 🔵 **Attraction and Angular Momentum**: When particles attract each other, their velocities must increase to maintain constant angular momentum.
- 🔴 **Repulsion and Angular Momentum**: Conversely, when particles repel, their velocities decrease to keep the angular momentum constant.
- 🔵➡️🔴 **Conservation of Linear Momentum**: Linear momentum is also conserved, starting and ending with a total linear momentum of zero.
Q & A
What is a constant quantity in the Universe according to the script?
-The total amount of energy, electric charge, momentum, and angular momentum are constant quantities in the Universe.
How is an object's momentum defined in the script?
-An object's momentum is defined as the product of its mass and velocity.
What happens to the total momentum when two particles repel each other?
-When two particles repel each other, they develop momentum in opposite directions, which cancels each other out, resulting in a total momentum of zero.
How does the mass of a particle affect its velocity and momentum during a repulsion?
-If a particle has a larger mass, it will move slower and have a smaller velocity. However, because momentum is mass times velocity, the momentums of the two objects can still be the same strength but in opposite directions, thus canceling each other out.
What is the conservation of momentum principle as described in the script?
-The conservation of momentum principle states that when one object collides with another, the momentum of one object can be transferred to another, but the total momentum remains the same as before the collision.
What is angular momentum and how is it related to momentum?
-Angular momentum is the rotational equivalent of linear momentum and is represented by the area of a parallelogram formed by the position vector and the momentum vector. It is conserved around a point and can be transferred between objects.
How does the script describe the conservation of angular momentum in a system of two repelling particles?
-The script describes that when two particles repel each other, they develop angular momentum around a point in opposite directions, which cancel each other out, maintaining the total angular momentum at zero.
What happens to the velocity and rotation of particles when they repel each other, according to the script?
-If two particles repel each other, the width of the rectangles representing their angular momentum increases, which means the length (and thus the velocity) must decrease to keep the area (angular momentum) constant, causing the rotation to slow down.
How does attraction between particles affect their velocity and angular momentum?
-If two particles attract each other, their velocity must increase to keep the area of the rectangles (representing angular momentum) constant, and hence maintain the total angular momentum.
What is the linear momentum conservation principle mentioned in the script?
-The linear momentum conservation principle mentioned in the script states that the total linear momentum of a system remains constant if it was initially zero, even after interactions or collisions within the system.
What additional information is available for understanding these concepts?
-More detailed information about momentum, angular momentum, and related concepts is available in a video titled 'Torque, Levers, and the Universal Law of Rotation' and other videos on the same channel.
How can one stay updated with new content from the channel discussing these topics?
-To stay updated with new content from the channel, one can subscribe for notifications on when new videos are released.
Outlines
🌌 Conservation of Momentum and Energy
The first paragraph discusses the fundamental principle of conservation in the Universe, particularly focusing on energy, electric charge, momentum, and angular momentum. It explains how the total momentum of two repelling particles remains zero due to their equal and opposite momentums. The concept of velocity and its relation to mass and momentum is also introduced. Furthermore, the paragraph touches on the transfer of momentum during collisions and the conservation of momentum in multiple directions, emphasizing that the Universe maintains a constant total momentum in each dimension.
🔄 Angular Momentum and its Conservation
The second paragraph delves into angular momentum, which is the rotational equivalent of linear momentum. It describes how the strength of angular momentum is represented by the area of a parallelogram formed by the position and momentum vectors. The paragraph explains that angular momentum can be transferred between objects and is depicted by an arrow perpendicular to the plane of the parallelogram. It also covers how angular momentum behaves when particles repel or attract each other, affecting the velocity and rotation of the particles to maintain a constant total angular momentum. The paragraph concludes by reiterating the conservation of linear momentum, aligning with the initial state of zero total linear momentum.
📚 Further Exploration of Rotational Dynamics
The third and final paragraph serves as a call to action, directing viewers to seek more detailed information on the topic by watching a specific video titled 'Torque, Levers, and the Universal Law of Rotation' and other related content on the channel. It encourages viewers to subscribe for updates on new video releases, indicating a broader educational resource for those interested in understanding the intricacies of torque and rotational dynamics.
Mindmap
Keywords
💡Conservation of Energy
💡Electric Charge
💡Momentum
💡Angular Momentum
💡Velocity
💡Collision
💡Mass
💡Parallelogram Law
💡Rotation
💡Linear Momentum
💡Attraction and Repulsion
Highlights
The total amount of energy, electric charge, momentum, and angular momentum in the universe are always constant.
An object's momentum is its mass multiplied by its velocity.
When two particles repel, they develop equal and opposite momenta, keeping the total momentum zero.
If one particle has a larger mass, it moves slower but the momenta of the two objects still cancel out.
Momentum can be transferred between objects during a collision, but the total momentum is conserved.
Objects can have momentum in multiple directions, with the total momentum in each dimension being conserved.
Angular momentum is the strength of an object's rotation around a point, represented by the area of a parallelogram formed by the position and momentum vectors.
Angular momentum can be transferred between objects and is conserved in the universe.
When two particles repel, their angular momenta around a point are opposite, canceling out to a total of zero.
The total angular momentum around any point in the universe remains constant for all time.
If two particles attract, their velocities must increase to keep the total angular momentum constant.
The linear momentum is also conserved, starting and ending with a total of zero.
More detailed information on torque, levers, and the universal law of rotation is available in the video series.
Conservation of energy, charge, momentum, and angular momentum are fundamental principles in physics.
Understanding these conservation laws is crucial for analyzing the behavior of particles and systems in physics.
The concepts of momentum and angular momentum are essential for studying motion and forces in physics.
This video provides a clear and concise explanation of the conservation of momentum and angular momentum.
The parallelogram formed by position and momentum vectors is used to visualize and calculate angular momentum.
The direction of angular momentum is perpendicular to the plane formed by the position and momentum vectors.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: