Angular momentum | Moments, torque, and angular momentum | Physics | Khan Academy
TLDRThis video script delves into the concepts of momentum and angular momentum, explaining how momentum is the product of mass and velocity and is a measure of an object's resistance to stopping. It introduces impulse as the product of force and time, equating to a change in momentum. The script then transitions to angular momentum, illustrating its relationship with mass, velocity, and the distance from the center of rotation. It highlights the conservation of angular momentum in the absence of torque and demonstrates how changing the radius of rotation affects angular velocity, using the example of figure skaters to make the concept relatable.
Takeaways
- 📦 The script reviews translational momentum, defined as the mass times the velocity, represented by the Greek letter rho.
- 🛑 Momentum indicates how hard it is to stop a moving object; the more momentum, the harder it is to stop.
- 📏 Changing momentum requires applying force over time, which is known as impulse.
- 🔄 The script introduces angular momentum, similar to translational momentum but in the rotational world.
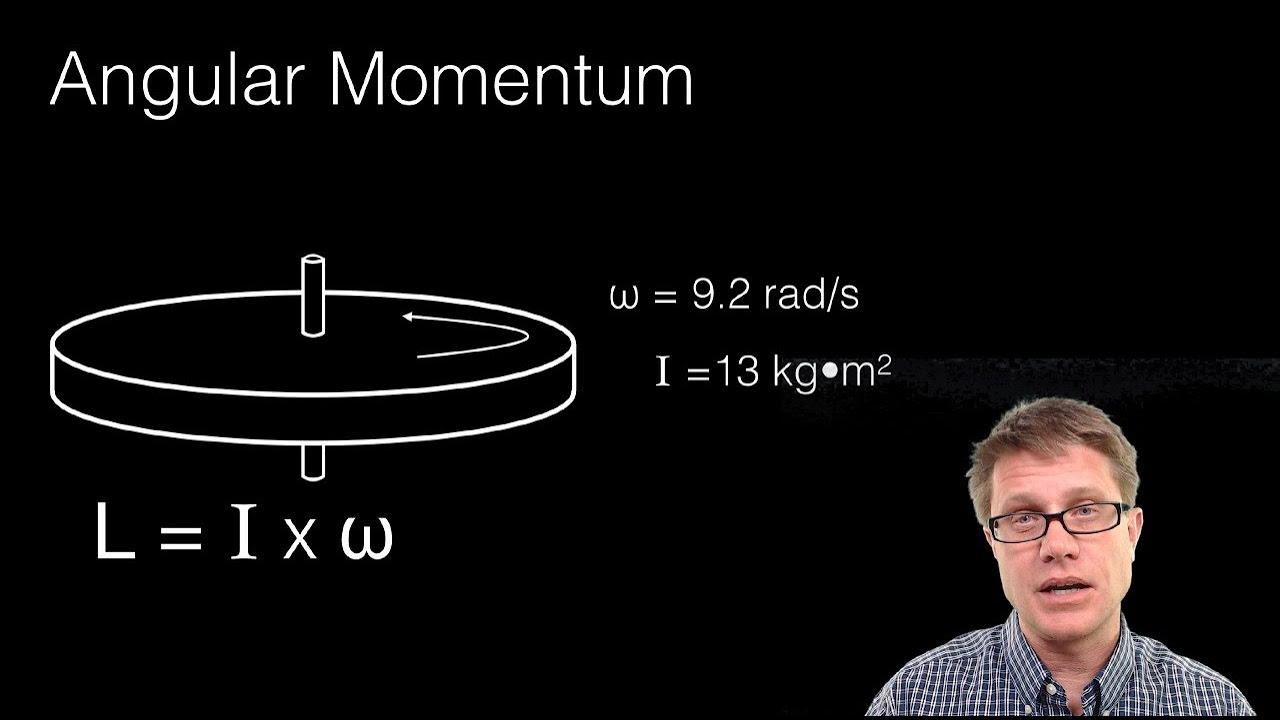
- 🌀 Angular momentum (L) is defined as the mass times the tangential velocity times the radius.
- ⚖️ In the absence of torque, angular momentum remains constant.
- 🌪️ Reducing the radius of rotation increases tangential velocity, while increasing the radius decreases it.
- ⏳ Tangential velocity is closely related to angular velocity, measured in radians per second.
- 🏋️♀️ The conservation of angular momentum explains why figure skaters spin faster when they pull their arms in and slower when they extend them.
- 🔧 Torque is the rotational equivalent of force, influencing how objects rotate.
Q & A
What is the definition of translational momentum?
-Translational momentum, represented by the Greek letter rho, is defined as the product of an object's mass and its velocity (m * v). It is a measure of how difficult it is to stop an object moving in a straight line.
How is impulse related to momentum?
-Impulse is the product of the magnitude of a force and the time over which it is applied (force * time). It is equal to the change in momentum of an object. If there is no net impulse (i.e., no net force acting over time), the momentum of an object remains constant.
What is the concept of conservation of momentum?
-The conservation of momentum states that if no net force acts on an object or a system of objects, the total momentum of the system remains constant. This principle is fundamental in various physics applications.
What is angular momentum and how is it related to rotation?
-Angular momentum is a measure of how difficult it is to stop an object from rotating. It is defined as the product of an object's mass, the component of its velocity that is perpendicular to the radius (tangential velocity), and the distance from the center of rotation (r). The formula for angular momentum (L) is mass * tangential velocity * r.
Why is angular momentum represented by the letter L?
-The script does not provide a definitive reason for using 'L' to represent angular momentum. It suggests that it might be due to other letters being already used for different concepts in physics.
What is the relationship between angular momentum and torque?
-Just as force can change an object's translational motion, torque can change an object's rotational motion. If the net torque on an object is zero, there will be no change in its angular momentum, indicating a conservation of angular momentum.
How does the radius affect the tangential velocity in the context of angular momentum?
-If angular momentum is constant and the radius (r) decreases, the tangential velocity must increase to maintain that constant angular momentum, according to the formula L = mass * tangential velocity * r.
What is the relationship between tangential velocity and angular velocity?
-Tangential velocity is the component of velocity that is perpendicular to the radius of rotation. Angular velocity (omega) is defined as tangential velocity divided by the radius (v = omega * r). If the radius is constant, an increase in tangential velocity results in an increase in angular velocity.
How do figure skaters use the principle of conservation of angular momentum?
-Figure skaters can change their angular velocity by altering their radius without the application of external torque. When they pull their arms in (decreasing the radius), their angular velocity increases, causing them to spin faster. Conversely, when they extend their arms out, they increase the radius and decrease their angular velocity, spinning slower.
What is the formula for angular momentum in terms of angular velocity?
-The formula for angular momentum in terms of angular velocity is L = mass * omega * r^2, where omega is the angular velocity, r is the radius, and mass is the mass of the object.
Outlines
🚀 Introduction to Momentum and Angular Momentum
This paragraph introduces the concept of translational momentum, represented by the Greek letter rho, and explains it as the product of mass and velocity. It emphasizes the difficulty of stopping an object with greater momentum and relates it to the need for force over time to change momentum, known as impulse. The paragraph also touches on the conservation of momentum when no net force is applied. It then transitions into the concept of rotational motion, introducing torque and angular momentum, and setting the stage for a deeper exploration of angular momentum in subsequent paragraphs.
🌀 Defining Angular Momentum and Its Conservation
The second paragraph delves into angular momentum, defining it as the product of mass, the component of velocity perpendicular to the radius (referred to as tangential velocity), and the distance from the center of rotation (radius). It highlights the conservation of angular momentum when the net torque is zero, drawing parallels with the conservation of linear momentum. The paragraph also introduces the idea that changing the radius while keeping angular momentum constant results in a change in tangential velocity, a concept that is mathematically explored through the relationship between tangential velocity, angular velocity, and radius.
🏒 The Relationship Between Radius and Angular Velocity
In this paragraph, the relationship between a figure's radius and its angular velocity is explored, particularly in the context of constant angular momentum. It explains that decreasing the radius results in an increase in tangential and angular velocity, while increasing the radius has the opposite effect. The concept is illustrated with the example of a figure skater who spins faster when bringing their arms closer to the body and slower when extending them, demonstrating the principle of angular momentum conservation in a real-world scenario.
Mindmap
Keywords
💡Momentum
💡Impulse
💡Conservation of Momentum
💡Rotational Motion
💡Torque
💡Angular Momentum
💡Radius
💡Tangential Velocity
💡Angular Velocity
💡Figure Skater
Highlights
Momentum is defined as the product of an object's mass and its velocity.
Momentum is a measure of how difficult it is to stop an object in motion.
Impulse is the product of force and the time it is applied, and is equal to the change in momentum.
Conservation of momentum occurs when no net force acts on an object.
Angular momentum is introduced as a concept similar to momentum but in the rotational context.
Angular momentum is defined as mass times the component of velocity perpendicular to the radius, times the radius.
Both momentum and angular momentum are vector quantities with direction.
The letter 'L' is used to represent angular momentum, though the reason for this choice is not clear.
Angular momentum is analogous to torque in the rotational world as force is to translation.
In the absence of torque, angular momentum remains constant, similar to the conservation of momentum.
Changing the radius of rotation can alter the tangential velocity while keeping angular momentum constant.
The relationship between tangential velocity, angular velocity, and radius is explored.
Angular velocity is directly proportional to the radius when angular momentum is constant.
An increase in radius results in a decrease in angular velocity, and vice versa.
The concept is exemplified by figure skaters who spin faster when they pull their arms in.
Figure skaters demonstrate the principle of angular momentum by changing their spin speed without external torque.
The video discusses the practical applications of angular momentum in real-world scenarios like sports.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: