Solving Conservation of Mechanical Energy Problems
TLDRIn this engaging video, the Physics Ninja explores the conservation of mechanical energy in two scenarios: one with frictionless conditions and another introducing friction. The first scenario involves a skier going down a slope, taking a jump, and flying through the air, aiming to calculate the maximum height reached by the skier. The second scenario adds friction at the bottom of the hill, leading to a loss of mechanical energy converted into heat. Through detailed calculations and step-by-step explanations, the Ninja demonstrates how to modify the equations to account for friction and its impact on the skier's maximum height.
Takeaways
- 🏂 The physics problem involves a skier going down a slope, taking a jump, and flying through the air, focusing on conservation of energy.
- 📈 Two cases are discussed: one with no friction between the skis and snow (icy surface), and another introducing friction at the bottom of the slope.
- 🌟 The goal in both cases is to calculate the maximum height the skier can attain, with the second case expecting a lower maximum height due to friction.
- 📊 Mechanical energy is the sum of kinetic and potential energy, which is conserved in the absence of non-conservative forces like friction.
- 🎯 The first case uses conservation of mechanical energy to find the skier's maximum height without friction, resulting in approximately 5 meters.
- 🔧 Friction is introduced in the second case, causing mechanical energy loss and conversion to heat, affecting the skier's maximum height.
- 📐 The skier's speed is calculated using the conservation of energy principles, with the speed at the top of the jump being crucial for the next calculations.
- 🔄 The process involves breaking down the skier's velocity into components, considering only the horizontal component (VX) for energy conservation calculations at the top of the jump.
- 🔢 The maximum height in the second case, with friction, is found to be approximately 4.75 meters, lower than the first case due to energy loss.
- 👍 The video encourages viewers to like and subscribe for more content, emphasizing support for the creator's work.
- 📚 The problem-solving approach is detailed, emphasizing the importance of understanding energy conservation with and without friction for solving such physics problems.
Q & A
What is the main topic of the video?
-The main topic of the video is solving a physics problem involving a skier going down a slope, taking a jump, and flying through the air, using the principle of conservation of energy.
How many cases does the presenter solve in the video?
-The presenter solves two cases in the video. The first case assumes no friction between the skis and the snow, and the second case introduces friction at the bottom of the hill.
What is the goal in the first case without friction?
-The goal in the first case is to set up the equations for conservation of mechanical energy and calculate the maximum height the skier can attain.
How does the introduction of friction in the second case affect the problem?
-The introduction of friction in the second case causes the skier to lose some mechanical energy, which is converted to heat due to the work done by the force of friction, resulting in a lower maximum height reached by the skier.
What are the two forms of energy considered in the problem?
-The two forms of energy considered in the problem are kinetic energy, which is energy due to the motion of the skier, and gravitational potential energy, which is due to the force of gravity.
What is the total mechanical energy in the system?
-The total mechanical energy in the system is the sum of the kinetic energy and the gravitational potential energy at any point in the system. This total mechanical energy is conserved, meaning it does not change when the skier goes down, takes the jump, and flies through the air in the absence of friction.
How is the speed of the skier at the top of the hill calculated?
-The speed of the skier at the top of the hill is calculated by using the conservation of energy principle. The initial total energy (E1) is set equal to the total energy at the top (E2), and by solving for the kinetic energy at the top (K2), the speed can be found using the formula for kinetic energy (K = 0.5 * m * v^2).
What is the significance of the angle of the jump in the problem?
-The angle of the jump is significant because it affects the trajectory of the skier after taking the jump. The speed of the skier is broken down into horizontal (X) and vertical (Y) components, with the vertical component being zero at the top of the jump, indicating that the skier is not moving vertically instantaneously at that point.
How does the work done by friction affect the skier's energy in the second case?
-In the second case, the work done by friction is calculated as the force of friction (mu * N) multiplied by the displacement (D) with a cosine of 180 degrees (since the force is opposite to the direction of motion). This work done by friction is negative, indicating that mechanical energy is lost from the system as heat due to friction.
What is the result of the maximum height calculation in the second case with friction?
-In the second case with friction, the maximum height the skier attains is approximately 4.75 meters, which is lower than the 5 meters calculated in the first case without friction.
How does the video demonstrate the concept of energy conservation?
-The video demonstrates the concept of energy conservation by showing that in the absence of non-conservative forces (like friction), the total mechanical energy of the system remains constant. However, when friction is introduced, some of this energy is converted to heat, resulting in a decrease in the skier's maximum height.
Outlines
🏂 Skier's Energy Conservation
This paragraph introduces a physics problem involving a skier going down a slope, taking a jump, and flying through the air. The goal is to solve this using the conservation of energy principle. Two cases are considered: one with no friction between the skis and the snow, and another introducing friction at the bottom of the hill. The focus is on setting up equations for mechanical energy conservation and calculating the maximum height the skier can attain in each scenario.
📚 Review of Mechanical Energy
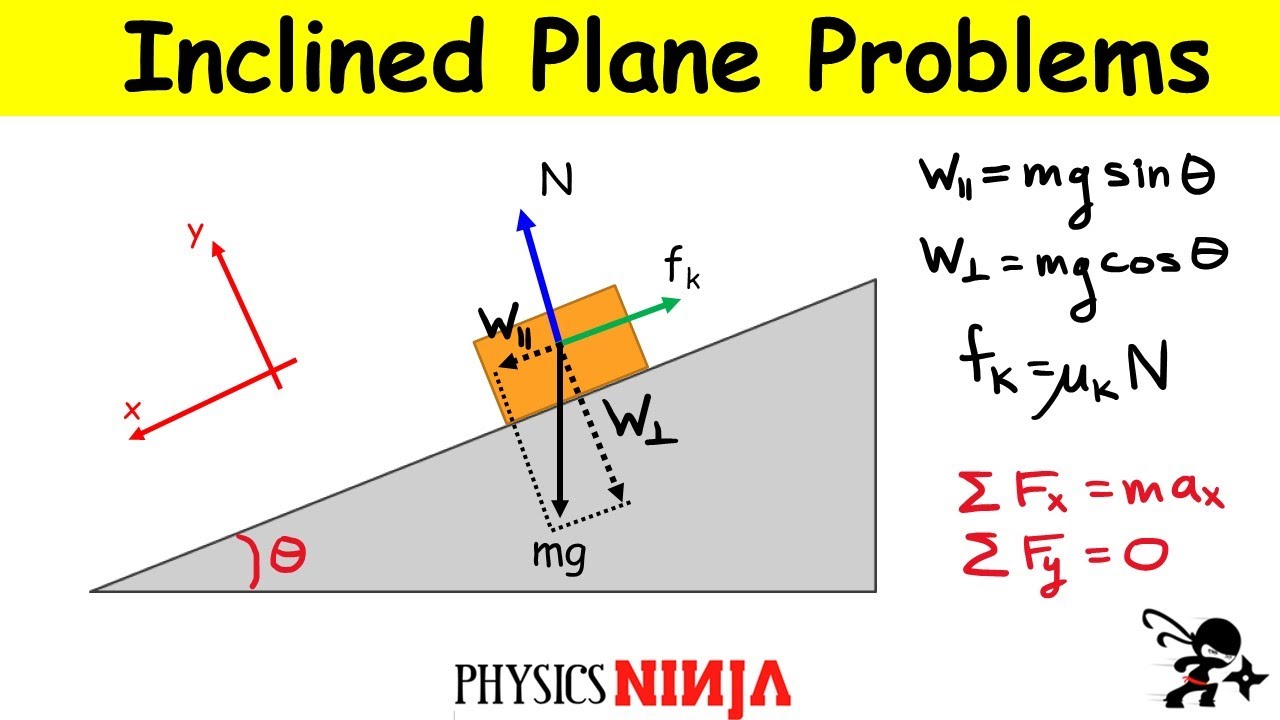
The speaker reviews the concept of mechanical energy, which includes kinetic and potential energy. Kinetic energy is due to the motion of the skier and is calculated as (1/2)mv^2, while potential energy is due to gravity and is calculated as mgh. The total mechanical energy is the sum of these two forms and remains constant in the absence of friction. The importance of setting a reference point for zero potential energy is emphasized, as it affects how potential energy is calculated at different points.
🌟 Solving the First Case: No Friction
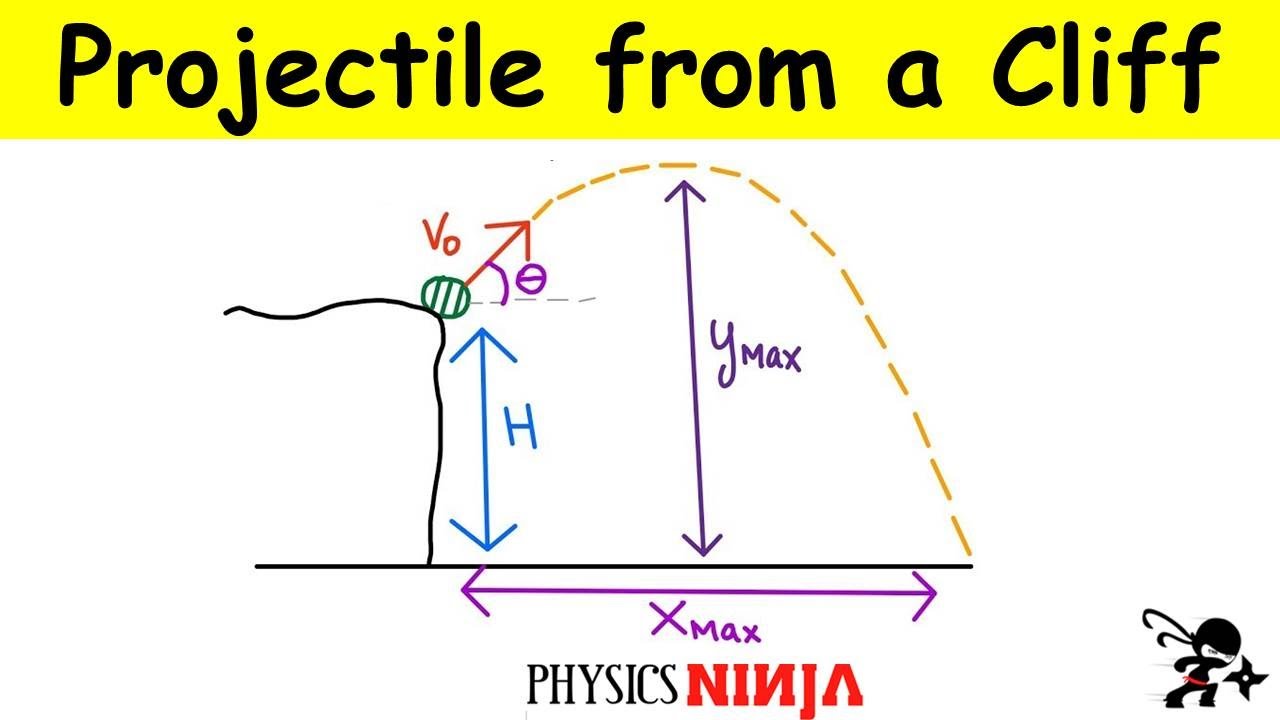
The first case without friction is solved by setting up the conservation of mechanical energy at three different points: the top of the hill, the top of the jump, and the maximum height. The skier's speed at the top of the hill is calculated using the conservation of energy principle. The speaker then uses vector components to find the horizontal velocity component and applies it to calculate the maximum height the skier can reach, which is found to be approximately 5 meters.
🚧 Introducing Friction in the Second Case
The second case introduces friction in a flat section of the slope. The speaker explains how friction affects the conservation of mechanical energy, leading to a loss of energy as heat. The force of kinetic friction is calculated based on the coefficient of kinetic friction and the normal force. The work done by friction is found to be negative, indicating energy loss. The skier's speed is recalculated after passing through the frictional section, and the conservation of energy principle is applied again to find the new maximum height, which is lower than in the first case due to the reduced initial speed.
📈 Calculating Maximum Height with Friction
The speaker continues to solve the second case with friction, focusing on the energy at different points. The kinetic energy after the rough patch is used to find the speed at the top of the jump. The horizontal component of this speed is used to calculate the maximum height achieved in the presence of friction. The final result shows that the maximum height is approximately 4.75 meters, which is lower than the height achieved without friction, demonstrating the impact of friction on the skier's trajectory.
Mindmap
Keywords
💡Conservation of Energy
💡Mechanical Energy
💡Kinetic Energy
💡Potential Energy
💡Friction
💡Projectile Motion
💡Work Done by Friction
💡Non-Conservative Forces
💡Energy Loss
💡Velocity Components
Highlights
The physics problem involves a skier going down a slope, taking a jump, and flying through the air, aiming to solve this using conservation of energy principles.
Two different cases are discussed: one with no friction between the skis and the snow, and another introducing friction at the bottom of the hill.
In the frictionless case, the goal is to set up equations for conservation of mechanical energy and calculate the skier's maximum height.
Friction is introduced in the second case, causing mechanical energy loss and conversion to heat due to the work done by friction.
Mechanical energy consists of kinetic and potential energy, with kinetic energy due to motion and potential energy due to gravity.
The total mechanical energy is the sum of kinetic and gravitational potential energy, which remains constant in a conservative system without friction.
The skier's speed at the top of the hill can be calculated using the conservation of energy principle and the known values of mass, height, and angle.
The maximum height (hmax) is calculated to be approximately 5 meters in the frictionless case.
In the case with friction, the skier's speed is reduced due to energy loss, affecting the maximum height achieved.
The work done by friction is calculated, showing a loss of 294 joules of mechanical energy.
The skier's speed at the top of the jump is adjusted for the energy loss due to friction, resulting in a lower maximum height.
The final calculated maximum height in the presence of friction is approximately 4.75 meters, which is lower than the frictionless case.
The problem demonstrates the impact of friction on the conservation of mechanical energy and the resulting changes in motion.
The video provides a detailed walkthrough of the equations and calculations involved in solving the problem with and without friction.
The physics ninja uses algebraic manipulation and trigonometry to solve for the skier's speed and maximum height in both scenarios.
The video concludes with a comparison of the two cases, emphasizing the intuitive understanding that friction reduces the skier's maximum height.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: