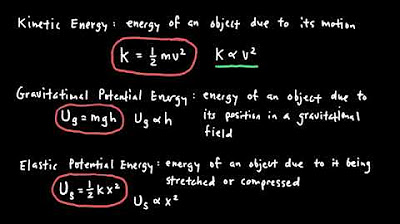Work and Energy

Bozeman Science
25 Nov 201407:00
EducationalLearning
32 Likes 10 Comments

TLDRIn this AP Physics essentials video, Mr. Andersen explores the relationship between work and energy through the sport of pole vaulting and provides a detailed explanation of how kinetic and potential energy transform during the process. He illustrates the concept with a practical example of a bus being pushed with a constant force, calculating the work done and the resulting kinetic energy. The video also covers the application of trigonometry for forces at an angle and emphasizes the importance of understanding work-energy principles for predicting changes in mechanical energy.

Takeaways
  • 🏆 The world record in women's pole vault is held by Elena Isinbekova, with a record height of 16 feet 7 inches.
  • 🔋 In pole vaulting, athletes convert kinetic energy from their run into elastic potential energy in the pole, and then into gravitational potential energy over the bar.
  • 🏃‍♀️ The concept of work in physics is central to understanding how force and displacement contribute to the energy transfer during physical activities like pole vaulting.
  • 📈 Work done can be visualized and calculated using a force-displacement graph, where the area under the curve represents the amount of work.
  • 🚌 An example of calculating work involves applying a 12,000 N force to move a bus 48 meters, resulting in 580,000 joules of work done and an increase in kinetic energy.
  • 📚 The work-energy theorem allows us to determine changes in mechanical energy and, given enough information, calculate final velocities based on work done.
  • 🔢 When dealing with forces at an angle, trigonometry is used to find the component of the force parallel to the displacement to accurately calculate work.
  • 🛠 The area under a force-displacement graph represents the total work done on an object and the energy transferred to the system.
  • 🏢 In the given example, a 3 kg block experiences work done by varying forces, which can be calculated by determining the area under the curve for each segment of the graph.
  • 🔄 The conservation of energy principle is key in understanding that the work done on a system is equivalent to the change in mechanical energy, assuming no energy is lost to friction or other resistive forces.
  • 🤔 The script encourages viewers to apply these concepts to make predictions about changes in mechanical energy and to solve practical problems involving work and energy.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is work and energy, specifically in the context of physics and how it relates to the pole vault event.

  • Who holds the world record in women's pole vault, and what is her record height?

    -Elena Isinbeava holds the world record in women's pole vault with a height of sixteen feet seven inches.

  • How does the pole vaulter increase their kinetic energy during the run-up?

    -The pole vaulter increases their kinetic energy by running down the runway, which involves gaining speed and momentum.

  • What type of energy is stored in the pole during the pole vault?

    -During the pole vault, the kinetic energy of the runner is stored as elastic potential energy in the pole.

  • What is the relationship between the energy stored on the runway and the potential energy at the top in a pole vault?

    -The amount of energy stored on the runway is equivalent to the amount of gravitational potential energy the vaulter has at the top of the jump.

  • What is the significance of displacement in the context of work done in physics?

    -Displacement is significant because it is the distance over which a force is applied, and it is necessary for work to be done. Work is defined as the force applied over a displacement.

  • How can a force versus displacement graph be used to calculate work done?

    -A force versus displacement graph can be used to calculate work done by finding the area under the curve of the graph, which represents the mechanical energy added to the system.

  • What is the formula for calculating work done on an object?

    -The formula for calculating work done on an object is W = F * s, where W is work, F is the force applied, and s is the displacement of the object.

  • How can the work done on the bus in the example be converted into kinetic energy?

    -The work done on the bus (580,000 joules) is converted into kinetic energy, which increases the bus's velocity from 0 to 13 meters per second, assuming no energy is lost to friction.

  • How can the angle of the force relative to the displacement affect the amount of work done?

    -The angle of the force relative to the displacement affects the amount of work done because only the component of the force parallel to the displacement does work. This can be calculated using trigonometry, such as finding the cosine of the angle.

  • What is the importance of understanding the work-energy theorem?

    -Understanding the work-energy theorem is important because it allows us to predict changes in mechanical energy within a system and to calculate quantities such as velocity, given the work done and the conservation of energy.

Outlines
00:00
🏆 Understanding Work and Energy through Pole Vaulting

This paragraph introduces the concept of work and energy using the example of pole vaulting, specifically highlighting the world record set by Elena Isinbeava. It explains how the kinetic energy gained by the vaulter during the run-up is converted into gravitational potential energy, and then back into kinetic energy. The paragraph also discusses the mechanical work done by the pole, the importance of displacement caused by an unbalanced force, and how work can be calculated using a force-displacement graph. A practical problem involving a bus being pushed with a constant force is used to illustrate the calculation of work and its equivalence to kinetic energy, leading to the determination of the bus's final velocity using the work-energy theorem.

05:02
📈 Calculating Work with Varying Forces and Displacements

This paragraph delves into the calculation of work when forces vary over time or distance. It uses the analogy of a block being pushed with a constant force for a certain distance, followed by a reduction in force. The concept of calculating work through the area under a force-displacement graph is emphasized, with a step-by-step explanation of how to determine the work done in each segment of the graph. The paragraph concludes with a review of the principles of mechanical energy conservation and the application of the work-energy theorem to predict changes in energy and velocity, reinforcing the concepts learned through the examples provided.

Mindmap
Keywords
💡Work
Work in the context of physics is defined as the amount of energy transferred by a force acting over a distance. It is a measure of energy transfer and is calculated as the product of the force applied and the displacement in the direction of the force. In the video, work is related to the mechanical energy transferred to the pole vaulter as she runs down the runway and vaults over the bar, as well as the work done on the bus when it is accelerated by a force, resulting in an increase in its kinetic energy.
💡Energy
Energy is a fundamental concept in physics that describes the ability to do work. It exists in various forms such as kinetic, potential, and mechanical energy. In the video, the focus is on mechanical energy, which includes both kinetic and potential energy. The conservation of mechanical energy is a key theme, as it is transferred and transformed but not lost in a closed system, such as the pole vaulter's system or the bus being pulled.
💡Kinetic Energy
Kinetic energy is the energy of motion. It is directly proportional to the mass of an object and the square of its velocity. In the video, kinetic energy is used to describe the energy a moving object possesses, such as the bus that gains kinetic energy when work is done on it, resulting in an increase in its velocity.
💡Potential Energy
Potential energy is the stored energy an object has due to its position or condition. In the video, gravitational potential energy is discussed in relation to the pole vaulter who gains potential energy as she moves upward, and elastic potential energy is mentioned in the context of the pole storing energy during the vault.
💡Mechanical Energy
Mechanical energy is the sum of kinetic and potential energies in a system. It is conserved in a closed system, meaning the total amount remains constant despite transformations between potential and kinetic forms. The video emphasizes the conservation of mechanical energy in the context of work done on a system, such as the pole vaulter and the bus.
💡Force
Force is any action that can cause an object to change its state of motion or rest. In physics, it is described as a push or pull acting upon an object resulting in acceleration. The video discusses the application of force in the context of work, such as the force applied to the bus and the force exerted by the pole during a pole vault.
💡Displacement
Displacement refers to the change in position of an object and is a vector quantity that has both magnitude and direction. In the context of work, displacement is the distance an object moves in the direction of the applied force. The video uses displacement to explain how work is calculated and how it relates to the transfer of energy.
💡Conservation of Energy
The principle of conservation of energy states that the total amount of energy in a closed system remains constant. It cannot be created or destroyed, only transformed from one form to another. In the video, this principle is applied to explain the relationship between work and energy, emphasizing that the work done on a system adds to its mechanical energy without loss.
💡Velocity
Velocity is a vector quantity that describes the rate of change of an object's position with respect to time, taking into account both speed and direction. In the video, velocity is used to describe the final speed of the bus after work has been done on it, and it is calculated using the work-energy principle.
💡Parallel Force
A parallel force is a force that acts in the same direction as the displacement of an object. In the context of work, only the component of the force that is parallel to the displacement contributes to the work done. The video emphasizes the importance of the parallel component of force in calculating work and understanding energy transfer.
💡Force-Displacement Graph
A force-displacement graph is a graphical representation that shows the relationship between the force applied to an object and the displacement it undergoes. The area under the curve on this graph represents the work done by the force. In the video, this graph is used as a tool to visualize and calculate work in different scenarios, including varying forces over time.
Highlights

The topic of the video is work and energy, with a focus on the pole vault as an example of the relationship between energy and work.

Elena Isinbeava holds the world record in women's pole vault at sixteen feet seven inches.

The physics of pole vaulting involves converting kinetic energy into elastic potential energy and then into gravitational potential energy.

The pole does work by applying a force over a distance, which is a key concept in understanding mechanical energy.

In a system, mechanical energy can be added or removed, and it can be in the form of potential or kinetic energy.

Displacement in a system is caused by an unequal parallel force, which is essential for work to be done.

Work can be calculated using a force versus displacement graph, where the area under the curve represents the amount of work done.

A constant force applied over a displacement results in work equal to the product of the force and the displacement.

An example problem involves calculating the work done on a bus with a 12,000 newton force over a 48-meter displacement, resulting in 580,000 joules of work.

The work done on an object is equivalent to the change in its kinetic energy.

Using the work-energy theorem, one can determine the final velocity of an object given the work done on it.

A bus with a mass of 6,500 kilograms reaches a velocity of 13 meters per second after work is done on it.

In a different scenario, a giant pulls a bus with a 12,000 newton force at a 38-degree angle, requiring trigonometry to calculate the work done.

The force versus displacement graph is a useful tool for visualizing and calculating work done on an object.

An example with a 3-kilogram block demonstrates how to calculate work done by analyzing the area under a force-displacement graph.

The total work done on the block is the sum of the work calculated from the areas of rectangles and triangles under the force-displacement graph.

The concept of work-energy theorem allows for predictions about changes in mechanical energy and the conservation of energy in a system.

The video aims to help viewers understand how to apply these concepts to real-world problems, such as determining the velocity of a bus.

Transcripts

Browse More Related Video

Work Energy Principle

Work and Energy

AP Physics C: Work, Energy, and Power Review (Mechanics)

AP Physics 1 Energy Review

Total Energy

A Level Physics Revision: All of Work, Energy and Power (in 18 minutes)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics PrinciplesWork-Energy TheoremPole VaultingMechanical EnergyAP PhysicsElena IsinbayevaKinetic EnergyPotential EnergyForce DisplacementProblem Solving