AP Physics 1 Work and Energy Practice Problems and Solutions
TLDRThe video script discusses various physics problems related to work, power, and energy, focusing on concepts such as constant velocity, net force, and the effects of friction and gravity on work done. It covers scenarios like lifting a box, pulling a trash can, and objects moving down an inclined plane, calculating the work done by applied forces and the resulting changes in kinetic energy. The script also explores the forces acting on a wheel rolling down an incline and derives expressions for linear acceleration and compares the speed of a wheel and a block of ice on the same ramp.
Takeaways
- 🏋️ Work done on an object is calculated as force times distance times the cosine of the angle between the force and displacement.
- 🔄 Constant velocity indicates no net force acting on an object, which means no net work is done.
- 📈 The work done by gravity on an object is negative when the object moves in the opposite direction to the force.
- 🔄 When an object moves at a constant velocity, its kinetic energy does not change.
- 🚯 The work done by friction is always negative and represents energy lost to heat or other forms.
- 📐 For a can being pulled on a flat surface, the net work done is the work done by the applied force minus the work done by friction.
- 🔄 The gravitational and normal forces do no work when they are perpendicular to the displacement.
- 🚴 For a bike rider descending an incline, the speed at the bottom can be found using energy conservation principles.
- 🛷 A box sliding down a frictionless incline will have its entire potential energy converted into kinetic energy.
- 🔄 The frictional force is the only force that causes a change in angular velocity (rotational speed) of an object.
- 🚀 The net force on an object determines its acceleration, which can be used to calculate its final speed when starting from rest.
Q & A
What is the work done by lifting a 4 kg box straight up by 2 meters at a constant velocity?
-The work done is 78.4 joules. This is calculated by using the formula work = force * distance * cos(theta), where the force is the weight (mass * gravity), distance is 2 meters, and theta is 0 degrees (since the force and displacement are in the same direction).
What is the work done by the gravitational force on the 4 kg box when lifted and moved at a constant velocity?
-The work done by gravity is -78.4 joules. This is because the gravitational force and the displacement are in opposite directions, making the angle 180 degrees, and the cosine of 180 degrees is -1.
What is the net work done on the 4 kg box when it is lifted and moved at a constant velocity?
-The net work done on the box is 0 joules. Since there is no net force acting on the box (as it is moving at a constant velocity), no net work is done according to the formula for work.
How does the speed of the 4 kg box change during its displacement at a constant velocity?
-The speed of the box does not change during its displacement. Since there is no net force acting on the box and net work is zero, according to the work-energy principle, there is no change in kinetic energy, implying constant speed.
What are the forces acting on a trash can when it is pulled across a flat surface with a rope?
-The forces acting on the trash can include the applied force (pulling force) of 50 newtons to the right, the frictional force of 30 newtons to the left, the weight acting downward (balanced by an equal and opposite normal force), and the normal force acting upward.
How much work is done by the applied force when pulling the trash can across the flat surface?
-The work done by the applied force is 500 joules. This is calculated using the formula work = force * distance * cos(theta), with the force being 50 newtons, the distance 10 meters, and theta being 0 degrees (since the force and displacement are in the same direction).
What is the work done by the frictional force on the trash can when it is pulled across the flat surface?
-The work done by the frictional force is -300 joules. This is because the frictional force is acting in the opposite direction to the displacement, making the angle 180 degrees, and the cosine of 180 degrees is -1.
How much net work is done on the trash can when it is pulled across the flat surface?
-The net work done on the trash can is 200 joules. This is calculated by taking the work done by the applied force (500 joules) and subtracting the work done by the frictional force (-300 joules).
What is the speed of the can at the end of the 10 meters when it is pulled by a force of 50 newtons with friction acting?
-The speed of the can is 10 meters per second. This is found by using the net work done on the can (200 joules) and equating it to the change in kinetic energy, which is given by the formula KE = 0.5 * m * v^2, where m is the mass of the can and v is its final velocity.
What is the speed of the bike rider at the bottom of a 500-meter long incline with an angle of 5 degrees, considering a combined mass of 90 kg and a frictional force of 60 newtons?
-The speed of the bike rider at the bottom of the incline is 13.7 meters per second. This is calculated by first determining the gravitational potential energy at the top of the hill, then accounting for the work done against friction, and finally equating the remaining energy to the kinetic energy at the bottom of the hill.
What is the speed of a box sliding down a frictionless inclined plane of length 10 meters and an angle of 20 degrees above the ground?
-The speed of the box at the bottom of the incline is 8.19 meters per second. This is found by equating the gravitational potential energy at the top of the incline to the kinetic energy at the bottom, without considering friction, and using the formulas for potential and kinetic energy.
Which object reaches the bottom of an incline with the greatest speed: a wheel or a block of ice?
-The block of ice reaches the bottom with the greatest speed. This is because the ice has less friction with the plane, leading to more efficient conversion of gravitational potential energy to kinetic energy, and thus a higher final speed compared to the wheel, which has more friction and also has to distribute some energy into rotational motion.
Outlines
🏋️ Work, Power, and Energy Problems
Matt Dean introduces a series of physics problems related to work, power, and energy. The first problem involves lifting a 4 kg box vertically 2 meters off the ground at a constant velocity. The key concept is that the box is in equilibrium, meaning the lift force equals the weight of the box. Using the formula for work (force times distance times cosine of the angle), the work done is calculated to be 78.4 Joules. The second problem examines the work done by gravity, resulting in -78.4 Joules, illustrating negative work. The third problem discusses net work, which is zero in this case since there is no net force or change in kinetic energy.
🚀 Traction and Friction
The fifth problem involves pulling a trash can on a flat surface with a 50 N force while overcoming 30 N of friction. A free body diagram is drawn to depict all forces acting on the can. The work done by the applied force is 500 Joules, as the angle between the force and displacement is zero. The sixth problem calculates the work done by friction, which is -300 Joules due to the 180-degree angle between the force and displacement. Problems seven and eight address the work done by gravitational and normal forces, both resulting in zero work due to the angles of 270 and 90 degrees, respectively. The ninth problem asks for the net work, which is 200 Joules, calculated by subtracting the work done by friction from the work done by the applied force.
🏎️ Kinetic Energy and Speed
In the tenth problem, the net work done is related to the change in kinetic energy. With 200 Joules of net work, the final kinetic energy is also 200 Joules, leading to a final speed of 10 meters per second for the can. The eleventh problem involves a bike rider descending a 500-meter long incline at a 5-degree angle. The initial gravitational potential energy is converted to kinetic energy, minus the work done by friction. The final kinetic energy is 8494 Joules, resulting in a final speed of 13.7 meters per second. The twelfth problem is similar but for a box sliding down a frictionless incline, where the entire potential energy is converted to kinetic energy, leading to a final speed of 8.19 meters per second.
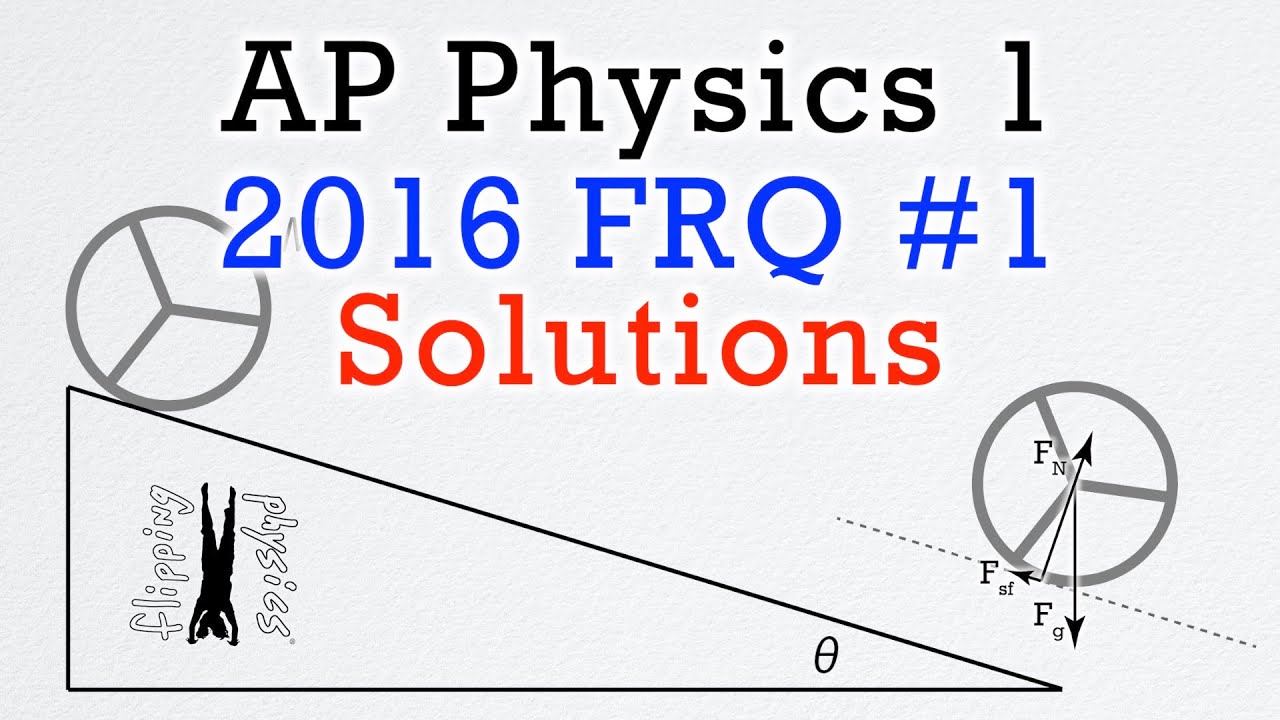
🔄 Forces and Torque on an Incline
The long free response question begins with identifying forces on a wheel rolling down an inclined plane, including weight, normal force, and frictional force. The frictional force is the only one causing torque and changing the wheel's angular velocity. In part b, the wheel's linear acceleration is derived using the net force (0.6 times the weight times the sine of the incline angle) and the mass, resulting in an acceleration of 0.6 times the gravitational acceleration times the sine of the angle. Part c compares a wheel and a block of ice on the same incline, concluding that the block of ice will reach the bottom with the greatest speed due to less friction and all potential energy being converted into translational kinetic energy.
Mindmap
Keywords
💡Work
💡Power
💡Energy
💡Force
💡Constant Velocity
💡Kinetic Energy
💡Gravitational Potential Energy
💡Friction
💡Cosine
💡Net Work
💡Inclined Plane
Highlights
Calculating work done on a box lifted vertically at constant velocity, with work equal to force times distance times cosine of the angle between force and displacement.
The work done on the box is 78.4 joules, as the angle is zero and cosine of zero is one.
Gravitational force does negative work on the box, amounting to -78.4 joules, due to the 180-degree angle between displacement and gravitational force.
Net work done on the box is zero, as there is no net force acting on it, and work is equal to change in kinetic energy, which is zero in this case.
The speed of the box does not change during displacement due to zero net work and constant kinetic energy.
In a different scenario, 50 newtons of force applied to a trash can on a flat surface moves it 10 meters, with work done by the applied force calculated as 500 joules.
Frictional force does -300 joules of work, as it acts in the opposite direction of motion with a cosine of 180 degrees equal to -1.
Gravitational and normal forces do zero work on the trash can since they are perpendicular to the displacement.
Net work done on the trash can is 200 joules, which is the work done by the applied force minus the work done by the frictional force.
The net work done on an object is equal to the change in its kinetic energy.
A can increases in speed and kinetic energy by 200 joules after 10 meters of displacement with an initial speed of zero.
Bike rider and bike with a combined mass of 90 kilograms gain a speed of 13.7 meters per second at the bottom of a 500-meter long incline with a 5-degree angle.
Frictional force cancels out 30,000 joules of the initial potential energy, leaving 8,494 joules to be converted into kinetic energy.
A box slides down a frictionless inclined plane gaining a speed of 8.19 meters per second at the bottom due to conversion of potential energy to kinetic energy.
Frictional force causes a change in the angular velocity of a wheel rolling down an inclined plane.
The linear acceleration of the wheel's center of mass is derived as 0.6 times the gravitational acceleration times the sine of the ramp angle.
Between a wheel and a block of ice starting from the same height on an incline, the block of ice will reach the bottom with the greatest speed due to less friction.
The block of ice will accelerate faster and have a greater speed at the bottom because it converts more potential energy into translational kinetic energy compared to the wheel.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: