Definition of the Derivative
TLDRThis lesson delves into the concept of derivatives, starting with a refresher on the difference quotient and its connection to the slope of a secant line over an interval, which represents the average rate of change. The focus then shifts to the limit of the difference quotient as 'h' approaches zero, which is key to defining the derivative. The derivative, symbolized as 'F Prime of X,' is the instantaneous rate of change or the slope of the tangent line at a specific point on a curve. The process of finding a derivative is known as differentiation. The video provides step-by-step examples of finding derivatives for different types of functions, such as quadratic, rational, and square root functions. It also demonstrates how to find the slope of the tangent line at a specific point and how to derive the equation of the tangent line using point-slope form. The lesson concludes with conditions under which a derivative does not exist, such as when a function is discontinuous, has a sharp corner, or a vertical tangent line. The instructor emphasizes the importance of understanding the underlying process of differentiation, regardless of the varying terminology used to describe it.
Takeaways
- 🧮 The derivative is defined as the limit of the difference quotient as h approaches zero, representing the slope of a tangent line at a specific point on a curve.
- 📈 The difference quotient, f(x + h) - f(x)/h, is used to approximate the slope of a secant line, which is the average rate of change over an interval.
- 🔍 When h approaches zero, the difference quotient transforms from representing the slope of a secant line to that of a tangent line, indicating an instantaneous rate of change.
- ✏️ Derivative notation is f'(x), and finding the derivative involves the same processes as finding the slope of the tangent line or the instantaneous rate of change.
- 📏 The derivative can be expressed as f'(x) = lim_{h to 0} (f(x + h) - f(x))/h, assuming the limit exists.
- 🔢 Calculating a derivative might require algebraic manipulation to simplify the difference quotient, making it possible to evaluate the limit as h approaches zero.
- 📚 If a function f is differentiable at x, it means the derivative f'(x) exists and the function is smooth (without breaks or sharp corners) at that point.
- 📖 Differentiability implies a continuous function without sharp corners or vertical tangent lines; the derivative does not exist in these cases.
- 🌐 The derivative at a specific point, such as f'(3), is the slope of the tangent line at that point, providing a concrete value for real-world applications.
- 🧑🏫 The process of differentiation, or finding derivatives, is fundamental in calculus for understanding how functions change, which is essential for fields ranging from physics to economics.
Q & A
What is the difference quotient and how does it relate to the concept of a derivative?
-The difference quotient is a formula that represents the slope of a secant line between two points on a curve. It is defined as (f(x + h) - f(x)) / h, where 'h' is the distance between the two points. As 'h' approaches zero, the difference quotient approximates the slope of the tangent line at a point on the curve, which is the instantaneous rate of change at that point. This concept is the foundation for the definition of the derivative.
How is the limit of the difference quotient as h approaches zero connected to the slope of a tangent line?
-When the difference quotient's 'h' approaches zero, the formula no longer represents the average rate of change over an interval but instead the instantaneous rate of change at a specific point. This is because as 'h' gets smaller, the secant line becomes closer and closer to the tangent line at the point x, thus the limit of the difference quotient as h approaches zero gives us the slope of the tangent line at that point.
What is the notation used to represent the derivative of a function f with respect to x?
-The derivative of a function f with respect to x is represented by the notation f'(x) or df/dx, which is read as 'f prime of x' or 'the derivative of f with respect to x'.
What does it mean for a function to be differentiable at a point x?
-A function is said to be differentiable at a point x if the derivative exists at that point. This means that the function has a well-defined slope or rate of change at that point, and it is continuous and smooth (without sharp corners or vertical tangents) at x.
What is the process called that produces the derivative of a function?
-The process of finding the derivative of a function is called differentiation. It involves finding the limit of the difference quotient as the interval between points approaches zero, which results in the instantaneous rate of change of the function at a given point.
How do you find the equation of the tangent line at a specific point on a curve?
-To find the equation of the tangent line at a specific point on a curve, you first find the derivative of the function, which gives you the slope of the tangent line. Then, you find the coordinates of the point on the curve by substituting the x-value of the point into the original function. Using the slope and the point, you can use the point-slope form of a linear equation to find the equation of the tangent line.
What are the conditions under which a function is not differentiable?
-A function is not differentiable if it is discontinuous, has a sharp corner, or has a vertical tangent line at a particular point. These conditions mean that the limit of the difference quotient does not exist at those points, and thus the derivative cannot be found.
What is the geometric interpretation of the derivative of a quadratic function, such as f(x) = x^2 + 1?
-The geometric interpretation of the derivative of a quadratic function, such as f(x) = x^2 + 1, is the slope of the tangent line to the curve of the function at any given point. For the given function, the derivative f'(x) = 2x represents the slope of the tangent line at any x-value on the curve.
How does the process of finding the derivative of a rational function differ from that of a polynomial function?
-The process of finding the derivative of a rational function (a function with a fraction) involves finding a common denominator and simplifying, which can be more complex due to the presence of fractions. For polynomial functions, the process is typically simpler as it involves only basic algebraic operations. However, the conceptual approach to finding the derivative, which involves limits and difference quotients, remains the same.
What is the derivative of the function f(x) = 4/x, and what does it represent?
-The derivative of the function f(x) = 4/x is f'(x) = -4/x^2. This derivative represents the slope of the tangent line to the curve of the function at any point x, indicating the instantaneous rate of change of the function at that point.
Can you find the derivative of a function if the function has a discontinuity at a certain point?
-No, you cannot find the derivative of a function at a point where the function has a discontinuity. A derivative requires the existence of a limit, and a discontinuity implies that the limit does not exist at that point.
What is the significance of the instantaneous rate of change in calculus?
-The instantaneous rate of change, as represented by the derivative, is significant in calculus as it allows for the study of how quantities change at a specific instant rather than over an interval. This concept is fundamental to analyzing the behavior of functions, including understanding local maxima and minima, concavity, and the tangent behavior of curves.
Outlines
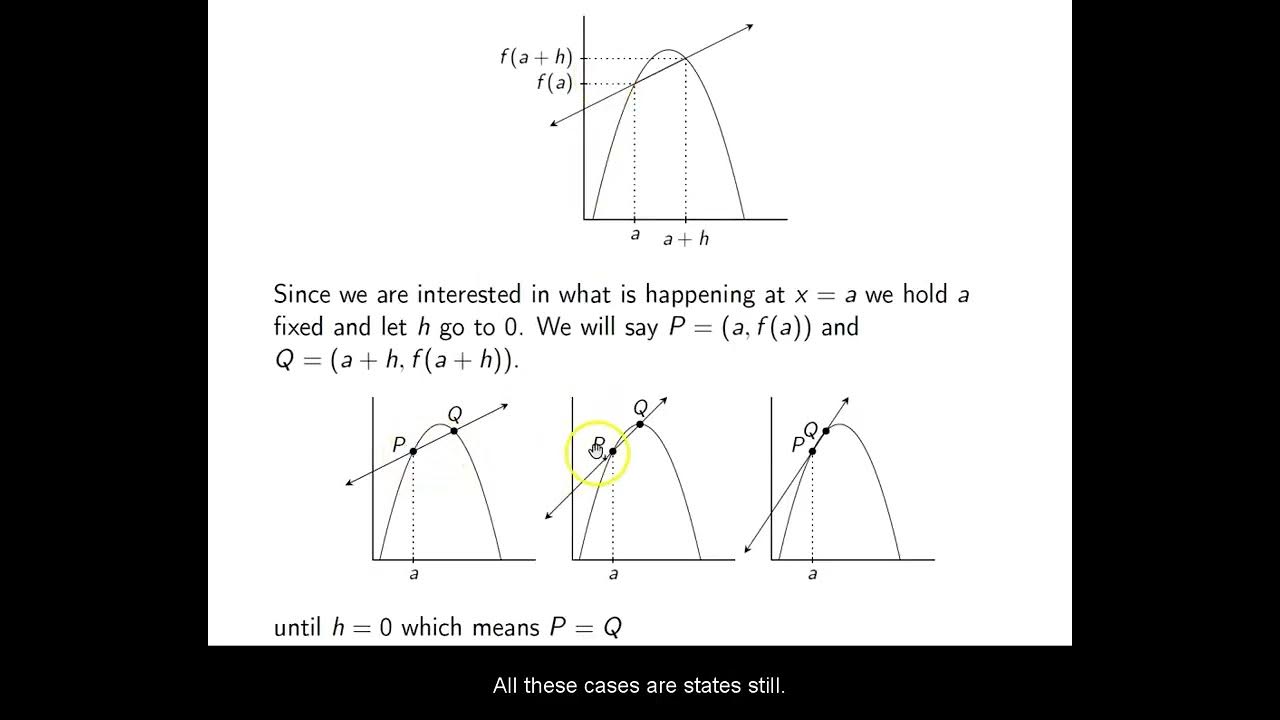
📘 Definition of the Derivative and Recap
The video begins with an introduction to the concept of the derivative, following a previous discussion on the difference quotient and its limit as H approaches zero. The difference quotient is a method to find the slope of a secant line over an interval, which is an approximation of the rate of change of a curve. The limit of the difference quotient as H approaches zero gives the slope of the tangent line at a point, representing the instantaneous rate of change. The derivative is defined as this limit and is denoted as F'(X). The video emphasizes that finding a derivative is essentially finding the slope of a tangent line or the instantaneous rate of change, with different terminologies used interchangeably.
📔 Derivative of a Quadratic Function
The second paragraph provides an example of finding the derivative of a quadratic function, f(x) = x^2 + 1. The process involves simplifying the difference quotient and then evaluating the limit as H approaches zero to find the derivative, F'(x) = 2x. The video also interprets the derivative at a specific point, x = 3, by substituting the value into the derivative to find the slope of the tangent line at that point, which is F'(3) = 6.
📒 Derivative of a Rational Function
The third paragraph focuses on finding the derivative of a rational function, f(x) = 4/x. The process includes simplifying the difference quotient and finding the limit as H approaches zero. After algebraic manipulation, the derivative is found to be F'(x) = -4/(x^2). The video also explains that this derivative can be used to find the slope of the tangent line at any point on the curve of the given function.
📕 Derivative of a Square Root Function
The fourth paragraph deals with finding the derivative of a square root function, specifically f(x) = √(x + 40). The process involves rationalizing the numerator to simplify the difference quotient and then evaluating the limit as H approaches zero. The correct derivative is found to be F'(x) = 1/(2√(x)). The video also demonstrates how to find the slope of the tangent line at a specific point, x = 4, and how to write the equation of the tangent line at that point.
📔 Conditions for Existence of a Derivative
The fifth paragraph discusses the conditions under which a derivative exists. A function f is differentiable if it is continuous, smooth (without sharp corners), and does not have a vertical tangent line. Discontinuities, sharp corners, and vertical tangents are conditions that prevent a function from being differentiable. The video provides visual examples of these conditions and explains why they disqualify a function from having a derivative at certain points.
📘 Summary of Derivative Finding Process
The final paragraph summarizes the process of finding derivatives, emphasizing that it involves determining the slope of a tangent line, which is the instantaneous rate of change. The video encourages viewers to apply what they have learned to their assignments and to reach out with any questions. It concludes with a sign-off until the next lesson.
Mindmap
Keywords
💡Derivative
💡Difference Quotient
💡Limit
💡Slope of a Tangent Line
💡Instantaneous Rate of Change
💡Function
💡Differentiable
💡Continuous Function
💡Algebraic Manipulation
💡Point-Slope Form
💡Rational Function
Highlights
The definition of the derivative is introduced as the limit of the difference quotient as h approaches 0
Derivatives represent the instantaneous rate of change or slope of a tangent line at a point
The difference quotient is a version of slope used to find the average rate of change over a closed interval
The derivative (f'(x)) is the limit of the difference quotient as the interval size (h) approaches 0
Differentiable functions must be continuous, smooth (no sharp corners), and have non-vertical tangent lines
The process of finding a derivative is called differentiation
f(x) is differentiable at x if f'(x) exists
The derivative of f(x) = x^2 + 1 is found to be f'(x) = 2x
The slope of the tangent line at a specific point x=a can be found by plugging a into the derivative
The derivative of f(x) = 4/x is found to be f'(x) = -4/x^2 using algebraic manipulation
The slope of the tangent line at x=4 for f(x) = sqrt(x) + 40 is found to be 1/(2*sqrt(4)) = 1/4
The equation of the tangent line at x=4 for f(x) = sqrt(x) + 40 is derived using point-slope form
Functions are not differentiable at points of discontinuity, sharp corners, or vertical tangents
Derivatives give the slope of a tangent line, which is the instantaneous rate of change of a function at a point
Differentiating a function involves finding its derivative, which represents the slope of the tangent line
The algebraic manipulation involved in simplifying the difference quotient can be challenging
Different wordings like 'find the slope of the tangent line' or 'find the instantaneous rate of change' all refer to finding the derivative
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: