AP Calculus AB Crash Course Day 9 - Limits, Continuity, and Differentiation

Dr. Steve Warner
26 Feb 202211:58
EducationalLearning
32 Likes 10 Comments

TLDRThe video script delves into various mathematical concepts and problem-solving techniques. It begins with the calculation of a limit involving trigonometric functions, factoring out terms and applying the Pythagorean identity to simplify the expression. The script then explores the properties of derivatives, differentiability, and continuity, using a function k as an example to illustrate these concepts. It challenges viewers to find a counterexample for a given statement about continuity. The discussion continues with limits at vertical asymptotes, using the behavior of functions near specific points to determine the nature of the limit. The script also addresses removable discontinuities in functions and how to redefine a function to make it continuous at a point of discontinuity. It concludes with an application of the fundamental theorem of calculus to approximate derivatives and analyze the concavity and monotonicity of a function defined by an integral. The summary provides a comprehensive overview of the mathematical content covered in the script, highlighting the key topics and the logical flow of the discussion.

Takeaways
  • 📚 **Factoring Out Common Terms**: In limits, factoring out common terms like '3 tan x' can simplify expressions, making them easier to evaluate.
  • 🔍 **Using Trigonometric Identities**: The Pythagorean identity (1 - cos²x = sin²x) is used to transform expressions, which can lead to more manageable forms for limits.
  • 📈 **Splitting Limits**: Limits can be split into separate components, which can then be evaluated individually, often simplifying the overall process.
  • 🔢 **Basic Limit Rules**: Knowing basic limit rules, such as lim (tan x / x) as x approaches 0 being 1, is crucial for solving complex limits.
  • 🔁 **L'Hôpital's Rule**: When basic limit rules are not enough, L'Hôpital's rule can be applied to find limits by taking derivatives of the numerator and denominator.
  • 📉 **Identifying Asymptotes**: Recognizing vertical asymptotes from the form of a function, such as 1/0, helps to determine that a limit does not exist or is infinite.
  • 🔮 **Sign Charts**: For limits involving asymptotes, sign charts can help determine the behavior of the function near the asymptote, indicating whether the limit is positive or negative infinity.
  • 🔧 **Removable Discontinuities**: Functions with removable discontinuities can be fixed by redefining the function at the point of discontinuity, making it continuous.
  • 📐 **Factoring Functions**: Factoring the numerator and denominator of a rational function can reveal simplifications and potential discontinuities.
  • ⏱️ **Approximating Derivatives**: Given data points, the derivative of a function at a specific point can be approximated using the secant line between two points.
  • 📊 **Understanding Graphs**: The behavior of a function's graph, such as increasing or concave up, can be determined by analyzing the sign of the first and second derivatives.
  • 📋 **Fundamental Theorem of Calculus**: The theorem is used to find antiderivatives and can also be applied to find derivative values at specific points from a given function's graph.
Q & A

  • What is the limit as x approaches zero of the expression (3tan(x) - 3cos^2(x)) / (x^3)?

    -The limit can be simplified by factoring out 3tan(x), which leaves us with (1 - cos^2(x)) / x^2, which is equivalent to sin^2(x) / x^2. Using the basic limit rules, we find that the limit as x approaches zero of tan(x)/x is 1 and the limit as x approaches zero of sin(x)/x is also 1. Therefore, the original limit is 3 * 1 * 1^2, which equals 3.

  • What is the value of the derivative of function k at x=7, given that the limit as h approaches zero of (k(7+h) - k(7)) / h equals 12?

    -The given limit is the definition of the derivative of k at x=7. Therefore, the derivative of k at x=7, denoted as k'(7), is 12.

  • Is the function k differentiable at x=7 based on the given limit condition?

    -Yes, since k'(7) exists and is equal to 12, the function k is differentiable at x=7.

  • Is the function k continuous at x=7 given that its derivative at that point is 12?

    -Yes, differentiability at a point implies continuity at that point. Therefore, k is continuous at x=7.

  • Can we conclude that the derivative of k, denoted as k', is continuous at x=7 from the given information?

    -No, the given information only states that k'(7) = 12. There is no information about the continuity of k' at x=7. One could construct a counterexample where k' is not continuous at x=7 despite k'(7) existing.

  • What is the limit as x approaches 11 of the function x / (x - 11)^2?

    -The function has a vertical asymptote at x=11, which means the limit does not exist in the traditional sense. However, as x approaches 11 from either side, the function values increase without bound, so the limit is positive infinity.

  • How can you determine the intervals on which the graph of the function f, defined by the given graph, is both increasing and concave up?

    -The graph of f is increasing where f' (the derivative of f) is greater than zero, and it is concave up where f'' (the second derivative of f) is greater than zero. From the graph, we can see that f is increasing and concave up on the intervals x between 2 and 4 and x between 6 and 8.

  • What is the value of f'(-4) for the function f defined on the closed interval [-5, 8] with the given graph?

    -By the Fundamental Theorem of Calculus, f'(-4) is equal to the value of the original function f at x = -4. From the graph, f(-4) is 2, so f'(-4) is 2.

  • Does f''(-4) exist, and if so, what is its value?

    -f''(-4) corresponds to the derivative of f' at x = -4, which is the slope of the tangent line to the graph of f at that point. Since the graph has a defined slope at x = -4, f''(-4) exists and its value is -1, the slope of the line segment at that point.

  • What is the value of f'(6) for the function f defined by the given graph?

    -At x = 6, the graph of f has a cusp, which means it is not differentiable at that point. Therefore, f'(6) does not exist.

  • What is the value of f''(6) for the function f defined by the given graph?

    -Since f'(6) does not exist due to the cusp at x = 6, f''(6), which is the derivative of f', also does not exist.

  • Given the function g(x) = (x^2 + 4x - 12) / (x^2 + 3x - 10), what value of x creates a removable discontinuity?

    -The function g(x) has a removable discontinuity at x = 2, as both the numerator and the denominator share a common factor of (x - 2), which can be canceled out for all x not equal to 2.

  • How can we define a continuous function G(x) that agrees with g(x) for all x, and is continuous at x = 2?

    -We can define G(x) to be equal to g(x) for all x not equal to 2, and set G(2) to be the limit of g(x) as x approaches 2, which is 8/7. This ensures that G(x) is continuous at x = 2 and agrees with g(x) elsewhere.

  • How can we approximate g'(1.5) using the given data points in the table?

    -We can approximate g'(1.5) by using the secant line through the points (1, g(1)) and (2, g(2)). The slope of this secant line is (g(2) - g(1)) / (2 - 1), which gives us an approximation of the derivative at 1.5.

  • What are the units of measure for g'(1.5) based on the given data?

    -Since t is measured in minutes and g(t) is measured in pints, the units for g'(1.5) would be pints per minute.

Outlines
00:00
📚 Calculus Limit Evaluation

This paragraph discusses the process of evaluating a complex limit as x approaches zero. The expression given is 'three tan x minus three cosine squared x, divided by x cubed'. The approach involves factoring out '3 tan x' and then using the Pythagorean identity to simplify the expression further. The limit is then split into smaller parts and evaluated using basic limit rules. The limits of 'tan x over x' and 'sine x over x' as x approaches zero are identified as one, and these are squared and multiplied by three to arrive at the final answer of three. The paragraph also touches on using L'Hôpital's rule to find these limits if they were not already known.

05:01
🔍 Derivative and Continuity Analysis

The second paragraph deals with a function 'k' and its derivative at a specific point. The limit expression given is used to determine that 'k prime of 7' equals 12, which is the definition of the derivative of 'k' evaluated at 7. This implies that 'k' is differentiable at 7, and therefore, continuous at 7 as well. However, the paragraph points out that there is no information given about the continuity of 'k prime' at x equals 7, leading to a challenge for the reader to find a counterexample where 'k prime' exists but is not continuous at x equals 7. The paragraph concludes with the evaluation of another limit as x approaches 11, identifying a vertical asymptote and concluding that the limit is positive infinity.

10:07
🔧 Function Modification for Continuity

The third paragraph focuses on a function 'g' with a removable discontinuity at x equals 'c'. The function 'g' is defined as 'x squared plus 4x minus 12' divided by 'x squared plus 3x minus 10'. By factoring the numerator and denominator, it is shown that 'g' simplifies to 'x plus 6' over 'x plus 5', except when x equals 2. The limit as x approaches 2 is calculated to be '8/7', confirming the removable discontinuity at x equals 2. The paragraph then instructs to redefine the function 'G' to be continuous at x equals 2 by assigning the limit value at that point. Lastly, the paragraph presents a data table and asks to approximate the derivative 'g prime of 1.5' using the secant line method, which is calculated to be '2.3 pints per minute'.

📈 Function Graph Analysis and Derivatives

The final paragraph introduces a function 'f' defined on a closed interval, with a graph consisting of three line segments and a semicircle. The task is to find the value of 'f prime' and 'f double prime' at specific points, using the Fundamental Theorem of Calculus. The value of 'f prime' at negative 4 is determined to be 2, and 'f double prime' at the same point is -1, based on the slope of the line segment. However, 'f prime' at 6 is 0, and 'f double prime' does not exist at 6 due to a cusp at that point. The paragraph concludes with an inquiry about the intervals where the graph of 'f' is both increasing and concave up, which are identified as between x equals 2 and 4, and between x equals 6 and 8.

Mindmap
Keywords
💡Limit
A limit in calculus is a value that a function or sequence approaches as the input (or index) approaches some value. In the video, the concept of limits is central to understanding how certain mathematical expressions behave as they approach specific points, such as when x approaches zero in the first example. Limits are used to define continuity, derivatives, and integrals.
💡Factoring
Factoring is a mathematical technique used to break down an expression into a product of simpler expressions. In the video, the presenter factors out '3 tan x' from the given expression, which simplifies the process of finding the limit and demonstrates a common technique in calculus for dealing with complex functions.
💡Pythagorean Identity
The Pythagorean identity is a fundamental trigonometric relationship stating that sin²θ + cos²θ = 1. In the video, the identity is used to transform 1 - cos²x into sin²x, which is a key step in simplifying the expression for the limit calculation.
💡Differentiability
Differentiability refers to the property of a function that can be represented by a derivative at a given point. In the video, it is mentioned that if a function's derivative exists at a point, then the function is differentiable at that point. This is important for the function k, where it is stated that k'(7) = 12, indicating differentiability at x = 7.
💡Asymptote
An asymptote is a line that a function approaches but never actually reaches. In the video, the presenter discusses a vertical asymptote at x = 11 for the function x/(x - 11)², which means the function's values get infinitely large as x approaches 11.
💡Continuity
Continuity in mathematics means that a function is unbroken and has no gaps at a given point. In the video, it is established that if a function is differentiable at a point, it is also continuous at that point, as is the case with the function k at x = 7.
💡Removable Discontinuity
A removable discontinuity is a type of discontinuity in a function that can be 'removed' by redefining the function's value at that point. In the video, the function g(x) has a removable discontinuity at x = 2, which is resolved by defining G(x) to be equal to g(x) for x ≠ 2 and to be 8/7 at x = 2.
💡Secant Line
A secant line is a straight line that intersects a function at two distinct points. In the video, the presenter uses the secant line passing through the points (1, 2.2) and (2, 4.5) to approximate the derivative of g at 1.5, which is a method for estimating derivatives when exact values are not readily available.
💡Derivative
The derivative of a function at a certain point is the rate at which the function's value changes with respect to changes in its input. In the video, the derivative of k at x = 7 is given as 12, and the concept is also used to approximate g'(1.5) using the secant line method.
💡Integral
An integral is a mathematical concept that represents the area under a curve, or the accumulation of a quantity. In the video, the function capital f is defined as the integral from 2 to x of the function f(t) dt, which is a way to construct an antiderivative of the function f.
💡Concavity
Concavity refers to the curvature of a function. A function is said to be concave up if its graph curves in a shape similar to an upside-down U. In the video, the presenter determines the intervals where the function f is both increasing and concave up, which is identified by the function f being above the x-axis and increasing.
Highlights

The limit as x approaches 0 of (3tan(x) - 3cos^2(x)) / (x^3) can be simplified by factoring out 3tan(x) and using the Pythagorean identity.

The limit can be split into the product of two limits: lim (tan(x)/x) and lim (sin^2(x)/x^2), both of which approach 1 as x approaches 0.

Using l'Hopital's rule, the limits lim (tan(x)/x) and lim (sin(x)/x) can be found by taking derivatives of the numerator and denominator.

The limit as x approaches 11 of (x / (x - 11)^2) is positive infinity due to the vertical asymptote at x = 11.

To find the limit, test points to the left and right of the asymptote to determine the sign of the function as x approaches the asymptote.

The function g(x) = (x^2 + 4x - 12) / (x^2 + 3x - 10) has a removable discontinuity at x = 2. Factor the numerator and denominator to simplify.

The limit as x approaches 2 of g(x) is 8/7, which can be used to define a continuous function G(x) that agrees with g(x) except at x = 2.

To approximate the derivative g'(1.5), use the secant line between the points (1, 2.2) and (2, 4.5). The result is 2.3 pints per minute.

The function f(x) is defined on the interval [-5, 8] and consists of three line segments and a semicircle. Find its antiderivative F(x).

F'(x) is equal to f(x) by the fundamental theorem of calculus. Compute F'(-4) and F'(6) using the graph of f(x).

F''(x) is equal to f'(x), which can be found by looking at the slope of the line segments in the graph of f(x).

The graph of F(x) is concave up on the intervals (2, 4) and (6, 8) since f(x) is increasing on those intervals.

The function f(x) is increasing and concave up on the intervals (2, 4) and (6, 8) based on the graph and the properties of f(x) and F(x).

The transcript provides a detailed step-by-step analysis of limits, derivatives, and antiderivatives using algebraic manipulation and calculus concepts.

The Pythagorean identity and l'Hopital's rule are used to simplify and evaluate limits involving trigonometric functions.

The concept of removable discontinuity is introduced and illustrated using the function g(x) = (x^2 + 4x - 12) / (x^2 + 3x - 10).

The secant line method is used to approximate derivatives at specific points using nearby data points.

The fundamental theorem of calculus is applied to find antiderivatives and derivatives of piecewise functions defined by graphs.

The intervals where a function is increasing and concave up can be determined by analyzing the graph and using calculus concepts.

Transcripts

Browse More Related Video

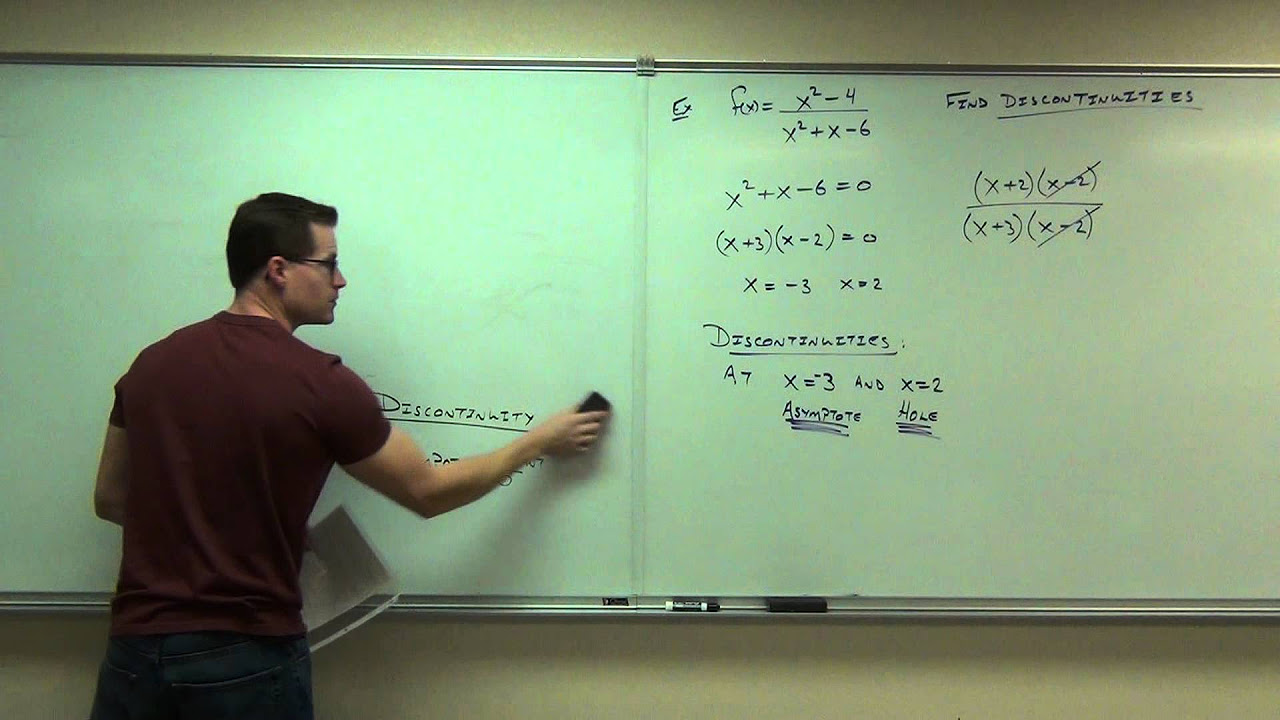
Calculus 1 Lecture 1.4: Continuity of Functions

AP Calculus AB Crash Course Day 3 - Limits, Continuity, and Differentiation

1.2 - Algebraic Limits and Continuity

BusCalc 16 Continuity and Differentiability

AP Calculus BC Practice Exam 2012 - Calculator Multiple Choice questions 76-92

Business Calculus - Math 1329 - Section 1.5 (and 1.6) - Limits and Continuity

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusLimitsDerivativesFunction AnalysisTrigonometric LimitsDifferentiabilityAsymptotesContinuityIntegral CalculusReal-world MathEducational ContentMathematical Concepts