k12.org exercise: Standard normal distribution and the empirical | Khan Academy

Khan Academy
8 Jan 201008:15
EducationalLearning
32 Likes 10 Comments

TLDRThis video script from ck12.org's AP Statistics FlexBook covers problem number 4, which involves ordering elements of a standard normal distribution. The presenter explains how to use the empirical rule (68-95-99.7 rule) to estimate percentages of data below certain z-scores, such as 1 and -1, and identifies the mean and standard deviation of the distribution. The script concludes with ordering the calculated values, clarifying potential ambiguity between percentages and their decimal equivalents.

Takeaways
  • πŸ“š The video discusses problem number 4 from the Normal Distribution chapter of an AP Statistics FlexBook available for free on ck12.org.
  • πŸ“ˆ The script explains how to order different values from a standard normal distribution (mean = 0, standard deviation = 1) from smallest to largest.
  • πŸ“Š A standard normal distribution is characterized by a bell curve with the mean at 0 and a standard deviation of 1, dividing the curve into symmetrical halves.
  • πŸ”’ The empirical rule, also known as the 68-95-99.7 rule, is introduced as a method to estimate percentages of data within certain standard deviations from the mean.
  • 🧠 The empirical rule states that approximately 68% of data falls within one standard deviation of the mean, 95% within two standard deviations, and 99.7% within three standard deviations.
  • πŸ“‰ The script calculates the percentage of data below 1 as 84% using the empirical rule, which includes the area within one standard deviation (68%) plus the left tail (16%).
  • πŸ“Œ The percentage of data below -1 is given as 16%, which corresponds to the left tail of the distribution.
  • 🎯 The mean of a standard normal distribution is defined as 0, and the standard deviation is 1, which are straightforward values in this context.
  • πŸ“Š The percentage of data above 2 is calculated to be 2.5%, derived from the empirical rule by subtracting the 95% within two standard deviations from 100%.
  • πŸ”„ The script highlights the importance of understanding the difference between percentages and their decimal equivalents when ordering values.
  • πŸ“ The final ordering of the values, considering their decimal equivalents, is c (0), e (0.025), b (0.16), a (0.84), and d (1), with a note on potential ambiguity in interpreting the question.
Q & A

  • What is the source of the problem discussed in the script?

    -The problem discussed in the script is from the Normal Distribution chapter of the AP Statistics FlexBook on ck12.org.

  • What does the speaker suggest to do with the problem?

    -The speaker suggests practicing the problem, which involves ordering various percentages and values related to a standard normal distribution.

  • What is a standard normal distribution?

    -A standard normal distribution is a normal distribution with a mean (mu) of 0 and a standard deviation of 1.

  • What is the empirical rule, also known as the 68-95-99.7 rule?

    -The empirical rule states that for a standard normal distribution, approximately 68% of the data falls within one standard deviation of the mean, 95% within two standard deviations, and 99.7% within three standard deviations.

  • How much of the data is below the value of 1 in a standard normal distribution according to the empirical rule?

    -According to the empirical rule, 84% of the data is below the value of 1 in a standard normal distribution.

  • What percentage of the data is below the value of -1 in a standard normal distribution?

    -16% of the data is below the value of -1 in a standard normal distribution.

  • What is the mean of a standard normal distribution?

    -The mean of a standard normal distribution is 0 by definition.

  • What is the standard deviation of a standard normal distribution?

    -The standard deviation of a standard normal distribution is 1 by definition.

  • What percentage of the data is above the value of 2 in a standard normal distribution?

    -2.5% of the data is above the value of 2 in a standard normal distribution.

  • How should one interpret the percentages given in the problem when ordering them from smallest to largest?

    -One should interpret the percentages as decimals when ordering them from smallest to largest, as the ambiguity arises from whether to consider the percentage value or the fraction of data.

  • What is the correct order of the values from smallest to largest based on the script?

    -The correct order, considering the values as decimals, is c (0), e (0.025), b (0.16), a (0.84), and d (1).

Outlines
00:00
πŸ“š Understanding the Standard Normal Distribution

This paragraph introduces problem number 4 from the AP Statistics chapter on the Normal Distribution found on ck12.org's FlexBook. The speaker encourages visiting the site to download the material for free. The problem involves ordering various elements related to a standard normal distribution from smallest to largest. The speaker explains the characteristics of a standard normal distribution, where the mean (mu) is 0 and the standard deviation is 1. A visual representation of the bell curve is described, illustrating the mean and standard deviations. The empirical rule, also known as the 68-95-99.7 rule, is introduced as a tool for estimating percentages of data within certain intervals around the mean. Using this rule, the speaker calculates the percentage of data below 1 and below -1, identifying these as 84% and 16% respectively. The mean and standard deviation of the standard normal distribution are also stated as 0 and 1, completing the ordering task.

05:01
πŸ“‰ Applying the Empirical Rule to Order Data Percentages

The second paragraph continues the discussion on the standard normal distribution, focusing on the application of the empirical rule to determine the percentage of data above 2 standard deviations from the mean. The speaker uses the 68-95-99.7 rule to deduce that 95% of the data lies within 2 standard deviations of the mean, leaving 5% in the tails. The symmetry of the normal distribution is highlighted to conclude that 2.5% of the data is above 2 standard deviations. The paragraph then addresses the ambiguity in the ordering task, whether to use percentages or their decimal equivalents. The speaker opts for the decimal interpretation, ordering the elements as follows: the mean (0), the percentage of data above 2 standard deviations (0.025), the percentage of data below -1 (0.16), the percentage of data below 1 (0.84), and finally the standard deviation (1). The speaker suggests clarifying such ambiguities with a teacher if encountered in an exam setting.

Mindmap
Keywords
πŸ’‘Normal Distribution
Normal distribution, also known as Gaussian distribution, is a probability distribution that is characterized by its symmetrical bell-shaped curve. In the context of the video, it is essential for understanding the problem-solving process as the script discusses ordering various data points in relation to a standard normal distribution where the mean (ΞΌ) is 0 and the standard deviation (Οƒ) is 1.
πŸ’‘Empirical Rule
The Empirical Rule, often remembered as the 68-95-99.7 rule, is a quick way to estimate where data falls in a normal distribution without using a calculator or table. The video uses this rule to estimate the percentage of data within one, two, and three standard deviations from the mean, which is central to solving the problem presented.
πŸ’‘Standard Deviation
Standard deviation is a measure of the amount of variation or dispersion in a set of values. In the video, the standard deviation is set to 1 for the standard normal distribution, which is used to determine the spread of data around the mean and to calculate the percentages of data within certain intervals.
πŸ’‘Mean
The mean, often denoted by the Greek letter mu (ΞΌ), is the average value of a data set. In the script, the mean of the standard normal distribution is defined as 0, which is a fundamental aspect of the distribution being discussed and is used as a reference point for data ordering.
πŸ’‘Percentage of Data
The script frequently refers to the 'percentage of data' in relation to the normal distribution curve, which is a way to describe the proportion of data points that fall within a certain range. This concept is crucial for solving the problem as it involves calculating and comparing these percentages.
πŸ’‘Symmetric
The term 'symmetric' describes a property of the normal distribution where the left and right sides of the distribution curve are mirror images of each other. The video script uses this property to simplify calculations by stating that the areas to the left and right of the mean are equal.
πŸ’‘Bell Curve
The bell curve is the visual representation of the normal distribution, characterized by its symmetrical, bell-shaped form. In the video, the bell curve is drawn to illustrate the distribution and to visually aid in understanding the problem-solving process.
πŸ’‘AP Statistics
AP Statistics refers to a course and exam offered by the College Board for high school students to earn college credit. The video script mentions a problem from the AP Statistics chapter, indicating that the content is geared towards students preparing for this exam.
πŸ’‘Ordering Data
The task in the video script involves ordering various data points from smallest to largest, which is a common problem-solving technique in statistics. The script explains how to use the empirical rule to determine the order of data percentages in relation to the standard normal distribution.
πŸ’‘Fraction of Data
The 'fraction of data' is another way to express the proportion of data points within a certain range, often used interchangeably with 'percentage of data' in the script. The video discusses the importance of understanding both representations when ordering data.
πŸ’‘Estimate
The script mentions the ability to estimate percentages of data within certain intervals of the normal distribution, which is a skill useful for quick calculations without the need for tools. This is part of the practical application of the empirical rule discussed in the video.
Highlights

Introduction to problem number 4 from the Normal Distribution chapter on AP Statistics.

Mention of ck12.org's FlexBook as a free resource for AP Statistics.

Explanation of the standard normal distribution with mean (mu) equal to 0 and standard deviation equal to 1.

Illustration of the standard normal distribution curve and its characteristics.

Use of the empirical rule (68, 95, 99.7 rule) to estimate percentages in a normal distribution.

Calculation of the percentage of data below 1 in a standard normal distribution as 84%.

Determination that the percentage of data below -1 is 16% using the empirical rule.

Identification of the mean of a standard normal distribution as 0 by definition.

Clarification that the standard deviation of a standard normal distribution is 1 by definition.

Application of the empirical rule to find the percentage of data above 2 as 2.5%.

Discussion on the ambiguity of interpreting percentages versus fractions in the context of the problem.

Ordering the calculated values from smallest to largest based on decimal representation.

Final ordering of the values: c (mean), e (above 2), b (below -1), a (below 1), d (standard deviation).

Advice on clarifying ambiguous questions with a teacher or exam proctor.

Emphasis on the importance of practice with the empirical rule for estimating percentages.

Highlight of the practical application of the standard normal distribution in statistics.

Transcripts

Browse More Related Video

ck12.org normal distribution problems: Empirical rule | Probability and Statistics | Khan Academy

Empirical Rule (68-95-99.7) for Normal Distributions

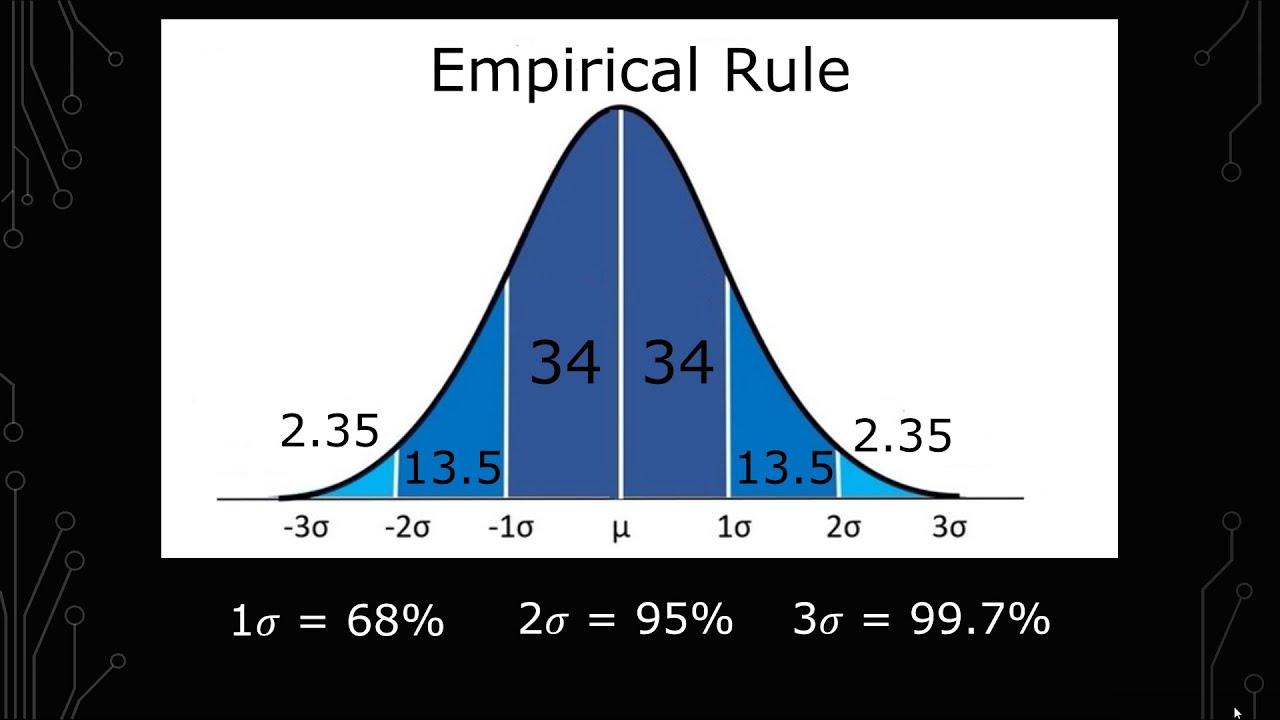
How to Use the Empirical Rule with Examples

ck12.org normal distribution problems: z-score | Probability and Statistics | Khan Academy

Standard Normal Distribution Tables, Z Scores, Probability & Empirical Rule - Stats

Elementary Statistics - Chapter 6 Normal Probability Distributions Part 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Empirical RuleStandard NormalAP StatisticsEducational ScriptData AnalysisStatistical PracticeNormal DistributionProbability TheoryCK12 ResourcesExam Preparation