Electric power | Circuits | Physics | Khan Academy
TLDRThe video script explains the phenomenon of a resistor heating up when current flows through it. It begins by discussing the conceptual reason behind the heating, which is the loss of electrical potential energy of charges moving through the resistor. This energy is not converted into kinetic energy but rather into thermal energy, causing the resistor to heat up. The script then introduces the formula for calculating the power (energy per time) dissipated by the resistor, which is the product of the current, voltage, and resistance. It also presents alternative expressions for power based on Ohm's law, offering different formulas for calculating power depending on the known variables. The explanation is clear, engaging, and informative, providing a solid understanding of the energy transformation in electrical circuits.
Takeaways
- 🔌 When current flows through a resistor, it generates heat due to the resistance causing the electrical energy to be converted into thermal energy.
- 💡 The reason a resistor heats up is that the electrons (negative charges) moving through it strike atoms and molecules, transferring energy and increasing the temperature of the resistor.
- 📐 The voltage difference across a resistor is the key factor in understanding how much electrical potential energy is converted into heat.
- 🔋 The loss of potential energy of the charges (electrons) flowing through the resistor is equal to the gain in thermal energy of the resistor.
- 🔍 The power (rate of energy transfer) can be calculated using the formula Power = Change in Electrical Potential Energy per Time.
- 🌡️ The heating of the resistor is a result of the work done by the electric current, which can be quantified using the power formula.
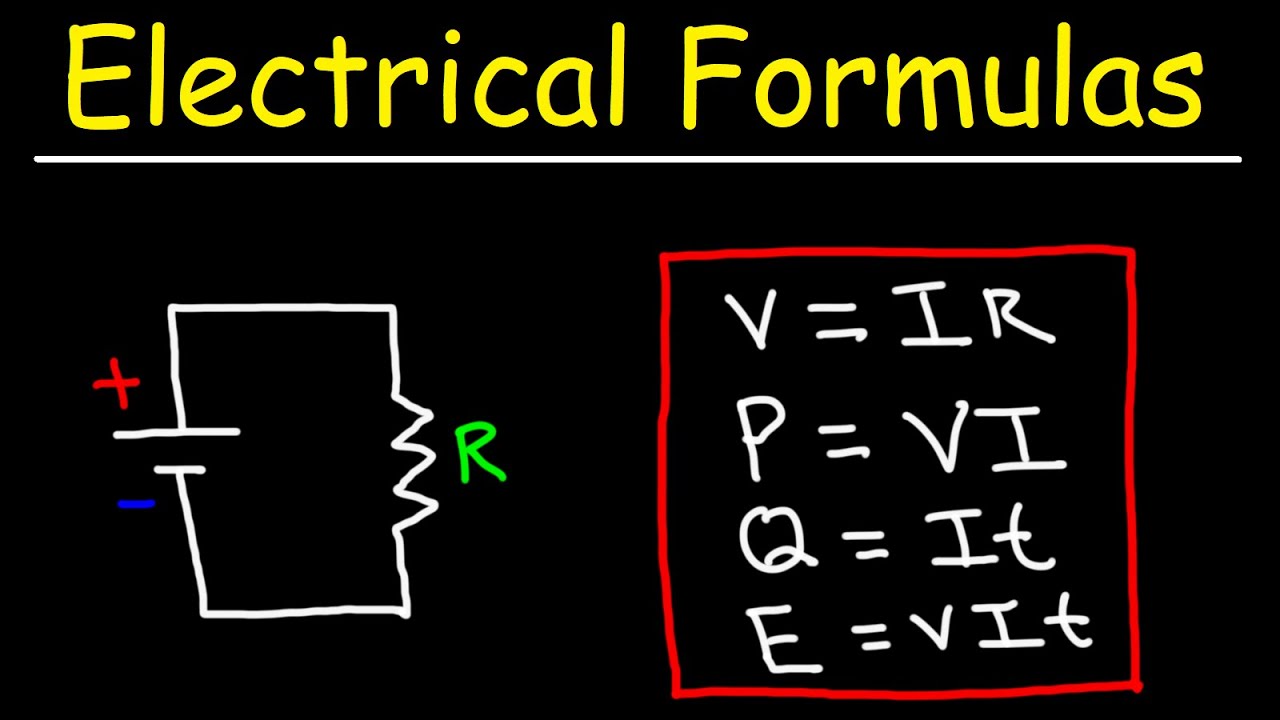
- 📈 The power consumed by a resistor can be calculated in various ways: P = IV (current times voltage), P = I²R (current squared times resistance), or P = V²/R (voltage squared divided by resistance).
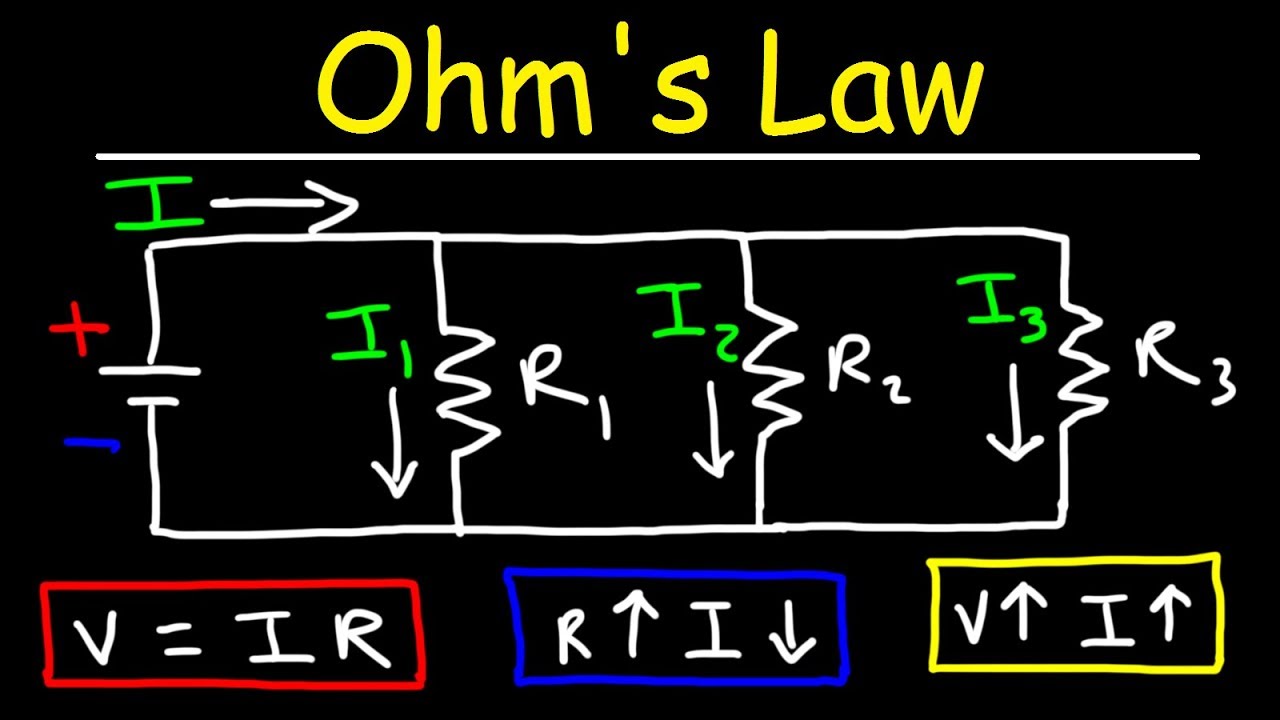
- 🔧 Ohm's law (V = IR) is fundamental in deriving the power formulas, as it relates voltage, current, and resistance in a simple electrical circuit.
- 🔄 The concept of energy conservation is central to understanding the heating effect of resistors; the potential energy lost by electrons is transformed into heat energy within the resistor.
- 🔧 The choice of power formula depends on the known quantities in a given situation, allowing for flexibility in problem-solving.
- 🔌 Understanding the relationship between electrical potential energy and thermal energy is crucial for analyzing the behavior of resistors and other electrical components.
Q & A
Why does a resistor heat up when current flows through it?
-A resistor heats up due to the conversion of electrical potential energy into thermal energy as charges flow through it. Charges moving from a region of high potential to a region of low potential lose potential energy, which is then transferred to the atoms and molecules of the resistor, causing it to heat up.
How can we calculate the amount of heat generated by a resistor over a given time?
-The amount of heat generated can be calculated using the formula for power, which is the product of the current (I) flowing through the resistor and the voltage (V) across it, P = IV, or using the resistance (R), P = I^2R or P = V^2/R. These formulas give the power in watts, which represents the energy converted per second.
What is the conceptual difference between potential energy and kinetic energy in the context of electrical charges flowing through a resistor?
-Potential energy is the energy a charge possesses due to its position in an electric field, while kinetic energy is the energy it has due to its motion. In a resistor, charges lose potential energy but do not gain kinetic energy proportionally; instead, this lost potential energy is converted into thermal energy, heating up the resistor.
Why can't the decrease in potential energy of charges flowing through a resistor be directly equated to an increase in kinetic energy?
-The decrease in potential energy cannot be directly equated to an increase in kinetic energy because the current on both sides of the resistor must be the same. This means that charges do not speed up as they pass through the resistor; instead, they transfer the lost potential energy to the resistor's atoms and molecules, causing it to heat up.
What is the relationship between the voltage across a resistor and the power dissipated by it?
-The power dissipated by a resistor is directly proportional to the square of the voltage across it, as given by the formula P = V^2/R. This means that for a given resistance, an increase in voltage across the resistor results in a greater amount of power being dissipated, leading to more heat generation.
How does Ohm's law relate to the calculation of power in electrical circuits?
-Ohm's law, which states that V = IR, relates to power calculations by providing a way to express the voltage across a resistor (V) in terms of the current (I) and resistance (R). This relationship allows us to calculate power using the formulas P = IV or P = I^2R when the resistance and current are known, or P = V^2/R when voltage and resistance are known.
What are the different forms of the power formula for electrical components?
-The power formula can be expressed in three main forms: P = IV (product of current and voltage), P = I^2R (current squared times resistance), and P = V^2/R (voltage squared divided by resistance). The choice of formula depends on which quantities are known for a given problem.
How does the concept of electric potential energy relate to the concept of electric potential?
-Electric potential energy is the energy per charge in an electric field, while electric potential is the amount of electric potential energy per unit charge. Essentially, electric potential energy (U) is the product of the charge (Q) and the electric potential (V) at a point, U = QV.
What happens to the charges as they move from a high potential region to a low potential region in a resistor?
-As charges move from a high potential region to a low potential region, they lose electric potential energy. This lost energy is not converted into kinetic energy of the charges but is instead transferred to the resistor's atoms and molecules, increasing their thermal energy and causing the resistor to heat up.
Why is the power formula for electrical components useful in understanding energy conversion?
-The power formula is useful because it quantifies the rate at which electrical potential energy is converted into other forms of energy, such as heat, light, or sound. This understanding is crucial for analyzing the efficiency and performance of electronic devices and systems.
How does the script's explanation of resistor heating relate to everyday phenomena like a light bulb glowing?
-The script's explanation of resistor heating is analogous to how a light bulb glows. In both cases, electrical potential energy is converted into thermal energy (and in the case of a light bulb, also into light energy). The resistance in the filament of the bulb heats up due to the current flowing through it, and this heat causes the filament to emit light.
Outlines
🔥 Understanding Resistor Heating
This section explains conceptually why a resistor heats up when current flows through it. The instructor begins by discussing the movement of positive charges through a wire, acknowledging that it's technically electrons moving in the opposite direction. The explanation progresses to how these charges moving from a high to a low electrical potential region lose electric potential energy, which then converts into thermal energy, heating up the resistor. This conversion occurs as the charges interact with the resistor's atomic structure, causing it to increase in temperature. The section sets the stage for calculating the exact amount of heat produced.
💡 Calculating Resistor Heat Generation
The second part introduces the concept of power as the rate of energy transfer to calculate how much energy the resistor gains as heat per unit time. It connects the loss of electric potential energy by the charges to the gain in thermal energy by the resistor. The instructor derives the formula for electrical power, P=IV, indicating the energy conversion rate into heat. Further, alternative forms of the power formula are derived using Ohm's Law, leading to P=I²R and P=V²/R, offering flexibility in calculations based on available data. This portion provides a comprehensive guide to quantifying thermal energy generated in resistors.
🎓 Practical Applications of Power Formulas
This final segment summarizes the electrical to thermal energy conversion process in resistors and presents three equivalent power formulas (P=IV, P=I²R, P=V²/R) for calculating the energy converted per second. It emphasizes the utility of these formulas in practical applications, allowing for flexibility based on known quantities. The instructor highlights that regardless of the chosen formula, the result remains accurate for determining the power used by a resistor, making it applicable to various electrical components and scenarios.
Mindmap
Keywords
💡Resistor
💡Current
💡Electric Potential
💡Electric Potential Energy
💡Thermal Energy
💡Power
💡Ohm's Law
💡Voltage
💡Kinetic Energy
💡Energy Transformation
💡Electrical Components
Highlights
Resistors warm up when current flows through them, potentially becoming hot enough to burn.
The conceptual reason for the heating of a resistor is due to the flow of current and the change in electric potential energy.
Positive charges are often used in descriptions for simplicity, despite it being electrons that actually move through a wire.
As charges move through a resistor, they decrease their electric potential energy, which is converted into thermal energy, heating the resistor.
The decrease in potential energy does not increase the kinetic energy of the charges, contrary to common assumptions based on gravitational potential energy.
The energy lost by the charges is transferred to the atoms and molecules within the resistor, causing an increase in temperature.
The power, or energy transferred per time, can be calculated using the formula Power = Change in Electrical Potential Energy / Time.
The formula for electrical power in terms of current and voltage is Power = Current * Voltage.
Ohm's law can be used to derive alternative power formulas: Power = Current^2 * Resistance and Power = Voltage^2 / Resistance.
The power formulas can be used to determine the energy usage of electronic devices, such as light bulbs or toasters.
Electrical potential energy is always converted into some form of energy, whether it's heat, light, or sound.
The power formulas are not limited to resistors but apply to all electrical components that convert electric potential energy.
The choice of power formula depends on the known quantities for a given problem, such as current, voltage, or resistance.
The concept of energy conversion in resistors is fundamental to understanding electrical circuits and their behavior.
The explanation provided demystifies the counterintuitive nature of energy conversion in electrical circuits.
The derivation of power formulas from first principles offers a deeper understanding of electrical power and energy.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: