2022 AP Physics 1 Free Response #3
TLDRThe video script discusses an experiment to demonstrate the conservation of energy as a block falls and a wheel rotates. The presenter outlines the steps to measure gravitational potential energy and kinetic energy, using a motion sensor and camera to record the block's velocity and height. The goal is to show the equivalence of energy transformation from potential to kinetic. Additionally, the script touches on the rotational kinetic energy of the wheel and how to graph it in relation to the block's fall, emphasizing the importance of accurate measurements for a successful experiment.
Takeaways
- 📝 The speaker is explaining an experimental procedure they started before realizing their microphone wasn't working.
- ⚖️ The experiment involves a wheel on a horizontal axle with light strings attached to the wheel's rim.
- 🏋️ The block's gravitational potential energy converts to kinetic energy as it falls, causing the wheel to rotate.
- 👩🔬 The students aim to compare the decrease in gravitational potential energy to the increase in the block's kinetic energy.
- 🔬 Required equipment includes a scale for mass, a meter stick for height, and a motion sensor for velocity.
- 🎥 The speaker debates using a camera versus a motion sensor and decides on a motion sensor for accuracy.
- 📐 The procedure involves measuring the block's mass, height, and velocity multiple times to account for experimental uncertainty.
- ⚙️ The kinetic energy of the block is calculated using the maximum velocity recorded by the motion sensor.
- 🔄 The graph should represent the total energy, including rotational kinetic energy of the wheel, remaining constant.
- 📈 The rotational kinetic energy is determined by plotting kinetic energy against angular velocity squared and finding the slope to be half the moment of inertia.
Q & A
What is the main objective of the experiment described in the script?
-The main objective of the experiment is to compare the increase in the block's translational kinetic energy with the decrease in its gravitational potential energy as it falls.
Why does the speaker decide to use a motion sensor instead of a camera to measure the block's velocity?
-The speaker chooses a motion sensor over a camera because the block is accelerating as it falls, making it difficult to accurately estimate its changing velocity using a camera. A motion sensor provides more accurate velocity measurements.
What steps are involved in setting up the experiment?
-The steps include weighing the mass of the block, setting up a meter stick behind the block, positioning a camera to observe the fall, placing the motion sensor directly below the block, releasing the block, and recording the height and velocity. The process is repeated three times for accuracy, with varying release heights.
How is the kinetic energy of the block calculated just before it hits the floor?
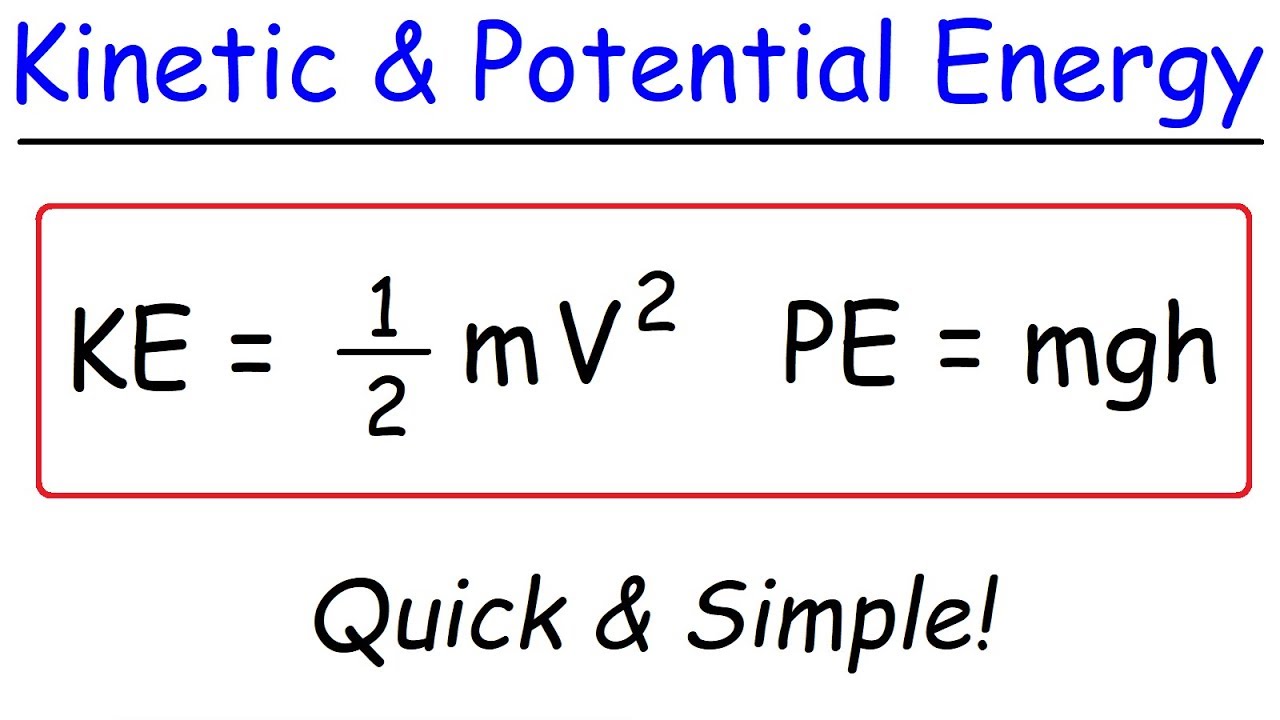
-The kinetic energy is calculated using the maximum velocity recorded by the motion sensor. The formula used is KE = 1/2 mv^2, where m is the mass of the block and v is the maximum velocity.
What is the purpose of repeating the experiment three times at different heights?
-Repeating the experiment three times at different heights ensures that the results are consistent and not influenced by specific conditions or errors. This helps account for experimental uncertainty.
How does the script suggest graphing the rotational kinetic energy of the wheel as a function of the block's distance?
-The script suggests starting the rotational kinetic energy at zero (since the wheel is initially not spinning) and then drawing a curve where the total energy (gravitational potential energy + translational kinetic energy + rotational kinetic energy) remains constant, reflecting energy conservation.
What does the slope of the graph represent when plotting rotational kinetic energy against omega squared?
-The slope of the graph represents one half of the moment of inertia (1/2 I) of the wheel, as the relationship between rotational kinetic energy (KR) and omega squared (ω²) is given by KR = 1/2 Iω².
Why is it important to choose points along the line of best fit when determining the moment of inertia?
-Choosing points along the line of best fit ensures that the calculation of the moment of inertia is based on the overall trend of the data, reducing the impact of any outliers or measurement errors.
What assumption is made about the system's energy during the experiment?
-The experiment assumes that the total energy of the system is conserved, with no work done on the system, aside from minor effects like air resistance and friction, which are considered negligible.
How does the experiment address potential experimental uncertainties?
-The experiment addresses uncertainties by repeating the measurements multiple times and varying the height from which the block is released. This helps to ensure that the results are reliable and not affected by random errors.
Outlines
🔧 Troubleshooting Microphone Issues and Energy Concepts
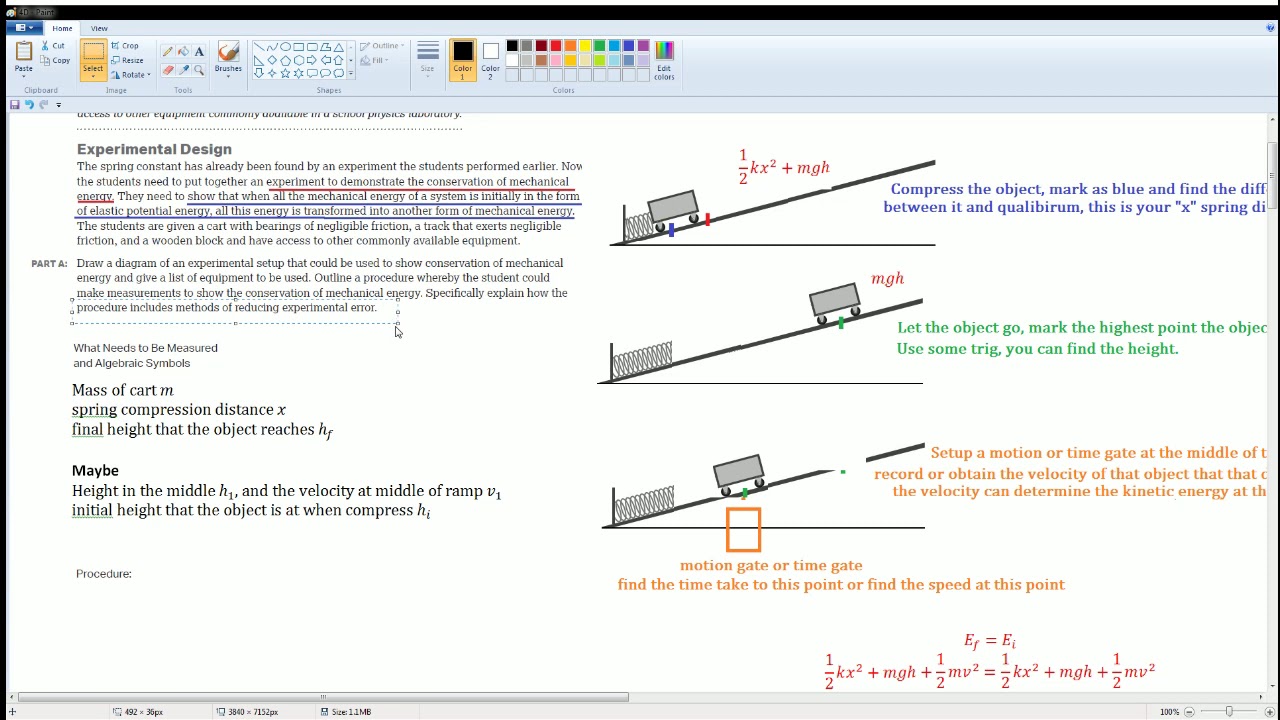
The author begins by explaining that they started working on the video script before realizing their microphone wasn't working. They decide to continue with their initial notes and provide explanations verbally. The main focus is on a physics problem involving a wheel, a block, and energy transformation. The scenario describes how the block's gravitational potential energy is converted into kinetic energy as it falls, leading to a discussion on designing an experiment to compare the decrease in gravitational potential energy with the increase in the block's kinetic energy.
📊 Experimental Design for Measuring Energy Changes
The author details an experimental procedure to measure the increase in the block's kinetic energy and the decrease in gravitational potential energy. The experiment involves weighing the block, measuring the height, and using a motion sensor to record the block's speed. They explain the reasoning behind using a motion sensor over a camera due to the accuracy needed for capturing changing speeds. The experiment is repeated multiple times at different heights to ensure reliability and account for experimental uncertainty. The summary also includes the calculation method for kinetic energy using the maximum velocity data.
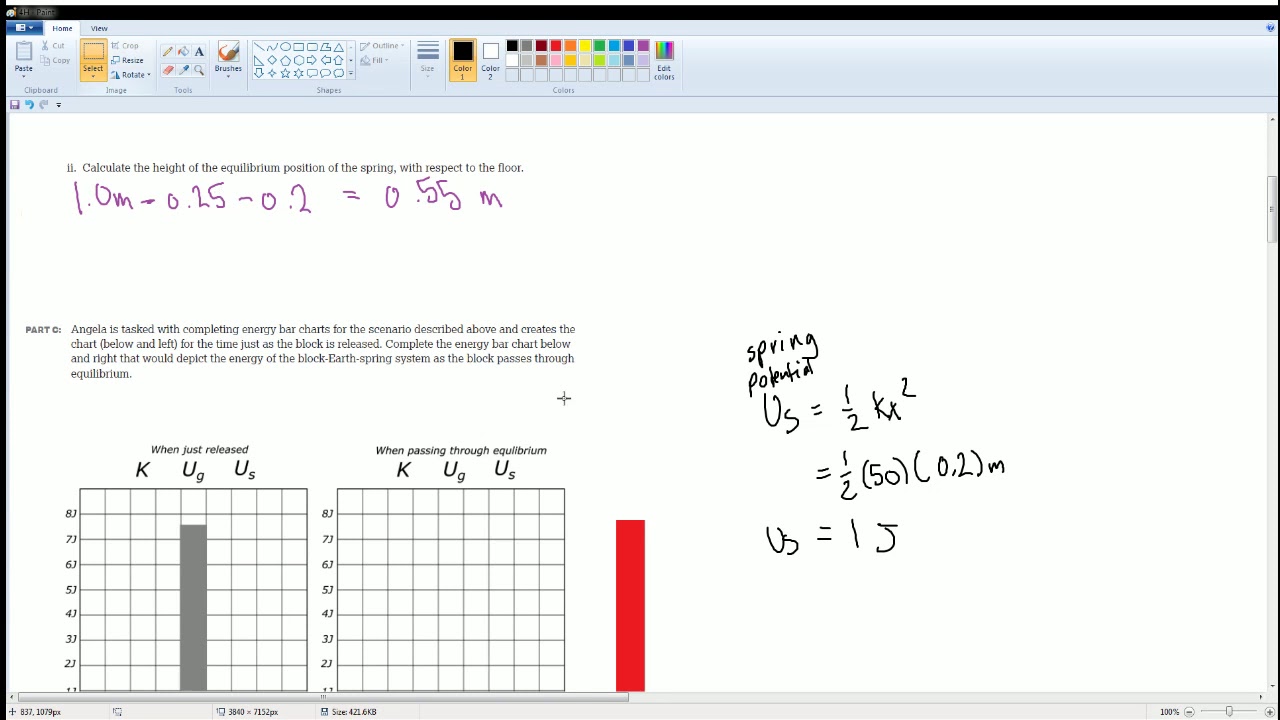
📉 Graphical Analysis of Energy Transformations
The author explains how to represent the rotational kinetic energy of the wheel on a graph that shows the changes in gravitational potential energy and translational kinetic energy as the block falls. They emphasize that the total energy in the system must remain constant, with the sum of kinetic and potential energies adding up to zero, accounting for negligible friction and air resistance. The graphical representation is detailed, showing how to ensure the vertical values of potential and kinetic energy sum to zero.
📏 Slope Calculation for Rotational Kinetic Energy
The author discusses how to determine the rotational kinetic energy of the wheel by plotting kinetic energy against the square of angular velocity (omega squared). They explain that the slope of the line of best fit represents half of the moment of inertia (I). The process involves picking two points on the graph, calculating the slope, and using it to determine the rotational kinetic energy, demonstrating the method with an example calculation.
Mindmap
Keywords
💡Wheel
💡Gravitational Potential Energy
💡Kinetic Energy
💡Conservation of Energy
💡Experimental Procedure
💡Motion Sensor
💡Camera
💡Translational Kinetic Energy
💡Rotational Kinetic Energy
💡Angular Velocity
💡Uncertainty
Highlights
The experiment explores the conversion of gravitational potential energy to kinetic energy as a block falls.
A wheel with light strings attached is used to demonstrate energy conversion with negligible friction.
Students aim to test the equality of gravitational potential energy decrease and kinetic energy increase.
The necessity to record one half mv squared and mgh to compare energy changes is emphasized.
A motion sensor is chosen for its accuracy in capturing variable speeds during the block's fall.
The challenge of capturing height with a meter stick and velocity with a camera is discussed.
An experimental procedure is designed to compare translational kinetic energy with gravitational potential energy.
Repeating the experiment from the same height multiple times to handle experimental uncertainty is suggested.
Changing the release point's height and repeating the experiment to ensure results are not height-specific.
The method to calculate the block's kinetic energy before it reaches the floor using motion sensor data is explained.
The importance of using the maximum velocity from motion sensor data to represent the block's speed is highlighted.
A graph is proposed to represent the change in gravitational potential energy and translational kinetic energy.
The graph also aims to illustrate the rotational kinetic energy of the wheel as a function of the block's falling distance.
The concept of conservation of energy is applied to ensure the total energy remains constant throughout the experiment.
The challenge of aligning energy changes on the graph to demonstrate conservation of energy is discussed.
Measuring angular velocity to determine rotational kinetic energy and its representation on a graph is explained.
The method of plotting rotational kinetic energy against omega squared and calculating the slope as one half I is detailed.
Estimation of values from the graph to ensure the conservation of energy in the system is demonstrated.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: