College Physics 1: Lecture 29 - Forms of Energy
TLDRThis lecture delves into the various forms of energy, focusing on defining and quantifying kinetic, gravitational potential, elastic potential, and thermal energy. It begins with kinetic energy, explaining its dependence on mass and velocity, and derives its equation from the work-energy principle. Gravitational potential energy is discussed next, highlighting its relation to height above a surface. Elastic potential energy is introduced with Hooke's law, leading to its equation. Lastly, thermal energy is explained as the sum of kinetic and potential energies at the atomic level. The lecture provides examples to illustrate the calculations and applications of these energy forms, setting the stage for the next lecture on the conservation of energy.
Takeaways
- 📚 The lecture reintroduces different forms of energy with definitions and equations, focusing on the conservation of total energy in a system.
- 🏃 Kinetic energy (K) is the energy of motion, calculated as \( \frac{1}{2} m V^2 \), where 'm' is mass and 'V' is velocity, and it depends heavily on speed as it is squared in the equation.
- 🔍 The derivation of kinetic energy equation starts from the basic kinematic equation and uses Newton's second law and the concept of work done.
- ⚖️ The unit of all forms of energy, including kinetic energy, is the joule, emphasizing the importance of using joules as the default unit for energy.
- 📘 Gravitational potential energy (Ug) is the stored energy due to an object's height above the ground, calculated as mgy, where 'g' is the acceleration due to gravity.
- 🔗 Gravitational potential energy depends only on the height above the ground, irrespective of the path taken to reach that height.
- 🈚 At ground level, gravitational potential energy is considered zero, simplifying calculations for changes in potential energy.
- 🔥 Elastic potential energy is associated with the compression or stretching of springs and is given by \( \frac{1}{2} KX^2 \), where 'K' is the spring constant and 'X' is the displacement.
- 🔗 Hooke's law (F = -kX or F = kX) defines the force needed to compress or stretch a spring, with 'k' being the spring constant and 'X' the displacement.
- 🌡 Thermal energy (Eth) is the energy associated with the hotness of an object, involving the kinetic and potential energies of its atoms and bonds, and is calculated from work done by friction.
- 🔄 The conservation of energy is a principle that will be discussed in the next lecture, emphasizing the interconversion of different forms of energy while the total energy remains constant.
Q & A
What is the primary goal of the lecture on forms of energy?
-The primary goal of the lecture is to reintroduce each of the forms of energy that were introduced in the previous lecture, this time with more definition and an equation for each, in order to discuss the concept of total energy conservation within a system.
What is kinetic energy and how is it calculated?
-Kinetic energy is the energy of motion. An object in motion possesses kinetic energy, which is calculated using the formula K = 1/2 * M * V^2, where K is the kinetic energy, M is the mass of the object, and V is its velocity.
How does the lecturer derive the equation for kinetic energy?
-The lecturer derives the equation for kinetic energy by starting with the kinematics equation V^2 = u^2 + 2*a*d, applying Newton's second law to express acceleration as force over mass, and then rearranging the equation to define kinetic energy as the work done over mass.
What is the unit of kinetic energy?
-The unit of kinetic energy is the joule, which is also the unit for all forms of energy, as derived from the equation where kinetic energy is expressed as work done over mass, and work is defined as force times displacement.
Can you provide an example of how to calculate the speed of a bobsled after being pushed on a frictionless surface?
-The speed of a bobsled can be calculated using the work-energy principle. Given the mass of the bobsled, the force applied, and the displacement, the final velocity can be found by rearranging the work-energy equation and solving for V, which involves steps like multiplying by 2 to eliminate the 1/2 factor, dividing by mass, and taking the square root.
What is gravitational potential energy and how is it related to an object's height above the ground?
-Gravitational potential energy is the stored energy an object has due to its position relative to a gravitational field, typically height above the ground. It is calculated using the formula U_g = m*g*y, where U_g is the gravitational potential energy, m is the mass, g is the acceleration due to gravity, and y is the height above the ground.
How does the path taken to reach a certain height affect the gravitational potential energy of an object?
-The path taken to reach a certain height does not affect the gravitational potential energy of an object. The gravitational potential energy depends only on the height above the ground, regardless of the path taken to get there.
What is Hooke's Law and how does it relate to the force needed to compress or stretch a spring?
-Hooke's Law defines the force needed to compress or stretch a spring. It states that the force (F) is proportional to the displacement (X) from the spring's equilibrium position, expressed as F = -k*X (for the restoring force of the spring) or F = k*X (for the force applied to the spring), where k is the spring constant.
How is elastic potential energy calculated and what is the significance of the spring constant in this calculation?
-Elastic potential energy is calculated using the formula E_pe = 1/2 * k * X^2, where E_pe is the elastic potential energy, k is the spring constant, and X is the displacement of the spring. The spring constant (k) represents the stiffness of the spring and is crucial in determining the energy stored in the spring.
What is thermal energy and how does it relate to the motion of atoms within an object?
-Thermal energy is the energy associated with the hotness of an object, which is a result of the kinetic and potential energies of its atoms. As the temperature of an object increases, the atoms move faster, increasing their kinetic energy, and the bonds between them stretch and compress more, increasing the potential energy stored in those bonds.
How is the change in thermal energy related to friction and displacement?
-The change in thermal energy is related to the work done by frictional force over a displacement. It is calculated using the formula ΔE_th = F_friction * ΔX, where ΔE_th is the change in thermal energy, F_friction is the kinetic friction force, and ΔX is the displacement in the direction of the force.
What is the relationship between the speed of a marble rolling down two hills of the same height, one steep and the other less steep, with negligible friction?
-The marble will be moving at the same speed at the bottom of both hills because the change in gravitational potential energy, and thus the change in kinetic energy, is the same for both hills, regardless of their steepness.
Outlines
📚 Introduction to Forms of Energy
The lecture begins with an introduction to different forms of energy, emphasizing the importance of understanding each type with definitions and equations. The goal is to discuss the conservation of total energy within a system. Starting with kinetic energy, it is defined as the energy of motion, dependent on an object's mass and the square of its velocity. The derivation of the kinetic energy equation from basic kinematic principles and Newton's second law is shown, leading to the understanding that kinetic energy is work done on an object, resulting in an increase in its kinetic energy.
🏋️♂️ Units and Examples of Kinetic Energy
The units of kinetic energy are derived, showing that it is measured in joules, the same unit used for all forms of energy. An example problem is solved involving a bobsled being pushed along a frictionless surface, demonstrating the application of the work-energy principle to find the final speed of the bobsled after a certain displacement.
🌐 Gravitational Potential Energy
Gravitational potential energy is introduced as the stored energy associated with an object's height above a surface. The equation for gravitational potential energy (mgy) is derived from the work-energy principle, considering the work done against gravity to lift an object. It is emphasized that gravitational potential energy depends only on the height above the ground, regardless of the path taken to reach that height. An example involving the Empire State Building run up is used to calculate the gravitational potential energy gained by a competitor.
🔄 Elastic Potential Energy and Hooke's Law
Elastic potential energy is discussed in relation to springs, with Hooke's law introduced to define the force required to compress or stretch a spring. The spring constant (k) and displacement (x) are used to calculate the elastic potential energy, which is given by the formula (1/2)kx^2. An example of an archer pulling back a bowstring is used to demonstrate the calculation of elastic potential energy, requiring the弓nderstanding of both the spring constant and the displacement.
🔥 Thermal Energy and its Relation to Friction
Thermal energy is described as the energy associated with the hotness of an object, resulting from the kinetic and potential energies of its atoms and bonds. The faster atoms move, the more kinetic energy they possess, and the more they stretch and compress their bonds, the more potential energy is stored. The equation for thermal energy is derived from the work-energy principle, specifically considering the work done by frictional force over a displacement. It is noted that thermal energy is always a change (ΔE_th) and cannot be calculated at an instant because it is caused by friction over a distance.
🔄 Energy Conversion and Conservation
The lecture concludes with two questions related to energy. The first question asks to rank the gravitational potential energies of balls at different heights, which is straightforward given the equation mgy and the assumption of constant mass and gravitational acceleration. The second question involves a marble rolling down two different hills of the same height, with the conclusion that the marble will have the same speed at the bottom of both hills due to the conservation of energy, assuming negligible friction.
Mindmap
Keywords
💡Kinetic Energy
💡Potential Energy
💡Work-Energy Principle
💡Conservation of Energy
💡Newton's Second Law
💡Spring Constant (k)
💡Elastic Potential Energy
💡Thermal Energy
💡Work (W)
💡Gravitational Potential Energy
Highlights
Introduction to Lecture 29 on forms of energy with a focus on defining and equating different energy forms for conservation of total energy in a system.
Kinetic energy defined as the energy of motion, dependent on mass and the square of velocity, with derivation from basic kinematics and Newton's second law.
Derivation of kinetic energy equation from work done, showcasing the connection between force, displacement, and kinetic energy.
Explanation of kinetic energy units, converting from kilogram meter squared per second squared to joules, emphasizing joules as the standard unit for all forms of energy.
Illustration of the range of kinetic energy values through a table, indicating the variability from small (like an ant) to large (like a supertanker).
Example problem involving a bobsled to demonstrate the application of the work-energy principle in calculating final velocity.
Introduction to gravitational potential energy as the stored energy associated with an object's height above a surface, with a formula of mgy.
Discussion on the independence of gravitational potential energy from the path taken, highlighting that it only depends on the initial and final height.
Example calculation of gravitational potential energy gained by a racer in the Empire State Building run up.
Introduction to Hooke's Law, defining the force required to compress or stretch a spring, with the formula f = kx.
Derivation of elastic potential energy from the work-energy principle, resulting in the formula for spring potential energy as (1/2)kx^2.
Example problem calculating the elastic potential energy stored in a bow when drawn by an archer.
Conceptual explanation of thermal energy as the sum of kinetic and potential energies at the atomic level, affected by temperature.
Equation for thermal energy change due to friction, Δeth = friction * Δx, applicable in scenarios involving friction.
Ranking of gravitational potential energies of balls based on their height above the ground, illustrating a direct relationship with height.
Comparison of speeds at the bottom of two different hills, concluding that the speed is the same due to equal changes in gravitational potential energy.
Transcripts
Browse More Related Video

College Physics 1: Lecture 28 - Work and Energy

College Physics 1: Lecture 30 - Conservation of Energy

Energy Stores and Transfers
Energy | Forms of Energy | Law of Conservation of Energy | Science Lesson for Kids
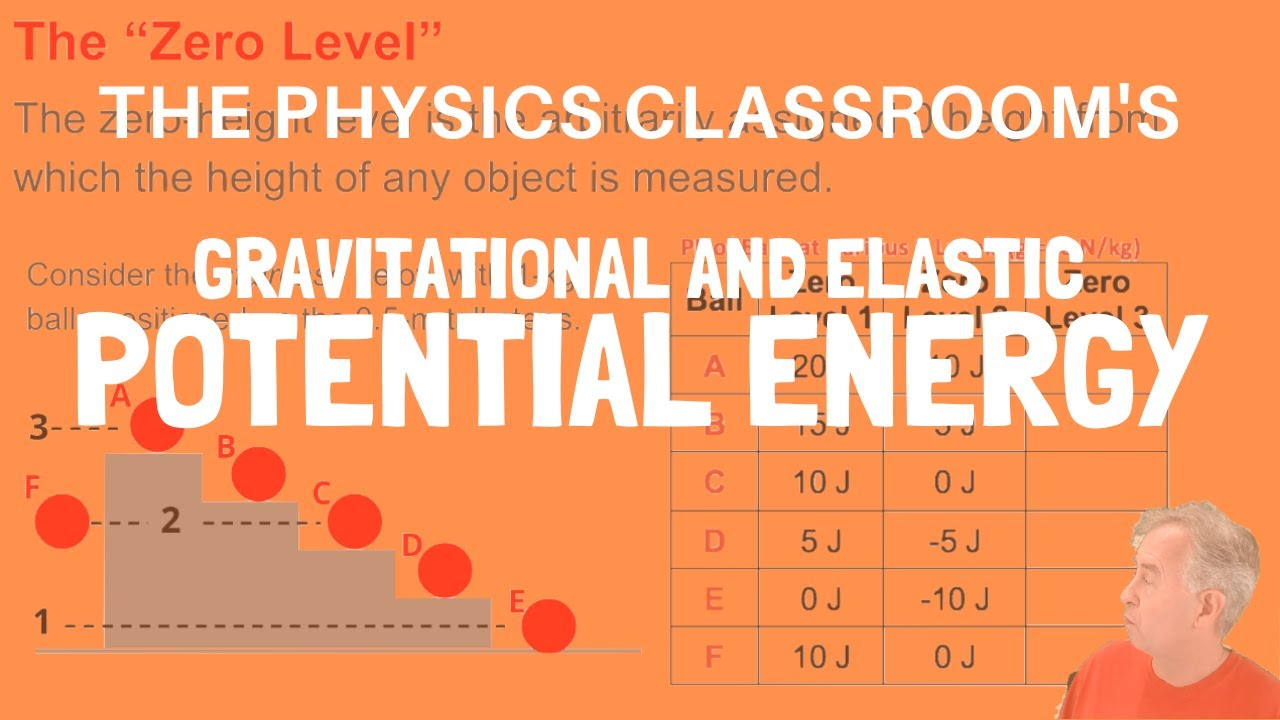
Gravitational and Elastic Potential Energy
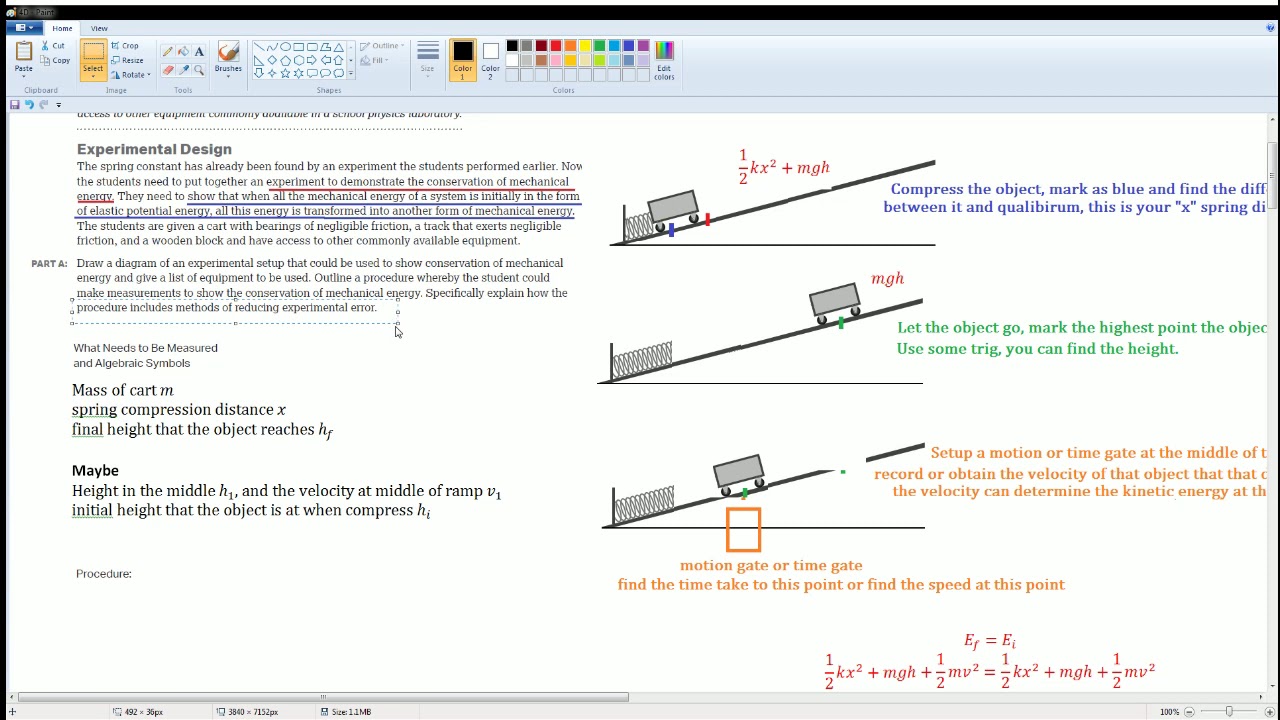
AP Physics Workbook 4.D Spring Potential Energy Lab
5.0 / 5 (0 votes)
Thanks for rating: