2. Resonance II
TLDRThe lecture delves into the complexities of the harmonic oscillator in physics, emphasizing the precision of frequency measurements and the relevance of Fourier theorem and Heisenberg's uncertainty principle. It explores the potential for exceeding these limits through high signal-to-noise ratios and quantum mechanics, particularly with nonlinear processes. The discussion extends to the comparison between two-level systems and harmonic oscillators, highlighting their similarities and differences, and introduces the concept of classical magnetic moments as an analog to quantum systems, including the dynamics in rotating frames and the distinction between Larmor and cyclotron frequencies.
Takeaways
- 📚 The lecture begins with a discussion on the importance of the harmonic oscillator in physics and the precision of frequency measurements, emphasizing the relevance of Fourier theorem in such measurements.
- 🔍 The professor highlights the connection between Fourier's limit and Heisenberg's uncertainty relation, both stemming from the wave nature of matter.
- 🤔 An interactive question is posed to students regarding the uncertainty in frequency measurement for classical systems, with the answer being that a good signal-to-noise ratio can improve precision beyond the theoretical limit.
- 📉 The concept of 'splitting the line' in spectral measurements is introduced, which refers to the ability to determine the center of a spectral peak with higher accuracy than the peak's width, based on the signal-to-noise ratio.
- 🚫 The lecture touches on the challenges of achieving high precision in measurements, especially when considering the line shape and ensuring the observed line center corresponds to the true frequency.
- 🌟 The discussion extends to quantum-mechanical oscillators and the possibility of surpassing the Heisenberg limit in frequency measurement through the use of nonlinear processes.
- 🔬 The professor delves into the comparison between two-level systems and harmonic oscillators, explaining the conditions under which their behaviors can be considered analogous.
- 🔄 The differences between two-level systems and harmonic oscillators are explored, particularly the phenomenon of saturation in two-level systems which is not present in harmonic oscillators.
- 🌀 The lecture introduces the topic of rotating systems, specifically gyroscopes, and draws parallels between their dynamics and those of two-level systems, despite fundamental differences.
- 🧲 The dynamics of classical magnetic moments in magnetic fields are discussed, including the concepts of torque, angular momentum, and the Larmor frequency.
- ⚙️ The gyromagnetic ratio, which relates magnetic moment to angular momentum, is defined, and its significance in determining the Larmor frequency for different particles like electrons and protons is explained.
Q & A
What is the main topic discussed in the script?
-The main topic discussed in the script is the harmonic oscillator in physics, with a focus on frequency measurements and the relationship between classical and quantum systems.
Why is frequency measurement important in physics?
-Frequency measurement is important in physics because it allows for precise measurements, as frequencies can be measured with high accuracy using synthesizers and clocks.
What is the connection between Fourier's theorem and Heisenberg's uncertainty relation?
-The connection between Fourier's theorem and Heisenberg's uncertainty relation is that both involve a trade-off between the precision of time and frequency measurements, with the product of the uncertainty in frequency and time being greater than or equal to 1/2.
How does the signal-to-noise ratio affect the precision of frequency measurements?
-The signal-to-noise ratio affects the precision of frequency measurements by allowing the center of a spectral peak to be determined with an accuracy that may be much better than the width of the peak, given by Fourier's theorem.
What is the significance of the Gaussian wave packet in the context of Fourier's theorem?
-The Gaussian wave packet is significant because it realizes the case where the product of the frequency uncertainty and the time uncertainty is exactly 1/2, which is the minimum uncertainty allowed by Fourier's theorem.
Can the Heisenberg uncertainty relation be surpassed in certain conditions?
-Yes, under certain conditions such as having a good signal-to-noise ratio or using nonlinear processes, the precision of measurements can surpass the limits set by the Heisenberg uncertainty relation.
What is the difference between a harmonic oscillator and a two-level system?
-A harmonic oscillator has an infinite number of equidistant energy levels and can be driven to arbitrarily large amplitudes, while a two-level system has only two energy levels and a maximum excitation limit, which is the phenomenon of saturation.
How does the script relate the classical description of a laser to a quantum-mechanical system?
-The script relates the classical description of a laser to a quantum-mechanical system by discussing the coherent state with a large photon number, which represents the classical limit of an electromagnetic field, while still being fundamentally quantum in nature due to the population of photons.
What is the concept of cavity Quantum Electrodynamics (QED) mentioned in the script?
-Cavity QED is a concept where the interaction between light and matter is studied within a confined space, such as an optical cavity. It allows for the investigation of quantum phenomena like the creation of non-classical states of the photon field.
How does the script explain the analogy between a classical magnetic moment and a quantum mechanical two-level system?
-The script explains the analogy by showing that the motion of classical magnetic moments, such as a precessing gyroscope, can capture many features of the dynamics of a quantum mechanical two-level system, including Rabi frequency and resonant conditions, despite the absence of quantum phenomena like spontaneous emission and quantum projection measurements.
What is the significance of the Larmor frequency in the context of magnetic resonance?
-The Larmor frequency is significant in magnetic resonance as it represents the natural precession frequency of a magnetic moment in a magnetic field. It is used to drive transitions in magnetic moments and is a key parameter in magnetic resonance experiments.
What is the difference between the Larmor frequency and the cyclotron frequency?
-The Larmor frequency is the frequency at which a magnetic moment precesses in a magnetic field, while the cyclotron frequency is the frequency at which a charged particle, like an electron, revolves in a magnetic field. The cyclotron frequency is twice the Larmor frequency for a free electron.
Outlines
📚 Introduction to Harmonic Oscillator and Frequency Measurement
The script begins with an introduction to the harmonic oscillator, a complex system in physics, emphasizing the importance of frequency measurement in precision physics. It mentions the accuracy of measurements done through frequency and introduces Fourier's theorem, which relates the time of oscillation to the spread of frequency components. The professor also discusses the relationship between Fourier's limit and Heisenberg's uncertainty principle, highlighting the relevance of these concepts to all precision measurements. The script then poses a question about the uncertainty in frequency measurement for classical systems, suggesting that a good signal-to-noise ratio could potentially allow for more precise measurements.
🔍 Signal-to-Noise Ratio and Frequency Measurement Precision
This paragraph delves into the impact of noise on wave-formed signals and the precision of frequency measurements. It explains that while Fourier's theorem sets a limit on the width of spectral components based on observation time, the center of the spectral peak can be determined more accurately, depending on the signal-to-noise ratio. The summary outlines the concept of 'splitting the line' to achieve greater measurement accuracy and touches on the challenges of ensuring the line shape is known and symmetric to accurately determine the frequency. The discussion also introduces the idea of measuring the frequency of a quantum-mechanical oscillator and an optical laser pulse, questioning whether the Heisenberg limit can be surpassed.
🌟 Quantum Harmonic Oscillator and Laser Pulse Frequency Measurement
The script explores whether the frequency of a quantum-mechanical harmonic oscillator or an optical laser pulse can be measured with accuracy beyond the Heisenberg limit. It clarifies that a laser, despite being quantum in nature, can behave classically under certain conditions. The professor discusses the use of a strong local oscillator to create a beat note with a laser, which can be measured with high precision, suggesting that the signal-to-noise ratio can be significantly improved to enhance measurement accuracy. The paragraph also addresses the subtleties of the Heisenberg uncertainty principle and its application to single quantum systems versus multiple measurements or photons.
🔬 Quantum Mechanics and the Heisenberg Limit in Frequency Measurement
This section discusses the possibility of surpassing the Heisenberg limit in frequency measurement for quantum harmonic oscillators and the impact of using multiple photons. It explains that with n uncorrelated photons, the signal-to-noise ratio improves, allowing for better frequency resolution. The script also touches on the concept of the Heisenberg limit in the context of correlated photons and the potential for even higher precision measurements. The audience's confusion about the applicability of the uncertainty relation in different scenarios is addressed, highlighting the importance of signal-to-noise ratio in achieving higher accuracy.
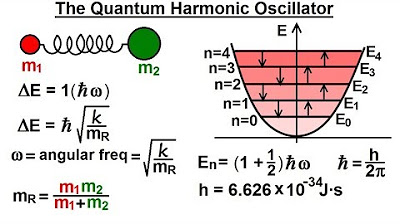
🔄 The Analogy Between Two-Level Systems and Harmonic Oscillators
The script examines the analogy and differences between two-level systems and harmonic oscillators. It explains that a two-level system can be considered a harmonic oscillator under conditions of weak excitation, where only a small fraction of the system is in the first excited state. The concept of saturation is introduced as a distinguishing feature, where a two-level system can be saturated while a harmonic oscillator cannot. The script also discusses the Lorentz model for an atom, which illustrates the equivalence of a two-level system and a harmonic oscillator in certain conditions, and the phenomenon of saturation that distinguishes them.
🌀 Cavity QED and the Creation of Non-Classical States
This paragraph discusses the creation of non-classical states in cavity quantum electrodynamics (QED). It explains the challenge of selectively exciting the n=1 state in a harmonic oscillator and the use of anharmonicities or nonlinearities to achieve this. The script describes how the interaction between an atom and photons in a cavity can lead to normal mode splitting, which is proportional to the Rabi frequency, allowing for the creation of non-classical states of the photon field. The discussion highlights the difference between a pure harmonic oscillator and a system with an atom that can help overcome the limitations of the harmonic oscillator in preparing certain states.
🌐 Classical Rotation and the Dynamics of Magnetic Moments
The script introduces the concept of classical rotation, specifically focusing on the dynamics of magnetic moments in a magnetic field. It discusses the properties of a gyroscope and how the amplitude of its excitation is limited, drawing parallels with a two-level system. The professor emphasizes that the motion of classical magnetic moments can serve as an exact model for the dynamics of a quantum mechanical two-level system, highlighting the surprising similarities in their behavior. The limitations of this analogy, such as the absence of spontaneous emission in certain quantum systems, are also touched upon.
🧲 The Classical Magnetic Moment and Precession in Magnetic Fields
This section delves into the dynamics of a classical magnetic moment in magnetic fields, including both static and time-varying fields. The script explains the interaction energy between a classical magnetic moment and a magnetic field, the torque involved, and the resulting precession of angular momentum. The concept of the gyromagnetic ratio is introduced, which relates the magnetic moment to its angular momentum. The Larmor frequency, the frequency of precession, is also discussed, with examples provided for an electron, a classical charge distribution, and a proton.
🔁 Precession Frequency and the Gyromagnetic Ratio
The script explores the precession frequency of classical charge distributions and contrasts it with quantum mechanical systems, specifically focusing on the electron. It explains the difference in precession frequency due to the gyromagnetic ratio and the G factor of the electron. The importance of factors of 2 in understanding magnetic moments and precession systems is highlighted, using simple examples to illustrate the concepts. The script also discusses the relationship between the precession frequency and the energy levels of the system.
🛠 Solving Dynamics in a Rotating Frame
This paragraph discusses the method of solving the dynamics of a rotating system by transforming into a rotating frame of reference. The script explains the operator equation that relates the time derivative in the rotating frame to that in the inertial frame. It then applies this concept to the angular momentum of a precessing classical magnetic moment, showing how the equation of motion is modified in the rotating frame. The script also touches on the conditions under which the effective magnetic field vanishes, leading to a constant angular momentum in the rotating frame.
🔁 Larmor Frequency and the Dynamics of Charged Particles
The script concludes with a discussion on the Larmor frequency and its relation to the cyclotron motion of electrons. It clarifies the difference between the Larmor frequency and the cyclotron frequency, highlighting the factor of 2 that separates them. The importance of understanding this distinction is emphasized, as it is crucial for comprehending the physics of magnetic moments and charged particles in magnetic fields. The script also references Larmor's theorem and its application to charge distributions, noting the differences between the exact dynamics of a magnetic moment and the approximated dynamics of a charge distribution.
Mindmap
Keywords
💡Harmonic Oscillator
💡Frequency Measurement
💡Fourier Theorem
💡Signal-to-Noise Ratio
💡Heisenberg Uncertainty Relation
💡Quantum Harmonic Oscillator
💡Laser
💡Coherent State
💡Two-Level System
💡Saturation
💡Cavity Quantum Electrodynamics (Cavity QED)
💡Gyromagnetic Ratio
💡Larmor Frequency
💡Magnetic Resonance
💡Classical Rotation
💡Quantum Measurement Process
Highlights
Introduction to the complex system of the harmonic oscillator in physics.
Discussion on the precision of frequency measurements and the relevance to all precision measurements in physics.
Explanation of the Fourier theorem and its relation to the frequency spectrum and Heisenberg's uncertainty relation.
The concept that good signal-to-noise ratio can improve the precision of frequency measurement beyond the uncertainty limit.
Investigation on whether it's possible to measure the frequency of a quantum-mechanical harmonic oscillator more accurately than the Heisenberg limit.
The use of a laser to create a beat note for high precision frequency measurement.
Understanding the conditions under which a laser can be considered a classical or quantum harmonic oscillator.
The possibility of surpassing the Heisenberg limit by using nonlinear processes in quantum mechanics.
The difference between a two-level system and a harmonic oscillator, particularly in the context of saturation.
Analogy between a classical magnetic moment and a quantum-mechanical two-level system in terms of dynamics.
The unique properties of quantum mechanics, such as the projection postulate in quantum measurement, not found in classical systems.
The classical model of magnetic resonance and its application in understanding quantum mechanical systems.
The importance of the gyromagnetic ratio in describing the dynamics of classical magnetic moments.
The Larmor frequency and its significance in the precession of magnetic moments.
The concept of a rotating frame and its utility in solving dynamics of precessing systems.
Clarification of the difference between the Larmor frequency and the cyclotron frequency of an electron.
The significance of factors of 2 in magnetic moments, precession frequencies, and quantum mechanics.
Transcripts
Browse More Related Video

Advanced Quantum Mechanics Lecture 3

3. Resonance III
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (39 of 92) What is the Quantum Oscillator?
Lecture 13: More on Scattering

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (41 of 92) What is Zero Point Vibration?

Advanced Quantum Mechanics Lecture 4
5.0 / 5 (0 votes)
Thanks for rating: