Natural Logs and Differentiation
TLDRThis educational video explores the concept of natural logarithms, emphasizing their definition as the area under the curve of 1/T from 1 to X, with base E. It explains the properties of natural logs, such as their graph characteristics, domain, range, and how they're always increasing and concave down. The instructor demonstrates logarithm properties, simplification techniques, and how to find derivatives using the fundamental theorem of calculus, showcasing examples and the chain rule. The video concludes with solving a differential equation using natural log derivatives, offering a comprehensive guide for students.
Takeaways
- 📚 The natural log, also known as the logarithm with base e, is defined as the area under the curve of 1/T from 1 to T and is only defined for positive values of X.
- 🔢 The base of the natural log is the number e, named after Leonard Euler, and e and natural log are inverse functions of each other.
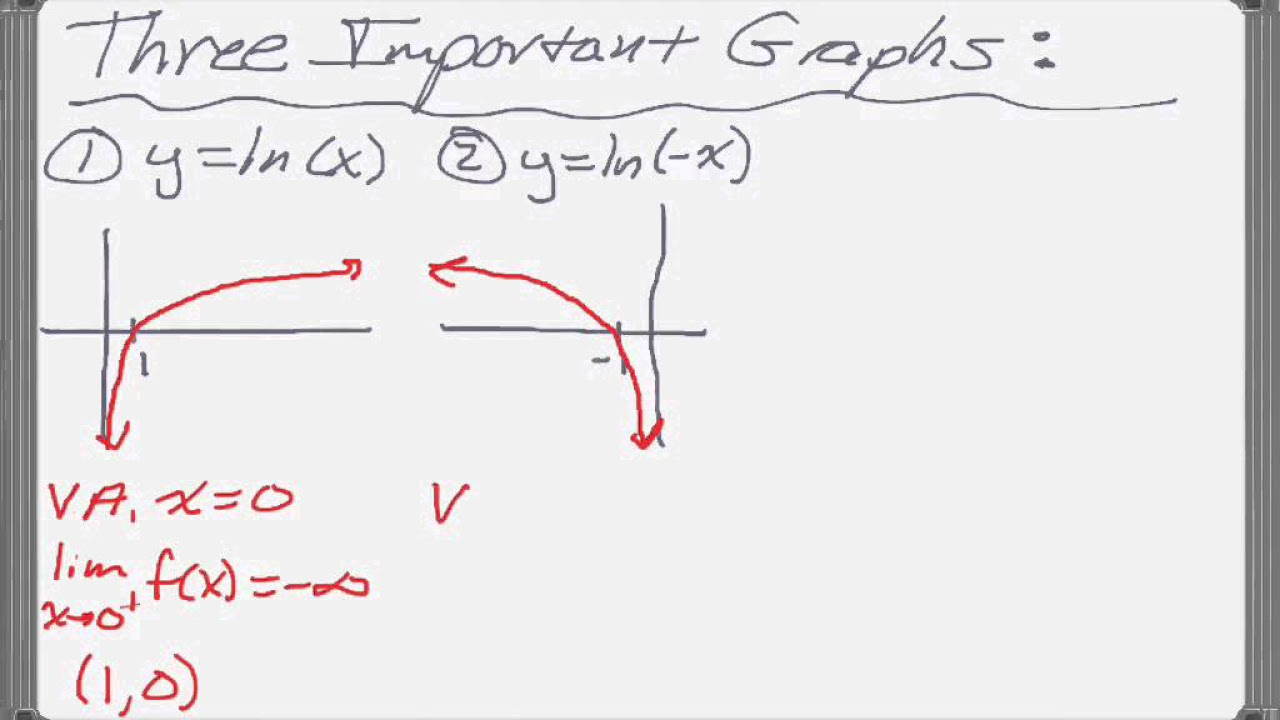
- 📈 The graph of the natural log of X has an x-intercept at (1, 0), is always increasing, and is always concave down with a domain of all positive numbers and a range of all real numbers.
- 🌐 The natural log of 1 is 0, and the natural log of e is 1, with several other properties of logarithms that are important to remember, such as how to handle multiplication, division, and exponentiation in logarithmic expressions.
- 🔑 The fundamental theorem of calculus helps in finding the derivative of the natural log, which is 1/X, indicating that the slope of the natural log graph is the reciprocal of the x-coordinate.
- 🔄 The derivative of a natural log function can be simplified using the chain rule, where the derivative of the inside function is divided by the inside function itself (u'/u).
- ✅ The product rule is used when differentiating expressions involving the natural log of a product, such as x times the natural log of x, resulting in 1 + the natural log of X.
- 🔄 The chain rule is essential for differentiating more complex expressions involving natural logs, such as the natural log of the square root of x plus 1, which simplifies to 1/2 times the derivative of (x + 1)^(1/2).
- 🔍 The natural log of the natural log of X can be differentiated using u-substitution, where u equals the natural log of X, resulting in a derivative of 1/X times the natural log of X.
- 💡 The example of y = x natural log of X - 4x being a solution to the differential equation X + y - xy' = 0 demonstrates the application of derivatives in solving differential equations.
Q & A
What is the definition of a natural log?
-The natural log, also known as the logarithm to the base e, is defined as the area under the curve of 1/T from 1 to T, and it is only defined for positive values of X.
Who was the natural logarithm named after?
-The natural logarithm was named after Leonard Euler, a prominent mathematician.
What is the domain of the natural log function?
-The domain of the natural log function is all positive real numbers, as it is undefined for zero or negative values.
What is the range of the natural log function?
-The range of the natural log function is all real numbers, as it can take on any real value.
What are some properties of the natural log graph?
-The graph of the natural log has an x-intercept at (1, 0), is always increasing, and is always concave down.
What is the relationship between the natural log and the number e?
-The natural log and the number e are inverse functions of each other.
What is the derivative of the natural log of X with respect to X?
-The derivative of the natural log of X with respect to X is 1/X.
How can you simplify the derivative of a natural log with an exponent?
-You can simplify the derivative by bringing the exponent down and multiplying it by the derivative of the natural log of the base expression.
What is the process to find the derivative of a natural log using the fundamental theorem of calculus?
-The process involves recognizing the natural log as the integral of 1/T from 1 to X, then applying the fundamental theorem of calculus which states that the derivative of an integral is the integrand evaluated at the upper limit of integration, multiplied by the derivative of the upper limit.
Can you provide an example of using the properties of logarithms to simplify a complex logarithmic expression?
-Yes, for example, the natural log of x squared plus 3 can be rewritten using logarithmic properties as -1/3 times the natural log of (x squared plus 1).
What is the significance of the natural log in solving differential equations?
-The natural log is significant in solving differential equations as it allows for the simplification of expressions and the application of logarithmic properties, making the process of finding solutions more manageable.
How does the chain rule apply to the derivative of a natural log of a function?
-The chain rule is applied by taking the derivative of the inside function, multiplying it by the derivative of the outside function, and then dividing by the inside function itself, which in the case of a natural log is 1/U times U'.
What is the derivative of the natural log of a squared function?
-The derivative of the natural log of a squared function, such as x squared, is twice the natural log of x, which simplifies to 2 times 1/x.
What is a common mistake when dealing with the derivative of a natural log with an exponent?
-A common mistake is to incorrectly bring the exponent outside the natural log, which is not allowed unless it is an exponent on the base of the natural log itself.
Outlines
📚 Introduction to Natural Logs and Derivatives
This paragraph introduces the concept of natural logs, explaining that they are defined as the area under the curve of 1/T from 1 to T, and are only valid for positive values of X. The base of the natural log is the number E, named after Leonard Euler. The paragraph also discusses the properties of the natural log function, such as its graph, domain, range, and behavior (always increasing, concave down). It highlights the relationship between natural logs and the number E, stating that they are inverse functions. The speaker also mentions various properties of logarithms that were likely covered in precalculus, such as how to handle logarithms when they are multiplied, divided, or raised to a power. The paragraph sets the stage for learning how to take derivatives of natural logs.
🔍 Derivatives of Natural Logs and Logarithmic Properties
This paragraph delves into the process of finding derivatives of natural log functions. It starts by discussing the fundamental theorem of calculus and how it can be used to find the derivative of the natural log of X, which is 1/X. The speaker emphasizes the importance of understanding what this derivative means in terms of the slope of the natural log graph. Examples are provided to illustrate how to apply the chain rule and product rule when dealing with more complex natural log expressions. The paragraph also covers how to simplify expressions involving natural logs before taking derivatives, which can make the process easier. The speaker uses specific examples, such as the natural log of a squared term or a cubed term, to demonstrate these concepts. Additionally, the paragraph touches on the difference between taking the derivative of a natural log raised to a power versus a natural log of a power, highlighting the importance of understanding the underlying mathematical rules.
🧩 Advanced Derivatives and Differential Equations
The final paragraph covers more advanced topics related to derivatives of natural logs, including the use of substitution and the handling of differential equations. The speaker demonstrates how to use u-substitution to find the derivative of the natural log of the natural log of X, resulting in a simplified expression. The paragraph also discusses the difference between bringing down an exponent in front of a natural log versus a power on the entire natural log expression. The speaker provides an example of a differential equation and shows how to solve it using the derivatives of natural logs. The example involves finding the derivative of an expression involving both X and the natural log of X, and then verifying that the given function satisfies the differential equation. This paragraph concludes with a summary of the key takeaway: that the derivative of a natural log of U is U'/U, reinforcing the foundational concept introduced earlier in the script.
Mindmap
Keywords
💡Natural Logarithm
💡Derivative
💡Euler's Number (e)
💡Properties of Logarithms
💡Graph of Natural Log
💡Fundamental Theorem of Calculus
💡Chain Rule
💡Product Rule
💡Quotient Rule
💡Differential Equation
Highlights
Introduction to natural logs and their derivatives.
Definition of natural log as the area under the curve of one over T DT.
Natural log is only defined for positive values of X.
The base of natural log is the number E, named after Leonard Euler.
Natural log and E are inverse functions of each other.
Properties of natural logs include an x-intercept at (1,0), always increasing, and concave down.
Domain of natural log is all positive numbers, range is all real numbers.
Natural log graph has an asymptote on the y-axis.
Natural log of 1 is 0 and natural log of E is 1.
How to separate logs in multiplication, division, or with a power of 1.
Derivative of natural log of X is 1 over X.
Slope of the natural log graph is the reciprocal of the x coordinate.
Derivative of natural log of 2x involves multiplying by the derivative of 2x.
Using u substitution to find the derivative of natural log of x squared plus 1.
Product rule applied to x times natural log of x.
Chain rule used for the derivative of natural log of the square root of x plus 1.
Natural log of the natural log of X involves u substitution with u = natural log of X.
Difference between bringing down an exponent in a natural log and a power on the whole natural log.
Example of solving a differential equation using derivatives of natural logs.
Transcripts
Browse More Related Video
Calc AB & Calc BC Natural Logs Review

Calculus 2 Lecture 6.3: Derivatives and Integrals of Exponential Functions

Derivatives of Exponential Functions

Exponential Functions and Natural Log Review for the AP Calculus Exam

Unit V: Lec 1 | MIT Calculus Revisited: Single Variable Calculus

Math1325 Lecture 11 1 Derivatives of Logarithm
5.0 / 5 (0 votes)
Thanks for rating: