Calc AB & Calc BC Natural Logs Review
TLDRThis video script offers an insightful review of natural logarithms, a topic that can be daunting for many. It begins by explaining the basic principles of logarithm expansion and contraction, highlighting that the natural log of a product (a * b) equals the sum of the natural logs of a and b, provided both are greater than zero. The video then demonstrates how exponents within natural logs transform into coefficients outside, using the example of ln(a^b) = b * ln(a). It proceeds to illustrate the simplification of exponential functions involving natural logs, such as e^(ln(x)) simplifying to x. The script also emphasizes key values like ln(e) = 1 and ln(1) = 0, and discusses the treatment of natural logs of fractions by using the exponent property. Three important graphs of the natural log function are introduced: ln(x), ln(x) reflected, and ln(abs(x)), each with its distinct characteristics and domain. The video concludes with strategies for converting between logs and exponentials, using properties of exponents to navigate between different forms. The speaker encourages viewers to rewatch parts they find challenging and wishes them luck with their understanding of natural logs.
Takeaways
- 📚 The natural log of a product (A * B) is the sum of the natural logs: ln(A * B) = ln(A) + ln(B), with A and B > 0.
- 🔢 The natural log of a quotient (A / B) is the difference of the natural logs: ln(A / B) = ln(A) - ln(B), also with A and B > 0.
- 🔁 Exponents inside natural logs can be moved outside as coefficients: ln(A^B) = B * ln(A).
- 🚀 e raised to the power of a natural log collapses to the argument of the log: e^(ln(x)) = x.
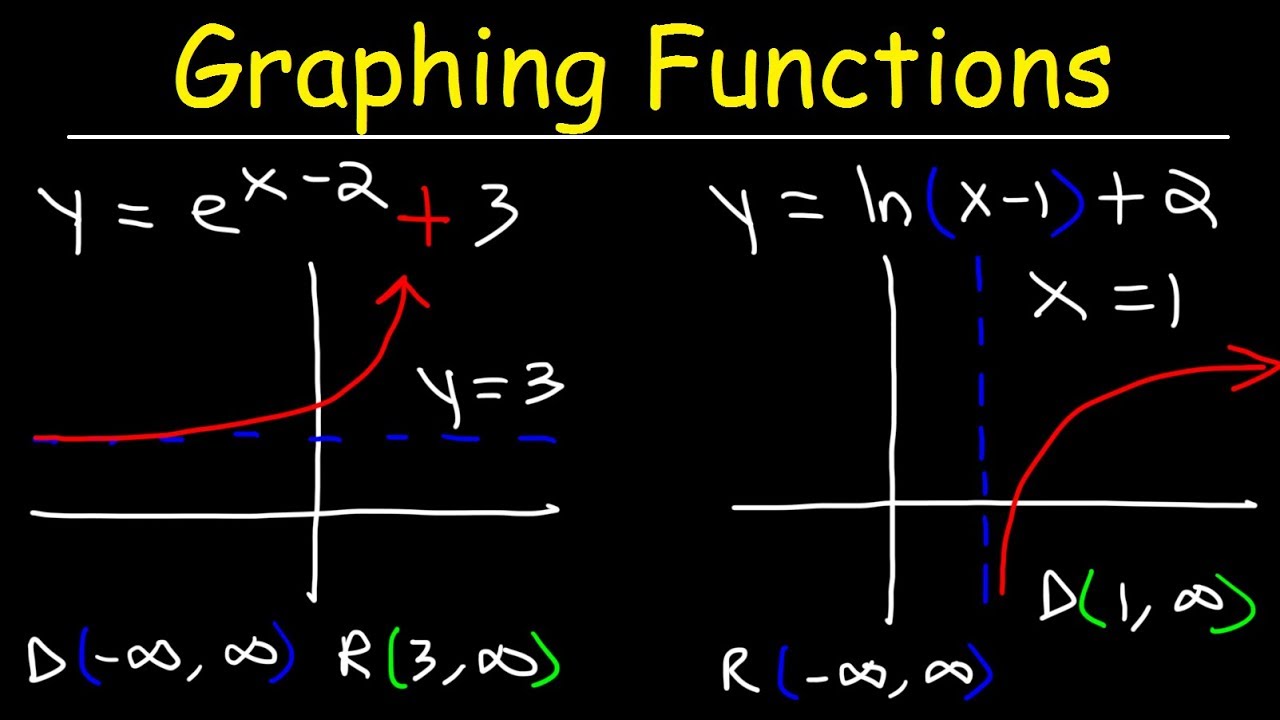
- 📉 The graph of y = ln(x) has a vertical asymptote at x = 0, passes through (1, 0), and the domain is x > 0.
- 🤔 The natural log of e is 1 because e^1 = e, and the natural log of 1 is 0 because e^0 = 1.
- ⛔ The natural log of a fraction can be expressed as the negative of the log of the denominator: ln(1/A) = -ln(A).
- 🔄 The graph of y = ln(-x) is a reflection of y = ln(x) over the y-axis, with a domain of x < 0.
- ➗ To convert from logs to exponentials, use the property e^(ln(y)) = y.
- 🔧 Getting rid of absolute value in a natural log expression may introduce a new constant but does not change the overall process.
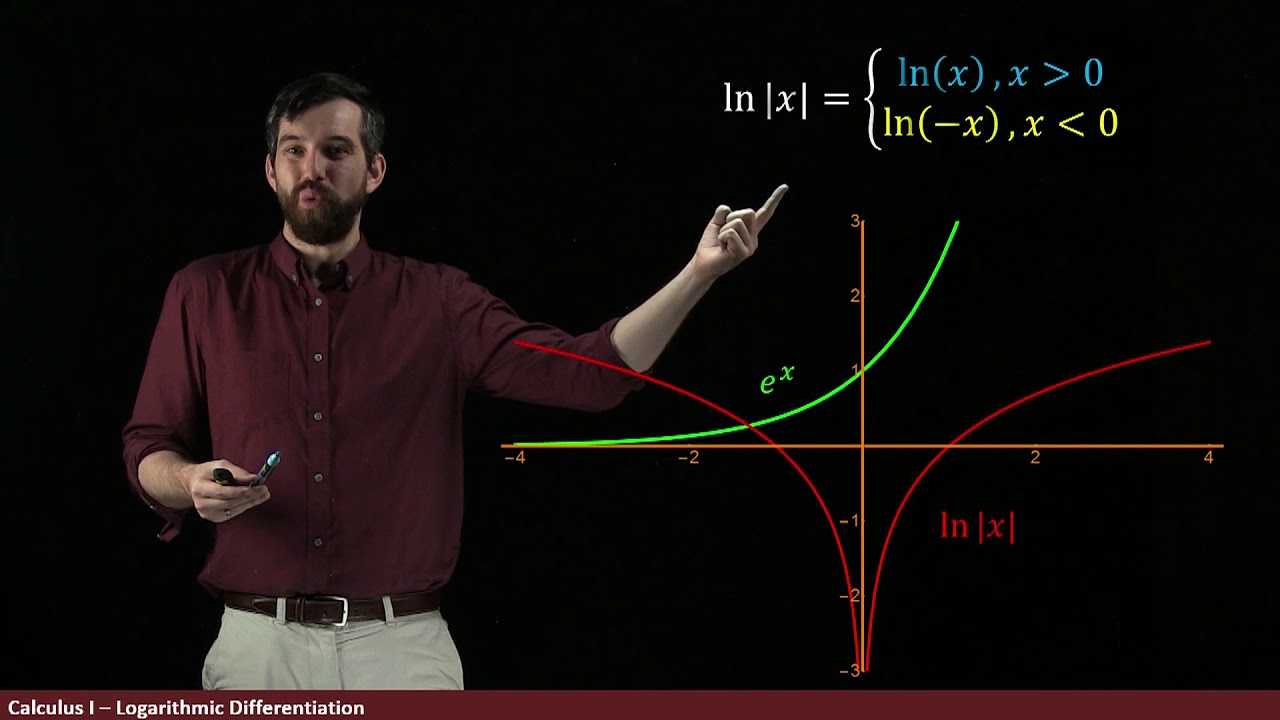
- 📈 The graph of y = ln(|x|) is an even function with a vertical asymptote at x = 0 and passes through the same points as y = ln(x) but for both positive and negative x values.
Q & A
What is the basic property of natural logarithms that allows for the expansion or contraction of logs?
-The natural log of a product (a * b) can be expanded to the natural log of a plus the natural log of b, and the natural log of a quotient (a / b) can be contracted to the natural log of a minus the natural log of b, provided that both a and b are greater than zero.
How does the presence of an exponent inside a natural log affect the expression?
-Exponents inside natural logs can become coefficients outside the log. For example, the natural log of a to the power of b is equal to b times the natural log of a.
What happens when you raise an exponential function to a natural logarithm?
-When you have an expression like e to the power of the natural log of x, it simplifies to just x. This is because e raised to the power of its own natural log is the original number, x.
What are two special values of natural logarithms that should be known?
-The natural log of e is 1, because e raised to the first power is e. The natural log of 1 is 0, because e raised to the power of 0 is 1.
How can you deal with the natural log of a fraction?
-You can use the property of exponents to transform the natural log of a fraction, such as 1/5, into the negative exponent of the natural log of the denominator, which is -1 times the natural log of 5.
What are the three important graphs of the natural logarithm function?
-The three important graphs are: y = ln(x), which has a vertical asymptote at x = 0 and passes through the point (1, 0); y = ln(-x), which is a reflection of the first graph across the y-axis; and y = ln(|x|), which is an even function with a vertical asymptote at x = 0 and passes through the same points as the first graph.
What is the domain of the natural logarithm function?
-The domain of the natural logarithm function is all x values greater than zero, as the natural log is undefined for zero and negative numbers.
How do you convert a natural logarithm equation to an exponential form?
-To convert a natural logarithm equation to an exponential form, you use the property that e to the power of the natural log of y is equal to y. For example, if you have ln(y) = 6x, you can rewrite it as e^(ln(y)) = e^(6x), which simplifies to y = e^(6x).
What is the process to handle the absolute value in a natural logarithm equation?
-To handle the absolute value in a natural logarithm equation, such as ln(|y|) = x, you can remove the absolute value by creating a new constant that accounts for both the positive and negative scenarios that the absolute value encompasses.
What is the rule for combining logarithms with different coefficients when inside a natural log?
-When you have a natural log with a coefficient, such as ln(3x), you can distribute the coefficient outside the log, resulting in the coefficient times the natural log of the variable, which is 3 times ln(x).
How can you use the properties of exponents to simplify expressions involving natural logs?
-You can use the properties of exponents to move terms involving e to the front of the expression and combine them as coefficients. This is particularly useful when you have multiple terms inside a natural log that can be simplified using the expansion or contraction rules.
What is the significance of the vertical asymptote in the graph of the natural logarithm function?
-The vertical asymptote at x = 0 in the graph of the natural logarithm function signifies that the function is undefined at that point and approaches negative infinity as x approaches zero from the right.
Outlines
📚 Understanding Natural Logs: Basic Concepts and Properties
This paragraph introduces the concept of natural logarithms, focusing on their basic properties and how they can be manipulated. It explains that the natural log of a product (a * b) is the sum of the natural logs of a and b, and the natural log of a quotient (a / b) is the difference of the natural logs of a and b, with the domain restriction that a and b must be greater than zero. The paragraph also demonstrates how to expand and contract logs using these properties. It further illustrates the rule that exponents inside natural logs can be moved outside as coefficients, and the special case where an exponential function raised to a natural log collapses to the original function. The paragraph concludes with a few important values to remember, such as the natural log of e being 1 and the natural log of 1 being 0, and touches on the treatment of natural logs of fractions.
📈 Graphing Natural Logs and Exponential Functions
The second paragraph delves into the graphical representation of natural logarithms and their corresponding exponential functions. It describes the graph of y = ln(x), noting its vertical asymptote at x = 0, the fact that the function approaches negative infinity as x approaches zero from the right, and positive infinity as x approaches infinity. It also mentions the graph passing through the point (1, 0) and having a domain of x > 0. The paragraph then contrasts this with the graph of y = ln(x) reflected across the x-axis, which has a domain from negative infinity to zero. Lastly, it discusses the graph of y = ln(abs(x)), an even function with a vertical asymptote at zero and passing through the same points as the previous graphs. The paragraph also addresses the conversion from logarithmic to exponential form, using the example of ln(y) = 6x, which is rewritten as e^(ln(y)) = e^(6x). It emphasizes the use of properties of exponents to simplify expressions and the common practice of isolating constants in such calculations.
Mindmap
Keywords
💡Natural Logarithm
💡Exponential Function
💡Domain
💡Coefficient
💡Absolute Value
💡Graph
💡Vertical Asymptote
💡Exponent Property
💡Base e
💡Reflection
💡Even Function
Highlights
Natural logs (ln) are a common topic, especially in calculus, and understanding their basic properties is crucial.
The natural log of a product (ln(a * b)) is equal to the sum of the natural logs (ln(a) + ln(b)), provided a and b are greater than zero.
The natural log of a quotient (ln(a / b)) is the difference of the natural logs (ln(a) - ln(b)), also with a and b greater than zero.
Exponentiation and natural logs are inverse operations; e^(ln(x)) simplifies to x.
Exponents inside a natural log can be brought outside as coefficients (ln(a^b) = b * ln(a)).
The natural log of e is 1, since e^1 = e, and the natural log of 1 is 0, as e^0 = 1.
Natural logs of fractions can be transformed using properties of exponents (ln(1/5) = -1 * ln(5)).
Three important graphs to know are y = ln(x), y = ln(-x), and y = ln(|x|), each with distinct characteristics.
The graph y = ln(x) has a vertical asymptote at x = 0 and passes through the point (1, 0).
The graph y = ln(-x) is a reflection of y = ln(x) across the y-axis.
The graph y = ln(|x|) is an even function with a vertical asymptote at x = 0.
Converting from natural logs to exponentials involves using the property e^(ln(y)) = y.
When dealing with absolute values in natural logs, you can create a new constant to remove the absolute value.
Understanding the properties of natural logs is essential for solving calculus problems involving logarithms.
The video provides a comprehensive review of natural logs, suitable for those needing to understand or revisit these concepts.
Different methods are shown to solve the same problem, illustrating the flexibility in approaching logarithmic expressions.
The importance of domain restrictions when working with natural logs, specifically that the arguments must be greater than zero.
Practical applications of natural logs are not explicitly discussed, but the foundational knowledge is crucial for more advanced mathematical and scientific concepts.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: