Unit V: Lec 1 | MIT Calculus Revisited: Single Variable Calculus
TLDRThis MIT lecture introduces 'Logarithms Without Exponents,' exploring the concept of logarithms from a calculus perspective. The professor explains the natural logarithm, 'ln(x)', as a function with a derivative of '1/x' and a logarithmic property, without relying on traditional exponent notation. The lecture connects the natural logarithm to the area under the curve of '1/t' and discusses its properties, including its use in solving differential equations where the rate of change is proportional to the amount present. The video concludes with a preview of exploring the inverse logarithm in future lessons.
Takeaways
- 📚 The lecture is about exploring logarithms from a calculus perspective, specifically without relying on the concept of exponents.
- 🌱 The professor begins by setting the stage for the importance of logarithms in calculus, following the basics of differential and integral calculus.
- 🔍 The concept of a logarithm is introduced as a function that can be derived from a physical principle, the rule of compound interest, represented by the differential equation 'dm/dt = km'.
- 📉 The process of integrating '1/m' is discussed, highlighting the challenge of finding a function 'L(x)' such that its derivative is '1/x', leading to the definition of the natural logarithm.
- 📈 The natural logarithm is defined as the area under the curve y = 1/t from a fixed point 'a' to 'x', emphasizing the connection between integral calculus and the natural logarithm.
- 🔑 The natural logarithm function 'ln(x)' is characterized by its derivative being '1/x' and the property that ln(1) = 0, distinguishing it within its family of logarithmic functions.
- 🔍 The professor explains how the natural logarithm can be used to rederive the product rule in calculus, showcasing the utility of logarithmic differentiation.
- 📝 The script discusses the properties of logarithmic functions, such as ln(x*y) = ln(x) + ln(y), and how these properties are derived from the fundamental logarithmic definition.
- 🎯 The number 'e' is introduced as the base of the natural logarithm, defined as the value for which the natural logarithm equals 1, and is geometrically located between 2 and 4.
- 📌 The importance of the natural logarithm in solving differential equations where the rate of change is proportional to the amount present is emphasized.
- 🔚 The lecture concludes with a summary of how the natural logarithm was derived and its applications, hinting at the topic of inverse logarithms for the next lecture.
Q & A
What is the main topic of the lecture entitled 'Logarithms Without Exponents'?
-The main topic of the lecture is to explore the concept of logarithms from a calculus perspective, without referring to them as a notation for exponents, and to understand their properties and applications in calculus.
Why does the professor mention the rule of compound interest in the context of logarithms?
-The professor mentions the rule of compound interest to illustrate a real-life situation where the rate of change of a quantity is proportional to the amount present, which leads to a differential equation that can be solved using the concept of logarithms.
What is the differential equation that the professor derives from the principle of the rate of change being proportional to the amount present?
-The differential equation derived is 'dm/dt = km', where 'm' is the quantity being measured and 'k' is a constant of proportionality.
How does the professor approach the problem of finding a function whose derivative is '1 over x'?
-The professor approaches this problem by using both differential and integral calculus methods. He first defines the function 'L of x' whose derivative is '1 over x' and then explores its properties and graph. He also uses the second fundamental theorem of calculus to express 'L of x' as an area under the curve 'y = 1/t'.
What is the significance of the function 'L of x' in the context of this lecture?
-The function 'L of x' is significant because it is a function whose derivative is '1 over x', and it is used to solve the differential equation mentioned earlier. It is also shown to have properties that align with the definition of a logarithmic function.
What is the definition of a logarithmic function according to the lecture?
-A function 'f' is called logarithmic if for all 'x1' and 'x2' in the domain of 'f', the property 'f(x1 * x2) = f(x1) + f(x2)' holds.
How does the professor use calculus to show that 'L of bx' is almost a logarithmic function?
-The professor takes the derivative of both sides of the equation 'L of bx = L of b + L of x + c' with respect to 'x' and shows that since the derivatives are equal, the original functions differ by a constant 'c'. He then evaluates this constant by setting 'x' to 1 and concludes that 'L of x' is logarithmic if 'L of 1' is 0.
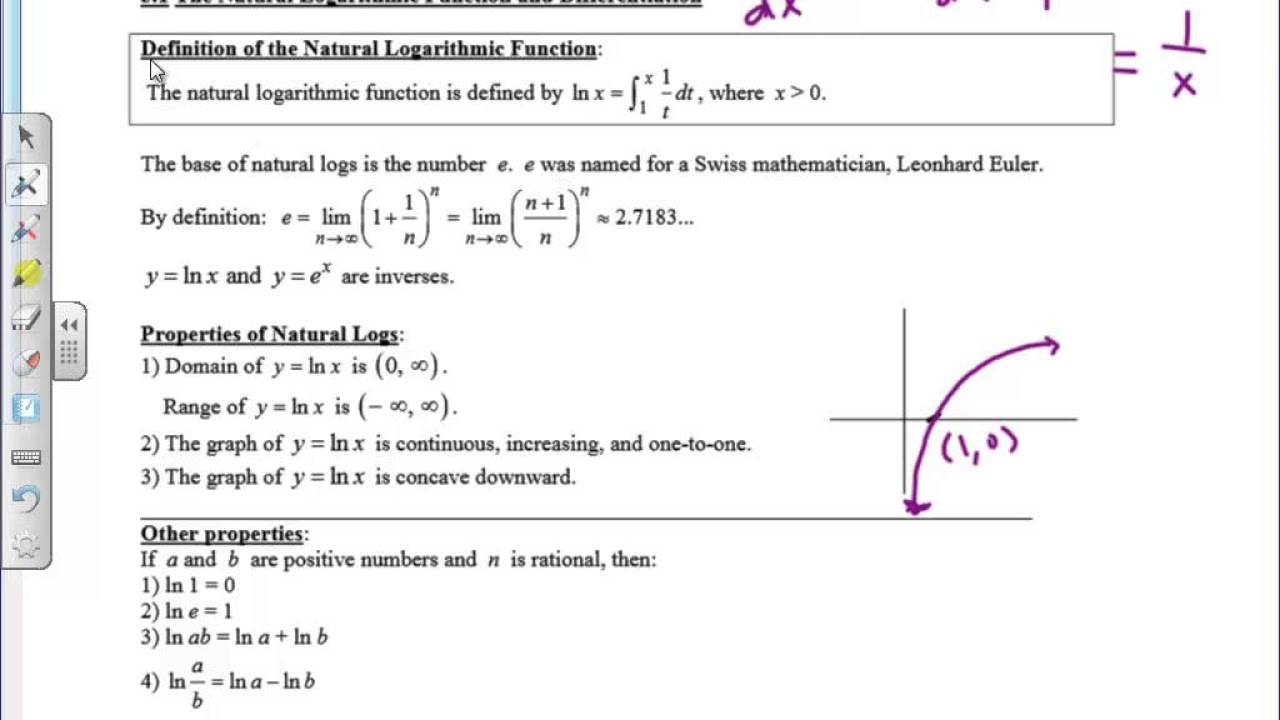
What is the natural logarithm 'ln of x' defined as in the lecture?
-The natural logarithm 'ln of x' is defined as the member of the family of 'L of x' functions that passes through the point (1,0), characterized by having a derivative of '1 over x' and satisfying the logarithmic property that the natural log of a product is the sum of the natural logs.
How does the professor relate the natural logarithm to the concept of 'e'?
-The professor relates the natural logarithm to 'e' by stating that 'e' is the number such that the natural log of 'e' is 1. He also explains that 'e' can be found either geometrically by locating where the line 'y = 1' intercepts the curve 'y = ln(x)' or by finding the area under the curve 'y = 1/t' from 1 to 'e' to be exactly 1.
What is the connection between the natural logarithm and the problem of the rate of change being proportional to the amount present?
-The connection is that the natural logarithm function, with its derivative being '1 over x', allows for the explicit solution to the problem where 'dm/dt = km'. The natural log function can be used to integrate 'dm/m', providing a solution to the differential equation in terms of 'kt + c'.
Outlines
📚 Introduction to Logarithms Without Exponents
The professor begins by acknowledging MIT OpenCourseWare's commitment to providing free educational resources, supported by donations. The lecture is titled 'Logarithms Without Exponents,' aiming to explore the concept of logarithms beyond their traditional association with exponents. The professor reviews the basics of calculus and expresses the intention to apply these principles to special functions, starting with the logarithm. A physical principle, the rule of compound interest, is introduced as an analogy to explain the logarithmic function without referencing exponents. The lecture aims to answer how to find a function whose derivative is the inverse of its argument, setting the stage for a deeper exploration of logarithms.
🔍 The Search for a Logarithmic Function
The professor delves into the challenge of finding a function, denoted as 'L of x', whose derivative is '1 over x'. This quest stems from the inability to integrate '1 over x' using standard recipes. The discussion highlights the limitations of the integral formula for 'x to the n' with respect to 'x', particularly when 'n' equals -1, which leads to a 0 denominator issue. The professor uses both differential and integral calculus to hypothesize the form of 'L of x', suggesting it belongs to a family of curves that are always rising but 'spilling water', indicating concavity. The domain of 'L' is restricted to positive numbers, reflecting the physical interpretation of the principle of compound interest.
📈 The Area Under the Curve and Logarithmic Properties
The lecture continues with an exploration of the area under the curve 'y equals 1 over t' from a fixed positive number 'a' to 'x', drawing parallels with the function 'L of x'. The professor explains how this area can be viewed as 'L of x' and how changing 'a' affects the area by a constant, akin to an indefinite integral. A brief digression into the definition of a logarithmic function is presented, highlighting the key property that the logarithm of a product is the sum of the logarithms. This leads to the deduction of other familiar logarithmic properties, such as the logarithm of 1 being 0 and the logarithm of the reciprocal being the negative of the original logarithm.
🌐 Establishing the Natural Logarithm
The professor introduces the concept of the natural logarithm, 'ln of x', as a member of the 'L of x' family that passes through the point (1,0). This function is characterized by its derivative being '1 over x' and its logarithmic property. The lecture demonstrates how to use calculus to verify whether two functions are equal by taking derivatives and comparing them. The natural logarithm is shown to satisfy the logarithmic property when 'L of 1' is set to 0, thus defining the natural logarithm without reference to exponents.
📘 Deriving the Product Rule Using Logarithms
Building on the properties of the natural logarithm, the professor rederives the product rule of differentiation using logarithmic differentiation. By taking the natural log of a product of two differentiable functions and applying the logarithmic property, the professor shows that the derivative of the product is the sum of the derivatives of the individual functions multiplied by the other function. This creative approach reinforces the utility of logarithms in calculus and their integral role in deriving fundamental rules.
🎯 The Natural Logarithm and the Number 'e'
The professor discusses the geometric and integral interpretations of the number 'e', which is defined as the base of the natural logarithm where 'ln of e' equals 1. Two methods for identifying 'e' are presented: one geometric, involving the intersection of the line 'y equals 1' with the curve 'y equals ln x', and the other integral, involving the area under the curve 'y equals 1 over t' from 1 to 'e'. The lecture also provides an estimation technique for 'e' using inscribed and circumscribed rectangles to show that 'e' lies between 2 and 4.
🔚 Conclusion and Preview of Future Topics
In conclusion, the professor summarizes the lecture by emphasizing the physical motivation for inventing the natural logarithm function, which is to solve differential equations where the rate of change is proportional to the amount present. The natural logarithm is shown to be a one-to-one function, allowing for the discussion of its inverse. The lecture ends with a preview of future topics, including the exploration of the inverse logarithm function, and a reflection on the acceleration of the course's pace as it builds upon previously introduced concepts.
Mindmap
Keywords
💡Logarithms
💡Differential Calculus
💡Integral Calculus
💡Compound Interest
💡Derivative
💡Integral
💡Logarithmic Function
💡Natural Logarithm
💡Chain Rule
💡Product Rule
💡Exponent
Highlights
The lecture introduces logarithms without the context of exponents, focusing on their intrinsic mathematical properties.
Differential and integral calculus principles are applied to special functions, starting with the logarithm function.
Logarithms are presented as a natural consequence of the rule of compound interest in a physical context.
A differential equation 'dm/dt = km' is explored to derive the logarithmic function.
The process of separating variables and integrating leads to the need for a function whose derivative is '1/m'.
The challenge of finding a function 'L(x)' such that its derivative is '1/x' is discussed.
The concept of a logarithmic function is generalized beyond exponents to any function following the logarithmic property.
Properties of logarithmic functions, such as the logarithm of a product being the sum of logarithms, are deduced.
The natural logarithm 'ln(x)' is defined as the area under the curve 'y = 1/t' from 1 to x.
The natural logarithm is shown to have a derivative of '1/x' and to satisfy logarithmic properties.
The base 'e' of the natural logarithm is characterized as the number whose natural log is 1.
The natural logarithm is used to rederive the product rule in calculus, showcasing its utility.
The geometric interpretation of the number 'e' is given, relating it to the area under the curve 'y = 1/t'.
An approximation method for estimating the value of 'e' using rectangles is introduced.
The relationship between the natural logs of 2, 'e', and 4 is established, placing 'e' between 2 and 4.
The lecture concludes by connecting the natural logarithm back to the initial problem of rates of change proportional to amount present.
The natural logarithm is identified as a key to solving differential equations of the form 'dm/dt = km'.
The concept of the inverse logarithm function is introduced as a potential topic for the next lecture.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: