Exponential Functions and Natural Log Review for the AP Calculus Exam
TLDRThe video script offers an in-depth review of exponential functions and logarithms, tailored for the AP Calculus exam. It begins with a quick overview of the properties of the exponential function e^x, emphasizing its graph, domain, range, and derivatives. The presenter then covers the natural logarithm, highlighting its graph, domain, range, and key values. The script delves into solving problems involving derivatives, integrals, and the limit definition of the derivative. It also discusses the application of exponential functions in separable differential equations and provides strategies for tackling limit problems using L'Hôpital's rule. The presenter encourages practice with separable differential equations for better exam preparation. The summary is designed to engage users by offering a comprehensive yet succinct overview of the video's content.
Takeaways
- 📈 Familiarize yourself with the properties of e^x, including its graph, domain, range, and derivatives, as they are fundamental for calculus problems.
- 🚀 Know the limits of e^x as x approaches infinity and negative infinity, which are crucial for understanding the behavior of exponential functions.
- 🔑 Memorize the derivative and antiderivative of e^x, which are e^x and e^x + C respectively, as they frequently appear in calculus problems.
- 📚 Understand the relationship between a^x, its derivative involving the natural log of a, and the antiderivative, which includes the natural log of a in its formulation.
- ✅ Recognize the common property e^(x+y) = e^x * e^y, which is often used in solving separable differential equations.
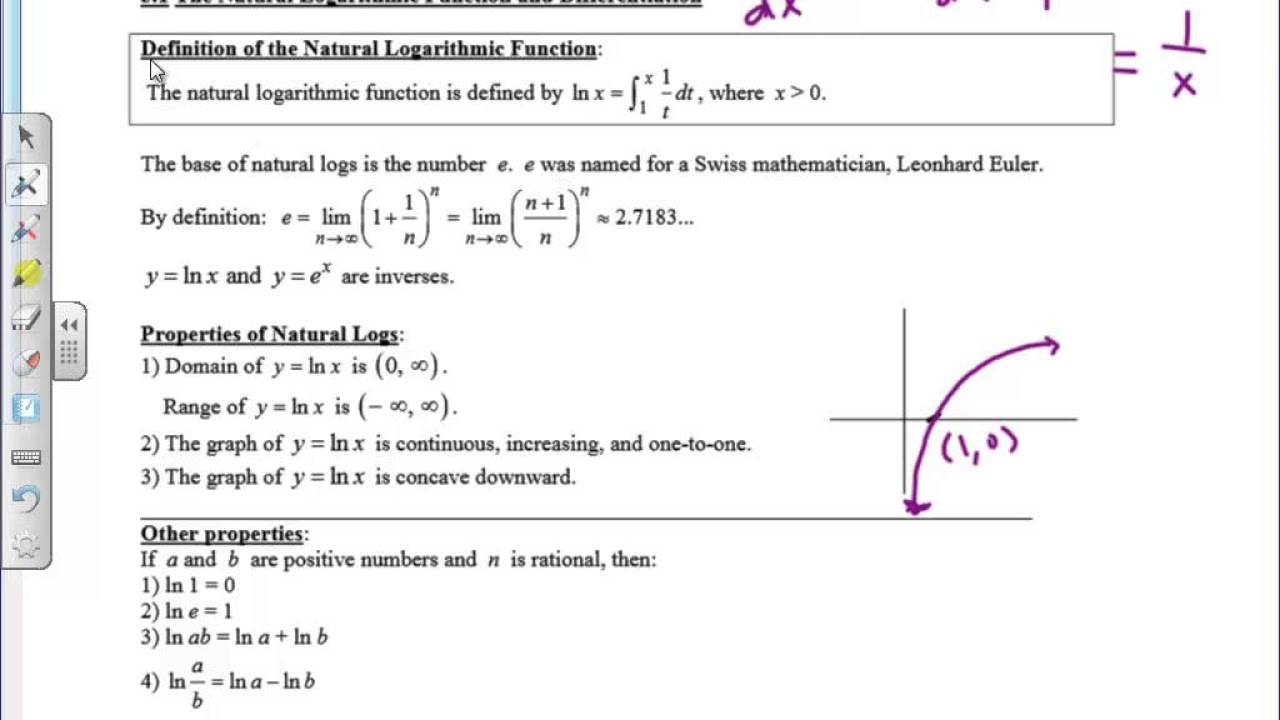
- 📊 Learn the graph of the natural log function, with an intercept at 1, 0, and understand its domain and range for solving related calculus problems.
- 🔍 Know the values of natural log for specific numbers, such as ln(1) = 0 and ln(e) = 1, which are frequently used in calculations.
- 🧮 Grasp the derivative of the natural log function, which is 1/x for x > 0, and be aware of the domain restriction to avoid negative values.
- 🧲 Master the antiderivative of the natural log, especially for the BC exam, which is x * ln(x) - x + C, often solved using integration by parts.
- 🔗 Learn the three main rules of natural logs for combining and simplifying expressions, which are essential for solving logarithmic equations.
- ⛓ Use L'Hôpital's rule when dealing with indeterminate forms such as 0/0 or ∞/∞ in limits, which is now included in the AP exam.
- 📝 Practice solving separable differential equations with logs and exponentials, as they are common in calculus exams and require a good understanding of these functions.
Q & A
What is the domain and range of the function e^x?
-The domain of e^x is all real numbers, and the range is all y values greater than zero.
What are the two important limits to remember for e^x?
-The two important limits are: as x approaches infinity, e^x approaches infinity, and as x approaches negative infinity, e^x approaches zero.
What is the derivative of e^x?
-The derivative of e^x with respect to x is e^x itself.
How can you express e^(x+y) using the properties of exponents?
-You can express e^(x+y) as e^x * e^y, which is a common property that comes up frequently in separable differential equations.
What is the value of the natural log at the intercept of its graph?
-The graph of the natural log has an intercept at the point (1, 0), meaning that the natural log of 1 is 0.
What is the domain of the natural log function?
-The domain of the natural log function is all x values greater than 0, which means there is a vertical asymptote at x equals 0.
What is the derivative of the natural log of x?
-The derivative of the natural log of x with respect to x is 1/x, with the condition that x is greater than 0.
What is the antiderivative of the natural log of x for the BC exam?
-The antiderivative of the natural log of x for the BC exam is x * ln(x) - x plus a constant C.
What are the three rules for natural logs that are commonly used in calculus?
-The three rules are: (1) a * ln(x) can be written as ln(x^a), (2) ln(a) + ln(b) is equal to ln(a * b), and (3) ln(a) - ln(b) is equal to ln(a/b).
What is the process of finding the slope of the tangent line to a function at a given point?
-To find the slope of the tangent line, you first find the derivative of the function using the chain rule if necessary. Then, substitute the given point into the derivative to find the slope.
How can you evaluate the limit as x approaches infinity of ln(e^(4x) - x) over 2x?
-You can either disregard the x term as it approaches zero compared to e^(4x), or use L'Hôpital's rule to find the limit, which will involve taking the derivative of the numerator and denominator and then re-evaluating the limit.
What is the general approach to solving separable differential equations involving exponentials and logs?
-You would separate the variables, integrate both sides, and then solve for the function. This often involves using properties of exponentials and logs to simplify the equation.
Outlines
📚 Exponentials and Logs Overview for AP Calculus
This paragraph introduces the video's focus on reviewing Exponentials and Logs for AP Calculus exams. It assumes the viewer has completed the course or the relevant topic in class. The speaker plans to cover properties of e^x, natural logs, and work through problems involving derivatives, integrals, and the limit definition of the derivative. Key properties such as the graph of e^x, its domain and range, and important limits are emphasized. The derivative and antiderivative of e^x are also discussed, alongside a mention of a common property involving e^(x+y). The paragraph sets the stage for a deep dive into Exponential functions and their role in calculus problems.
🔢 Derivatives, Exponentials, and Natural Logarithms
The second paragraph delves into the specifics of derivatives and exponentials. It begins with a straightforward application of the chain rule to e^(2x) and progresses to more complex examples such as the derivative of 5^(3x). The importance of the natural log properties is highlighted, with a focus on the graph of the natural log function, its domain and range, and key values. The rules of logarithms, such as the properties involving multiplication and division of logs, are also covered. The paragraph concludes with an invitation for viewers to attempt problems on their own before the speaker works through them, emphasizing the use of these properties in solving differential equations and limits.
📈 Calculus Problems Involving Limits and Implicit Differentiation
This paragraph presents a series of calculus problems that involve finding derivatives and dealing with limits. The speaker uses l'Hôpital's rule and the limit definition of the derivative to solve indeterminate forms. Implicit differentiation is also covered, where the derivative of e^(xy) is found, and a point is used to determine the value of dy/dx. The paragraph showcases a variety of techniques for tackling calculus problems, from basic differentiation to more advanced concepts like l'Hôpital's rule and implicit differentiation.
🧮 Integration, Average Value, and Separable Differential Equations
The focus shifts to integration problems, including reversing the chain rule and using u-substitution to find antiderivatives. The concept of an accumulation function is introduced when an explicit antiderivative cannot be calculated. Average value is calculated using definite integrals, and a separable differential equation is solved using integration. The paragraph emphasizes the importance of recognizing patterns in calculus problems and applying the appropriate method, whether it be u-substitution, integration by parts, or recognizing properties of functions to simplify the problem.
🎓 Final Thoughts and Practice Recommendations for AP Exam
In the final paragraph, the speaker wraps up the review by encouraging further practice, specifically with separable differential equations, as they frequently involve logarithms and exponentials. The speaker provides a brief review of key concepts and offers a word of encouragement for the upcoming AP exam. The paragraph ends with a reminder that practice and familiarity with various calculus techniques are crucial for success on the exam.
Mindmap
Keywords
💡Exponential Functions
💡Natural Logs
💡Derivatives
💡Integrals
💡Limits
💡Chain Rule
💡Product Rule
💡Separable Differential Equations
💡L'Hôpital's Rule
💡Antiderivatives
💡Average Value
Highlights
Review of Exponentials and Logs for AP Calculus exams, assuming completion of the course.
Quick overview of Exponentials and natural logs, followed by problem-solving involving derivatives, integrals, and limits.
Memorization of properties of e^x, including its graph, domain, range, and derivatives.
Understanding the limits of e^x as x approaches infinity and negative infinity.
Derivative of e^x is e^x itself, and the antiderivative includes a +C.
Properties of a^x, including the derivative involving natural log of a.
Common property e^(x+y) = e^x * e^y, important for separable differential equations.
Natural log graph has an intercept at 1, 0, and understanding its domain and range is crucial.
Derivative of the natural log of X is 1/X, with the domain X > 0.
Antiderivative of natural log includes X * ln|X| + C, especially for BC exam.
Three key rules for natural logs to be used in various problem-solving scenarios.
Using l'Hôpital's rule for limits as X approaches infinity, a common situation in exams.
Solving problems involving derivatives of Exponentials and Logs, such as chain rule applications.
Finding the tangent line to a function involving e^x at a specific point using derivatives.
Solving for velocity in a position function using product rule and chain rule.
Approaching limit problems using both direct substitution and l'Hôpital's rule.
Dealing with implicit differentiation and the common mistake of forgetting the derivative of a constant.
Solving integral problems using reverse chain rule and u-substitution techniques.
Understanding the concept of average value in integral calculus and its calculation.
Solving separable differential equations by integrating both sides and applying initial conditions.
Using the limit definition of the derivative in multiple-choice questions and the importance of recognizing functions.
Advice on practicing separable differential equations for better understanding and preparation.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: