Conditional & absolute convergence | Series | AP Calculus BC | Khan Academy

Khan Academy

3 Mar 201505:00

EducationalLearning

32 Likes 10 Comments

TLDRThis video script discusses the concept of conditional and absolute convergence in the context of infinite series. It starts with an example of an alternating series that converges, then explores what happens when taking the absolute value of its terms, leading to the divergence of the harmonic series. The script explains the difference between conditional convergence, where a series converges but the absolute values diverge, and absolute convergence, where the series still converges after taking absolute values, using a geometric series as an example. The video aims to clarify these nuanced aspects of series convergence.

Takeaways

📚 The script introduces the concept of the Alternating Series Test, a method used to determine if an infinite alternating series converges.
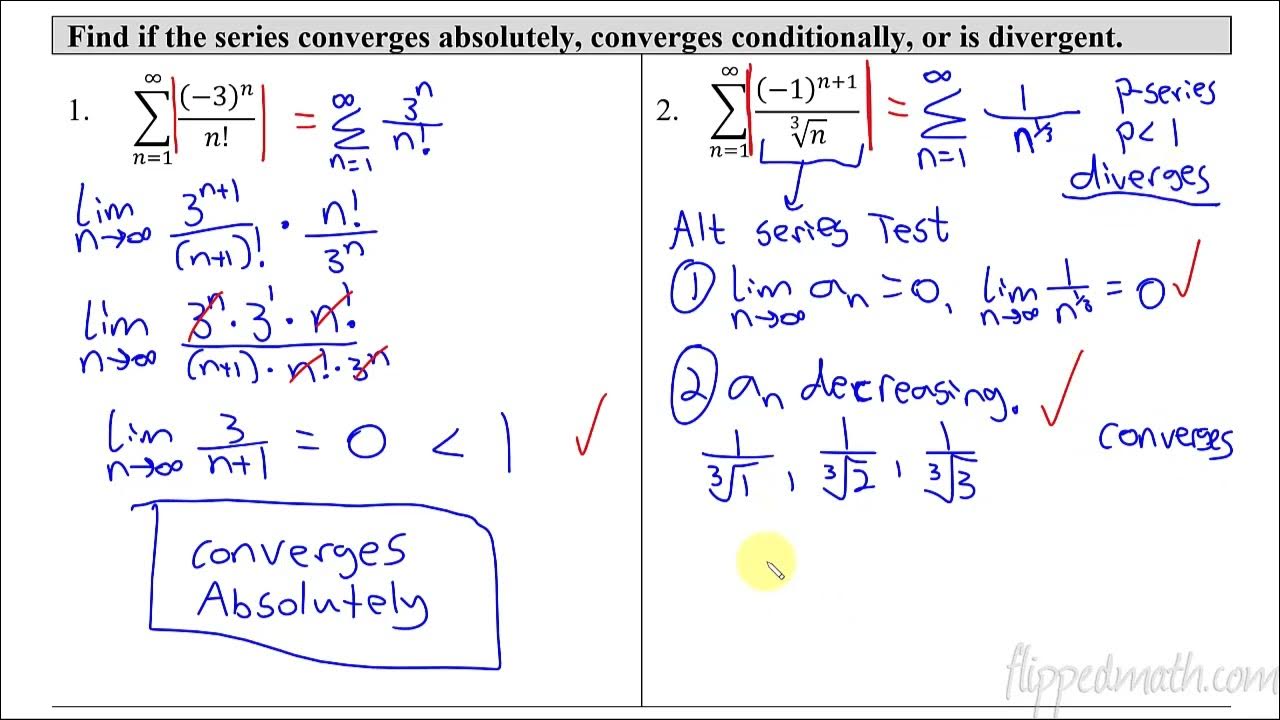
🌟 The example series provided is \( \sum_{n=1}^{\infty} (-1)^{n+1} \frac{1}{n} \), which converges according to the Alternating Series Test.
🔍 When taking the absolute value of each term in the series, the result is the Harmonic Series \( \sum_{n=1}^{\infty} \frac{1}{n} \), which is known to diverge.
📉 The divergence of the Harmonic Series highlights the conditional nature of convergence for the original alternating series.
🔑 A series that converges but diverges when taking the absolute value of its terms is said to converge conditionally.
🔄 The concept of 'absolute convergence' is introduced for series that still converge even after taking the absolute value of each term.
🌐 The script suggests reviewing the Alternating Series Test and the proof of divergence for the Harmonic Series on Khan Academy.
📈 An example of absolute convergence is given with a geometric series \( \sum_{n=1}^{\infty} (-\frac{1}{2})^{n+1} \), which also converges when considering the absolute value of its terms.
📝 The importance of understanding the nuances of convergence is emphasized, distinguishing between conditional and absolute convergence.
📚 The script serves as an educational resource to deepen the understanding of series convergence beyond the basic concepts of convergence or divergence.
🎓 It encourages viewers to explore further by watching relevant videos on Khan Academy for a deeper understanding of the topics discussed.

Q & A

What is the alternating series test?
-The alternating series test is a method used in calculus to determine whether an infinite series with alternating signs converges. It is applicable when the terms of the series decrease in absolute value and approach zero.
What is the series used as an example in the video to apply the alternating series test?
-The series used as an example is \( \sum_{n=1}^{\infty} (-1)^{n+1} \frac{1}{n} \), which starts with 1, -1/2, 1/3, -1/4, and so on.
What happens when you take the absolute value of each term in the alternating series?
-Taking the absolute value of each term in the series results in the sum \( \sum_{n=1}^{\infty} \frac{1}{n} \), which is the harmonic series.
Is the harmonic series convergent or divergent?
-The harmonic series is divergent, as shown in the video and proven in various mathematical sources.
What does it mean for a series to converge conditionally?
-A series converges conditionally if it converges when the terms are not necessarily non-negative, but the series formed by taking the absolute value of each term diverges.
What is the difference between conditional convergence and absolute convergence?
-A series converges absolutely if it converges and remains convergent even when the absolute values of the terms are taken. Conditional convergence occurs when the series converges but diverges when the absolute values of the terms are taken.
What is an example of a series that converges absolutely?
-An example given in the video is the geometric series \( \sum_{n=1}^{\infty} (-1/2)^{n+1} \), which converges and also converges when the absolute values of the terms are taken.
Why is the geometric series \( \sum_{n=1}^{\infty} (-1/2)^{n+1} \) considered to converge?
-This geometric series converges because the absolute value of the common ratio (1/2) is less than one, which is a condition for convergence in geometric series.
What is the sum of the series \( \sum_{n=1}^{\infty} (-1/2)^{n+1} \) when the absolute value of each term is taken?
-When the absolute value of each term is taken, the series becomes \( \sum_{n=1}^{\infty} \frac{1}{2^{n+1}} \), which is still a convergent geometric series.
What is the significance of the absolute value in determining the type of convergence of a series?
-The absolute value of the terms in a series can determine whether the series converges absolutely or conditionally. If the series converges regardless of the signs of the terms, it is said to converge absolutely. If it only converges when the signs are ignored (i.e., when the absolute values are taken), it converges conditionally.

Outlines

00:00

🔍 Introduction to Alternating Series Test

This section introduces the concept of the alternating series test using an infinite series example: the series from n equals one to infinity of (-1)^(n+1)/n. The voiceover mentions that this series, represented by 1 - 1/2 + 1/3 - 1/4 and so on, converges according to the alternating series test. It briefly reviews the proof of convergence and sets up the exploration of absolute values of the series terms.

📈 Absolute Value and Harmonic Series

The narrator discusses the result of taking the absolute value of each term in the alternating series. The absolute value changes the series to the famous harmonic series, 1 + 1/2 + 1/3 + ..., which diverges. This section highlights the concept of conditional convergence, where a series converges but its absolute value counterpart diverges.

🌀 Conditional vs Absolute Convergence

The explanation differentiates between conditional and absolute convergence. If a series converges but its absolute value diverges, it is conditionally convergent. Conversely, if the series and its absolute value both converge, it is absolutely convergent. An example of a geometric series is used to illustrate absolute convergence, showing that when the absolute value of terms still leads to a convergent series, it is absolutely convergent.

🔄 Geometric Series Example

The narrator provides an example of a geometric series, demonstrating that the series and its absolute value both converge. The geometric series considered is the sum from n equals one to infinity of (-1/2)^(n+1). The absolute value of this series, 1/2^(n+1), also converges, confirming that the series converges absolutely. This example reinforces the distinction between conditional and absolute convergence.

🧩 Nuances of Convergence

This part concludes the discussion by summarizing the nuances of convergence. It reiterates that understanding whether a series converges conditionally or absolutely provides deeper insight into its behavior. The importance of considering the absolute value of series terms in determining the type of convergence is emphasized, rounding off the video’s exploration of these convergence concepts.

Mindmap

Keywords

💡Alternating Series Test

The Alternating Series Test is a mathematical test used to determine the convergence of an infinite series where the terms alternate in sign. In the video, it is used to prove that the series with terms like -1^(n+1)/n converges. The test checks if the absolute value of the terms decreases and approaches zero, and if the series of the absolute values converges, then the original alternating series converges as well.

💡Convergence

Convergence in the context of series refers to the property where the sum of an infinite series approaches a finite value. The video discusses this in relation to the alternating series test, where the series converges, and in the case of the absolute value of terms, where the convergence might change, indicating conditional or absolute convergence.

💡Harmonic Series

The Harmonic Series is a well-known infinite series where each term is the reciprocal of a natural number, i.e., 1 + 1/2 + 1/3 + ... The video mentions this series as an example of a series that diverges, contrasting with the series that converges conditionally when the absolute values are not taken.

💡Conditionally Convergent

A series is said to be conditionally convergent if it converges, but the series of its absolute values diverges. The video uses the example of the series with alternating signs and demonstrates that when the absolute values are taken, the resulting series diverges, hence it is conditionally convergent.

💡Absolutely Convergent

A series is absolutely convergent if both the series itself and the series of its absolute values converge. The video contrasts this with conditional convergence by showing a geometric series with a common ratio of absolute value less than one, which converges regardless of the sign of its terms.

💡Geometric Series

A geometric series is an infinite series where each term after the first is found by multiplying the previous term by a constant called the common ratio. In the video, a geometric series with a negative common ratio and its absolute value is used to illustrate absolute convergence.

💡Common Ratio

In the context of a geometric series, the common ratio is the factor by which we multiply each term to get the next. The video discusses the importance of the absolute value of the common ratio being less than one for the series to converge, which is a key property of geometric series.

💡Absolute Value

The absolute value of a number is the non-negative value of that number without regard to its sign. The video script explores the impact of taking the absolute value of terms in a series, which can change the convergence properties of the series.

💡Divergence

Divergence in the context of series means that the sum of an infinite series does not approach a finite value but instead increases without bound. The video contrasts divergence with convergence, specifically mentioning the harmonic series as an example of divergence.

💡Infinite Series

An infinite series is a series that has an infinite number of terms. The video discusses various types of infinite series, such as alternating series and geometric series, and their properties of convergence or divergence.

💡Reciprocal

A reciprocal of a number is the value which, when multiplied by the original number, yields the product one. In the context of the harmonic series mentioned in the video, each term is the reciprocal of a natural number, highlighting the series' divergence.

Highlights

Introduction of the alternating series test with an example series.

Proof of convergence for the example series using the alternating series test.

Exploration of the effect of taking the absolute value of terms in a series.

Transformation of an alternating series into a harmonic series by taking absolute values.

Explanation of the harmonic series and its divergence.

Conditional convergence concept introduced through the harmonic series example.

Definition of 'conditionally convergent' series.

Definition of 'absolutely convergent' series.

Geometric series example demonstrating absolute convergence.

Verification of the geometric series' convergence after taking absolute values.

Discussion on the importance of the common ratio in determining series convergence.

Clarification of the difference between conditional and absolute convergence.

Introduction of nuances in series convergence through examples.

Highlighting the practical application of understanding conditional and absolute convergence in series.

Encouragement to review the video on the alternating series test for further understanding.

Suggestion to look up the proof of divergence for the harmonic series on Khan Academy.

Transcripts

Browse More Related Video

Absolute and Conditional Convergence

Conditional and Absolute Convergence for Infinite Series

AP Calculus BC Lesson 10.9

Calculus BC – 10.9 Determining Absolute or Conditional Convergence

Ratio test | Series | AP Calculus BC | Khan Academy

Alternating Series, Types of Convergence, and the Ratio Test