Mean deviation, variance and standard deviation of grouped data.

Oninab (Educational) Resources
23 Jul 201912:29
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial video guides viewers through calculating the mean, mean deviation, variance, and standard deviation of grouped data, using a dataset of students' recorded weights. The presenter demonstrates the process step-by-step, from finding the midpoints of class intervals to summing products of frequency and midpoint values, and finally applying the respective formulas to derive the statistical measures. The video concludes by encouraging viewers to subscribe to the YouTube channel for more educational content.

Takeaways
  • ๐Ÿ“š The tutorial focuses on calculating statistical measures for grouped data, specifically mean, mean deviation, variance, and standard deviation.
  • ๐Ÿ”ข To find the mean, the formula used is the summation of frequency times the mid-value (FX) divided by the summation of frequencies (F).
  • ๐Ÿ“‰ The mid-value (X) for each class interval is calculated by taking the average of the lower and upper bounds of the interval.
  • ๐Ÿ“ˆ The FX value is obtained by multiplying the frequency (F) of each class interval by its respective mid-value (X).
  • ๐Ÿงฎ The summation of frequencies and the summation of FX are used to calculate the mean of the distribution, which in this case is 66.94 kg.
  • ๐Ÿ“Š Mean deviation is calculated by summing the absolute values of the difference between each X and the mean (X-bar), multiplied by their respective frequencies, and then dividing by the total frequency.
  • โž— The formula for variance involves summing the squared differences between each X and X-bar, multiplied by their frequencies, and then dividing by the total frequency.
  • ๐Ÿ“ The standard deviation is the square root of the variance, providing a measure of the dispersion of the data points around the mean.
  • ๐Ÿ“ Additional columns for X, FX, absolute value of X - X-bar, and the product of frequency and X - X-bar are created to facilitate calculations.
  • ๐Ÿ“‰ The tutorial provides step-by-step calculations for each statistical measure, ensuring a clear understanding of the process.
  • ๐Ÿ“š The final calculated values for the distribution are a mean of 66.94 kg, a mean deviation of 5.7472, a variance of 51.2064, and a standard deviation of approximately 7.1559.
Q & A

  • What is the main topic of the tutorial?

    -The main topic of the tutorial is the calculation of mean, mean deviation, variance, and standard deviation for grouped data in statistics.

  • What is the first step in calculating the mean of grouped data?

    -The first step is to calculate the mid-value (X) for each class interval, which represents the average value of the data within that interval.

  • How is the mid-value of a class interval calculated?

    -The mid-value of a class interval is calculated by adding the lower and upper bounds of the interval and dividing by 2.

  • What does 'FX' represent in the context of the tutorial?

    -'FX' represents the product of the frequency (F) and the mid-value (X) of a class interval.

  • What is the formula used to calculate the mean of grouped data?

    -The formula used to calculate the mean of grouped data is the summation of FX divided by the summation of F.

  • What is the mean weight calculated in the tutorial?

    -The mean weight calculated in the tutorial is 66.94 kg.

  • How is mean deviation calculated for grouped data?

    -Mean deviation is calculated by summing the absolute values of the difference between each mid-value (X) and the mean (Xฬ„), multiplied by the frequency, and then dividing by the total frequency.

  • What is the mean deviation of the distribution in the tutorial?

    -The mean deviation of the distribution in the tutorial is 5.7472.

  • How is variance calculated for grouped data?

    -Variance is calculated by summing the squares of the differences between each mid-value (X) and the mean (Xฬ„), multiplied by the frequency, and then dividing by the total frequency.

  • What is the variance of the distribution in the tutorial?

    -The variance of the distribution in the tutorial is 51.2064.

  • How is standard deviation derived from variance?

    -Standard deviation is derived from variance by taking the square root of the variance value.

  • What is the standard deviation of the distribution in the tutorial?

    -The standard deviation of the distribution in the tutorial is approximately 7.1559.

Outlines
00:00
๐Ÿ“Š Introduction to Calculating Statistics for Grouped Data

The video tutorial introduces the topic of calculating the mean, mean deviation, variance, and standard deviation for grouped data. The example problem involves calculating these statistics for weights recorded in kilograms by final-year students. The mean is calculated using the summation of FX over the summation of F, and additional columns for mid-values (X) and the product of frequency and mid-values (FX) are created.

05:02
๐Ÿ”ข Calculating Mean Deviation

The process for calculating mean deviation is explained. The mean deviation formula involves the summation of the absolute value of X - mean (Xฬ„) multiplied by the frequency, divided by the summation of the frequency. Additional columns for the absolute difference and the product of this difference with the frequency are created. The tutorial then substitutes the values into the mean deviation formula to get the result.

10:07
๐Ÿ“ Calculating Variance and Standard Deviation

This section covers the calculation of variance and standard deviation. The variance is calculated using the formula that involves squaring the differences (X - Xฬ„) and multiplying by the frequency. A new column for these squared differences multiplied by the frequency is created, summed, and substituted into the variance formula. The standard deviation is then found by taking the square root of the variance. The final results for variance and standard deviation are provided, concluding the tutorial.

Mindmap
Keywords
๐Ÿ’กStatistics
Statistics is the discipline that concerns the collection, analysis, interpretation, presentation, and organization of data. In the video, the theme revolves around statistical concepts applied to grouped data, showcasing how to calculate various statistical measures such as mean, mean deviation, variance, and standard deviation.
๐Ÿ’กGrouped Data
Grouped data refers to the arrangement of data into groups or intervals, which is a common method in statistics for handling large datasets. In the video, the script discusses the calculation of statistical measures for grouped data, specifically the weight of students, which is organized into class intervals.
๐Ÿ’กMean
Mean, often referred to as the average, is a measure of central tendency that is calculated by summing all the values in a dataset and dividing by the number of values. The video script explains the formula for calculating the mean of grouped data, using the summation of the product of frequency and the mid-value of each class interval.
๐Ÿ’กMean Deviation
Mean deviation is a measure of the average distance of data points from the mean. It is calculated as the sum of the absolute differences between each data point and the mean, divided by the total number of data points. The script demonstrates how to calculate the mean deviation for the grouped data of students' weights.
๐Ÿ’กVariance
Variance is a measure of the dispersion or spread of a set of data points. It is calculated as the average of the squared differences from the mean. In the video, variance is computed for the grouped data by summing the squared differences between each data point's mid-value and the mean, multiplied by their respective frequencies.
๐Ÿ’กStandard Deviation
Standard deviation is a measure that indicates the amount of variation or dispersion of a set of values. It is the square root of the variance and provides a sense of the average distance of each data point from the mean. The script concludes with the calculation of the standard deviation from the previously calculated variance.
๐Ÿ’กClass Interval
A class interval is a range of values that groups data into manageable segments. In the context of the video, class intervals are used to represent the weight ranges of the students, and the mid-value of each interval is calculated to facilitate the computation of statistical measures.
๐Ÿ’กFrequency
Frequency refers to the number of occurrences of a particular value or set of values in a dataset. In the video, the frequency is used in conjunction with the mid-value of class intervals to calculate the product FX, which is essential for determining the mean and other statistical measures.
๐Ÿ’กMid-Value
The mid-value of a class interval is the arithmetic mean of the lower and upper bounds of that interval. The script uses mid-values to represent the central value of each class interval in the calculation of statistical measures for grouped data.
๐Ÿ’กFX
FX, in the context of the video, represents the product of the frequency (f) and the mid-value (X) of a class interval. This product is used in the calculation of the mean and other statistical measures for grouped data, as it accounts for the contribution of each interval to the overall dataset.
๐Ÿ’กAbsolute Value
Absolute value is a mathematical operation that returns the non-negative value of a number, regardless of its sign. In the video, the absolute value is used in the calculation of mean deviation to ensure that all differences from the mean are considered positively, regardless of whether they are above or below the mean.
Highlights

Introduction to the tutorial on statistics for grouped data.

Explanation of terms like mean, mean deviation, variance, and standard deviation.

Demonstration of calculating the mean using the formula summation of FX over summation of F.

Creation of additional columns for X (mid-value) and FX (frequency multiplied by X) for grouped data.

Calculation of the mid-value for each class interval to represent X.

Determination of FX by multiplying frequency by the mid-value.

Summation of frequency and the product of frequency and X to find the mean.

Calculation of the mean weight distribution as 66.94 kg.

Introduction to mean deviation and its formula.

Calculation of absolute values of x - x-bar for each class interval.

Determination of the product of frequency and the absolute value of x - x-bar.

Calculation of the mean deviation of the distribution as 5.7472.

Explanation of variance calculation using the formula summation of F(x - x-bar) squared.

Process of squaring the differences and multiplying by frequency for variance calculation.

Summation of squared differences multiplied by frequency to find the variance.

Calculation of the variance of the distribution as 51.2064.

Introduction to standard deviation as the square root of variance.

Calculation of the standard deviation as 7.1559.

Conclusion of the tutorial with a summary of calculated statistics.

Encouragement to subscribe to the YouTube channel for more educational content.

Transcripts

Browse More Related Video

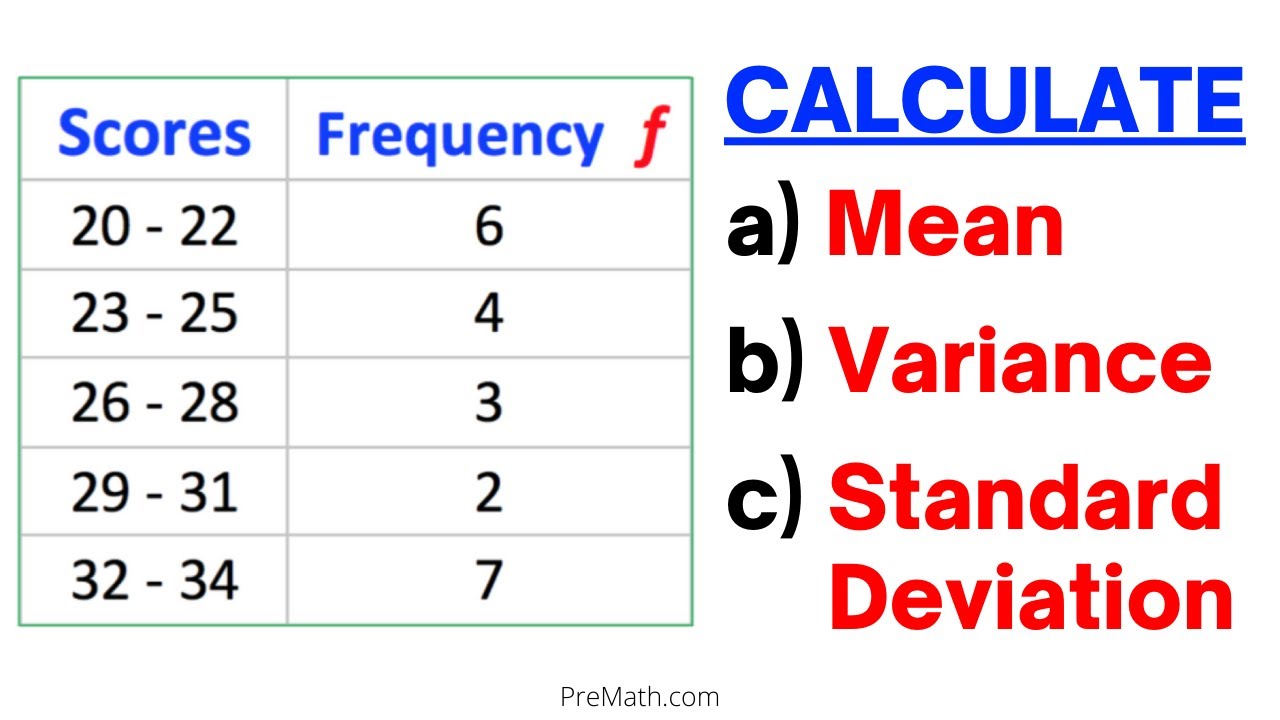
Can You Find Mean, Variance, & Standard Deviation of a Frequency Grouped DataTable? Simple Tutorial

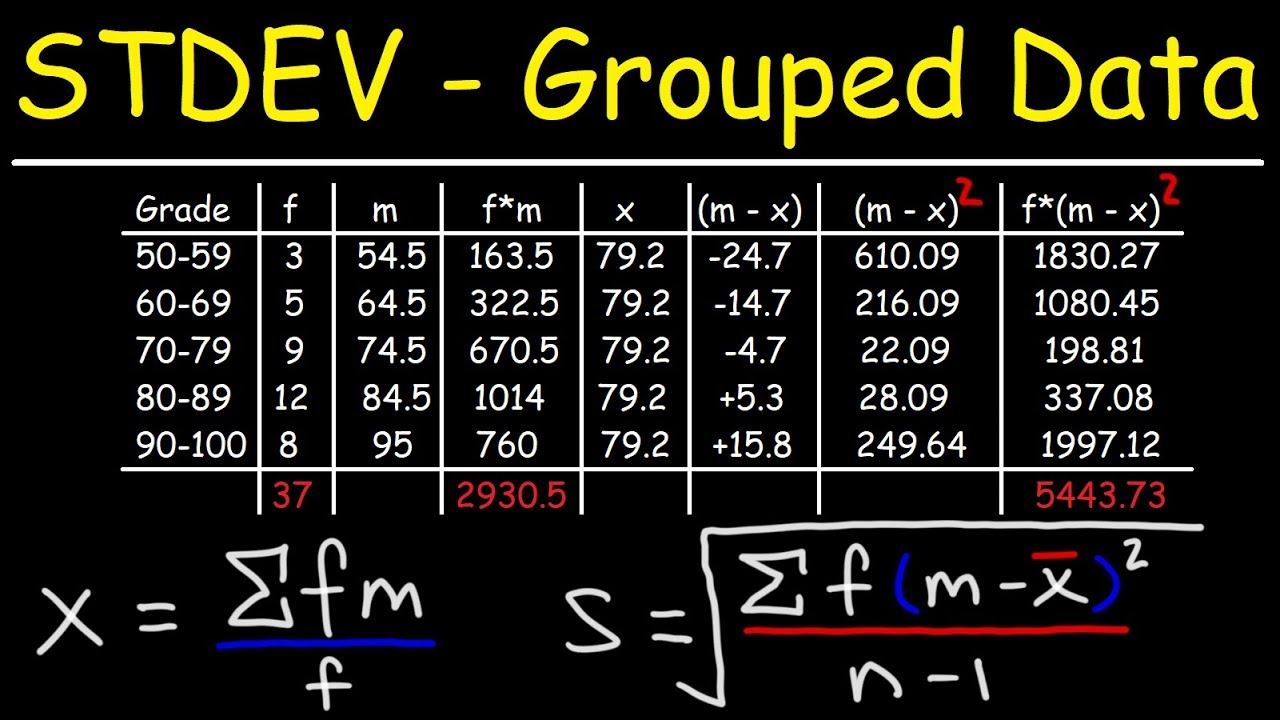
How To Calculate The Standard Deviation of Grouped Data

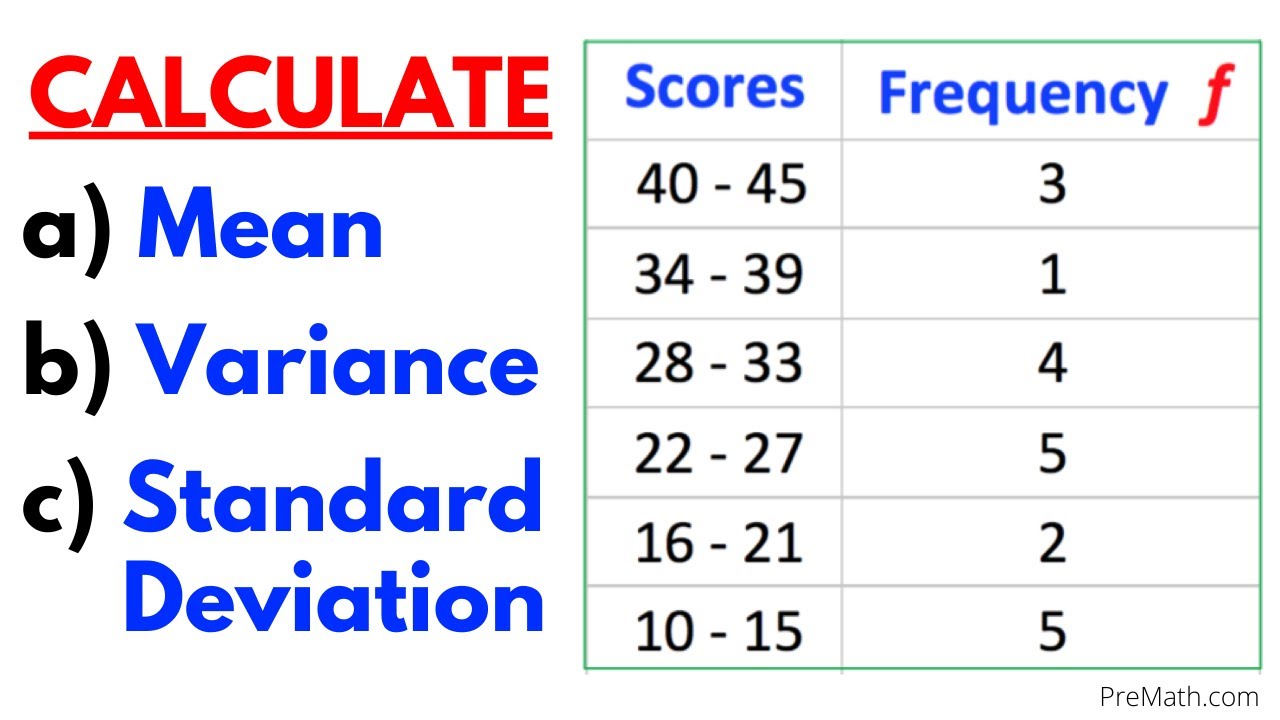
Find the Mean, Variance, & Standard Deviation of Frequency Grouped Data Table| Step-by-Step Tutorial

Variance - Clearly Explained (How To Calculate Variance)

Measures of Dispersion (Grouped Data) | Basic Statistics

Measures of Dispersion (Ungrouped Data) | Basic Statistics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
StatisticsGrouped DataMean CalculationDeviationVarianceStandard DeviationTutorialEducationalData AnalysisMathematicsYouTube Channel