Consolidation: Two-Body Collision Problems
TLDRThis video script explains the key differences between elastic and inelastic collisions, emphasizing the conservation of momentum and energy principles. It clarifies that while total kinetic energy is not conserved in inelastic collisions, it remains unchanged in elastic ones. The script uses a 2 kg and 3 kg collision example to illustrate the outcomes, highlighting that perfectly inelastic collisions minimize kinetic energy, whereas perfectly elastic ones maintain it. The video also visually demonstrates the momentum and velocity time graphs for each case, showing how impulse and relative speeds of separation vary, and concludes with an invitation for viewers to solve similar problems and analyze the graphs themselves.
Takeaways
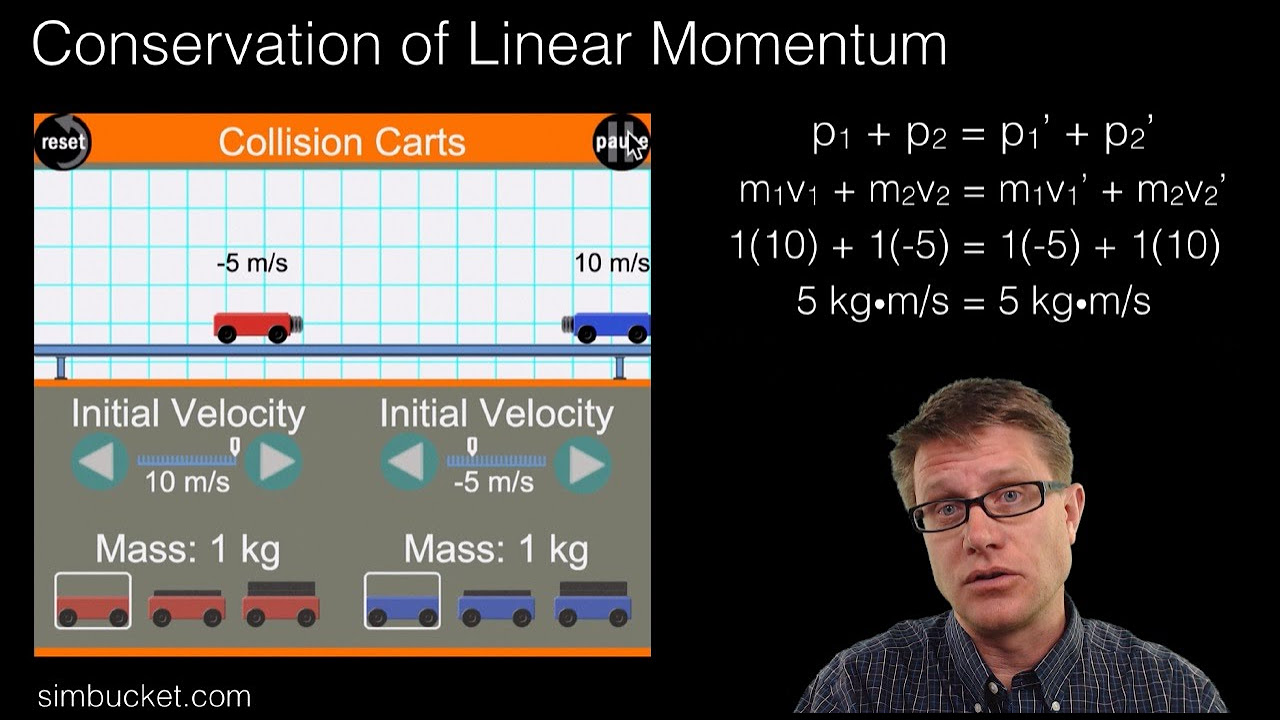
- 🌟 The principle of conservation of momentum (pcom) states that total momentum is conserved during a collision.
- 🔄 The principle of conservation of energy (pcoe) asserts that total energy is conserved during a collision.
- ⚖️ Elastic vs. inelastic collisions differ in the conservation of total kinetic energy (K), with elastic collisions conserving total K and inelastic collisions resulting in minimum K.
- ❌ The statement 'total K is conserved during an elastic collision' is incorrect; it should be 'total K is unchanged after an elastic collision'.
- 🚫 In a perfectly inelastic collision, the total K is not zero but minimum, as both masses cannot come to rest without violating pcom.
- 🔢 For a 2 kg and 3 kg mass colliding at 4 m/s and 5 m/s, the perfectly inelastic collision results in both masses moving leftward at 1.4 m/s.
- 🔄 In a perfectly elastic collision, the relative speed of separation equals the relative speed of approach, leading to the total K remaining unchanged.
- 📈 The momentum-time graph for perfectly inelastic and elastic collisions shows a horizontal line representing the conservation of total momentum.
- 🏎️ The velocity-time graph for inelastic collisions indicates different rates of velocity change due to mass differences and equal but opposite forces.
- 🔥 In inelastic collisions, lost kinetic energy is converted into heat, sound, or deformation energy, whereas in elastic collisions, all energy is returned.
Q & A
What is the principle of conservation of momentum (pcom)?
-The principle of conservation of momentum (pcom) states that the total momentum of a system is conserved during a collision. This means that the sum of the momenta of the objects involved in the collision is the same before and after the collision.
What is the principle of conservation of energy (pcoe)?
-The principle of conservation of energy (pcoe) asserts that the total energy in a system remains constant during a collision. This implies that the sum of all forms of energy, including kinetic and potential energy, is the same before and after the collision.
What is the difference between an elastic and an inelastic collision?
-The difference between an elastic and an inelastic collision lies in the total kinetic energy. In an elastic collision, the total kinetic energy before the collision is equal to the total kinetic energy after the collision. In contrast, in an inelastic collision, some of the kinetic energy is converted into other forms of energy, such as heat or sound, resulting in a decrease in the total kinetic energy.
Why is the statement 'total K is conserved during an elastic collision' incorrect?
-The statement 'total K is conserved during an elastic collision' is incorrect because we do not have a principle of conservation of kinetic energy (KE). While the total kinetic energy remains the same before and after an elastic collision, it is not 'conserved' throughout the collision process as the kinetic energy is temporarily stored in other forms of energy during the interaction.
What is the outcome of a perfectly inelastic collision between a 2 kg and a 3 kg object?
-In a perfectly inelastic collision between a 2 kg and a 3 kg object, the two objects move together at the same velocity after the collision. The resulting velocity can be calculated using the pcom equation, and in this case, both masses will be traveling leftward at the same speed of 1.4 m per second.
How can you determine the outcome of a perfectly elastic collision?
-To determine the outcome of a perfectly elastic collision, you need to set up both the pcom equation and an equation derived from the principle that the relative speed of approach equals the relative speed of separation. Solving these two equations simultaneously will yield the velocities of the two objects after the collision.
What happens to the kinetic energy that is 'lost' in an inelastic collision?
-In an inelastic collision, the 'lost' kinetic energy is converted into other forms of energy, such as heat, sound, or stored permanently as deformation energy in the objects involved in the collision.
How does the impulse experienced by the objects in a collision compare between perfectly inelastic and elastic collisions?
-The impulse, which is the change in momentum, is greater in elastic collisions compared to inelastic collisions. This is because the force acting on the objects is equal but opposite, and due to the smaller mass, the object with less mass experiences a larger acceleration and thus a greater change in momentum.
What does the velocity-time graph of a perfectly inelastic collision look like?
-In the velocity-time graph of a perfectly inelastic collision, the velocity of the object with greater mass decreases to zero, while the object with lesser mass increases in velocity. The change in velocity is proportional to the mass ratio, and the total momentum is represented by a horizontal line, indicating conservation of momentum.
How does the total kinetic energy (KE) change during an elastic collision?
-During an elastic collision, the total kinetic energy is not constant throughout the collision. The KE temporarily decreases during the interaction as energy is transferred and stored in other forms, but it is fully returned by the end of the collision, maintaining the total KE unchanged before and after the collision.
What is the relationship between the relative speed of separation and the total kinetic energy in an inelastic collision?
-The relative speed of separation in an inelastic collision is directly related to the total kinetic energy. In a perfectly inelastic collision, where the relative speed of separation is zero, there is a maximum loss of kinetic energy. In other inelastic collisions, the relative speed of separation is less than that in a perfectly inelastic collision, resulting in less kinetic energy being lost.
Outlines
📚 Principles of Collision - Elastic vs Inelastic
This paragraph introduces the fundamental concepts of collisions, focusing on the difference between elastic and inelastic collisions. It clarifies misconceptions regarding the conservation of momentum (PCM) and the conservation of kinetic energy (KE). The speaker corrects a common mistake about the total KE being conserved in an elastic collision, explaining that it's not about conservation but rather the total KE remaining unchanged after the collision. The paragraph also delves into the specifics of perfectly inelastic and perfectly elastic collisions, using a numerical example involving 2 kg and 3 kg masses to illustrate the outcomes. The key takeaway is understanding the principles of momentum and kinetic energy in collisions and how they apply to different scenarios.
📈 Visualizing Collision Outcomes - Momentum and Velocity Graphs
This paragraph expands on the concept of collisions by discussing how to visually represent the outcomes using momentum and velocity graphs. The speaker explains how the conservation of momentum is reflected in the stiffness of the momentum graph lines, indicating that the change in momentum for one object must be exactly offset by the other. The velocity graphs are used to illustrate the differences in how mass and force affect the rate of velocity change during collisions. The paragraph also addresses the concept of impulse and how it varies between perfectly inelastic, perfectly elastic, and non-ideal inelastic collisions. The speaker emphasizes the loss of kinetic energy in inelastic collisions, which is converted into other forms of energy like heat or sound. The summary encourages the audience to practice solving collision problems and sketching the corresponding graphs to deepen their understanding.
Mindmap
Keywords
💡Conservation of Momentum
💡Conservation of Energy
💡Elastic Collision
💡Inelastic Collision
💡Total Kinetic Energy (K)
💡Impulse
💡Relative Speed
💡Momentum-Time Graph
💡Velocity-Time Graph
💡Perfectly Inelastic Collision
💡Deformation Energy
Highlights
The principle of conservation of momentum (pcom) is fundamental in understanding collisions, stating that total momentum is conserved during a collision.
The principle of conservation of energy (pcoe) is also crucial, asserting that total energy is conserved during a collision.
The difference between elastic and inelastic collisions lies in the conservation of total kinetic energy (K), which is a key factor in analyzing the outcomes of these collisions.
An elastic collision is characterized by the conservation of total kinetic energy, meaning the total K remains unchanged after the collision.
In a perfectly inelastic collision, the total kinetic energy is at its minimum, but not zero, as this would violate the conservation of momentum.
The use of numerical examples, such as a 2 kg and a 3 kg mass colliding at specific velocities, helps to illustrate the outcomes of both elastic and inelastic collisions.
In a perfectly inelastic collision, the two masses move with the same velocity after the collision, resulting in the maximum loss of kinetic energy.
For elastic collisions, an additional equation derived from the relative speed of approach equaling the relative speed of separation is required to determine the outcome.
The perfectly elastic and perfectly inelastic collisions represent the two extreme outcomes for a collision, with actual collisions falling somewhere in between.
The possibility of one mass coming to rest after a collision (e.g., the 3 kg mass) depends on the specifics of the collision and can occur in inelastic but not perfectly elastic collisions.
Graphical representations, such as momentum-time and velocity-time graphs, effectively demonstrate the conservation of momentum and changes in kinetic energy during collisions.
The stiffness of the lines in momentum-time graphs indicates the conservation of total momentum, which must be equal and opposite for the two colliding masses.
Velocity-time graphs show that the change in velocity is not the same for both masses due to differences in mass and the resulting acceleration.
The total kinetic energy (K) graph for inelastic collisions shows a loss of K, which is converted into other forms of energy like heat, sound, or deformation energy.
In elastic collisions, the total K is not constant throughout, temporarily stored in the deformation of the objects before being returned in full.
The gap between velocity lines in graphs represents the relative speed of approach and separation, with the gap being zero in perfectly inelastic collisions where the relative speed of separation is minimal.
The impulse experienced by the masses in a collision, reflected by the change in momentum, varies depending on the type of collision and the masses involved.
The perfectly elastic collision has the largest impulse, indicating it is the most violent type of collision among the considered scenarios.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: