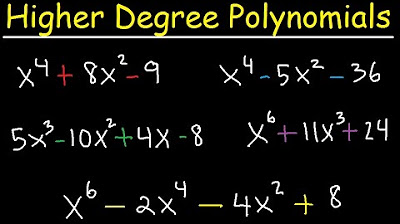Factoring Polynomials - By GCF, AC Method, Grouping, Substitution, Sum & Difference of Cubes

The Organic Chemistry Tutor

15 Feb 201645:43

EducationalLearning

32 Likes 10 Comments

TLDRThis video tutorial comprehensively covers various polynomial factoring techniques, including extracting the greatest common factor (GCF), factoring by grouping, using difference of squares, sum and difference of cubes, and completing the square. It also addresses trinomial factoring with and without a leading coefficient of 1, along with synthetic division and advanced factoring of expressions involving multiple variables and imaginary numbers. The script is an educational guide for students tackling algebra, trigonometry, precalculus, and even calculus.

Takeaways

📚 Always start by looking for the Greatest Common Factor (GCF) when factoring polynomials, as it simplifies the expression by dividing out the common factor from all terms.
🔍 In binomials like 7x + 21, factoring out the GCF (7 in this case) simplifies the expression to x and 3, showcasing the basic principle of factoring by GCF.
📉 For expressions involving perfect squares or cubes, use the difference of squares (A^2 - B^2 = (A + B)(A - B)) or sum/difference of cubes formulas to factor them efficiently.
📈 Factoring trinomials can be done by looking for two numbers that multiply to the product of the squared term and add up to the linear coefficient.
🌐 When the leading coefficient is not 1, methods like factoring by grouping or substitution can be used to break down and simplify the polynomial.
🔑 The AC method is a systematic approach to factor trinomials by creating a new variable (a) to represent the middle term and solving for factors that satisfy the equation.
📝 Factoring by grouping is possible when the ratios of the outer and inner coefficients are the same, allowing you to factor out common terms from groups of terms.
🔍📐 Completing the square is a technique used for quadratic expressions, where you add and subtract the square of half the linear coefficient to transform the expression into a perfect square trinomial.
📊 Synthetic division is a useful tool for factoring higher-degree polynomials by testing potential factors and dividing the polynomial by these factors.
🤔 Factoring expressions with multiple variables can be approached by focusing on parts of the expression that resemble known factoring patterns, such as perfect squares or differences of squares.
🧩 Advanced factoring techniques involve recognizing patterns and structures within polynomials that can be broken down using a combination of the methods discussed, such as grouping, completing the square, and using identities.

Q & A

What is the first step in factoring a polynomial?
-The first step in factoring a polynomial is to look for the Greatest Common Factor (GCF) between the terms and factor it out.
How do you factor a binomial like 7x + 21?
-To factor 7x + 21, you identify the GCF, which is 7, and then factor it out, resulting in 7(x + 3).
What is the difference between factoring by taking out the GCF and using the difference of perfect squares?
-Factoring by taking out the GCF involves finding the common factor in all terms, while the difference of perfect squares involves recognizing a pattern of A^2 - B^2, which factors into (A + B)(A - B).
Can you factor a sum of perfect squares?
-A sum of perfect squares cannot be factored using real numbers. However, it can be factored using imaginary numbers, such as X^2 + 4, which factors into (X + 2i)(X - 2i).
What is the method for factoring trinomials with a leading coefficient of 1?
-For trinomials with a leading coefficient of 1, you look for two numbers that multiply to the constant term and add up to the linear coefficient, then factor the trinomial into the product of two binomials.
How do you factor a trinomial when the leading coefficient is not 1?
-When the leading coefficient is not 1, you can try factoring by grouping or using the APE method (Arranging, Picking, and Expanding), or by trial and error if the coefficients are small.
What is the process of factoring by substitution for a polynomial with three terms?
-For factoring by substitution, you identify a term with an exponent that is a multiple of another, let that term represent a new variable (a), and then factor the resulting trinomial in terms of 'a'. Afterward, replace 'a' with the original expression.
How do you factor a polynomial using the sum and difference of perfect cubes?
-For the sum of perfect cubes, A^3 + B^3, the factored form is (A + B)(A^2 - AB + B^2). For the difference, A^3 - B^3, it is (A - B)(A^2 + AB + B^2).
What is the purpose of synthetic division in factoring polynomials?
-Synthetic division is used to test potential factors of a polynomial. It helps to determine if a given number is a root of the polynomial, which can then be used to factor the polynomial.
How do you factor a polynomial by completing the square?
-To factor by completing the square, you first ensure the polynomial is a quadratic expression, then adjust the constant term to create a perfect square trinomial. The expression can then be rewritten as the square of a binomial minus the adjusted constant, and factored accordingly.
Can you provide an example of factoring by grouping?
-Yes, for a polynomial like x^3 + 2x^2 + 3x + 6, if the ratio of the first two coefficients (1:2) matches the ratio of the last two (3:6), you can factor by grouping. Factor out the GCF from the first two terms and the last two terms, resulting in x(x + 2) + 3(x + 2), which simplifies to (x + 3)(x + 2).
What are the conditions for using the difference of cubes factoring method?
-The difference of cubes factoring method is applicable when the polynomial has the form A^3 - B^3. Both A and B must be expressible as perfect cubes, and the polynomial should not have any other terms.
How do you factor a polynomial with variables and multiple terms?
-For polynomials with variables and multiple terms, you can look for common factors, group terms with similar variables, or use substitution to reduce the expression to a simpler form that can be factored.
Can you give an example of a polynomial that cannot be factored using real numbers but can using imaginary numbers?
-An example of a polynomial that cannot be factored using real numbers but can using imaginary numbers is x^2 + 4. Using imaginary numbers, it can be factored as (x + 2i)(x - 2i).

Outlines

00:00

📚 Introduction to Factoring Polynomials

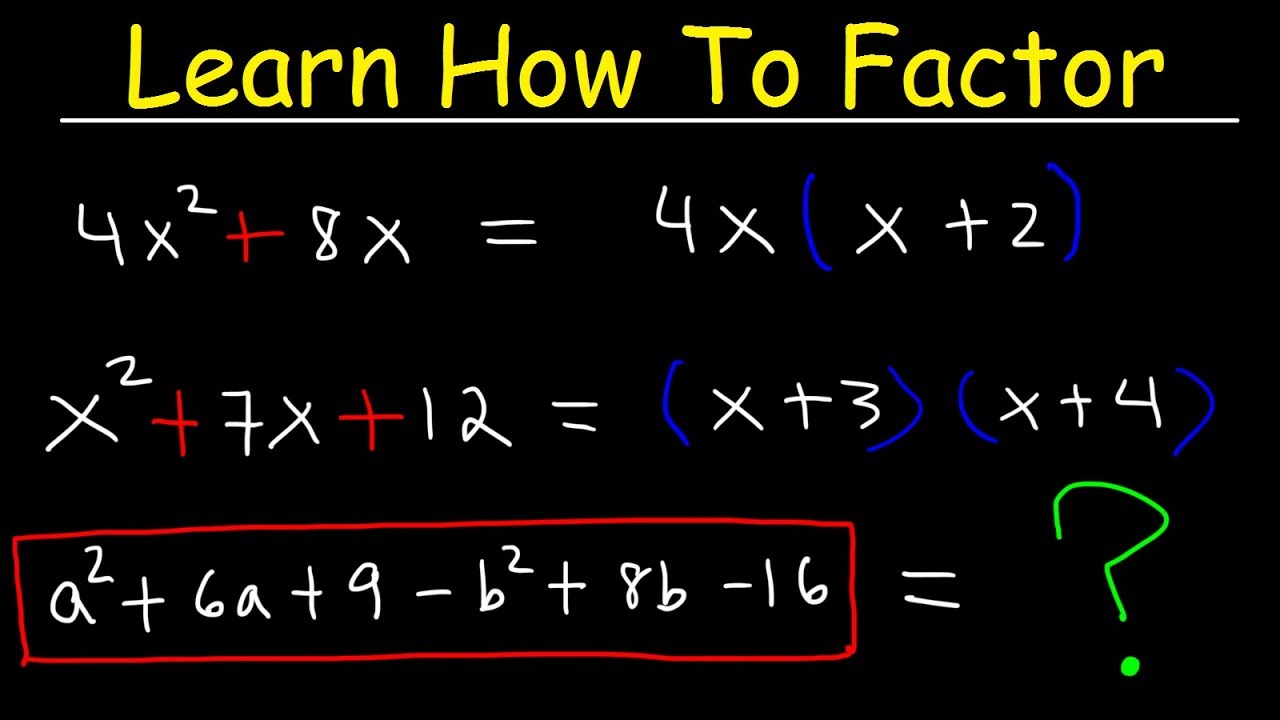
The video begins with an introduction to various polynomial factoring techniques, such as extracting the Greatest Common Factor (GCF), factoring by using difference of squares, sum and difference of cubes, and factoring trinomials through substitution and grouping. The presenter demonstrates how to factor simple binomials and progressively complex expressions, emphasizing the importance of identifying the GCF in polynomials like 7x + 21 and 8x^2 + 12x, and how to simplify expressions by removing common factors.

05:00

🔍 Factoring Techniques and GCF

This paragraph delves deeper into factoring by GCF, showcasing examples like 36x^3 + y^2 - 60x^4y^3, where the GCF is used to simplify the expression. The video also covers the difference of squares formula, demonstrating how to factor expressions like x^2 - 25 and y^2 - 64 + 8x^2. The presenter encourages viewers to practice by attempting similar problems, such as 81x^2 - 36y^2 and 200x^4 - 288y^6, highlighting the process of taking square roots and factoring out common terms.

10:03

📈 Factoring Trinomials and Special Cases

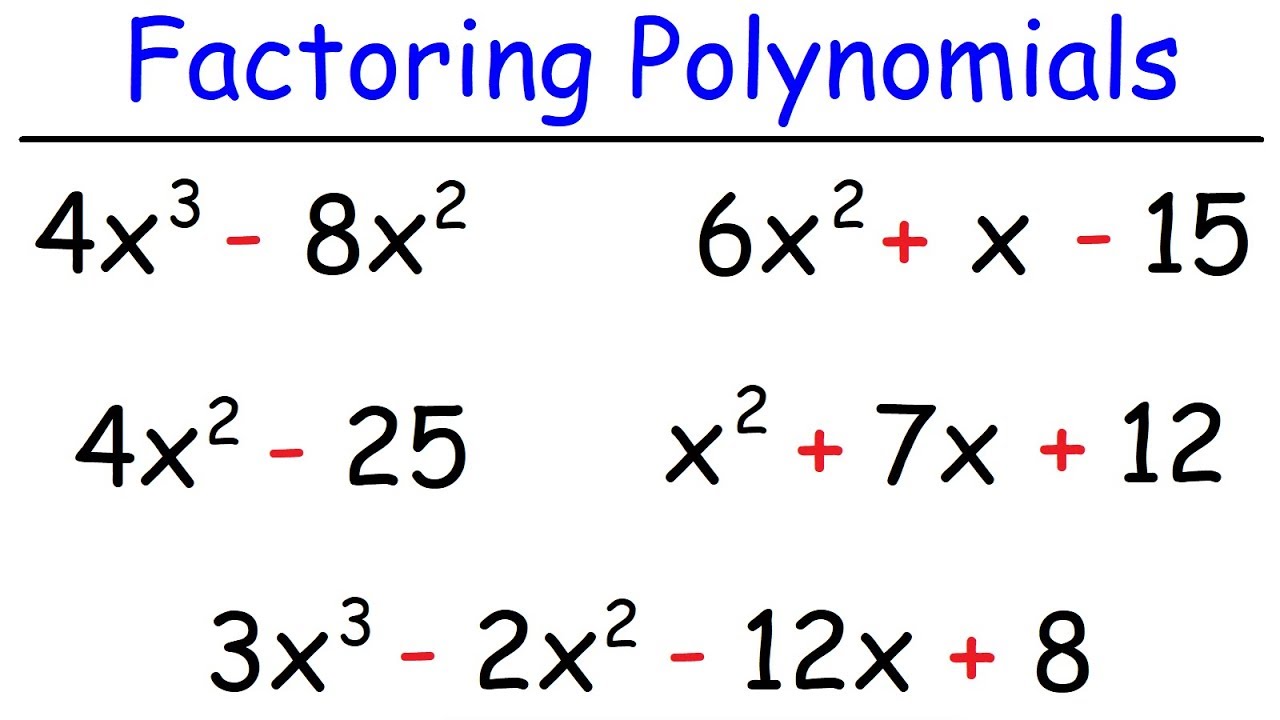
The focus shifts to factoring trinomials, especially when the leading coefficient is 1, as seen in x^2 + 11x + 30. The method involves finding two numbers that multiply to the constant term and add up to the linear coefficient. The video also addresses trinomials with a leading coefficient other than 1, such as 2x^2 - 5x - 3, and introduces the Athey method and trial and error for factoring. Examples are provided to illustrate the process, including solving for the zeros of a polynomial by setting each factor to zero.

15:04

🌐 Advanced Factoring Methods

The script introduces more advanced factoring methods, including factoring by grouping, which is applicable when the first two coefficients have the same ratio as the last two coefficients. Examples like x^3 - 2x^2 - 5x + 6 are used to demonstrate synthetic division for finding factors, and the process is shown step by step, including how to handle a trinomial resulting from the division.

20:06

📝 Factoring with Substitution and Perfect Cubes

This section covers factoring polynomials with three terms using substitution, where the middle term is replaced with a variable (e.g., a = x^2 for x^4 + 7x^2 + 12). The method is applied to expressions like x^6 - 2x^6 + 6x^3 + 56, and the presenter shows how to factor perfect cubes, both sum and difference, using specific formulas and examples such as x^3 + 8 and y^3 - 125.

25:06

🔢 Factoring Polynomials with Higher Degrees

The video moves on to factor higher degree polynomials using synthetic division, starting with identifying possible factors and applying the method to expressions like x^4 + 2x^3 + x^2 + 8x - 12. The process involves dividing the polynomial by potential factors and checking for a remainder of zero to confirm a valid factor. The presenter also explains how to handle missing terms in synthetic division and the importance of including all terms.

30:07

🔍 Factoring by Grouping and Completing the Square

The script discusses factoring cubic polynomials by grouping and when it's applicable, using the ratio of coefficients to determine if grouping is possible. Examples such as 4x^3 - 8x^2 + 3x - 6 illustrate the process. Additionally, the video introduces completing the square as a method for factoring trinomials, demonstrating how to manipulate the expression to form a perfect square trinomial plus or minus a constant.

35:08

📉 Completing the Square with Variables and Constants

This part of the video script provides a detailed explanation of completing the square, including how to adjust for the addition of a square term by subtracting the same value to maintain the expression's original value. Examples like x^2 - 4x + 12 and x^2 - 3x + 1 are used to show the step-by-step process, with a focus on manipulating the expression to create a perfect square and then adjusting the constant term accordingly.

40:10

📚 Factoring Advanced Polynomial Expressions

The final section of the script addresses advanced factoring of polynomial expressions with multiple variables, such as x^2 - 2xy + y^2 - 9. The presenter uses specific factoring formulas for sums and differences of squares and products to simplify complex expressions. The video concludes with a summary of the various factoring techniques covered, emphasizing their utility across algebra, trigonometry, precalculus, and calculus.

Mindmap

Keywords

💡Greatest Common Factor (GCF)

The Greatest Common Factor (GCF) is the largest number that divides two or more numbers without leaving a remainder. In the context of the video, the GCF is used as the first step in factoring expressions by taking out the common factors from all terms, simplifying the expression. For example, in the binomial 7x + 21, the GCF is 7, which is factored out to simplify the expression to 7(x + 3).

💡Difference of Perfect Squares

The Difference of Perfect Squares is a factoring technique used when an expression is in the form of A^2 - B^2. It can be factored into (A - B)(A + B). The video demonstrates this concept with the expression x^2 - 25, which factors into (x - 5)(x + 5), as 25 is a perfect square and the expression fits the pattern of a difference of squares.

💡Sum of Perfect Cubes

The Sum of Perfect Cubes is a factoring method applicable to expressions of the form A^3 + B^3. It can be factored into (A + B)(A^2 - AB + B^2). The video introduces this technique with the example of x^3 + 8, which factors into (x + 2)(x^2 - 2x + 4), showcasing the sum of cubes factorization.

💡Factoring Trinomials

Factoring Trinomials refers to the process of expressing a trinomial (an expression with three terms) as the product of two binomials. The video covers various methods for factoring trinomials, such as looking for two numbers that multiply to the product of the squared term and the constant term while adding up to the linear coefficient, as seen in the trinomial x^2 + 11x + 30 which factors into (x + 5)(x + 6).

💡Synthetic Division

Synthetic Division is a simplified form of polynomial division used to determine if a binomial is a factor of a polynomial. The video demonstrates synthetic division to factor a cubic polynomial, such as x^3 - 2x^2 - 5x + 6, by testing possible factors and dividing the polynomial by these potential factors to see if the remainder is zero.

💡Completing the Square

Completing the Square is a method used to rewrite a quadratic expression in the form of a perfect square trinomial plus or minus a constant. The video explains this technique by taking a quadratic term, adding and subtracting the square of half the coefficient of the linear term, and then factoring it into the square of a binomial, as shown with the expression x^2 + 6x + 7 which becomes (x + 3)^2 - 2.

💡Factoring by Grouping

Factoring by Grouping is a technique used when a polynomial has more than three terms. The terms are grouped in pairs, and factors are taken out from each group, revealing a common binomial factor which is then factored out of the expression. The video shows this method with the polynomial 4x^3 - 8x^2 + 3x - 6, where the first two and last two terms are grouped and factored to reveal a common (x - 2) factor.

💡Substitution

Substitution is a method where a complex expression within a polynomial is replaced with a single variable, simplifying the factoring process. In the video, this technique is used with expressions like x^4 + 7x^2 + 12, where x^2 is substituted with a new variable 'a', transforming the expression into a + 7a + 12, which is easier to factor.

💡Perfect Squares and Cubes

Perfect Squares and Cubes refer to numbers that are the product of another number multiplied by itself for squares (A^2) or three times for cubes (A^3). The video discusses factoring expressions that are differences or sums of these perfect powers, such as x^2 - 64, which is a difference of squares and factors into (x - 8)(x + 8), and x^3 + 8, which is a sum of cubes and factors into (x + 2)(x^2 - 2x + 4).

💡Imaginary Numbers

Imaginary Numbers are a set of numbers in the complex number system that, when squared, result in a negative number, represented by the symbol 'i' where i^2 = -1. The video mentions the use of imaginary numbers in the context of factoring expressions that are sums of squares, such as x^2 + 4, which factors into (x + 2i)(x - 2i) using imaginary units.

Highlights

Introduction to factoring polynomials by extracting the Greatest Common Factor (GCF) and using various methods such as difference of perfect squares, sum of perfect cubes, and factoring by grouping.

Demonstration of factoring a binomial by removing the GCF, illustrated with the example 7x + 21.

Explanation of factoring by removing GCF from polynomials with multiple terms, like 8x^2 + 12x and 36x^3 + y^2 - 60x^4y^3.

Guide on factoring expressions using the difference of perfect squares formula, shown with x^2 - 25.

Method for factoring expressions with a GCF other than 1, using the example 2x^2 - 5x - 3.

Technique for factoring trinomials with a leading coefficient of 1, by finding two numbers that multiply to the constant term and add up to the linear coefficient.

Process of factoring trinomials when the leading coefficient is not 1, using the Athey method and trial and error.

Illustration of factoring by grouping, applicable when the first two coefficients have the same ratio as the last two coefficients.

Introduction to factoring polynomials with three terms using substitution, as shown with x^4 + 7x^2 + 12.

Explanation of sum and difference of perfect cubes factoring, demonstrated with x^3 + 8.

Factoring cubic polynomials using synthetic division, starting with possible factors of the constant and leading coefficients.

Guide on factoring trinomials by completing the square, adjusting for changes in the expression's value.

Application of completing the square to factor expressions with fractions, as shown with x^2 - 4x + 12.

Discussion on the inability to factor sum of perfect squares using real numbers, with an alternative using imaginary numbers.

Advanced factoring techniques for polynomials with multiple variables, such as x^2 - 2xy + y^2 - 9.

Conclusion summarizing the wide range of factoring techniques covered, useful for algebra, trigonometry, precalculus, and calculus courses.

Transcripts

Browse More Related Video

Factoring Trinomials & Polynomials, Basic Introduction - Algebra

How To Factor Polynomials The Easy Way!

Factoring Review

How To Find The Real & Imaginary Solutions of Polynomial Equations

Factoring Higher Degree Polynomial Functions & Equations - Algebra 2

Factor Polynomials - Understand In 10 min

Related Tags

Algebra Factoring Polynomials Greatest Common Factor Perfect Squares Trinomials Synthetic Division Completing the Square Educational Content Mathematics Tutorial