Factoring Higher Degree Polynomial Functions & Equations - Algebra 2
TLDRThis video tutorial delves into the art of factoring higher degree polynomials, offering step-by-step methods for various examples. It begins with the substitution technique for a quartic polynomial, then explores the difference of squares and sum of cubes for factoring. The script also covers factoring by grouping for a quartic and a cubic polynomial, and concludes with a demonstration of factoring a sextic polynomial using grouping and difference of squares, providing a comprehensive guide for students to master polynomial factorization.
Takeaways
- 📚 The video focuses on factoring higher degree polynomials by using substitution and other techniques.
- 🔍 The first example demonstrates factoring \(x^4 + 8x^2 - 9\) by substituting \(x^2\) with \(a\), resulting in \((a+9)(a-1)\), and then replacing \(a\) back with \(x^2\).
- 🔑 For the first example, the difference of squares technique is used to factor \(x^2 - 1\) into \((x+1)(x-1)\).
- 🤔 The video mentions that if imaginary numbers are known, \(x^2 + 9\) can be represented as \(x + 3i\) and \(x - 3i\).
- 📝 In the second example, \(x^4 - 5x^2 - 36\) is factored by substituting \(x^2\) with \(a\), and finding numbers that multiply to -36 and add up to -5, resulting in \((x^2 + 4)(x + 3)(x - 3)\).
- 🔢 The third example, \(x^6 + 11x^3 + 24\), uses substitution with \(a = x^3\) and factors to \((x^3 + 3)(x^3 + 8)\), then further factors \(x^3 + 8\) using the sum of cubes formula.
- 🧩 The fourth example, \(5x^3 - 10x^2 + 4x - 8\), is factored by grouping, taking advantage of the common ratio between the coefficients of the first two and last two terms.
- 🔄 The last example, \(x^6 - 2x^4 - 4x^2 + 8\), also uses factoring by grouping, with the common ratio of -2 between the coefficients, and then applies the difference of squares technique.
- 📉 The process of factoring involves identifying patterns, such as the sum or difference of squares, and using algebraic identities to simplify expressions.
- 📈 The video script provides a step-by-step guide for factoring various types of polynomials, emphasizing the importance of recognizing the correct technique for each scenario.
Q & A
What is the main topic of the video?
-The main topic of the video is factoring polynomials of higher degree.
What substitution method is used in the first example to simplify the polynomial x^4 + 8x^2 - 9?
-The substitution method used is to replace x^2 with a variable 'a', which allows the polynomial to be rewritten in a quadratic form.
What technique is used to factor the quadratic form a^2 + 8a - 9 after substitution?
-The technique used is to find two numbers that multiply to -9 and add up to 8, which are 9 and -1, and then factor the quadratic as (a + 9)(a - 1).
How is the expression x^4 + 8x^2 - 9 factored completely?
-After substitution and factoring, the expression is rewritten as x^2 + 9 and x^2 - 1, with the latter being factored further using the difference of squares to (x + 1)(x - 1).
What alternative representation can be used for x^2 + 9 if imaginary numbers are considered?
-If imaginary numbers are considered, x^2 + 9 can be represented as (x + 3i)(x - 3i).
In the second example, what is the method used to factor the polynomial x^4 - 5x^2 - 36?
-The method used is to set a = x^2 and then find two numbers that multiply to -36 and add up to -5, which are 4 and -9, and factor the polynomial accordingly.
How is the polynomial x^6 + 11x^3 + 24 factored using the substitution method?
-By setting a = x^3 and rewriting the polynomial as a^2 + 11a + 24, then factoring it as (a + 3)(a + 8) and substituting back to get (x^3 + 3)(x^3 + 8).
What technique is used to factor x^3 + 8 in the polynomial x^6 + 11x^3 + 24?
-The sum of cubes technique is used, which follows the formula a^3 + b^3 = (a + b)(a^2 - ab + b^2).
In the example with the polynomial 5x^3 - 10x^2 + 4x - 8, what method is suggested for factoring?
-The method suggested is factoring by grouping, which is applicable when the first two coefficients have the same ratio as the last two coefficients.
How is the polynomial 5x^3 - 10x^2 + 4x - 8 factored using factoring by grouping?
-By taking out the greatest common factor from the first two terms (5x and -10x^2) and the last two terms (4x and -8), and then factoring out (x - 2) to get (x - 2)(5x^2 + 4).
In the final example, what technique is used to factor the polynomial x^6 - 2x^4 - 4x^2 + 8?
-The technique used is again factoring by grouping, taking out the greatest common factor from the first two and last two terms, and then factoring out (x^2 - 2) to get (x^2 + 2)(x^4 - 2^2).
How is the expression x^4 - 4 factored in the final example?
-The expression x^4 - 4 is factored using the difference of squares technique to (x^2 + 2)(x^2 - 2).
Outlines
📚 Factoring Higher Degree Polynomials
This paragraph introduces the topic of factoring polynomials of higher degree, specifically focusing on the example of x to the fourth power plus eight x squared minus 9. The method of substitution is used, where x squared is replaced with a variable 'a'. The polynomial is then rewritten in a quadratic form, and the factors that multiply to -9 and add up to 8 are identified as 9 and -1. The expression is factored into (a + 9)(a - 1), which is then translated back to x squared terms, resulting in (x^2 + 9) and (x^2 - 1). The difference of squares technique is applied to factor (x^2 - 1) into (x + 1)(x - 1). The paragraph also mentions an alternative approach involving imaginary numbers for x^2 + 9 and presents another example, x to the fourth power minus five x squared minus thirty-six, using a similar substitution method with 'a' equal to x squared.
🔍 Advanced Factoring Techniques
The second paragraph delves into more advanced factoring techniques, starting with the sum of cubes formula to factor x cubed plus eight. It explains the process of identifying 'a' and 'b' in the formula and applies it to the given example, resulting in the factored form of (x + 2)(x^2 - 2x + 4). The paragraph then moves on to factoring a polynomial with four terms by grouping, where the coefficients of the first two terms and the last two terms have the same ratio. The greatest common factor is extracted, and the expression is factored by grouping, resulting in (x - 2)(5x^2 + 4). Another example, x to the sixth power minus two x to the fourth power minus four x squared plus eight, is also factored by grouping, using the common ratio and resulting in (x^2 + 2)(x^2 - 2)^2.
🔚 Conclusion of Factoring Examples
The final paragraph concludes the video script with a brief mention of the completion of the factoring examples. It does not provide additional information or a new example, serving as a closure to the previous discussions on polynomial factoring techniques.
Mindmap
Keywords
💡Factoring
💡Polynomials
💡Substitution
💡Quadratic Form
💡Difference of Squares
💡Imaginary Numbers
💡Sum of Cubes
💡Factor by Grouping
💡Greatest Common Factor (GCF)
💡FOIL Method
Highlights
Introduction to factoring polynomials of higher degree with an example.
Using substitution to factor by replacing x squared with a variable.
Rewriting the polynomial in quadratic form for easier factoring.
Identifying two numbers that multiply to -9 and add to 8 for factoring.
Factoring the quadratic expression into (a + 9)(a - 1).
Substituting a back with x squared to get the factored form.
Applying the difference of squares technique to factor x squared - 1.
Handling the case of x squared + 9 with imaginary numbers if applicable.
Setting up a new example with x to the fourth minus 5x squared - 36.
Finding two numbers that multiply to -36 and add to -5 for factoring.
Factoring the polynomial into (x squared + 4)(x squared - 9).
Using the difference of squares to factor x squared - 9 into (x + 3)(x - 3).
Introducing the sum of cubes technique for factoring x cubed + 8.
Factoring x cubed + 8 into (x + 2)(x squared - 2x + 4).
Exploring factoring by grouping for a polynomial with four terms.
Checking for the same ratio in coefficients for factoring by grouping.
Factoring the polynomial 5x cubed - 10x squared + 4x - 8 by grouping.
Using the FOIL method to confirm the factored expression.
Factoring x to the sixth - 2x to the fourth - 4x squared + 8 by grouping.
Applying the difference of squares technique to x squared - 4.
Final factored form of the expression x squared + 2 times (x squared - 2 squared).
Transcripts
Browse More Related Video
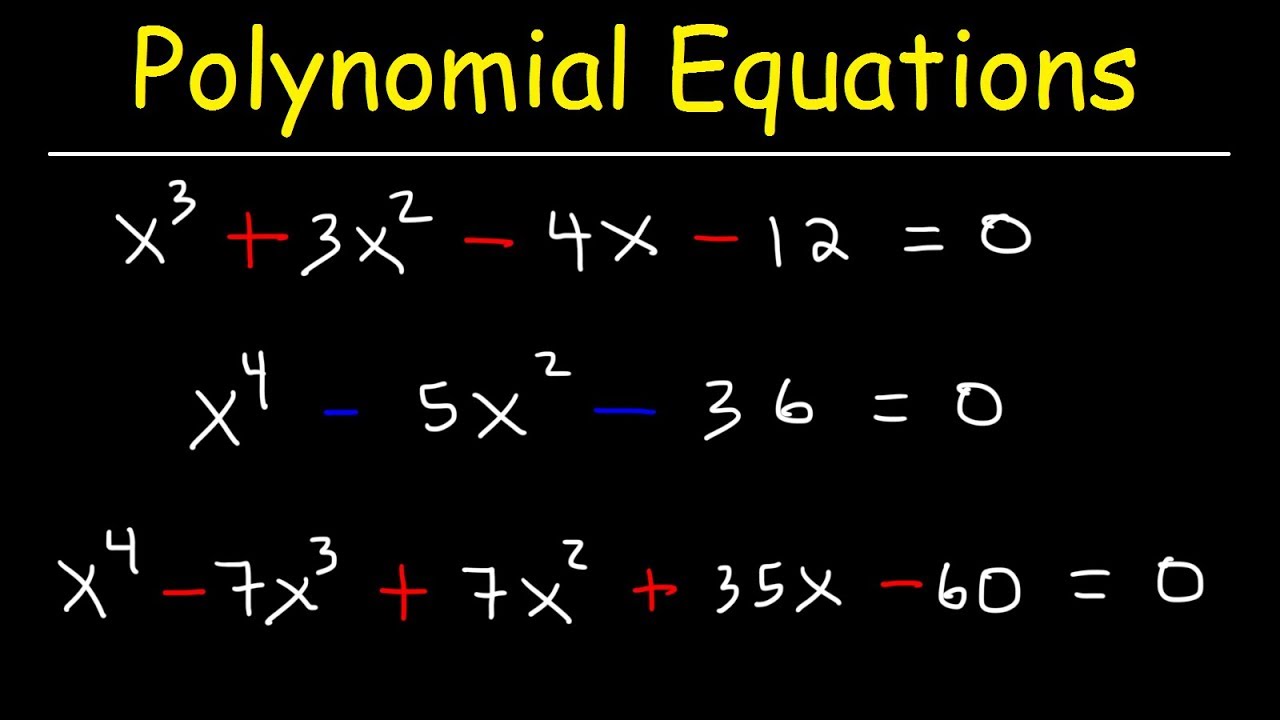
Solving Polynomial Equations By Factoring and Using Synthetic Division

Factoring Polynomials - By GCF, AC Method, Grouping, Substitution, Sum & Difference of Cubes
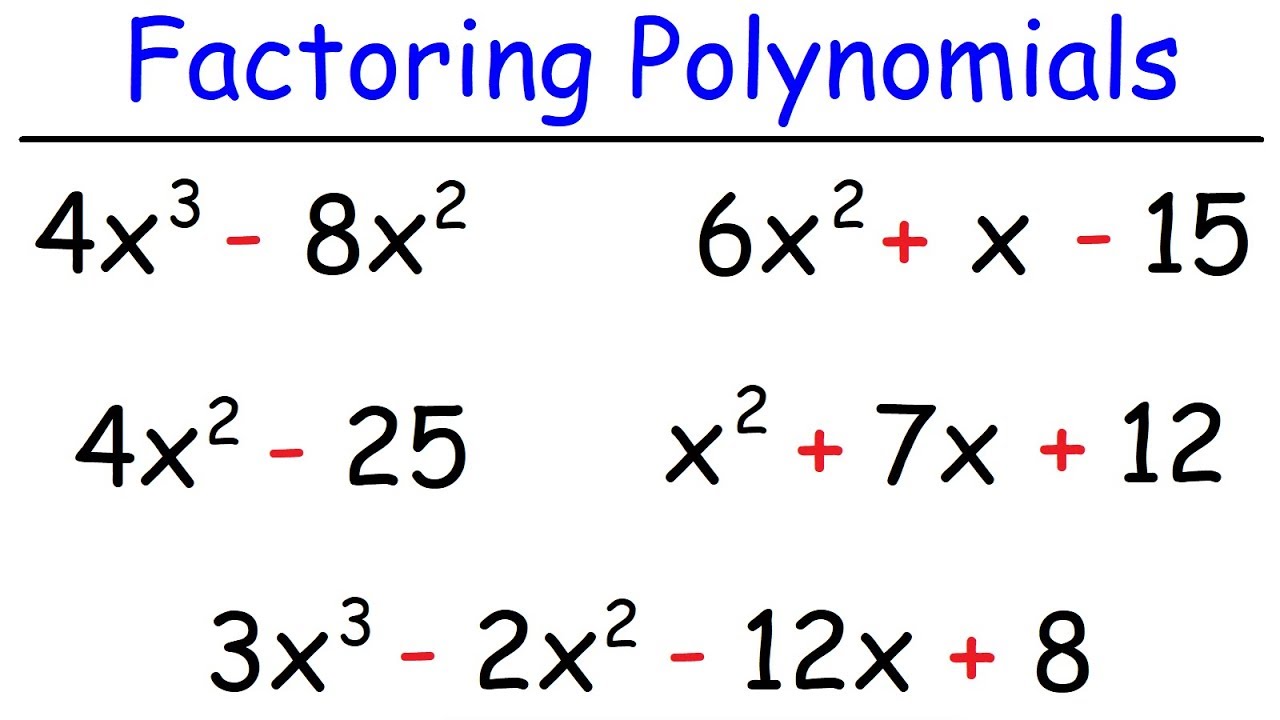
How To Factor Polynomials The Easy Way!
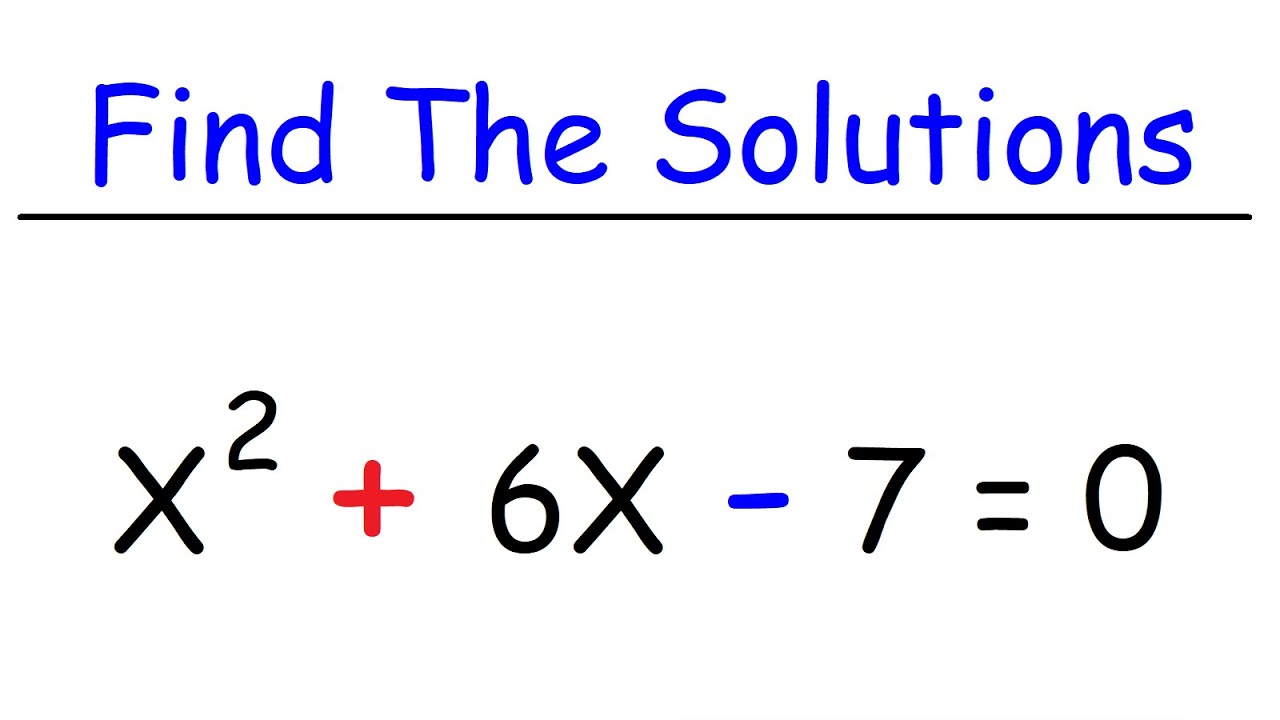
How To Find The Real & Imaginary Solutions of Polynomial Equations

Factoring Review

AP Calculus AB - 1.6a Review of Expanding and Factoring Techniques
5.0 / 5 (0 votes)
Thanks for rating: