Newtonian Gravity: Crash Course Physics #8
TLDRIsaac Newton revolutionized physics with his laws of motion and his law of universal gravitation, which describes the gravitational force between any two objects. His inspiration came in part from watching an apple fall from a tree. Newton realized the same force causing the apple to drop also kept the moon orbiting Earth. He devised an equation showing that gravitational force depends on the masses of objects and distance between them. Newton used his theory to prove Johannes Kepler's laws describing planetary orbits. Today, over 300 years later, NASA still relies on Newton's equations, like when testing spacesuits for Mars in reduced gravity.
Takeaways
- 👨🔬 Isaac Newton's contributions to physics, especially his understanding of gravity, significantly advanced the field.
- 🍏 The anecdote of Newton and the falling apple symbolizes his realization of gravity affecting all objects, no matter their size.
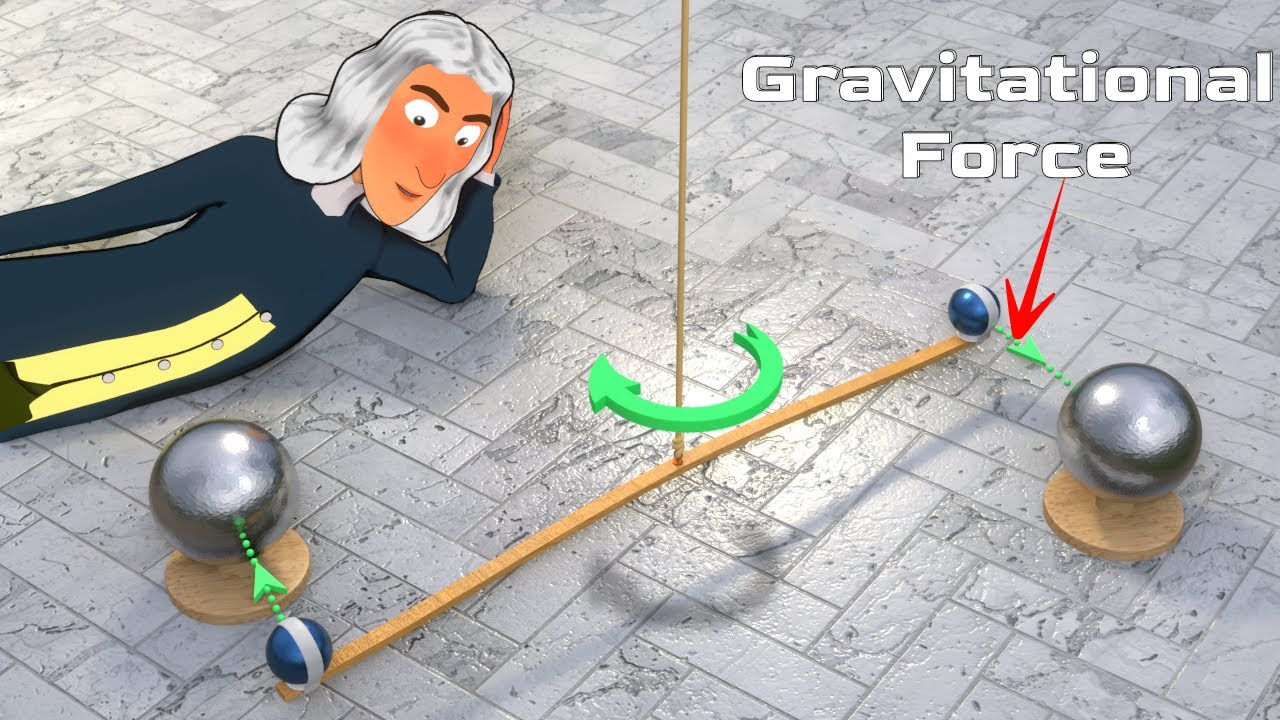
- 📊 Newton's law of universal gravitation (F = GMm/r^2) describes the force of gravity between two objects, based on their masses and the distance between them.
- ✅ Newton introduced a gravitational constant (G) in his equation, acknowledging its existence long before its value was measured by Henry Cavendish.
- 🌍 The law of universal gravitation explained the elliptical orbits of planets and moons, aligning with and proving Johannes Kepler's laws of planetary motion.
- 🚀 Newton's work on gravity, combined with his three laws of motion and calculus, enabled precise predictions of celestial mechanics and the orbits of planets.
- ⭐ Newton's insights into gravity and motion underpin modern space exploration efforts, including calculating the conditions for human missions to Mars.
- 👨💻 Kepler's laws of planetary motion, validated by Newton's gravitational theory, reveal the consistent, elliptical nature of planetary orbits.
- ⏱ Kepler's third law establishes a proportional relationship between the cube of a planet's orbital radius and the square of its orbital period, applicable to all planets.
- ⚡ The application of Newton's law of universal gravitation extends to practical challenges, such as designing spacesuits for use in the differing gravitational forces of other planets.
Q & A
What are Newton's three laws of motion?
-Newton's three laws of motion describe how things move: (1) An object at rest stays at rest and an object in motion stays in motion unless acted on by an outside force; (2) Force equals mass times acceleration; (3) For every action there is an equal and opposite reaction.
How did Newton connect the idea of gravity to more distant objects like the Moon?
-Newton realized that the same force that causes apples to fall to the ground might also affect more distant objects like the Moon. He saw that the Moon orbits Earth instead of crashing to the ground because it has a sideways motion that causes it to keep missing the Earth.
What is Newton's law of universal gravitation?
-Newton's law of universal gravitation states that every object in the universe attracts every other object with a force that depends on the masses of the objects and the distance between them. He expressed this in the equation F = GMm/r^2.
How did Newton prove Kepler's three laws of planetary motion?
-By combining his laws of motion and the law of universal gravitation with calculus, Newton was able to mathematically prove Johannes Kepler's three empirical laws describing planetary orbits.
What are Kepler's three laws?
-Kepler's three laws state that: (1) planetary orbits are ellipses with the Sun at one focus; (2) a line connecting a planet to the Sun sweeps out equal areas in equal times; and (3) the ratio relating a planet's orbital period and semi-major axis is the same for all planets orbiting the Sun.
What is the gravitational acceleration at the surface of Mars?
-Using Newton's law of universal gravitation and the known mass and radius of Mars, the gravitational acceleration at the surface of Mars can be calculated as 3.7 m/s^2. This is about 38% of Earth's surface gravity.
How did Newton not know the value of the gravitational constant G?
-When formulating his law of universal gravitation, Newton knew there had to be a very small constant to make the gravitational force between everyday objects negligible. But the precise value of this constant, known as big G, wasn't measured until over 100 years later.
How did the possibly apocryphal story of the apple inspire Newton?
-The famous story says Newton saw an apple fall from a tree and wondered if the same force causing the apple to fall straight down could also cause the Moon to continuously "fall" around Earth in orbit due to the Moon's sideways motion.
How did Newton improve upon Kepler's laws?
-Newton showed how very slight deviations in the planets' orbits from Kepler's precise laws could be explained by gravitational tugs from other planets and moons in the solar system.
How is small g related to Newton's equation?
-Small g, the acceleration due to gravity at Earth's surface, is equivalent to Newton's equation for gravitational acceleration: g = G x M_Earth / R_Earth^2.
Outlines
🍎 Newton's Law of Universal Gravitation
This paragraph discusses how Isaac Newton transformed physics by proposing the concept of gravity and formulating the law of universal gravitation. It talks about how scientists previously did not connect the motion of falling objects to the orbits of planets and moons. Newton realized the same gravitational force governs both. The story behind his inspiration from an apple falling from a tree is also recounted. Newton derived an equation calculating the gravitational force between any two objects based on their masses and distance.
🔭 Newton Proves Kepler's Laws
This paragraph explains how Newton used his law of gravitation along with his laws of motion and calculus to prove the three empirical laws about planetary orbits formulated by Johannes Kepler. Kepler's laws describe the elliptical shape of orbits with the Sun at one focus point, the equivalent areas swept in equal times, and a mathematical relation between orbit size and period. Newton also explained the slight deviations from Kepler's predictions as due to gravitational pulls between the planets and moons.
Mindmap
Keywords
💡gravity
💡orbit
💡law of universal gravitation
💡calculus
💡Kepler's laws
💡acceleration
💡NASA
💡Vomit Comet
💡physics
💡mathematics
Highlights
Newton transformed physics with his three laws of motion and understanding of gravity
Newton connected the falling apple to the orbiting moon through the concept of gravity
Newton proposed the law of universal gravitation to calculate gravitational force between objects
Newton used his laws to prove Johannes Kepler's three laws describing planetary orbits
Newton's equation calculates gravitational acceleration from mass and distance
Henry Cavendish later calculated the value of the gravitational constant G
Newton explained slight deviations in orbits from Kepler's laws
Law of universal gravitation matches form of Newton's second law relating force, mass and acceleration
Gravitational acceleration g on Earth's surface can be calculated from G and Earth's mass/radius
NASA uses Newton's gravitation equations to test spacesuits for Mars' gravity
Calculated Mars surface gravity acceleration to be 3.7 m/s^2 for spacesuit testing
Newton inspired practical applications of gravity calculations centuries later
Newton developed concept of gravitational force affecting distant objects like Moon
Realized gravitational force depends on mass of objects and distance between them
Newton's gravitation law applies universally between any two objects with mass
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: