Chain rule | Derivative rules | AP Calculus AB | Khan Academy

Khan Academy
30 May 201805:07
EducationalLearning
32 Likes 10 Comments

TLDRThe video script introduces the chain rule, a fundamental concept in calculus, which is used to find the derivative of composite functions. The instructor demystifies the chain rule by walking through a step-by-step example, differentiating the function h(x) = sin(x^2) with respect to x. The process involves recognizing the outer and inner functions, applying the chain rule to find the derivative of the outer function with respect to the inner function, and then multiplying by the derivative of the inner function with respect to the original variable. The example concludes with the derivative of sin(x^2) being 2x*cos(x), highlighting the chain rule's utility and encouraging further exploration through additional examples in subsequent videos.

Takeaways
  • πŸ“š The core principle discussed is the chain rule in calculus, which is used when taking the derivative of complex functions.
  • πŸ€” Initially, the chain rule may seem daunting, but with more examples, it becomes simpler and more intuitive.
  • 🌟 The chain rule applies when a function is composed of more than one function, which might not be obvious at first.
  • πŸ“ˆ The example given is h(x) = sine of x squared, and the goal is to find the derivative h'(x) or dh/dx.
  • πŸ”„ The chain rule involves finding the derivative of the outer function with respect to the inner function.
  • 🧠 A thought experiment involves understanding the derivative with respect to x, a, and sine of x.
  • πŸ“Š The derivative of x squared with respect to x is 2x, and by analogy, the derivative with respect to sine of x yields 2 sine of x.
  • πŸ”’ The chain rule formula is applied as (derivative of outer function with respect to inner function) times (derivative of inner function with respect to x).
  • πŸ’‘ The derivative of sine of x squared with respect to sine of x is 2 sine of x times the derivative of sine of x with respect to x, which is cosine of x.
  • πŸ“ The final derivative is the derivative of the original function, sine of x squared, with respect to x, which is the chain rule applied to the composition of functions.
  • πŸŽ“ Further examples and abstraction of the concept will be provided in subsequent videos to enhance understanding.
Q & A

  • What is the main topic of this video?

    -The main topic of this video is the chain rule, a core principle in calculus used when taking the derivative of complex functions.

  • How does the instructor describe the initial impression of the chain rule?

    -The instructor describes the chain rule as initially seeming a little daunting and convoluted, but it becomes more understandable and even intuitive with more examples.

  • What function is used as an example to illustrate the chain rule?

    -The function used as an example to illustrate the chain rule is h(x) = sine of x squared, or h(x) = sin^2(x).

  • What is the chain rule applied to in this context?

    -In this context, the chain rule is applied to the derivative of the function h(x) = sin^2(x) with respect to x.

  • What does the chain rule state?

    -The chain rule states that the derivative of a composite function is the derivative of the outer function with respect to the inner function, multiplied by the derivative of the inner function with respect to the variable of interest.

  • What is the derivative of x squared with respect to x?

    -The derivative of x squared with respect to x is 2x.

  • What is the derivative of sine of x squared with respect to sine of x?

    -The derivative of sine of x squared with respect to sine of x is 2 sine of x.

  • What is the final result when applying the chain rule to the example function?

    -The final result when applying the chain rule to the example function, h(x) = sin^2(x), is the derivative with respect to x, which is 2x multiplied by the derivative of sine of x with respect to x, resulting in 2x*cos(x).

  • How does the instructor suggest approaching the notation of differentials in the chain rule?

    -The instructor suggests that while the notation of differentials in the chain rule might look like a fraction and one might be tempted to treat them as such, it is important to remember that they are not numbers and should not be canceled out in a rigorous mathematical sense.

  • What is the derivative of sine of x with respect to x?

    -The derivative of sine of x with respect to x is cosine of x, denoted as cos(x).

  • What will be covered in the next video?

    -In the next video, the instructor plans to provide more examples and attempt to abstract the concept of the chain rule further to enhance understanding.

Outlines
00:00
πŸ“š Introduction to the Chain Rule in Calculus

This paragraph introduces the chain rule, a fundamental concept in calculus that is essential for differentiating complex functions. The instructor explains that while the chain rule may initially seem challenging, it becomes more intuitive with practice and examples. The paragraph begins with a hypothetical function, h(x) = sin(x^2), and poses the question of finding its derivative, h'(x). The chain rule is then explained as a method for differentiating composite functions, demonstrated through a thought experiment involving the derivative of x^2 with respect to x, and then generalized to functions of the form f(g(x)). The process of applying the chain rule to the given function is detailed, leading to the conclusion that the derivative of sin(x^2) with respect to x is 2sin(x)cos(x). The paragraph emphasizes the importance of understanding the chain rule not just as a formula, but as a conceptual tool for differentiating composite functions.

05:01
πŸ”„ Further Explanation and Abstraction of the Chain Rule

This paragraph continues the discussion on the chain rule, with the instructor expressing intent to provide more examples and abstract the concept further in the subsequent video. The goal is to solidify the understanding of the chain rule and its application in calculus. Although the content of the next video is not detailed in this paragraph, the expectation is set for a deeper exploration of the chain rule, which will likely include additional practice problems and varied examples to reinforce the concepts introduced in the current video.

Mindmap
Keywords
πŸ’‘Calculus
Calculus is a branch of mathematics that deals with the study of change and motion, primarily through the use of limits, derivatives, and integrals. In the context of this video, calculus is the subject matter being taught, with a focus on understanding and applying one of its core principles, the chain rule.
πŸ’‘Chain Rule
The chain rule is a fundamental principle in calculus that is used to find the derivative of a composite function. It states that the derivative of a function that is made up of one function nested inside another function is the derivative of the outer function times the derivative of the inner function. The chain rule is essential for solving more complex problems in calculus and is the main focus of this video.
πŸ’‘Derivative
In calculus, a derivative represents the rate of change or the slope of a function at a particular point. It is a fundamental concept used to analyze the behavior of functions, such as their increases and decreases, and the points at which they change direction. The video script introduces the concept of finding the derivative of a function as a part of explaining the chain rule.
πŸ’‘Composite Function
A composite function is a function that is formed by combining two or more functions in such a way that the output of one function becomes the input of another. This concept is crucial for understanding the chain rule because the rule is specifically used to find the derivative of these types of functions.
πŸ’‘Sine Function
The sine function is a trigonometric function that relates the ratio of the opposite side to the hypotenuse in a right triangle to the angle in that triangle. It is periodic and oscillates between -1 and 1, making it useful for modeling various real-world phenomena. In the video, the sine function is used as an example to demonstrate the application of the chain rule.
πŸ’‘Square
In mathematics, squaring a number refers to multiplying that number by itself. The concept of squaring is used in the video to create a function, x squared, which is then used to construct the composite function h(x) = sine of x squared.
πŸ’‘Rate of Change
The rate of change is a concept in calculus that describes how a quantity changes in relation to another quantity. It is often represented graphically as the slope of a curve at a specific point. The derivative is a measure of the rate of change and is central to the study of how functions behave as their inputs change.
πŸ’‘Trigonometry
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles, particularly right triangles. It involves the use of trigonometric functions such as sine, cosine, and tangent, which are essential for solving problems involving periodic phenomena. In the video, trigonometry is relevant because the sine function is used to illustrate the chain rule.
πŸ’‘Function Composition
Function composition is the process of applying one function to the result of another function. It is a fundamental concept in mathematics where one function 'composes' another, resulting in a new function. In the context of the video, understanding function composition is essential for grasping the chain rule, as it often involves the composition of multiple functions.
πŸ’‘Slope
The slope of a curve at a particular point is a measure of how steep the curve is at that point. It is a concept closely related to derivatives in calculus, as the derivative of a function at a point gives the slope of the tangent line to the graph of the function at that point. The video script discusses the concept of slope in the context of finding the derivative of a function.
πŸ’‘Intuition
Intuition in the context of mathematics refers to the ability to understand or grasp concepts without the need for explicit reasoning or derivation. It is the 'gut feeling' or 'sense' one develops for how mathematical concepts work and relate to each other. The video script emphasizes the importance of developing intuition for the chain rule to make it seem less daunting and more straightforward over time.
Highlights

The video introduces the chain rule, a fundamental principle in calculus.

The chain rule is used when taking the derivative of a function composed of multiple functions.

The chain rule can initially seem complex, but with examples, it becomes more intuitive.

The example function h(x) = sin(x^2) is used to illustrate the chain rule.

The derivative h'(x) is sought, which is written as the derivative of h with respect to x.

A thought experiment is presented to help understand the chain rule's application.

The derivative of x^2 with respect to x is demonstrated to be 2x.

The derivative of a squared function is shown to be the same when replacing x with another variable.

The concept of differentiating with respect to a function (like sin(x)) is introduced.

The chain rule is expressed as the derivative of the outer function times the derivative of the inner function.

The derivative of sin(x^2) with respect to sin(x) is calculated as 2sin(x).

The process of applying the chain rule is demonstrated with a step-by-step calculation.

The derivative of sin(x) with respect to x is identified as cos(x).

The chain rule helps to develop an intuition for derivatives involving composite functions.

The final result of the chain rule application is the derivative of the original function with respect to x.

The video promises further examples and abstraction in the next installment.

Transcripts

Browse More Related Video

Worked example: Derivative of cos_(x) using the chain rule | AP Calculus AB | Khan Academy

Exponential functions differentiation | Advanced derivatives | AP Calculus AB | Khan Academy

The Chain Rule

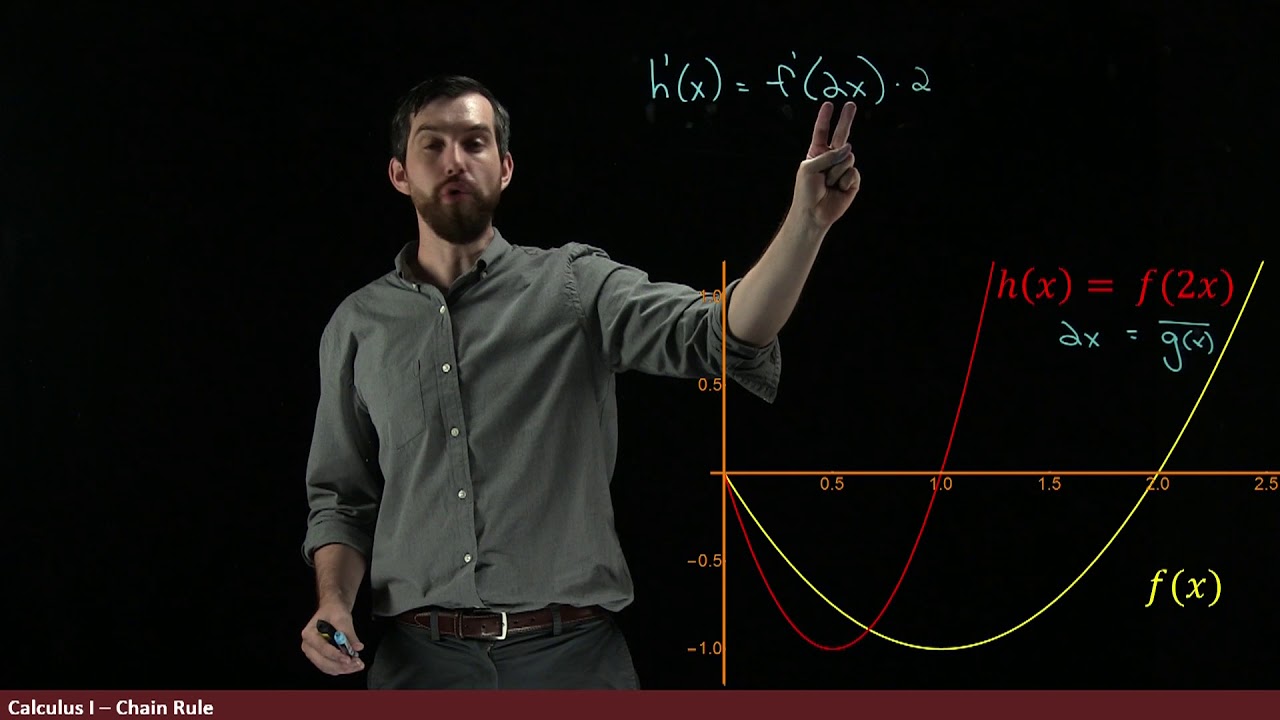
Interpreting the Chain Rule Graphically

Applying the chain rule twice | Advanced derivatives | AP Calculus AB | Khan Academy

Worked example: Derivative of Ã(3x_-x) using the chain rule | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus PrinciplesChain RuleDerivative ConceptsMathematical InsightEducational ContentComplex FunctionsTrigonometryAlgebraOnline Learning