Derivatives... How? (NancyPi)

NancyPi
15 May 201814:30
EducationalLearning
32 Likes 10 Comments

TLDRThe video script offers a comprehensive guide on calculating derivatives using various rules, emphasizing their significance in calculus and scientific applications. It explains the concept of derivatives as the instantaneous rate of change and introduces the Power Rule, Constant Multiple Rule, and Sum and Difference Rule for polynomials. The script further delves into the Product Rule for multiplying functions and the Quotient Rule for dividing functions, providing clear examples and mnemonics for better understanding. The video assures viewers that while calculus can be challenging, mastering these rules can simplify the process and emphasizes the availability of additional resources for further learning.

Takeaways
  • 📚 Derivatives are a fundamental concept in calculus and have wide applications in scientific fields.
  • 🔍 The derivative of a function represents the instantaneous rate of change or the slope of the tangent line at any point on the curve.
  • 📈 To find the derivative of a polynomial, apply the derivative rules to each term individually and combine them.
  • 🌟 Use the Power Rule for terms with x raised to a power: bring the power down as a coefficient and decrease the exponent by 1.
  • 🔢 For terms with a constant multiplied by x (e.g., 2x^3), keep the constant and apply the Power Rule to the x term.
  • 📊 The derivative of x (x^1) is 1, as per the Power Rule, since x^0 equals 1.
  • 🚫 The derivative of a constant is 0, as constants do not change over time or with respect to x.
  • 🔍 For products of functions (u*v), use the Product Rule: (u derivative)v + (v derivative)u.
  • 📈 For quotients of functions (u/v), apply the Quotient Rule: (bottom derivative * top) - (top derivative * bottom) / (bottom squared).
  • 🔄 The Chain Rule is necessary for more complex functions and can be explored in dedicated resources for further understanding.
Q & A

  • What is the main concept discussed in the video?

    -The main concept discussed in the video is how to take derivatives using various rules in calculus.

  • What does the derivative represent in a function?

    -The derivative represents the instantaneous rate of change at each point of a function, giving the slope of the tangent line to the curve at every point of the original function.

  • What is the Power Rule for differentiation?

    -The Power Rule states that to take the derivative of a power of x, you bring down the power as a coefficient and then decrease the power by 1.

  • How is the Constant Multiple Rule applied in differentiation?

    -The Constant Multiple Rule states that when you have a constant multiplied in front of a term, you keep the constant and then take the derivative of the rest of the term.

  • What is the Sum and Difference Rule in differentiation?

    -The Sum and Difference Rule allows you to find the derivative of a polynomial by differentiating each term individually and then combining them to form the full derivative.

  • What is the Product Rule used for in differentiation?

    -The Product Rule is used to take the derivative of two functions multiplied together. It involves taking the derivative of one function and multiplying it by the other function, and vice versa, and then combining the results.

  • How do you apply the Quotient Rule in differentiation?

    -The Quotient Rule states that to take the derivative of a quotient, you take the bottom (denominator) times the derivative of the top (numerator) minus the top times the derivative of the bottom, all divided by the bottom squared.

  • What is the Chain Rule in differentiation?

    -The Chain Rule is a method used in differentiation for handling more complex functions, especially when dealing with composite functions. It involves differentiating the outer function and then multiplying it by the derivative of the inner function.

  • How does the video address the concept of negative and fraction powers in differentiation?

    -The video explains that for negative and fraction powers, the same process of bringing down the power as a coefficient and decreasing the power by 1 is followed, but with additional considerations such as handling more negative numbers for negative powers and finding a common denominator for fraction powers.

  • What is the derivative of x to the power of 1?

    -The derivative of x to the power of 1 (x^1) is 1, because using the Power Rule, you bring down the power of 1 to the front as a coefficient, and then decrease the power by 1, which results in x^0, and x to the power of 0 is 1.

  • What is the significance of the derivative in the context of the mathematical underpinnings of science?

    -The derivative is significant in the mathematical underpinnings of science as it provides a way to determine the instantaneous rate of change and the slope of a curve at any given point, which is crucial for modeling and understanding various scientific phenomena.

Outlines
00:00
📚 Introduction to Derivatives and Derivative Rules

This paragraph introduces the concept of derivatives, emphasizing their importance in calculus and scientific applications. It defines derivatives as new functions that represent the instantaneous rate of change or the slope of the tangent line at any point on the original function. The video aims to simplify the process of finding derivatives using derivative rules, which are shortcuts to the more complex definition involving limits. The paragraph outlines the Power Rule for basic power functions and the Constant Multiple Rule, illustrating how to find derivatives of polynomials by applying these rules to each term individually. It also mentions that a video on the definition of the derivative with limits is available for those who need it.

05:05
📈 Derivative of Polynomials and Constant Rule

This paragraph delves deeper into the process of finding the derivative of a polynomial by differentiating each term separately. It explains the Sum and Difference Rule, which allows the derivative of a polynomial to be found by summing the derivatives of its individual terms. The Constant Rule is introduced, stating that the derivative of a constant is zero. The paragraph also touches on handling negative and fraction powers, cautioning viewers to be mindful of the potential undefined nature of derivatives involving fraction powers at x=0. It then transitions into discussing the Product Rule for differentiating the product of two functions, providing an example to illustrate the application of the rule.

10:06
📊 Quotient Rule and Chain Rule

The final paragraph focuses on the Quotient Rule for differentiating a quotient of two functions and the Chain Rule for more complex functions. The Quotient Rule is explained with a step-by-step breakdown, including the need to account for the denominator squared in the final result. An example is worked through to demonstrate the process clearly. The Chain Rule is mentioned as a more advanced topic, with a reference to a dedicated video for those interested in learning more. The paragraph concludes by encouraging viewers to practice and seek further resources if needed, and it ends with a call to action for viewers to like and subscribe to the video.

Mindmap
Keywords
💡Derivatives
Derivatives are a central concept in calculus, representing the rate of change or the slope of a function at any given point. In the video, the presenter explains how to calculate derivatives using various rules, emphasizing their importance in understanding the behavior of functions and their graphical representations, such as the slope of the tangent line at any point on a curve.
💡Instantaneous Rate of Change
The instantaneous rate of change refers to the derivative's ability to capture the exact speed or rate at which a function changes at a specific moment or point. In the context of the video, this concept is crucial for understanding the steepness or flatness of a curve at any given x-value, which is represented by the derivative of the function at that point.
💡Power Rule
The Power Rule is a fundamental derivative rule that states when taking the derivative of a term of the form x^n, where n is a positive integer, you bring the exponent down as a coefficient and subtract one from the exponent. This rule is used extensively in the video to find the derivatives of terms involving x raised to various powers.
💡Constant Multiple Rule
The Constant Multiple Rule states that when taking the derivative of a term that has a constant multiplied by a variable, the constant remains and the derivative is taken only of the variable part. This rule is important for understanding how to handle constants in polynomial expressions when differentiating.
💡Sum and Difference Rule
The Sum and Difference Rule allows for the differentiation of a polynomial by finding the derivative of each term separately and then combining them according to their signs. This rule simplifies the process of differentiating complex polynomials by breaking them down into simpler parts.
💡Product Rule
The Product Rule is a calculus rule used when differentiating a product of two functions. It states that the derivative of a product is the derivative of the first function times the second function, plus the first function times the derivative of the second function. This rule is essential for handling more complex functions that are the result of multiplying two simpler expressions.
💡Quotient Rule
The Quotient Rule is a calculus rule for differentiating a quotient of two functions. It states that the derivative of a quotient is the derivative of the numerator times the denominator, minus the numerator times the derivative of the denominator, all divided by the square of the denominator. This rule is crucial for handling expressions that involve division.
💡Chain Rule
The Chain Rule is a powerful calculus rule used when differentiating a composite function, which is a function made up of other functions. It involves differentiating the outer function and then multiplying by the derivative of the inner function. The Chain Rule is essential for breaking down complex functions into manageable parts.
💡Slope of the Tangent Line
The slope of the tangent line is a concept used to describe the steepness of a curve at a particular point. It is the value of the derivative at that point and provides a measure of how quickly the function is changing at that specific location. In the video, the slope of the tangent line is used to explain the geometric interpretation of derivatives.
💡Limits
Limits are a fundamental concept in calculus that describe the behavior of a function as the input approaches a certain value. While not the main focus of the video, the mention of limits is important because the definition of the derivative involves limits, specifically the limit of the difference quotient.
💡Negative and Fractional Powers
Negative and fractional powers extend the concept of exponents beyond positive integers, allowing for the differentiation of functions with terms involving variables raised to non-integer powers. The rules for differentiating such powers involve adjustments to the exponent, including finding a common denominator when necessary.
Highlights

Derivatives are a central concept in calculus and essential for understanding the mathematical underpinnings of science.

The derivative represents the instantaneous rate of change at each point of a function, providing the slope of the tangent line at that point.

Derivative rules are shortcuts that simplify the process of finding derivatives, making it more manageable.

The Power Rule states that for a power of x, you bring the power down as a coefficient and decrease the power by 1.

The Constant Multiple Rule allows you to keep a constant in front of a term and take the derivative of the rest of the term.

For the term -x^2, the derivative is found by applying the Power Rule, resulting in -2x.

The derivative of a term like +4x is simply the number in front of x, which is 4 in this case.

The derivative of x is 1, as x is x^1 and applying the Power Rule gives 1*x^0, which is 1.

The Constant Rule states that the derivative of a number, a constant, is 0.

The Sum and Difference Rule allows you to differentiate each term in a polynomial individually and then combine them for the full derivative.

For negative powers, you can apply the same process as with positive powers, but be cautious as reducing the power by 1 results in a more negative exponent.

With fraction powers, you bring the power down as a coefficient, subtract 1 from the original power, and find a common denominator for the new power.

The Product Rule is used when you have two functions multiplied together, and it involves taking the derivative of one function and multiplying it by the other, and vice versa.

The Quotient Rule is applied when you have one function divided by another, and it involves complex manipulation of the numerator and denominator, including their derivatives.

The Chain Rule is another important concept in calculus for dealing with more complex functions, and there is a dedicated video for learning how to use it.

Understanding derivatives and their rules is crucial for various applications in mathematics, physics, and engineering.

The video provides a comprehensive guide to taking derivatives, making it accessible even for those who might not enjoy math.

Transcripts

Browse More Related Video

Derivatives of Trig Functions - Calculus | MCV4U

Business Calculus - Math 1329 - Section 2.3 - Product and Quotient Rules; Higher-order Derivatives

Review of all Derivative Rules | Calculus | jensenmath

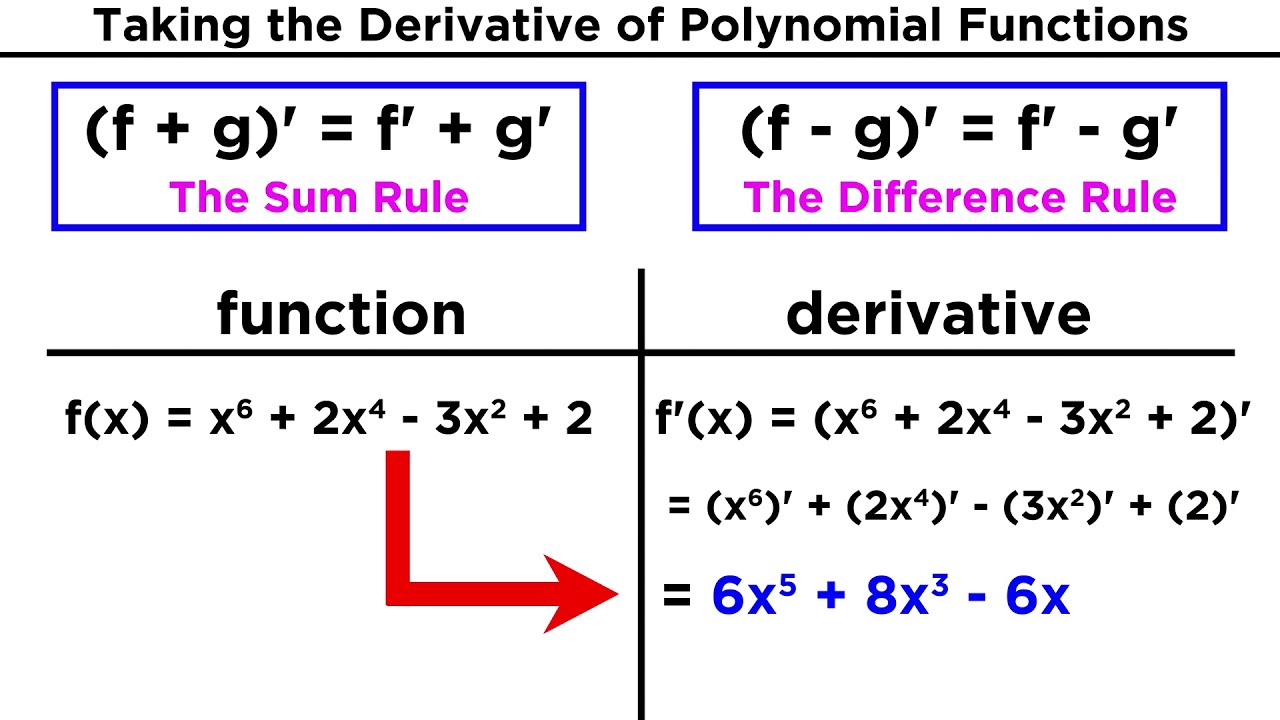
Derivatives of Polynomial Functions: Power Rule, Product Rule, and Quotient Rule

AP Calculus AB and BC Unit 2 Review [Differentiation: Definition and Basic Derivative Rules]

Differentiation Rules - Power/Product/Quotient/Chain

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativesCalculusMathematicsEducationPower RuleProduct RuleQuotient RuleChain RuleLearningWebinar