85% of My Students Failed this Math Exam
TLDRIn this educational video, the host explores the concept of derivatives and their applications, particularly in the context of crafting and interpreting mathematical functions. The video kicks off with a discussion about a disappointing exam result, where students struggled to understand the basics of derivatives. The host then introduces an online learning platform, 'Brian,' which offers interactive courses to enhance STEM learning. The main content delves into exercises involving finding slopes, zeros, and analyzing the behavior of functions and their derivatives. The video also covers the process of sketching tangents, identifying inflection points, and calculating equations of tangents to functions. The host emphasizes the importance of understanding these mathematical concepts and provides resources for further learning, including a free trial for the 'Brian' platform and a link to the exam for viewers' practice.
Takeaways
- 📚 The video is part of an educational series focusing on math, specifically derivatives and their applications.
- 👨🏫 The presenter is a teacher who has conducted an exam on derivatives for 12th-grade students, which did not go well due to a lack of understanding.
- 🎓 The video includes an introduction to an online learning platform called 'Brian' that offers interactive courses in STEM fields.
- 📈 Brian's platform is recommended for its visualization tools, helping students understand complex concepts through graphics and interactive exercises.
- 🎁 The video offers a 30-day free trial for Brian's services, with a special link provided in the video description for additional discounts.
- 📝 The exam structure is explained, consisting of two parts: one without support materials and one with, covering topics like function interpretation and derivative application.
- 📉 The video provides detailed solutions to the exam questions, including finding zeros of functions, analyzing graphs, and calculating derivatives.
- 🤔 The presenter expresses frustration with the students' performance on the exam, despite the exam's perceived ease and the availability of educational tools.
- 📑 The exam paper and solutions are available for viewers to use for their own studies or as teaching material.
- 🔍 The video emphasizes the importance of understanding derivatives and their applications, as well as the need for clear communication and problem-solving skills.
Q & A
What series is the video part of?
-The video is part of the 'educational exam' series on the channel.
What is the topic of the exam in the video?
-The exam is about derivatives and their applications, particularly in crafts and interpreting crafts.
What was the average grade students received on this exam?
-The average grade of the students on this exam was two or three out of a possible 15.
What platform does the teacher recommend for visual learners?
-The teacher recommends the online learning platform 'Brian' for visual learners.
What kind of courses does Brian offer?
-Brian offers nearly 70 interactive courses in various STEM fields such as mathematics, physics, chemistry, and computer science.
What is the structure of the first part of the exam?
-The first part of the exam is without support materials and includes checking boxes and basic understanding of concepts like continuity and function zeros.
How much time do students have for the first part of the exam?
-Students have 20 minutes to complete the first part of the exam.
What is the second exercise in the first part of the exam?
-The second exercise requires students to decide which of the graphs does not correspond to a certain function and justify their answer.
What is the duration of the second part of the exam?
-Students have 80 minutes to complete the second part of the exam.
What is allowed in the second part of the exam?
-Support materials such as a table of formulas and calculators are allowed in the second part of the exam.
What is the first exercise in the second part of the exam?
-The first exercise in the second part is interpreting a given function and discussing inflection points and the behavior of the derivative function.
What is the function F(x) in the context of the exam?
-The function F(x) is a real-valued function with a slope of 12 at x being equal to 2.
What is the domain of the function f in the exam?
-The domain of the function f is the real numbers, excluding where the function is undefined, such as at x = 3 where there is a vertical asymptote.
How many zeros does the polynomial function f(x) have in the exam?
-The polynomial function f(x) has two zeros: one at x = -4 with algebraic multiplicity of 2, and another at x = 0.
What is the equation of the tangent to the graph of a polynomial function of degree 4 at the inflection point?
-The equation of the tangent is y = 8x + 8, derived from the slope of -4 at the inflection point (4, 18).
What is the set of functions FK in the exam?
-The set of functions FK is defined as FK(x) = k*x*(x - 8) where k is an element of the positive real numbers excluding zero.
What is the maximum point of the graph of FK and how is it shown?
-The maximum point of the graph of FK is at (4, 16k). It is shown by differentiating the function, setting the derivative equal to zero to find the x-value of the maximum, and then showing that the second derivative at this point is negative.
How many inflection points does the graph of FK have?
-The graph of FK does not have any inflection points, as it is a parabola with only one maximum.
What is the equation for the first derivative of the function x/(x-1)^2?
-The first derivative, using the product and chain rules, is (x-1)^(-2) + x*(-3)*(x-1)^(-3).
What is the volume formula for a sphere and its derivative with respect to radius r?
-The volume formula for a sphere is (4/3)πr^3. Its derivative with respect to radius r is 4πr^2.
What is the first derivative of the function H(x) = 3x^2*(2 - x^3) + 6.9?
-The first derivative, using the product and chain rules, is 6x*(2 - x^3) - x^2*(6x^2).
Outlines
📚 Introduction to the Educational Series on Derivatives
The video begins with an enthusiastic greeting to fellow mathematicians and introduces the topic of derivatives, which is part of an ongoing educational series. The speaker reflects on the performance of students in a recent exam, expressing dissatisfaction with their average grade of two or three out of fifteen. The poor performance is attributed to a lack of understanding of the material. The video is sponsored by Brian, an online learning platform with interactive courses in STEM fields, which is praised for its effectiveness in teaching complex concepts through visualization. The sponsor offers a 30-day free trial and a 20% discount for the first 200 users who sign up through the provided link.
📘 Exam Structure and Student Performance
The script outlines the structure of a recent math exam, which consists of two parts. The first part involves basic understanding and multiple-choice questions, while the second part allows for support materials like formula tables and calculators. The exam covers topics such as interpreting functions, finding inflection points, and applying derivative rules. The speaker expresses frustration with the students' performance, noting that despite the exam not being particularly difficult, they struggled significantly. The video script also includes a link to the exam papers for viewers who wish to use them for study or teaching purposes.
🔍 Detailed Analysis of Polynomial Functions and Their Graphs
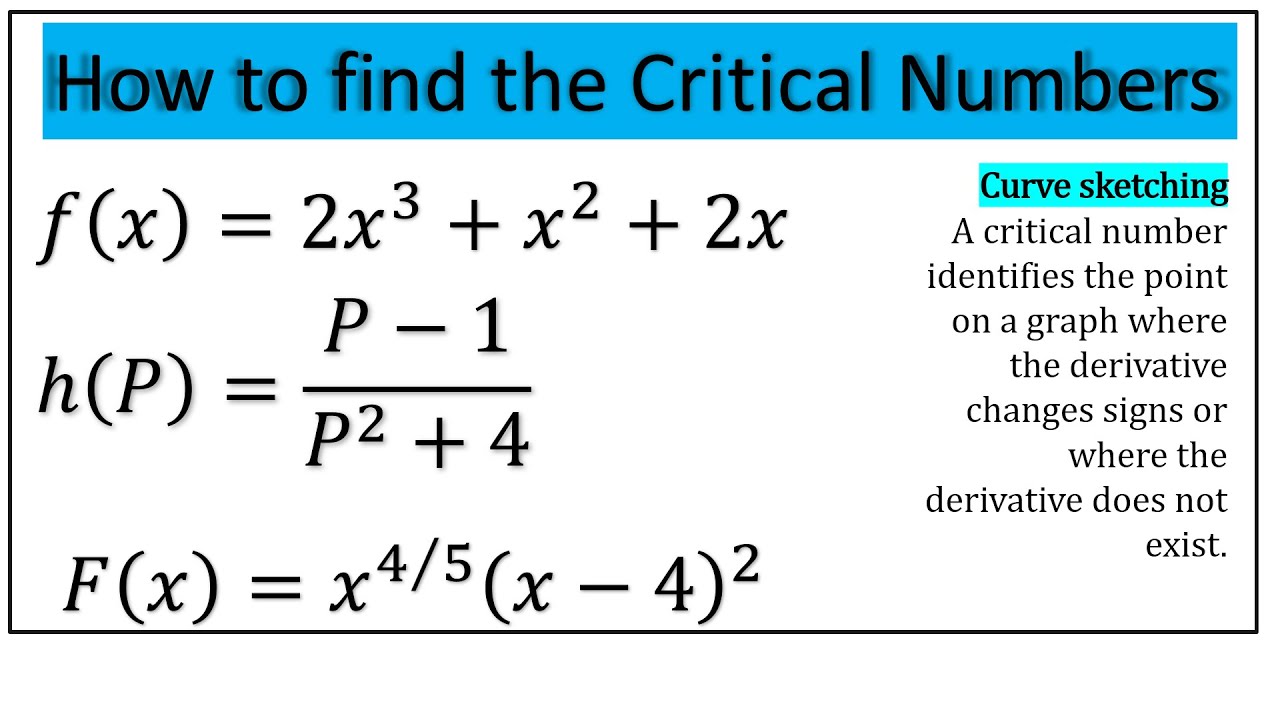
This paragraph delves into the specifics of the exam questions, which involve analyzing polynomial functions and their graphical representations. The speaker guides viewers through solving problems related to finding the zeros of functions, determining the domains, and identifying vertical asymptotes. The importance of understanding the behavior of functions and their derivatives is emphasized, with the speaker providing step-by-step solutions to the exam questions. The paragraph also discusses common student mistakes and misunderstandings regarding the properties of polynomial functions.
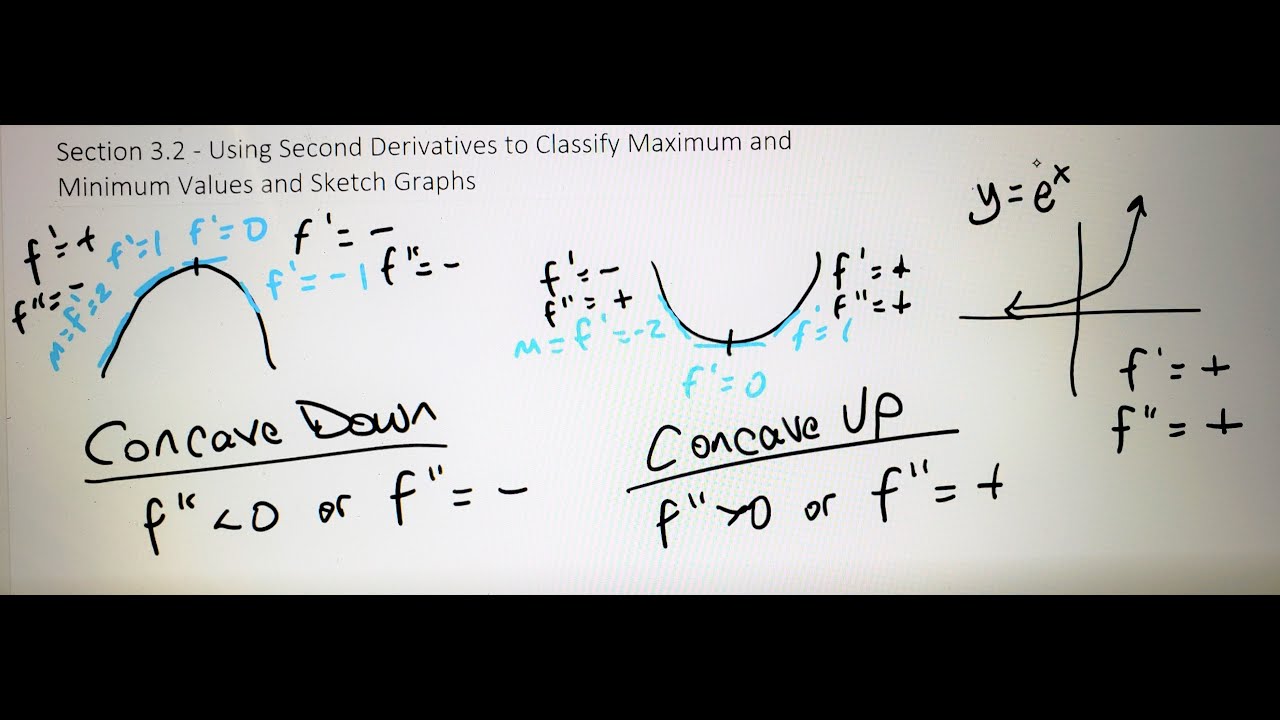
📉 Investigation of Derivatives and Inflection Points
The focus shifts to a higher-level analysis involving the second derivative and inflection points of a fourth-degree polynomial function. The speaker explains how to determine the zeros of the derivative function, which indicate the points of extrema (maximum or minimum). The video demonstrates how to use the graph of the function to infer the presence of inflection points and argues that a fourth-degree polynomial can have at most three extreme points and two inflection points. The speaker also addresses common errors made by students in interpreting the graphical information and applying calculus concepts.
📈 Derivative Calculations and Function Analysis
The script continues with a series of calculus problems that require finding the first derivative of given functions. The speaker uses the product rule and chain rule to differentiate functions and emphasizes the importance of understanding these rules for solving calculus problems. The paragraph also includes a discussion on the volume formula of a sphere and how to differentiate it with respect to the radius. The speaker highlights the simplicity of the problems and expresses surprise at the students' difficulty in solving them.
🎓 Reflecting on the Exam and Encouraging Self-Study
In the concluding paragraph, the speaker reflects on the overall difficulty of the exam, considering it to be quite easy and structured in a way that builds upon previous concepts. The speaker is puzzled by the students' performance and encourages viewers to use the exam as a learning tool. There is an invitation for feedback in the comments section and a reminder about the sponsor, Brian, and the support for the channel through Patreon. The video ends with well-wishes for the viewers and a teaser for the next video.
Mindmap
Keywords
💡Derivatives
💡Educational Exam
💡Inflection Points
💡Sponsorship
💡Online Learning Platform
💡Visualization
💡Stem Field
💡Parameterized Functions
💡Tangents
💡Differentiability
Highlights
Introduction of an educational exam series focused on derivatives and their applications.
The exam results were poor, with an average grade of two or three out of fifteen, indicating a lack of understanding of the topic among students.
Sponsorship mention of Brian, an online learning platform with interactive courses beneficial for visual learners.
Brian's platform offers nearly 70 interactive courses covering various STEM fields.
The presenter uses Brian to visualize mathematical concepts and functions for better understanding in his classes.
A 30-day free trial is offered for Brian's platform, with an additional discount for the first 200 users through the provided link.
Structure of the exam is explained, consisting of two parts with varying time limits and allowed materials.
The first part of the exam tests basic understanding of continuity and function properties without support materials.
The second part of the exam allows support materials and includes exercises on interpreting functions and using derivative rules.
An exercise is presented to find the function with a slope of 12 at x=2 by evaluating derivatives of given polynomial functions.
A function is defined with a domain issue at x=3, leading to a vertical asymptote and undefined behavior.
An exercise to determine which function is not defined at x=-3 by evaluating the function's behavior at that point.
An analysis of a polynomial function's graph to determine which statements about its derivatives are true.
A task to identify zeros and analyze the behavior of a function defined as 2x * (x + 4)^2.
An exercise to determine which graphs correspond to a given function and to justify the answer.
A challenge to sketch a tangent to a given inflection point on a graph and justify the absence of additional inflection points.
An exercise to calculate zeros of a derivative function and to determine the coordinates of its minimum.
A task to show that a parabola defined by FK(x) = k*x*(x - 8) has a maximum at the point (4, 16k) and no inflection points.
An exercise to prove that the graph of a function is always increasing at a specific point by using the derivative.
Calculation of the equation of a tangent to a function at a given point and finding its y-intercept.
Differentiation of various functions, including a sphere's volume formula and a density function.
Reflection on the exam's difficulty and the presenter's surprise at the students' performance.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: