What is a derivative?
TLDRThe video script provides an in-depth explanation of derivatives, focusing on their role as a method to determine the instantaneous rate of change. It begins by illustrating the concept using a curve with two points, explaining how the slope between these points represents the average rate of change. The script then introduces the concept of limits to refine this into the instantaneous rate of change, leading to the definition of a derivative. The difference quotient is presented as a preliminary form of the derivative, which is later identified as 'f prime of X'. The script demonstrates the calculation of derivatives through examples, including finding the slope of a tangent line to a parabola and determining turning points of a cubic function. It emphasizes the utility of derivatives in understanding the behavior of functions and their graphs, particularly in identifying maximum and minimum points. The summary concludes with an encouragement to continue learning about derivatives and their applications.
Takeaways
- 📈 A derivative is a way to find the instantaneous rate of change, which is related to the slope of a curve at a particular point.
- 📐 The concept of a derivative involves finding the slope between two points on a curve, which is initially an average rate of change.
- 🔍 The instantaneous rate of change is obtained by using limits, specifically as the distance between the two points (h) approaches zero.
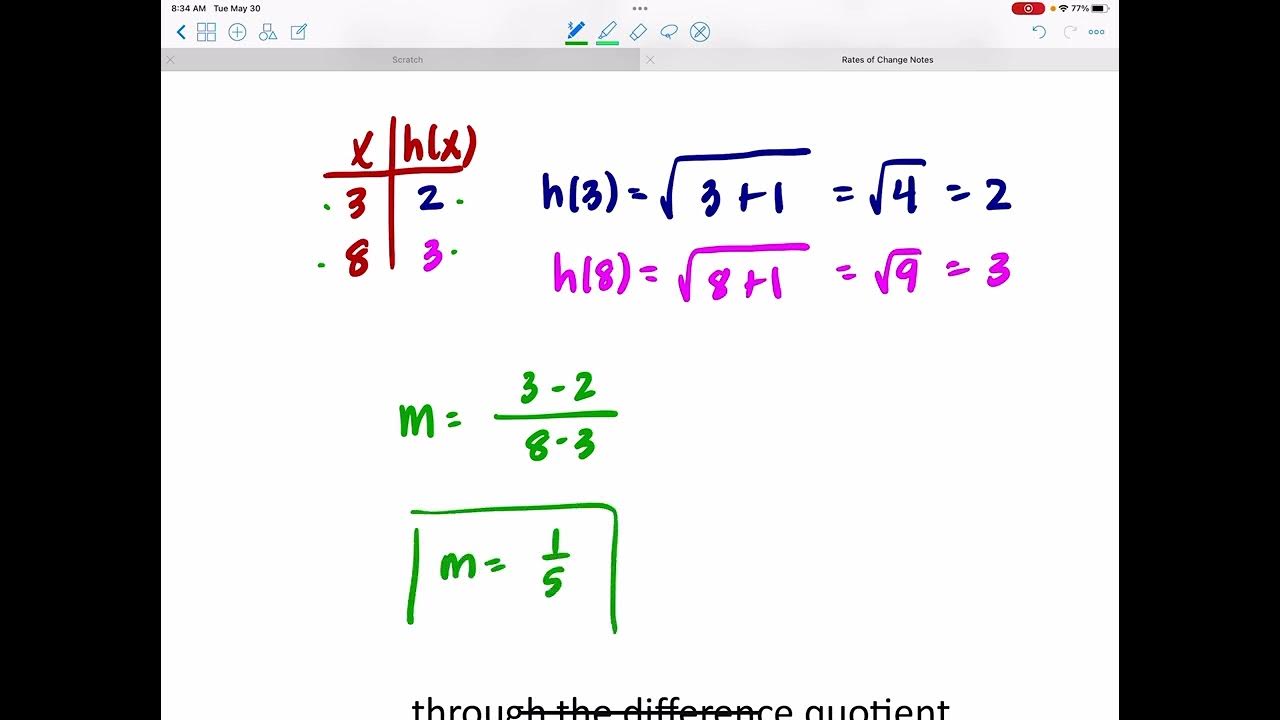
- 📊 The formula for the slope between two points is (f(x + h) - f(x)) / (x + h - x), which simplifies to (f(x + h) - f(x)) / h and is known as the difference quotient.
- 🎓 As h approaches zero, the difference quotient becomes the derivative, represented as f'(x) or the slope of the tangent line at a point on the curve.
- 🧮 The derivative of a function like f(x) = x^2 is found by applying the difference quotient and taking the limit as h approaches zero, resulting in 2x.
- 📌 The slope of the tangent line at a specific point on a curve can be found by substituting the x-coordinate of that point into the derivative.
- 🤔 To find turning points or where the graph changes direction, set the derivative equal to zero and solve for x.
- 📋 The process of finding turning points involves factoring, applying the binomial expansion theorem, and taking the limit as h approaches zero in the derivative formula.
- 📈 The derivative not only gives the slope of the tangent line but also helps in understanding the behavior of the graph, such as its maxima and minima.
- 🔑 Memorizing the derivative formula is crucial for calculating the instantaneous rate of change and analyzing the slope of a curve at any given point.
- 🌟 Understanding derivatives is fundamental to calculus and has wide applications in various fields, including physics, engineering, and economics.
Q & A
What is the basic concept of a derivative?
-A derivative is a way of finding the instantaneous rate of change, which is essentially the slope of the tangent line to a curve at a particular point.
How is the slope between two points on a curve calculated?
-The slope between two points is calculated using the formula (y2 - y1) / (x2 - x1), where y2 and y1 are the y-values (or f(x) values) of the two points and x2 and x1 are their corresponding x-values.
What is the term used for the limit of the difference quotient as h approaches 0?
-The term used is 'derivative,' which is also represented as f'(x) or the derivative of the function f at point x.
How does the derivative help in finding the equation of a tangent line to a curve at a specific point?
-The derivative gives the slope of the tangent line at a specific point. By using the point-slope form of a line, the equation of the tangent line can be found using the derivative as the slope and a point on the curve.
What is the derivative of the function f(x) = x^2?
-The derivative of the function f(x) = x^2 is f'(x) = 2x, which represents the slope of the tangent line to the curve at any point x.
How can you find the turning points of a graph?
-To find the turning points, you set the derivative of the function equal to zero and solve for x. These x-values correspond to the points where the graph changes direction, indicating a local maximum or minimum.
What is the significance of a horizontal tangent line on a graph?
-A horizontal tangent line indicates a point on the graph where the slope is zero, which typically corresponds to a local maximum or minimum, also known as a turning point.
How does the derivative help in understanding the behavior of a polynomial graph?
-The derivative can reveal where the graph is increasing or decreasing by showing where the slope is positive or negative. It can also help find the exact turning points and inflection points, giving a clearer picture of the graph's behavior.
What is the binomial expansion theorem used for in the context of finding derivatives?
-The binomial expansion theorem is used to expand terms like (x + h)^n in the difference quotient, which simplifies the process of finding the derivative by allowing you to distribute and combine like terms more easily.
Why is the limit process important when finding the derivative?
-The limit process is crucial as it allows the transition from the average rate of change to the instantaneous rate of change. By taking the limit as h approaches 0, you get the exact slope of the tangent line at a specific point on the curve.
How does the concept of a derivative relate to real-world applications?
-Derivatives are used in various real-world applications to model and analyze phenomena that involve change, such as physics (motion and forces), engineering (optimization problems), and economics (optimizing profit and cost functions).
What is the difference between the average rate of change and the instantaneous rate of change?
-The average rate of change is the slope of the secant line between two points on a curve, while the instantaneous rate of change is the slope of the tangent line at a specific point, which is found by taking the limit of the average rate of change as the distance between the points approaches zero.
Outlines
📐 Introduction to Derivatives and Instantaneous Rate of Change
The first paragraph introduces the concept of a derivative as a method for determining the instantaneous rate of change, which is essentially the slope of a curve at a given point. A diagram is used to illustrate the concept, showing two points on a curve and how the slope between them can be calculated using the formula (f(x + h) - f(x)) / (x + h - x). This average rate of change is then refined to an instantaneous rate of change by applying limits as the distance 'h' approaches zero, resulting in the difference quotient, which is also known as the derivative of the function. The derivative is further explained as a formula for the slope of the tangent line at any point on a curve, with an example using the function f(x) = x^2 provided to demonstrate how to calculate it.
🔍 Derivatives in Action: Finding Tangent Lines and Turning Points
The second paragraph delves into practical applications of derivatives, such as finding the equation of a tangent line at a specific point on a curve and identifying turning points where the slope of the tangent line is zero. It uses the point-slope form of a line to derive the equation of the tangent line given a point on the curve and its slope. The process of finding turning points by setting the derivative equal to zero is also explained, using the example of the polynomial function f(x) = x^3 - 4x. The derivative of this function is calculated, and the points where the slope is zero are found by solving the equation 3x^2 - 4 = 0, leading to the turning points (1.15, -3.08) and (-1.15, 3.08).
🎓 Summary of Derivatives: Understanding and Application
The final paragraph summarizes the key points about derivatives, emphasizing their role as a formula for the slope of the tangent line and how they are derived from the average rate of change to the instantaneous rate of change using limits. It also highlights the importance of understanding derivatives not just as a theoretical concept but as a tool for practical applications in mathematics, such as analyzing the behavior of functions and their graphs. The speaker encourages viewers to subscribe for more math tutoring and expresses a desire to continue assisting in future videos.
Mindmap
Keywords
💡Derivative
💡Instantaneous Rate of Change
💡Slope
💡Limit
💡Difference Quotient
💡f Prime of X (f'(x))
💡Tangent Line
💡Point-Slope Form
💡Turning Points
💡Binomial Expansion Theorem
💡Polynomial
Highlights
A derivative is a method for finding the instantaneous rate of change.
The concept of 'rate of change' refers to the slope of a curve at a given point.
The slope between two points on a curve can be found using the formula (y2 - y1) / (x2 - x1).
The instantaneous rate of change is obtained by taking the limit as the distance between two points approaches zero.
The difference quotient, limit as h approaches 0 of [f(x + h) - f(x)] / h, is used to find the instantaneous rate of change.
The derivative, f'(x), is another term for the derivative and represents the slope of the tangent line to a curve at a point.
The derivative of f(x) = x^2 is found by applying the difference quotient and taking the limit as h approaches 0, resulting in 2x.
The slope of the tangent line at a specific point can be found by substituting the x-coordinate into the derivative formula.
The equation of the tangent line can be written using the point-slope form: y - y1 = m(x - x1).
Derivatives help in determining the behavior of polynomial graphs, such as finding x-intercepts and turning points.
Turning points on a graph occur where the slope of the tangent line is zero, which can be found by setting the derivative equal to zero.
The derivative of a function can be used to find the maximum and minimum values of the function.
The process of finding the derivative involves algebraic manipulation and application of the limit concept.
The derivative is a powerful tool in calculus for understanding how quantities change and for solving optimization problems.
Mario's Math Tutoring provides comprehensive explanations and examples to help understand the concept and application of derivatives.
The video concludes with an encouragement to subscribe for more math tutoring videos and a promise of future assistance.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: