Getting data from expected value | Probability and Statistics | Khan Academy

Khan Academy
9 Apr 201409:52
EducationalLearning
32 Likes 10 Comments

TLDRIn this video, Jamie tests the fairness of a die she received as a birthday gift by rolling it 500 times and recording the outcomes. After calculating the expected value of the sum of 20 rolls to be 67.4, she encounters a mishap where two values are washed away due to rain. The video challenges viewers to find the two missing frequencies. It guides them through the process of calculating the expected value per roll and setting up equations to solve for the missing values, ultimately revealing that both the number of times a 1 and a 6 were rolled are 75.

Takeaways
  • 🎲 Jamie's dad gifted her a die for her birthday, and she wanted to test its fairness by rolling it 500 times.
  • 📊 To analyze the die's fairness, Jamie recorded the frequency of each number rolled and later calculated the expected value for the sum of 20 rolls to be 67.4.
  • 🌧️ Unfortunately, two values from Jamie's data table were washed away due to rain on her way home from school.
  • 🔢 The expected value of a single roll can be found by dividing the expected value of 20 rolls (67.4) by 20, resulting in 3.37.
  • 📉 Jamie had recorded specific frequencies for numbers 2, 3, 4, and 5, but the frequencies for numbers 1 and 6 were lost.
  • 📚 The expected value of a roll is calculated by taking the weighted sum of each possible outcome, multiplied by their respective frequencies.
  • 🧩 To find the missing frequencies, Jamie set up an equation based on the expected value and the known frequencies.
  • 🔍 By multiplying the expected value equation by 500 (the total number of rolls), Jamie simplified the equation to find a relationship between the missing frequencies.
  • 📉 The sum of all frequencies (including the missing ones) must equal 500, providing a second equation to solve for the missing values.
  • 🔑 Using the two equations, one for the expected value and one for the total number of rolls, Jamie solved for the missing frequencies.
  • 🎉 The solution revealed that both the frequency for number 1 (A) and number 6 (B) were 75, completing Jamie's data table.
Q & A

  • What did Jamie's dad give her for her birthday?

    -Jamie's dad gave her a die for her birthday.

  • Why did Jamie take the die to school and roll it 500 times?

    -Jamie wanted to make sure the die was fair, so she took it to school and rolled it 500 times to track the frequency of each number rolled.

  • What was the expected value of the sum of 20 rolls of the die that Jamie calculated?

    -Jamie calculated the expected value of the sum of 20 rolls to be 67.4.

  • What happened to Jamie's data table on her way home from school?

    -It was raining on her way home, and 2 values were washed away from her data table.

  • What were the absolute frequencies of rolling a 2 and a 3 that Jamie recorded?

    -Jamie recorded rolling a 2, 110 times and a 3, 95 times.

  • What was the absolute frequency of rolling a 4 and a 5 that Jamie recorded?

    -Jamie recorded rolling a 4, 70 times and a 5, 75 times.

  • How did Jamie calculate the expected value of one roll of the die?

    -Jamie calculated the expected value of one roll by dividing the expected value of the sum of 20 rolls (67.4) by 20, which resulted in 3.37.

  • What is the formula for calculating the expected value of a roll based on Jamie's frequency table?

    -The formula for calculating the expected value of a roll is the weighted sum of each value times its frequency (e.g., A/500*1 + 110/500*2 + ... + B/500*6), which should equal 3.37.

  • How did Jamie set up the equation to find the missing absolute frequencies (A and B) for rolling a 1 and a 6?

    -Jamie set up the equation by multiplying the expected value equation by 500 and simplifying it to A + 6B = 525, and knowing that A + B must equal 150 (since the total rolls sum to 500).

  • What were the values of A and B that Jamie found for the missing absolute frequencies?

    -Jamie found that A equals 75 and B equals 75, which means she rolled a 1 and a 6 each 75 times out of 500.

  • What method did Jamie use to solve for the two unknowns (A and B) in her equation?

    -Jamie used a system of two equations with two unknowns, solving them by substitution and simplification to find that A and B both equal 75.

Outlines
00:00
🎲 Jamie's Dice Experiment and Expected Value Calculation

In this paragraph, Jamie's dad gifted her a die for her birthday, and she conducted an experiment to test its fairness. She rolled the die 500 times, recording the frequency of each number's appearance. From this data, she calculated the expected value of the sum of 20 rolls to be 67.4. Unfortunately, two values were washed away due to rain. The paragraph then explains how to find the missing frequencies by using the expected value of a single roll, which is 3.37 (67.4 divided by 20). The process involves calculating the weighted sum of the frequencies multiplied by their respective numbers, setting it equal to 3.37 times 500, and then simplifying the equation to find the missing values.

05:01
🔍 Solving for the Missing Frequencies in Jamie's Dice Data

This paragraph continues the analysis of Jamie's dice experiment. The speaker sets up an equation based on the expected value of a single roll (3.37) and multiplies it by 500 to find a total sum. By subtracting the known frequencies and their respective numbers from this total, the speaker isolates the terms for the missing frequencies (A for the number 1 and B for the number 6). The equation A + 6B equals 525 is derived. Additionally, knowing that the sum of all frequencies must equal 500, the speaker deduces that A + B must equal 150. Solving this system of equations, the speaker finds that B equals 75 (since 5B equals 375 when simplified) and subsequently determines that A also equals 75, as A + B equals 150. The paragraph concludes with the discovery that Jamie rolled a 1 and a 6 each 75 times out of 500.

Mindmap
Keywords
💡Expected Value
The expected value is a fundamental concept in probability theory and statistics, representing the average outcome of an experiment if it were repeated a large number of times. In the video, Jamie calculates the expected value of the sum of 20 dice rolls to be 67.4, which indicates the average result of a single roll is 3.37. This concept is central to understanding the fairness of the die and is used to solve for the missing frequencies.
💡Die
A die is a small cube, typically with six faces, each marked with a different number of dots ranging from one to six. In the script, Jamie's dad gives her a die for her birthday, and she conducts an experiment by rolling it 500 times to test its fairness. The die is the central object of the video's narrative, and its properties are essential to the problem-solving process.
💡Frequency Table
A frequency table is a statistical tool used to organize data into categories and count how often each category occurs. In the video, Jamie uses a frequency table to record the number of times each number on the die appears after 500 rolls. The table is crucial for calculating the expected value and for identifying the missing data after the rain washes away some information.
💡Absolute Frequencies
Absolute frequency refers to the actual count of how many times an event occurs. In the context of the video, Jamie's absolute frequencies for the numbers 2, 3, 4, and 5 are given, but the frequencies for the numbers 1 and 6 are missing due to the rain. The goal is to determine these missing absolute frequencies using the expected value and the total number of rolls.
💡Weighted Frequency
Weighted frequency is the product of the frequency of an event and its corresponding value. In the script, the expected value calculation involves taking the weighted frequency of each die face (e.g., the number of times a 2 appears multiplied by the value 2). This concept is used to find the sum of the weighted frequencies, which equals the expected value of a roll.
💡Roll
To roll the die means to throw or toss it, allowing it to land and display one of its faces. In the video, Jamie rolls the die 500 times to gather data for her experiment. The act of rolling is a fundamental part of the experiment and is necessary to generate the data that leads to the discovery of the missing frequencies.
💡Fairness
Fairness, in the context of a die, refers to the equal probability of each face landing face up. Jamie wants to ensure her die is fair, which is why she conducts the experiment. The fairness is tested by comparing the actual frequencies of each number rolled to the expected frequencies if the die were fair.
💡Calculation
Calculation in this video refers to the mathematical process Jamie uses to determine the expected value and to solve for the missing frequencies. The calculations involve arithmetic operations and algebraic manipulation to find the values of A (the number of times a 1 was rolled) and B (the number of times a 6 was rolled).
💡System of Equations
A system of equations is a set of two or more equations that are solved simultaneously. In the script, Jamie uses a system of equations to find the values of A and B. One equation comes from the total sum of frequencies equaling 500, and the other from the sum of weighted frequencies equaling 1685 (500 times the expected value of 3.37).
💡Algebraic Manipulation
Algebraic manipulation involves changing the form of an equation without changing its solutions, often to make it easier to solve. In the video, Jamie uses algebraic manipulation to combine the equations and isolate the variables A and B, ultimately solving for the missing frequencies.
Highlights

Jamie's dad gave her a die for her birthday, and she wanted to test its fairness.

Jamie rolled the die 500 times to gather data on each number's frequency.

She calculated the expected value of the sum of 20 rolls to be 67.4.

Two values from Jamie's data table were washed away due to rain.

The task is to find the two missing absolute frequencies from Jamie's data table.

Jamie recorded the frequency of rolling a 2, 3, 4, and 5.

The expected value of one roll is calculated by dividing the expected sum of 20 rolls by 20.

The expected value of one roll is found to be 3.37.

The expected value is calculated using a weighted sum of the frequencies and numbers.

A system of equations is set up to solve for the missing frequencies A and B.

Multiplying both sides of the equation by 500 simplifies the calculations.

The sum of all frequencies must equal 500, leading to the equation A + B = 150.

By subtracting the equations, 5B is found to equal 375, giving B a value of 75.

With B determined, A is also found to be 75 by using the equation A + B = 150.

The problem-solving process encourages viewers to pause and think before the solution is given.

The video demonstrates the application of expected value in a practical scenario.

The solution involves a step-by-step approach to solving a system of linear equations.

The final answer reveals that both missing frequencies A and B are 75.

Transcripts

Browse More Related Video

Calculating Expected values and Chi Squared Values

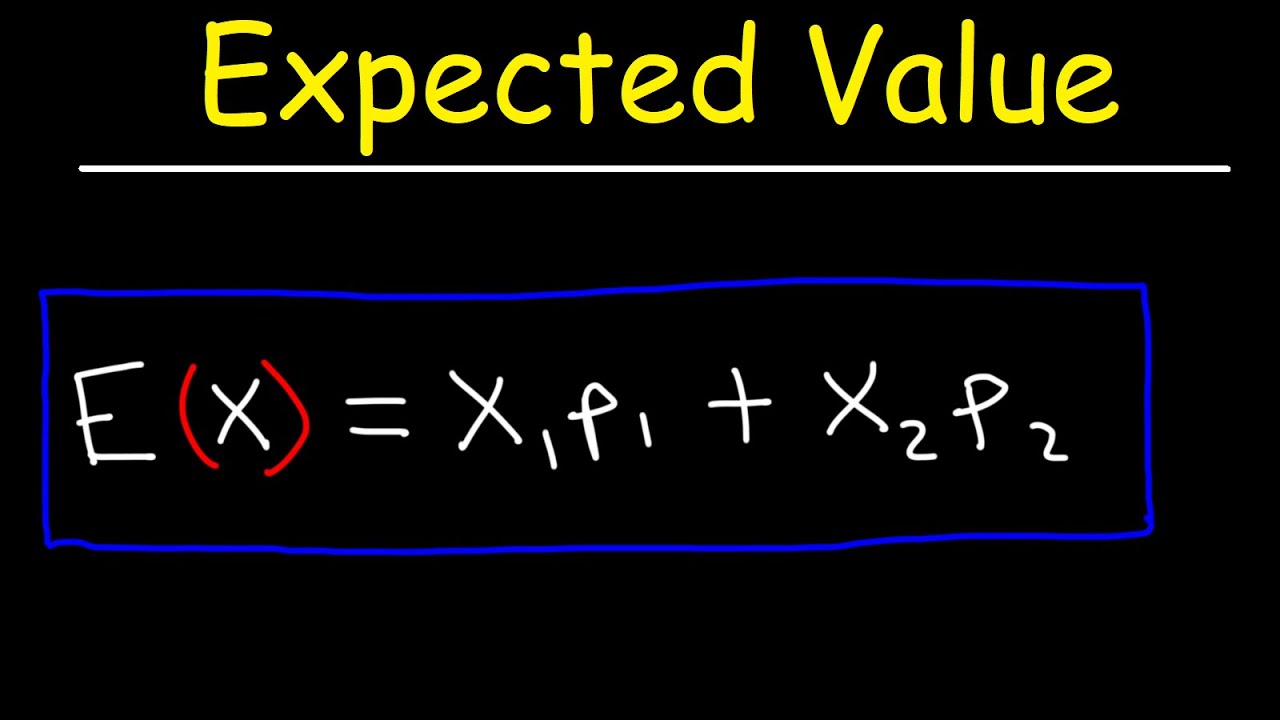
How To Calculate Expected Value

Expected Value: E(X)

5.1.6 Discrete Probability Distributions - Expected Value and Decision Theory

Mean (expected value) of a discrete random variable | AP Statistics | Khan Academy

Probability density functions | Probability and Statistics | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ProbabilityMath PuzzleExpected ValueData AnalysisEducationalCritical ThinkingProblem SolvingDice GameStatisticsSTEM