Calculus: What Is It?
TLDRThe video from New Planet School explores the fundamental concepts of calculus without delving into complex details. It explains calculus as a mathematical tool essential for understanding change, using limits to analyze slopes, areas, and volumes. The script illustrates how calculus builds upon algebra, trigonometry, and geometry, highlighting its practical applications in various fields like engineering, physics, and finance. It introduces key calculus concepts such as derivatives and integrals, and their significance in optimization and modeling real-world phenomena, including the historical context of calculus' invention by Sir Isaac Newton.
Takeaways
- 🏠 Calculus is part of the broader field of mathematics, building upon foundational areas such as algebra, trigonometry, and geometry.
- 🔍 Calculus is essential for understanding rates of change and the behavior of things that are constantly changing, like in real-world applications involving curves and motion.
- 📈 The concept of a limit is fundamental to calculus, allowing mathematicians to understand the behavior of functions and sequences as they approach certain values.
- 📚 Differential and integral calculus are two main branches of calculus; differential calculus focuses on finding slopes (derivatives), while integral calculus deals with areas and volumes (integrals).
- 📉 A derivative in calculus represents the slope of a curve at a particular point, which can be used to determine rates of change, such as speed or acceleration.
- 🎛️ Integrals are used to calculate the area under a curve, which can help in various practical applications like determining the amount of material needed for a project.
- 🔧 Calculus is used in optimization problems to find the maximum or minimum values of functions, which can be applied in fields like economics, engineering, and decision-making.
- 🌐 Differential equations, which involve derivatives, are used to model real-world phenomena and are central to physics and engineering for describing the motion of objects.
- 🛠️ Calculus was invented to handle change and understand complex shapes, which is evident in its application in various fields such as medicine, architecture, and finance.
- 📊 The notation used in calculus, while it may seem complicated at first, is a precise language that allows for the systematic solving of calculus problems, often by looking up values in tables.
- 🌟 Calculus is not just a theoretical subject; it is a practical tool that is used everywhere in the world to innovate, improve designs, and solve complex problems.
Q & A
What is the main concept introduced in calculus to handle change?
-The main concept introduced in calculus to handle change is the concept of limits.
Why is calculus considered necessary in understanding the real world?
-Calculus is necessary because everything around us is composed of curves and changing phenomena, and calculus provides the tools to understand and work with these concepts in fields like medicine, architecture, engineering, entertainment, and finance.
What does the transcript suggest as the first step in motivating the need for calculus?
-The first step suggested is to consider simple things like the slope and length of a line, and then to think about how these concepts become more complex when dealing with curves, which is where calculus becomes essential.
How does the video script connect the concept of limits to real-world applications?
-The script connects the concept of limits to real-world applications by demonstrating how calculus, through limits, can be used to find the area under a curve, which can represent things like income over time, and to understand the properties of objects with unusual shapes.
What mathematical concept does the transcript use to explain the concept of a limit?
-The transcript uses the concept of a sequence to explain the concept of a limit, showing how a sequence of numbers can approach a limiting value.
How does the script illustrate the process of taking a limit?
-The script illustrates the process of taking a limit by showing a sequence of numbers approaching a limiting value, and by demonstrating the calculation of the circumference and area of a circle using trigonometry and limits.
What are the two main branches of calculus mentioned in the transcript?
-The two main branches of calculus mentioned are differential calculus, which deals with slopes and rates of change, and integral calculus, which deals with areas and volumes.
How does the script explain the relationship between derivatives and integrals?
-The script explains that derivatives and integrals are related as concepts of rates of change and accumulation, respectively. It also mentions that integrals are the inverse operations of derivatives.
What is the significance of the sine function and its derivative in the context of the script?
-The sine function and its derivative, cosine, are significant in the script as they illustrate the concept of a derivative representing the slope of a curve. The script shows that the derivative of sine is cosine, which represents the slope of the sine curve at any point.
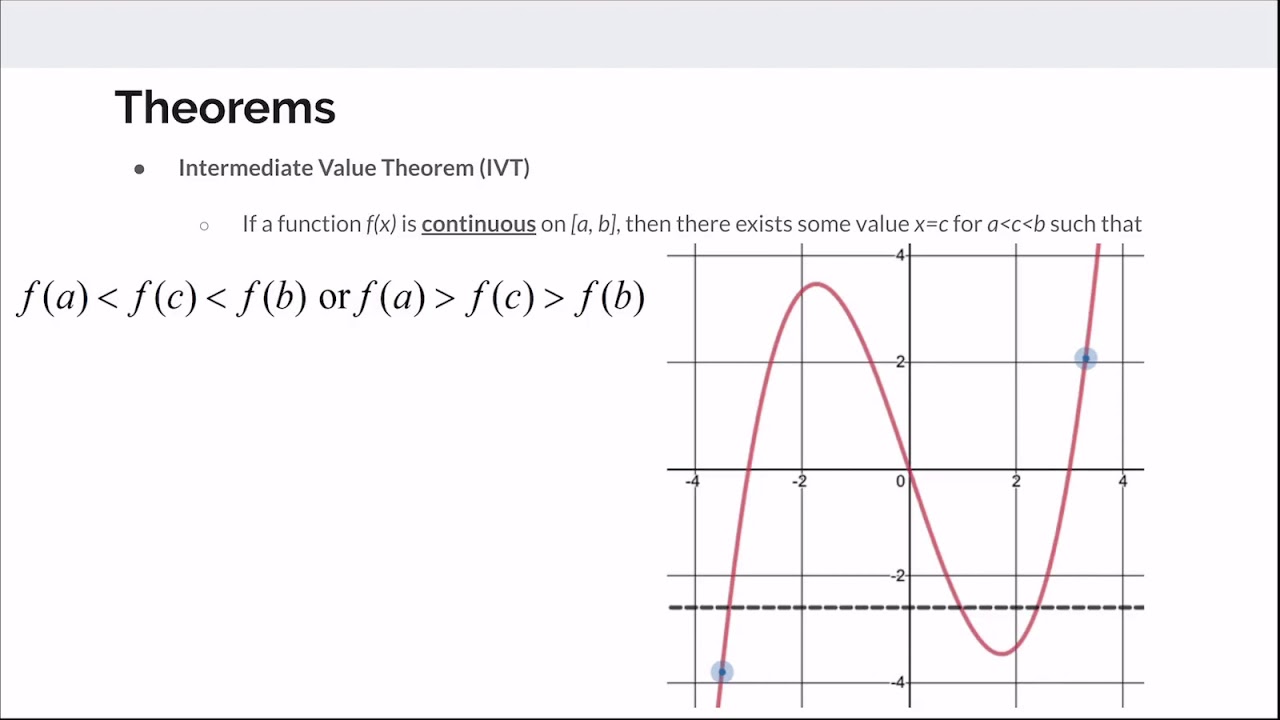
How does the script use the concept of a limit to derive the area of a circle?
-The script uses the concept of a limit to derive the area of a circle by approximating the circle with polygons (like triangles and squares), and then taking the limit as the number of sides of the polygon approaches infinity, which yields the area as pi times the radius squared.
Outlines
📚 Introduction to Calculus and Its Relevance
The video begins by introducing the topic of calculus and its significance in the broader field of mathematics. It emphasizes that calculus is part of a mathematical hierarchy, building upon algebra, trigonometry, and geometry. The instructor uses the analogy of a house to illustrate how each branch of math supports the others. The video also highlights common mistakes made by students, often due to misunderstandings in the foundational areas of math rather than calculus itself. It sets the stage for a deeper dive into calculus by discussing its necessity and utility, particularly in understanding rates of change and accumulation, which are central to the subject.
📐 Understanding the Basics: Lines, Slopes, and Areas
This paragraph delves into the concepts of lines, slopes, and areas, using a visual example of a line with a constant slope. The presenter explains how to calculate the slope and the length of a line, as well as the area under the line, by employing algebra and trigonometry. The discussion then shifts to the complexities introduced by curves, where traditional methods of calculating slope, length, and area become inapplicable. This sets up the motivation for calculus, which is designed to handle these more complex geometrical forms and their associated rates of change.
🔍 The Concept of Limits and Sequences in Calculus
The script introduces the concept of limits, which is fundamental to calculus. It begins by discussing sequences and their limits, using the example of a sequence that approaches a limiting value. The presenter explains how to graph sequences and interpret their behavior as they approach infinity. The concept of a limit is then connected to practical applications, such as determining the area under a curve, which is a common problem in calculus. The explanation provides a foundation for understanding how calculus deals with change and accumulation over infinitesimally small intervals.
📉 The Practical Application of Calculus: Estimating Circle Dimensions
This section explores the practical application of calculus concepts to estimate the dimensions of a circle, specifically its area and circumference. The presenter uses the idea of approximating a circle with polygons, such as squares and rectangles, to demonstrate how calculus can be used to derive these measurements. The process involves taking limits to refine the approximations, leading to the well-known formulas for the area and circumference of a circle. This example illustrates the power of calculus in solving geometric problems that are not easily solvable with traditional geometry.
📚 General Rules and Systematic Approaches in Calculus
The script discusses the need for general rules and systematic approaches in calculus to handle complex problems efficiently. It provides an example of calculating the interior angles of polygons and how these angles change as the number of sides increases. The presenter shows how the concept of limits can be used to find the circumference of a circle by approximating it with polygons and taking the limit as the number of sides approaches infinity. This paragraph emphasizes the importance of creating general formulas and procedures in calculus to solve a wide range of problems.
🔄 Using Trigonometry and Limits to Derive Circle Properties
This paragraph focuses on the process of using trigonometry and the concept of limits to derive the properties of a circle, specifically its circumference and area. The presenter explains how to calculate the side length of a polygon inscribed in a circle and how to use the limit as the number of sides approaches infinity to find the circumference. The process involves trigonometric identities and algebraic manipulation, culminating in the derivation of the formula for the circumference (2πr) and area (πr²) of a circle.
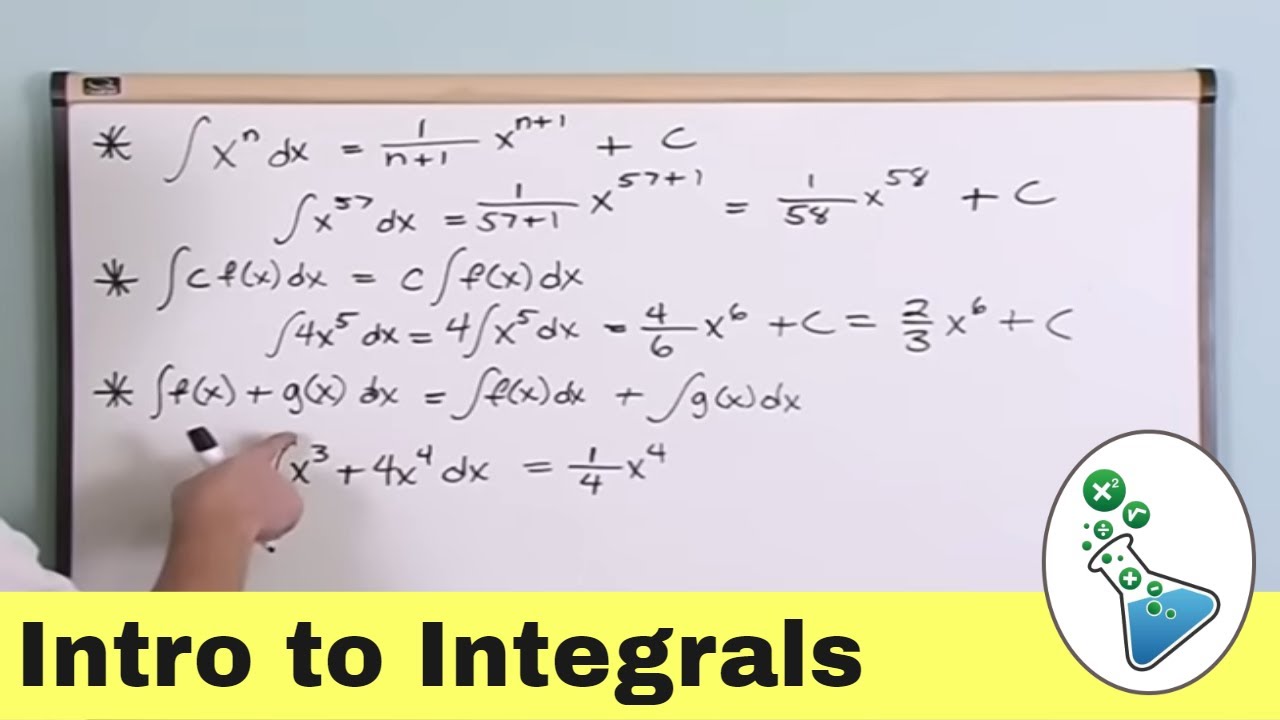
📈 Derivatives and Integrals: The Branches of Calculus
The script introduces the two main branches of calculus: differential calculus and integral calculus. Differential calculus is concerned with finding the slopes of curves, represented by derivatives, while integral calculus deals with finding areas under curves, represented by integrals. The presenter explains that these concepts are derived from the fundamental idea of limits and are essential for understanding how things change. The paragraph also touches on the notation used in calculus, such as the symbols for derivatives and integrals, and how these concepts are applied in various fields.
📘 Calculus Notation and Its Practical Significance
This section provides an overview of the notation used in calculus, emphasizing its precision and utility in various applications. The presenter explains the symbols for derivatives and integrals and how they are used to represent complex mathematical operations. The script also discusses the importance of understanding these symbols and their meanings, as they are fundamental to performing calculus operations. Examples are given to illustrate how derivatives can be used to find the slope of a function, and how integrals can be used to find the area under a curve, both of which have practical applications in fields like engineering and physics.
🚀 Applications of Calculus in Real-World Problems
The script concludes by highlighting the real-world applications of calculus, emphasizing its role in solving problems related to change and motion. Examples include optimizing functions to improve decision-making in various fields, such as economics and engineering, and using differential equations to model physical phenomena, such as the motion of a spring or a vehicle. The presenter also mentions the historical development of calculus by Sir Isaac Newton, who invented it to solve problems related to motion and gravity. The paragraph reinforces the idea that calculus is a fundamental tool for understanding and improving the world around us.
📝 Summary and Quiz on Calculus Concepts
In the final paragraph, the script provides a summary of the key concepts covered in the video, including the importance of limits, derivatives, and integrals in calculus. It also introduces the idea of approximating unknown quantities using known quantities, which is a central theme in calculus. The presenter encourages viewers to take a quiz to test their understanding of these concepts, suggesting that a solid grasp of these fundamentals will make learning calculus much easier. The paragraph serves as a recap and a call to action for viewers to engage with the material and ensure they have a firm understanding of the basics before proceeding.
Mindmap
Keywords
💡Calculus
💡Limits
💡Derivatives
💡Integrals
💡Trigonometry
💡Geometry
💡Algebra
💡Optimization
💡Differential Equations
💡Sequence
💡Tangent Line
Highlights
Calculus is introduced as a branch of mathematics that deals with the big picture of change and motion.
Mathematics is compared to a house that is built from foundational concepts like algebra, trigonometry, and geometry, with calculus building on top.
The importance of understanding the foundational math concepts when learning calculus is emphasized, as many calculus problems involve mistakes in these areas.
Calculus is needed to understand concepts like the slope of a curve, which cannot be defined in the same way as a straight line's slope.
The concept of limits is introduced as fundamental to calculus, necessary for understanding rates of change and motion.
Sequences are used to illustrate the concept of limits, showing how values approach a certain number as they increase indefinitely.
The function sine X over X is used to demonstrate how limits can be used to find values that would otherwise be indeterminate.
Calculus was invented to handle change and introduces the concept of limits, which are essential for understanding the real world composed of curves.
The area and circumference of a circle are derived using the concept of limits, showcasing practical applications of calculus.
Differentiation and integration, two main branches of calculus, are explained as dealing with rates of change and accumulation, respectively.
Derivatives and integrals are introduced as specific types of calculus operations, with derivatives relating to slopes and integrals to areas.
The notation and formulas for derivatives and integrals are explained, showing how they are used to solve calculus problems.
Optimization is discussed as a practical application of calculus, used to find maximum or minimum values in various fields such as engineering and finance.
Differential equations are introduced as a way to model real-world phenomena, with historical context provided about their development.
Calculus is highlighted as a tool for understanding and improving the world through optimization, engineering, and scientific discovery.
The transcript concludes with a review of the fundamental concepts of calculus, emphasizing its ubiquity and importance in describing change.
A quiz is suggested at the end to ensure understanding of the material, promoting active learning and engagement with the concepts.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: