The ENTIRE Calculus 3!

KoothBrush
20 Oct 202308:03
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers an insightful overview of multivariable calculus, covering key concepts such as multivariable functions, contour maps, partial derivatives, directional derivatives, double and triple integrals, the Jacobian, vector fields, and line integrals. It explains how these tools are used to analyze surfaces in 3D space, calculate volumes, and understand fields like electromagnetic and fluid dynamics, emphasizing their practical applications in various scientific fields.

Takeaways
  • 📈 Multivariable functions, such as 3D functions, take multiple inputs (like x and y) and output a single value (like z), creating surfaces in space rather than 2D graphs.
  • 🗺 Contour maps provide a top-down view of surfaces, showing the z-values at specific points and helping to visualize the steepness of slopes between adjacent contours.
  • 🅿️ Partial derivatives are used to differentiate multivariable functions, allowing for the application of standard differentiation rules by differentiating with respect to one variable while holding the others constant.
  • 🔄 Higher-order derivatives in multivariable functions include mixed partial derivatives, which are differentiated with respect to different variables.
  • 🧭 Directional derivatives capture the rate of change of a function at a specific point in a given direction, encapsulating the concept of slope in 3D space.
  • 🌐 Double and triple integrals are used to calculate volumes under surfaces and integrate over three-dimensional regions, with the order of integration being interchangeable.
  • 🔄 The Jacobian is a matrix of partial derivatives that is crucial for changing variables in a function, such as converting from Cartesian to polar coordinates.
  • 📊 Vector fields assign a vector to each point in space, visualizing the field with arrows representing magnitude and direction, and have properties like divergence and curl.
  • 🛤️ Line integrals involve integrating a function along a curve, summing the contributions over the path, and are essential for problems involving fields like electric and magnetic fields or fluid flow.
  • 🌡️ An example of a line integral's application is determining the average temperature along a curved metal rod by dividing the integral by the rod's length.
Q & A

  • What is the primary difference between functions in 2D and multivariable functions in 3D?

    -Functions in 2D take a single input (X) and output a value, whereas multivariable functions in 3D take multiple inputs (X and Y) and output a Z value, creating a surface in space rather than a 2D graph.

  • How are contour maps used to visualize multivariable functions?

    -Contour maps provide a top-down view of a 3D surface, with lines connecting points of equal Z value (height). The closeness of these contours indicates the steepness of the slope of the function at those points.

  • What is the significance of partial derivatives in multivariable calculus?

    -Partial derivatives allow for the differentiation of multivariable functions by considering one variable at a time while holding the others constant, enabling the application of differentiation rules learned in single-variable calculus.

  • How do directional derivatives relate to the slope of a function?

    -Directional derivatives capture the rate of change of a function at a specific point in a given direction. The slope of the function varies depending on the direction chosen at that point.

  • What is the role of double and triple integrals in calculus?

    -Double integrals are used to calculate volumes under a surface, while triple integrals help integrate over a three-dimensional region. The order of integration in these processes is interchangeable and does not affect the result.

  • What is the Jacobian in the context of changing variables?

    -The Jacobian is a matrix composed of partial derivatives that serves as a scaling factor when changing variables in a function, such as from Cartesian to polar coordinates.

  • How do vector fields represent physical quantities?

    -Vector fields assign a vector with both magnitude and direction to each point in space, visually represented by arrows, to depict physical quantities like gravitational or electric fields.

  • What are the two main properties of vector fields?

    -The two main properties of vector fields are divergence, which measures how much the field spreads out from a point, and curl, which measures the rotation around a point.

  • How can line integrals be applied in practical scenarios?

    -Line integrals can be used to calculate the accumulation of scalar quantities along a path, such as the total change in temperature along a curved metal rod or the work done by a force in physics.

  • What is the formula for a line integral of a scalar function along a curve?

    -The line integral of a scalar function f(X, Y, Z) along a curve C is given by the integral of f(X, Y, Z) dS, where dS is the differential arc length along the curve.

  • How can the average temperature along a curved metal rod be calculated?

    -By dividing the line integral of the temperature distribution along the rod by the length of the rod, one can find the average temperature of the rod.

Outlines
00:00
📚 Introduction to Multivariable Calculus and Surfaces

This paragraph introduces the fundamental concepts of multivariable calculus, specifically focusing on 2D and 3D functions. It explains how multivariable functions operate on multiple inputs to produce a single output value, and how these functions can be visualized in 3D space as surfaces rather than 2D graphs. The concept of contour maps as a way to represent the height (z-value) of a function at specific points is introduced, and the steepness of slopes between contour lines is discussed. The paragraph also touches on the application of calculus in fields like topography, with the example of a contour map of Mount Everest.

05:00
📈 Derivatives and Integrals in Multivariable Calculus

This paragraph delves into the specifics of differentiation and integration in the context of multivariable calculus. It begins by explaining the concept of partial derivatives, which are necessary for differentiating functions with multiple variables. The process of differentiating with respect to one variable while keeping others constant is described, along with the concept of mixed partial derivatives. The paragraph then moves on to discuss directional derivatives, which capture the rate of change of a function in a specific direction, and their importance in understanding the slope of 3D surfaces. The discussion of double and triple integrals follows, highlighting their use in calculating volumes under surfaces and their interchangeability of order. The paragraph concludes with an introduction to the Jacobian, a matrix of partial derivatives that aids in changing variables for integration, using the example of converting a double integral from Cartesian to polar coordinates.

Mindmap
Keywords
💡Multivariable Functions
Multivariable functions are mathematical functions that take more than one input variable and produce one output value. In the context of the video, these functions operate in a 3D space where an input of X and Y values results in a Z value, creating a surface in space rather than a 2D graph. An example from the script is the function that takes X and Y as its inputs and outputs a Z value, mapping all these values to form a shape in 3D space.
💡Contour Maps
Contour maps are graphical representations used to depict the value of a function at various points in its domain. In the video, contour maps are described as top views of a 3D surface, with each contour line connecting points of equal Z value. The steepness of the slope between contour lines indicates the rate of change or gradient of the function at that location. The script provides an example of a contour map of Mount Everest, illustrating how such maps can represent the elevation changes of a geographical area.
💡Partial Derivatives
Partial derivatives are a method of differentiating multivariable functions by considering one variable at a time while keeping the other variables constant. This technique allows for the application of standard differentiation rules to functions with multiple variables. In the video, partial derivatives are used to find the rate of change of a function with respect to one variable, such as differentiating with respect to X while considering Y as constant, or vice versa.
💡Directional Derivatives
Directional derivatives measure the rate of change of a function in a specific direction at a particular point. Unlike regular derivatives, which measure the rate of change in all directions, directional derivatives focus on a fixed direction. In the video, it is mentioned that the slope of a function can vary depending on the direction chosen at a given point, highlighting the concept of directional derivatives as the rate of change with a fixed direction.
💡Double and Triple Integrals
Double and triple integrals are mathematical tools used to calculate the volume under a surface or within a three-dimensional region, respectively. Double integrals are evaluated by first integrating with respect to one variable over a certain range, and then integrating with respect to another variable over its range. Triple integrals follow a similar process but integrate over three variables. The order of integration can be interchanged without changing the result. The video mentions that these integrals have significant applications, such as calculating enclosed current in electromagnetic physics.
💡Jacobian
The Jacobian is a determinant of a matrix composed of partial derivatives that is used to change the variables of a function. It acts as a scaling factor when transforming integrals from one coordinate system to another. In the video, the Jacobian is introduced as a crucial tool for converting double integrals in Cartesian coordinates to polar coordinates, simplifying complex area calculations, such as finding the area of a disk.
💡Vector Fields
Vector fields are mathematical objects that assign a vector to every point in space, where each vector has a specific magnitude and direction. These fields can be visualized as a collection of arrows, each representing a vector at a point. Vector fields are essential in physics for mapping various fields like gravitational, electric, and magnetic fields. The video describes vector fields as having properties such as divergence, which measures how much a field spreads out from a point, and curl, which measures the rotation about a point.
💡Line Integrals
Line integrals involve integrating a function along a curve or path. They are used to calculate the accumulated effect of a scalar field, such as temperature or elevation, along a specific path. The line integral for a scalar function f(X, Y, Z) along a curve C is given by an integral involving the differential arc length along the curve. In the video, line integrals are described as adding up all the elevations along a hilly path, and in the context of temperature distribution along a curved metal rod, the line integral divided by the length of the rod would yield the average temperature.
💡Divergence and Curl
Divergence and curl are operators used in vector calculus to analyze the behavior of vector fields. Divergence measures the rate at which a vector field spreads out or diverges from a given point, indicating the magnitude of source or sink at that point. Curl, on the other hand, measures the rotation or swirling effect of the vector field around a point. Both divergence and curl are essential in understanding physical phenomena such as fluid flow and electromagnetic fields. In the video, these concepts are introduced as properties of vector fields, with divergence indicating how much a field spreads out from a point and curl indicating the rotation about the point.
Highlights

Introduction to multivariable functions in 2D and 3D, which take multiple inputs and output a value, creating surfaces in space.

Explanation of how contour maps represent surfaces and their corresponding height (z value) at specific x, y points.

Use of contour maps in fields like topography, with an example of a contour map of Mount Everest.

Partial derivatives as a method to differentiate multivariable functions by differentiating with respect to one variable while holding others constant.

Clarification on higher-order derivatives and mixed partial derivatives as key skills in calculus 3.

Directional derivatives, which capture the rate of change of a function at a point with a fixed direction.

Description of double and triple integrals, their use in obtaining volumes under surfaces, and the interchangeability of their order of integration.

Application of double integrals in electromagnetic physics, such as calculating enclosed current in Ampere's law.

Introduction to the Jacobian, its role as a matrix of partial derivatives, and its use in changing variables of functions.

Example of calculating the area of a disk in Cartesian versus polar coordinates, demonstrating the utility of the Jacobian.

Explanation of vector fields as assignments of vectors to each point in space, visualized as a set of arrows with specific magnitude and direction.

Properties of vector fields, including divergence, which measures how much a field spreads out from a point, and curl, which measures rotation.

Practical applications of vector fields, such as mapping gravitational fields in specific regions.

Line integrals, their concept, and application in integrating a function along a curve, with an example of temperature distribution along a metal rod.

Formula for line integrals and their importance in problems involving fields like electric and magnetic fields or fluid flow.

The transcript's summary emphasizes the complexity and depth of calculus 3, suggesting that a comprehensive understanding requires more than a brief overview.

Transcripts

Browse More Related Video

ALL of calculus 3 in 8 minutes.

Partial derivatives of vector fields, component by component

Conservative Vector Fields & Potential Functions

The 4 Maxwell Equations. Get the Deepest Intuition!

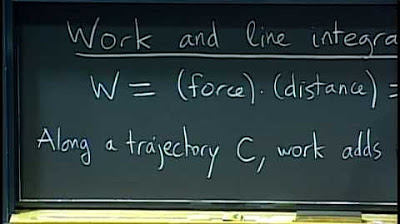
Lec 19: Vector fields and line integrals in the plane | MIT 18.02 Multivariable Calculus, Fall 2007

Vector Fields (Introduction)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Multivariable Calculus3D FunctionsSurfacesContour MapsPartial DerivativesDirectional DerivativesDouble IntegralsVector FieldsLine IntegralsPhysics Applications