College Physics 1: Lecture 18 - Friction and Drag
TLDRThis physics lecture delves into friction and drag, two fundamental resisting forces. It explains static and kinetic friction, detailing how they resist motion and their equations, f=μsN and f=μkN, respectively. The lecture also covers air resistance, introducing the drag equation and the concept of terminal velocity. Through examples, it illustrates calculating forces on a sofa and terminal speeds for a skydiver and a mouse, highlighting the impact of mass, area, and coefficients on these forces. The session concludes with questions reinforcing the concepts discussed.
Takeaways
- 📚 The lecture covers static and kinetic friction, as well as drag, which are forces that resist the motion of objects.
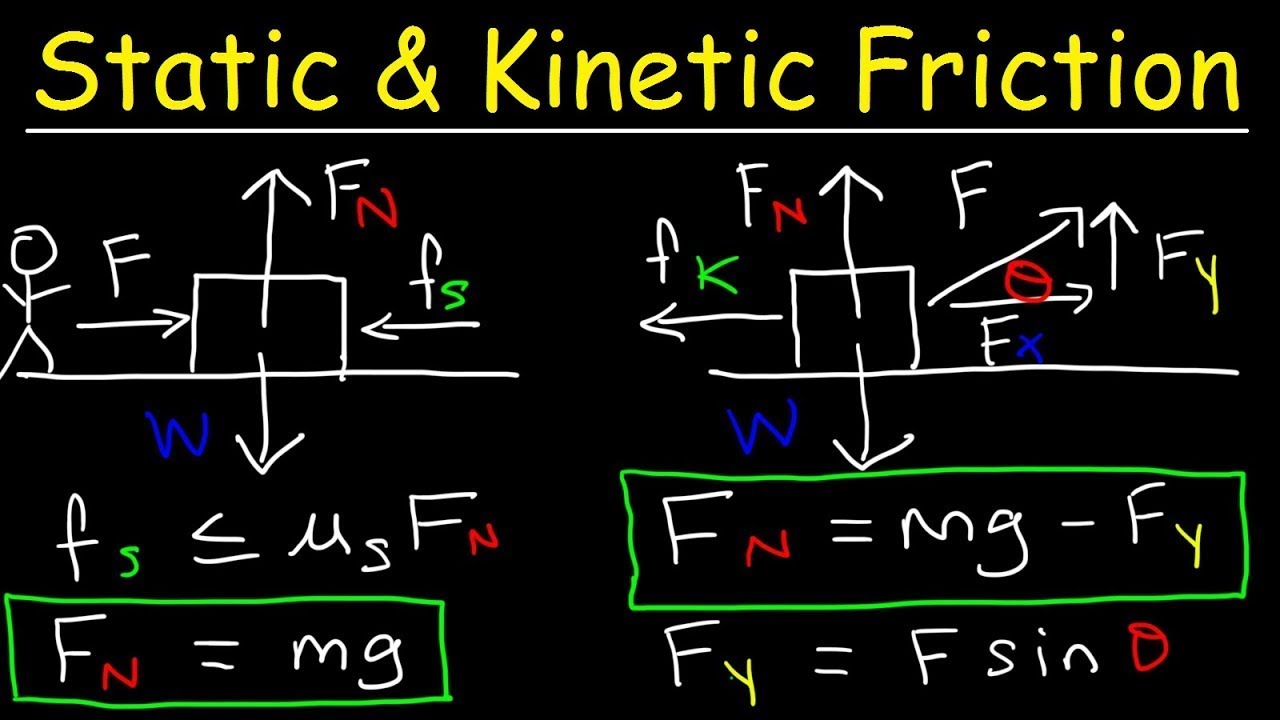
- 🧱 Static friction is the force that prevents an object from moving across a surface and is always opposite to the direction of the potential movement.
- 🔄 Static friction adjusts itself to balance the applied force until it reaches its maximum limit, which is determined by the equation f_{s_max} = μ_s × n, where μ_s is the coefficient of static friction and n is the normal force.
- 🚀 Kinetic friction, or the friction force during motion, is similar to static friction but acts in the opposite direction of the object's motion and is calculated using f = μ_k × n, with μ_k being the coefficient of kinetic friction.
- 🔍 The coefficients of friction (μ_s and μ_k) are material-dependent and can be found in tables or given in problems, affecting how 'sticky' a surface is.
- 📉 The normal force (n) is related to the weight of the object and is balanced by the force the surface exerts against the object.
- 🔌 Air resistance, or drag, opposes the motion of an object moving through a fluid like air or water and is calculated using the equation D = (1/2) C_d ρ A v^2, where C_d is the drag coefficient, ρ is the fluid density, A is the object's cross-sectional area, and v is the velocity.
- 🌟 Terminal speed is the constant speed an object reaches when the drag force equals the force pulling or pushing it, such as weight for a falling object.
- 🐁 An example problem involves calculating the force needed to move a sofa, requiring the use of multiple equations and understanding the relationship between weight, mass, normal force, and friction.
- 🪂 Another example explores terminal velocity, comparing the falling speeds of a skydiver and a pet mouse, highlighting the role of mass, area, and drag coefficient in determining terminal speed.
Q & A
What is static friction?
-Static friction is the force that a surface exerts on an object to prevent it from moving. It acts in the opposite direction of the force that would move the object if there was no friction.
How is static friction related to the normal force?
-The maximum possible magnitude of static friction force is given by the equation f_s max = μ_s * n, where μ_s is the coefficient of static friction and n is the normal force. This shows that static friction is dependent on the normal force acting on the object.
What is the role of the coefficient of static friction (μ_s)?
-The coefficient of static friction (μ_s) is a dimensionless number that depends on the materials of the surfaces in contact. It represents the 'stickiness' of the surface; a higher μ_s value indicates a harder time moving an object over the surface.
What happens when an object is pushed with a force greater than static friction can handle?
-When a force greater than the maximum static friction is applied, the object starts to move. At this point, static friction transitions to kinetic friction.
What is kinetic friction?
-Kinetic friction, or dynamic friction, is the frictional force that opposes the motion of an object that is already sliding or rolling. It is a resisting force and acts in the opposite direction to the motion of the object.
How does kinetic friction differ from static friction in terms of its equation?
-Kinetic friction has the same form of equation as static friction, which is f_k = μ_k * n, where f_k is the kinetic friction force, μ_k is the coefficient of kinetic friction, and n is the normal force.
Why is the direction of kinetic friction always opposite to the direction of motion?
-The direction of kinetic friction is opposite to the direction of motion because it is a resisting force that acts to slow down or stop the object's movement.
What is the concept of terminal speed?
-Terminal speed, also known as terminal velocity, is the constant speed that a freely falling object eventually reaches when the resistance of the medium (air or water) through which it is falling prevents further acceleration.
How does the drag force acting on a moving object depend on its speed?
-The drag force is heavily dependent on the speed of the object. It is proportional to the square of the object's speed, meaning that if the speed doubles, the drag force quadruples.
What factors influence the drag force on an object moving through a fluid?
-The drag force is influenced by the object's speed (v), the density of the fluid (ρ), the cross-sectional area of the object (a), and the drag coefficient (C_d), which depends on the shape of the object.
Why is it that small animals can survive falls from great heights?
-Small animals can survive falls from great heights because they have a lower ratio of mass to surface area (m/a), which results in a lower terminal speed. This reduced speed means less impact upon landing.
Outlines
📚 Introduction to Friction and Drag Forces
The lecture begins by introducing the topic of friction and drag, which are considered resisting forces in physics. Static friction is explained as the force that prevents an object from moving, exerted by a surface and always directed opposite to the potential movement direction. The maximum static friction force is given by the equation fs_max = μs * n, where μs is the coefficient of static friction, a dimensionless number that depends on the materials of the surfaces in contact. Kinetic friction, which acts on a moving object, is also discussed, with its force equation being fk = μk * n, similar to static friction but with μk being the coefficient of kinetic friction. The lecture emphasizes that these forces are independent of the object's velocity and highlights the importance of understanding the normal force's role in calculating friction.
🔍 Exploring Friction with a Practical Example
The lecture continues with a practical example involving Carol moving a sofa. It explains the forces at play, including friction, weight, and the normal force, and how these forces relate to static equilibrium. The problem-solving process involves using Newton's second law and the equations for friction and weight to calculate the force Carol applies to the sofa. The example illustrates the concept of normal force being equal to the weight of the object and how the friction force can be determined using the coefficient of kinetic friction (μk) and the normal force. This example serves to clarify the application of multiple physics equations in a single problem.
🌪️ Understanding Air Resistance and Drag
The third paragraph delves into air resistance, or drag, which is the resistive force experienced by an object moving through a fluid like air or water. The drag force is described by the equation D = (1/2) * Cd * ρ * A * v^2, where v is the speed, ρ is the fluid density, A is the object's cross-sectional area, and Cd is the drag coefficient, which depends on the object's shape. The lecture provides examples of Cd values for different objects and discusses how drag force increases with the square of the speed, leading to the concept of terminal velocity, where drag equals the force of gravity, and the object stops accelerating.
🪂 Calculating Terminal Velocity: Skydiver and Mouse
This section presents a problem involving a skydiver and a mouse falling from a plane to illustrate the concept of terminal velocity. The problem provides dimensions for the objects, allowing for the calculation of their cross-sectional areas. Using the drag equation and the concept that at terminal velocity, the drag force equals the gravitational force, the lecture guides through the calculation of the terminal velocity for both the skydiver and the mouse. The example demonstrates how terminal velocity depends on the mass-to-area ratio and how smaller animals can survive falls from great heights due to their lower terminal velocities.
🧐 Analyzing the Effects of Drag on Animals and Objects
The lecture explores how animals in nature utilize the concept of terminal velocity and drag to survive falls from significant heights. It explains that small animals have a lower mass-to-area ratio, resulting in a lower terminal velocity, which allows them to withstand the impact. Additionally, some animals can increase their drag by expanding their surface area, as seen when a frog jumps or a cat lands, effectively reducing their terminal speed. This section highlights the fascinating ways in which physics principles are applied in the natural world without the organisms necessarily understanding the underlying science.
📉 Terminal Speed and the Impact of Drag on Motion
The final paragraph discusses the implications of terminal speed and drag on the motion of objects, particularly in the context of a box being pulled over a rough surface. It addresses common misconceptions about what happens when an object is subjected to a pulling force and when that force is removed. The lecture emphasizes that an object will not maintain a constant speed without an external force and will slow down and eventually stop due to friction once the pulling force is removed. This section reinforces the importance of understanding the interplay between forces and motion in physics.
Mindmap
Keywords
💡Friction
💡Static Equilibrium
💡Coefficient of Static Friction (μs)
💡Normal Force
💡Kinetic Friction
💡Terminal Speed
💡Drag
💡Cross-Sectional Area
💡Drag Coefficient (Cd)
💡Fluid Density (ρ)
Highlights
Introduction to lecture 18 on friction and drag as resisting forces in physics.
Explanation of static friction (fs) as a force that prevents an object from moving.
Static friction's direction is always opposite to the direction of the applied force.
Static equilibrium concept where forces are balanced, preventing object movement.
Friction adjusts to balance the pushing force until it reaches its maximum.
Static friction force equation: fs max = μs * n, introducing coefficient of static friction.
The coefficient of static friction (μs) depends on the materials of the surfaces in contact.
Kinetic friction (fk) is the friction force when an object is in motion.
Kinetic friction's direction opposes the direction of motion.
Kinetic friction equation is similar to static friction: fk = μk * n.
Mistaken belief that velocity affects friction force, which it does not.
Microscopic explanation of how surfaces interlock and friction depends on normal force.
Example problem involving moving a sofa with kinetic friction and weight calculations.
Concept of terminal speed and its relation to drag force and weight balance.
Drag force equation components: 1/2 * Cd * ρ * A * v^2.
Terminal velocity equation derivation and its significance.
Example problem calculating terminal speed for a skydiver and a mouse.
Discussion on how animals use terminal speed and drag to their advantage.
Three questions to test understanding of friction, forces, and motion.
Conclusion and preview of the next lecture on interacting objects and connected systems.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: