High School Physics - Friction

Dan Fullerton
4 Nov 201112:16
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative talk, Mr. Fullerton discusses the concept of friction, explaining its role in opposing motion. He differentiates between static and kinetic friction, emphasizing how the coefficient of friction (μ) and the normal force (FN) determine the magnitude of frictional forces. Through practical examples, he illustrates how to calculate frictional forces and apply this knowledge to solve physics problems, highlighting the differences in friction for moving versus stationary objects and the impact of surfaces on the frictional outcome.

Takeaways
  • 📌 Friction is a force that opposes motion; kinetic friction opposes sliding motion while static friction acts on objects at rest.
  • 🔢 The magnitude of frictional force depends on the nature of the surfaces in contact and is quantified by the coefficient of friction (μ).
  • 📈 The frictional force is calculated by multiplying the coefficient of friction (μ) by the normal force (FN).
  • 🚀 Kinetic and static coefficients of friction are different, with kinetic usually being less than static, meaning less friction when an object is sliding.
  • 🛷 Examples of frictional forces include a sled sliding down a hill (kinetic friction) and a stationary refrigerator being pushed (static friction).
  • 🚗 When tires of a car skid on the road, kinetic friction is at play, but if the tires are rolling freely, static friction is considered.
  • 🔄 The coefficient of friction remains the same when the angle of inclination increases because the surfaces in contact do not change.
  • 🔧 Solving problems involving friction often requires the use of free body diagrams and Newton's Second Law to determine the forces involved.
  • 📐 Given a scenario with a 4 kg object on a rough horizontal surface, the frictional force can be calculated using the known applied force and acceleration.
  • 📌 When an object is moving at a constant velocity, the net force (and thus the forward force from the engines and the backward frictional drag force) must be zero.
  • 📝 To solve for the mass and acceleration of an object, one can use the known values of weight, frictional force, and the net force in the X direction.
Q & A

  • What is friction and how does it oppose motion?

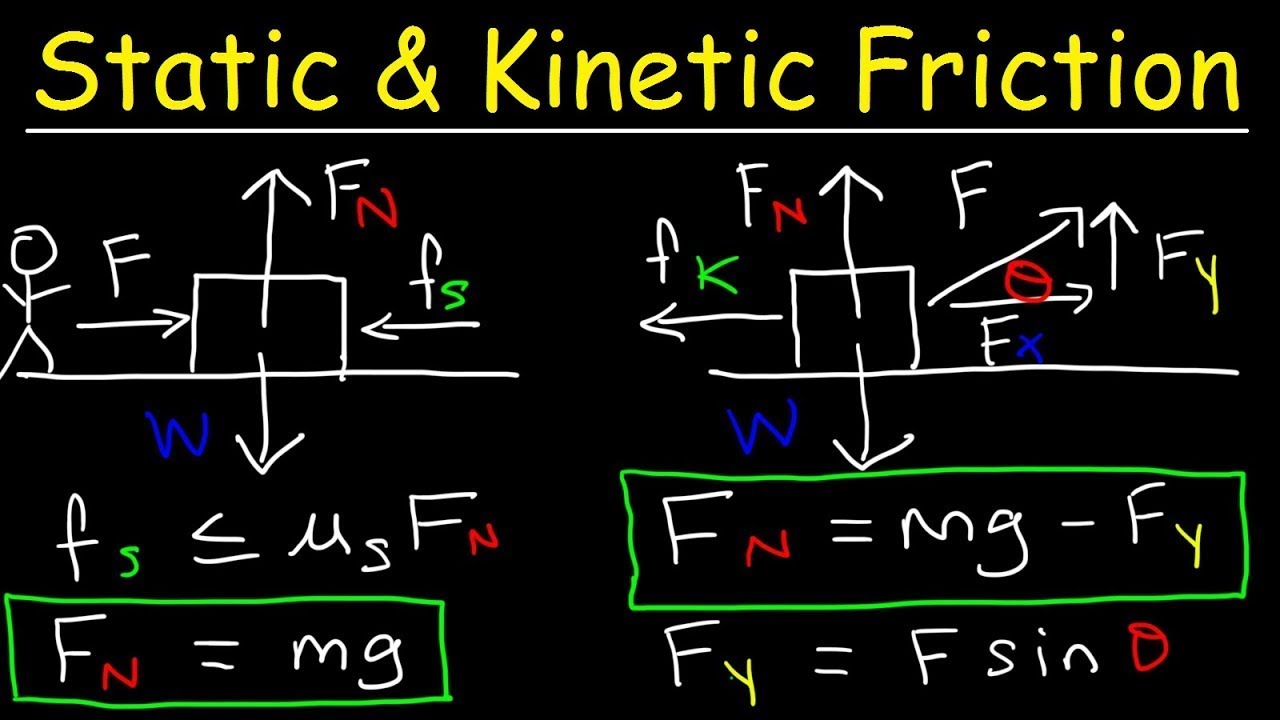
    -Friction is a force that opposes motion between two surfaces in contact. Kinetic friction opposes the motion of an object sliding along another surface, while static friction acts on an object that is not sliding.

  • What factors determine the amount of friction between two surfaces?

    -The amount of friction between two surfaces is determined by the nature of the surfaces in contact and the normal force acting on the object. The nature of the surfaces is characterized by the coefficient of friction (μ), which is an empirically determined value.

  • What is the relationship between the frictional force and the normal force?

    -The frictional force is directly proportional to the normal force acting on an object. The magnitude of the frictional force can be calculated as the product of the coefficient of friction (μ) and the normal force (FN).

  • How does the coefficient of friction differ between static and kinetic friction?

    -The coefficient of friction for static friction (μs) is usually higher than that for kinetic friction (μk). This means there is more friction preventing the start of motion compared to maintaining motion once an object is already sliding.

  • What is the significance of the coefficient of friction values for different surfaces?

    -The coefficient of friction values for different surfaces indicates the degree of friction expected between those surfaces. Higher values mean more friction, while lower values indicate less friction. These values are crucial for solving problems involving friction and determining the required forces for motion.

  • How does the angle of inclination affect the coefficient of kinetic friction?

    -The angle of inclination does not change the coefficient of kinetic friction between the surfaces in contact, as the coefficient is determined solely by the nature of the surfaces and not by the angle of contact.

  • In the scenario of a car braking on various road surfaces, which surface would offer the greatest friction?

    -Rubber tires on dry concrete offer the greatest coefficient of kinetic friction, which would result in the greatest force of friction to stop the car.

  • What is the formula to calculate the force of friction?

    -The force of friction can be calculated using the formula: Force of friction = μ * FN, where μ is the coefficient of friction and FN is the normal force.

  • How can Newton's Second Law be applied to find the frictional force on an object?

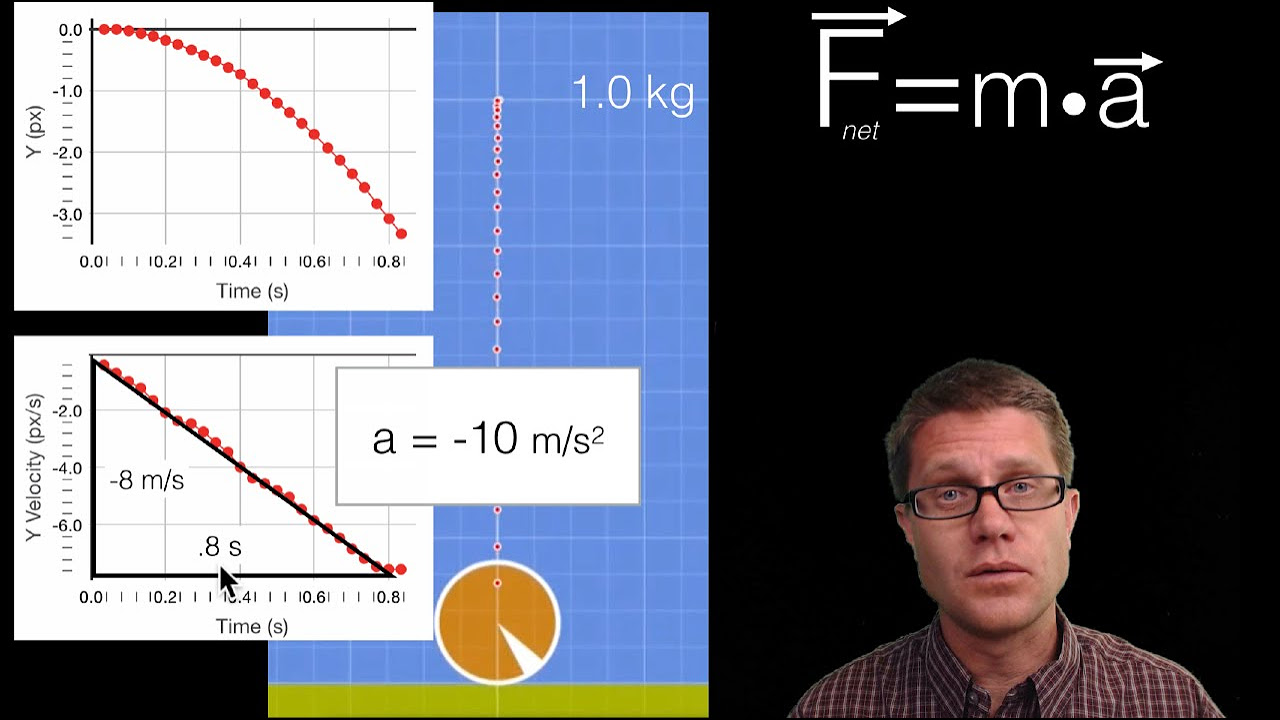
    -Newton's Second Law can be applied by setting up a free body diagram and considering the net force in the direction of motion (X direction). The frictional force can then be found by equating the net force to the product of the object's mass and its acceleration (F_net = m * a_x).

  • What happens to the force needed to maintain motion once an object is already sliding compared to starting it?

    -The force needed to maintain motion once an object is already sliding is usually less than the force required to start it moving. This is because the kinetic coefficient of friction is typically less than the static coefficient of friction.

  • In the case of an airplane moving with constant velocity, what can be said about the forces acting on it?

    -For an airplane moving with constant velocity, the net force must be zero, meaning the forward force provided by the engines is equal in magnitude to the backward frictional drag force.

Outlines
00:00
📘 Introduction to Friction and its Concepts

This paragraph introduces the topic of friction, explaining it as a force that opposes motion. It distinguishes between kinetic friction, which opposes motion for an object sliding along another surface, and static friction, which acts on an object that isn't sliding. The paragraph emphasizes the importance of understanding the factors that determine the amount of friction, such as the nature of the surfaces in contact and the normal force. It introduces the coefficient of friction (μ), an empirically determined value that characterizes the amount of friction between different types of surfaces. The paragraph also explains how the coefficient of friction can be calculated and how it varies between static and kinetic scenarios, providing examples to illustrate these concepts.

05:01
📊 Mathematical Representation and Problem Solving with Friction

This paragraph delves into the mathematical representation of frictional forces, explaining how the force of friction depends on the nature of the surfaces in contact and the normal force. It presents the formula for calculating frictional force as the product of the coefficient of friction (μ) and the normal force (FN). The paragraph then demonstrates how this understanding can be applied to solve more complex physics problems, using Newton's Second Law and free body diagrams. It provides examples of calculating frictional forces for different scenarios, such as an object accelerating on a rough horizontal surface and a block sliding down an inclined plane, highlighting the application of the frictional force formula and the importance of understanding the direction of forces.

10:06
🚀 Real-world Applications and Comparisons of Frictional Forces

The final paragraph discusses the real-world implications and applications of frictional forces. It compares the force needed to initiate motion (static friction) with the force needed to maintain motion (kinetic friction), noting that less force is required to keep an object sliding than to start it moving. The paragraph presents a problem involving a crate on a rough level floor to illustrate this concept. It also discusses the scenario of an airplane in level flight, where the forward force provided by the engines is balanced by the backward frictional drag force, resulting in a net force of zero and constant velocity. The paragraph concludes by encouraging further exploration of friction through sample problems and resources, emphasizing the practical importance of understanding friction in various contexts.

Mindmap
Keywords
💡Friction
Friction is a force that opposes motion between two surfaces in contact. In the context of the video, it is explained as having both kinetic and static forms. Kinetic friction opposes the motion of an object sliding along another surface, while static friction acts on an object that isn't sliding. The magnitude of frictional force depends on the nature of the surfaces in contact and the normal force acting on the object. For example, the video mentions that walking on ice with slippery shoes results in less friction compared to walking on dirt with cleats.
💡Coefficient of Friction (μ)
The coefficient of friction (μ) is a dimensionless value that characterizes the friction between two surfaces. It is determined empirically and varies depending on the materials in contact. The video explains that there are different coefficients for kinetic and static friction, with the kinetic coefficient typically being less than the static one. This value is used to calculate the frictional force using the formula: frictional force = μ * normal force (FN).
💡Normal Force (FN)
The normal force is the force exerted by a surface that supports the weight of an object resting on it, acting perpendicular to the surface. It is a reaction force that balances the weight of the object. In the context of friction, the normal force plays a crucial role in determining the magnitude of the frictional force, as friction is directly proportional to the normal force. The video explains that the frictional force can be calculated by multiplying the coefficient of friction (μ) by the normal force (FN).
💡Kinetic Friction
Kinetic friction, also known as sliding friction, is the type of friction that occurs when an object is in motion along a surface. It is generally less than the static friction, meaning it takes less force to keep an object sliding than to initiate the motion. The kinetic coefficient of friction (μk) is used to calculate the kinetic frictional force. The video provides examples of how kinetic friction is applied in real-world scenarios, such as a sled sliding down a snowy hill.
💡Static Friction
Static friction is the frictional force that acts on an object at rest, preventing it from starting to move. It adjusts to match the applied force up to a maximum value, which is determined by the coefficient of static friction and the normal force. Once the applied force exceeds this maximum, the object begins to move, and kinetic friction takes over. The video emphasizes that static friction is typically greater than kinetic friction, requiring more force to initiate movement than to maintain it.
💡Newton's Second Law
Newton's Second Law of Motion states that the net force acting on an object is equal to the mass of the object multiplied by its acceleration (F = ma). This law is fundamental to understanding how forces affect the motion of objects and is used in the video to analyze problems involving friction. By considering the forces acting on an object, such as applied forces, frictional forces, and gravitational forces, and applying Newton's Second Law, one can determine the acceleration or the required force to achieve a certain motion.
💡Free Body Diagram
A free body diagram is a graphical representation that shows all the forces acting on an object. It is a tool used in physics to analyze and solve problems involving forces and motion. In the video, Mr. Fullerton uses free body diagrams to visualize and calculate the forces acting on objects in different scenarios involving friction. By drawing the forces as arrows and labeling them, one can apply physical laws, such as Newton's Second Law, to find unknown forces or object properties.
💡Acceleration
Acceleration is the rate of change of velocity of an object with respect to time. It is a vector quantity, meaning it has both magnitude and direction. In the context of the video, acceleration is used to analyze the motion of objects under the influence of various forces, including friction. Newton's Second Law (F = ma) is often used to calculate acceleration when the forces acting on an object are known.
💡Net Force
Net force, also known as the resultant force, is the vector sum of all the individual forces acting on an object. It determines the overall force that affects the object's motion. When all forces are balanced, meaning their vector sum is zero, the object either remains at rest or continues to move at a constant velocity. The video emphasizes the importance of net force in understanding the motion of objects, especially in relation to frictional forces.
💡Mass
Mass is a measure of the amount of matter in an object, typically measured in kilograms (kg). It is a fundamental property of an object that affects its motion when forces are applied. In the context of the video, mass is used in conjunction with Newton's Second Law to calculate the acceleration of objects under the influence of frictional forces.
💡Inclined Plane
An inclined plane is a surface that is set at an angle to the horizontal. It is one of the simple machines used to change the relationship between force and distance. In the context of the video, the inclined plane is used to discuss how the coefficient of friction might change with the angle of inclination. The video clarifies that the coefficient of friction remains the same because the surfaces in contact do not change, regardless of the angle.
💡Constant Velocity
Constant velocity refers to an object moving at a steady speed in a straight line, without any change in its magnitude or direction. In physics, when an object is moving at a constant velocity, it means that the net force acting on the object is zero, indicating a balance of all forces. The video uses the concept of constant velocity to explain that in level flight, the forward force provided by the engines is equal in magnitude to the backward frictional drag force.
Highlights

Friction is a force that opposes motion.

Kinetic friction opposes motion for an object that slides along another surface.

Static friction acts on an object that isn't sliding.

The magnitude of the frictional force is determined by the nature of the surfaces in contact and the normal force.

The coefficient of friction (μ) characterizes the amount of friction between two surfaces.

The coefficient of friction is an empirically determined value that can be looked up for different types of objects.

Kinetic and static coefficients of friction are different, with the kinetic usually being less than the static.

It takes more force to start an object in motion than to keep it in motion due to static and kinetic friction differences.

The coefficient of friction can be calculated using the formula: frictional force = μ * normal force (F = μ * FN).

Frictional force depends only upon the nature of the surfaces in contact and the normal force.

The force of friction is a key factor in solving problems involving Newton's Second Law and free body diagrams.

In the example of a car's brakes, the greatest force of friction is encountered on dry concrete due to the highest kinetic coefficient.

The coefficient of kinetic friction remains the same as the angle of inclination increases because the surfaces in contact do not change.

In the example of a 4 kg object on a rough horizontal surface, the frictional force is calculated to be 10 Newtons.

For a wooden box on a wood surface, the frictional force is determined by using the given kinetic coefficient of friction (0.3) and the normal force.

The net force acting on an object is the applied force minus the frictional force, which can be used to find the object's acceleration.

The force needed to keep an object sliding is less than the force needed to start it moving due to the difference between static and kinetic friction.

In level flight, the forward force provided by the engines is equal to the backward frictional drag force since the net force and acceleration are zero.

Transcripts

Browse More Related Video

Frictional Forces: Static and Kinetic

Friction: Crash Course Physics #6

AP Physics Workbook 7.J Translation vs Rotation

Static Friction and Kinetic Friction Physics Problems With Free Body Diagrams

Newton's Second Law

AP physics Workbook 3.C Centrigual Force Part 2

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsEducationFrictionTypesCoefficientOfFrictionKineticFrictionStaticFrictionFrictionalForceNewtonsLawProblemSolvingPhysicsConceptsEducationalContentLearnPhysics