Compound interest and e (part 3) | Exponential and logarithmic functions | Algebra II | Khan Academy

Khan Academy
22 Apr 200810:04
EducationalLearning
32 Likes 10 Comments

TLDRThis video explores the concept of compound interest, using Excel to demonstrate how it converges to the mathematical constant e (approximately 2.7183). The presenter explains the process of compounding interest over different periods, generalizes to various interest rates, and derives the formula for continuous compounding. Through examples, the video illustrates the relationship between compounding frequency and the amount owed. The discussion touches on the deeper mathematical significance of constants like e and pi, suggesting their fundamental role in the universe. Future videos will delve further into these intriguing mathematical relationships.

Takeaways
  • πŸ“ˆ The script discusses the concept of compound interest as a natural way to introduce the mathematical constant 'e'.
  • πŸ“Š The presenter demonstrates how 'e' converges using an Excel formula, showing that as the number of compounding periods increases, the result approaches 2.7183, which is the value of 'e'.
  • πŸ” Google is highlighted as a tool for quickly finding the value of 'e', as it functions as a calculator and can provide the constant to arbitrary decimal places.
  • 🧐 The script suggests that numbers like 'e', pi, and imaginary numbers appear frequently and mysteriously in various areas, hinting at a deeper, underlying connection.
  • πŸ’‘ The presenter expresses a belief that these mathematical constants are fundamental and appear 'everywhere' in the universe, indicating a profound significance.
  • πŸ“š The video aims to motivate viewers, possibly in a humorous way, suggesting starting a 'cult' to emphasize the importance of understanding these mathematical concepts.
  • πŸ’° The script generalizes the concept of compound interest beyond 100% to any percentage 'r', explaining how the interest rate is applied as a decimal.
  • πŸ“ˆ The formula for calculating the amount owed after one compounding period is presented, with 'p' representing the initial principal and 'n' the number of compounding periods.
  • πŸ€” An example is given to illustrate the calculation, using a 10% interest rate compounded semi-annually on a $50.00 loan.
  • πŸ”¬ The concept of continuous compounding is introduced, where the number of compounding periods approaches infinity, leading to the formula involving 'e' raised to the power of 'r'.
  • 🌐 The final formula for continuous compounding is presented as the original principal 'p' times 'e' to the power of 'r', showing the relationship between the interest rate and the constant 'e'.
Q & A

  • What was the original topic intended for the videos?

    -The original topic intended for the videos was about the mathematical constant e.

  • How does compound interest relate to the introduction of the mathematical constant e?

    -Compound interest is a natural way to introduce the mathematical constant e because as the number of compounding periods increases, the formula for compound interest converges to e.

  • What is the formula used in the Excel demonstration to show the convergence to e?

    -The formula used in the Excel demonstration is (1 + 1/n)^n, where n represents the number of compounding periods.

  • How does Google provide the value of e?

    -Google provides the value of e because it functions as a calculator, and users can search for e to get the numerical value.

  • What is the significance of the numbers pi and e in mathematics?

    -Pi and e are significant in mathematics because they appear in various formulas and equations across different fields, and they are considered fundamental constants that are deeply interconnected.

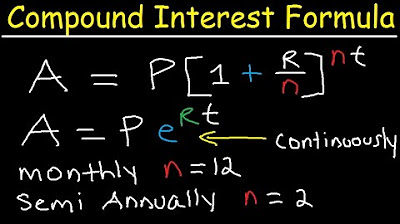
  • What is the general formula for calculating compound interest?

    -The general formula for calculating compound interest is P(1 + r/n)^(nt), where P is the principal amount, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the time the money is invested or borrowed for in years.

  • How does the interest rate affect the final amount owed in a compound interest scenario?

    -The interest rate (r) directly affects the final amount owed as it determines the growth factor of the initial principal when compounded over time.

  • What happens when the compounding period (n) approaches infinity?

    -When the compounding period (n) approaches infinity, the formula for compound interest converges to e^(rt), where e is the base of the natural logarithm, r is the annual interest rate, and t is the time in years.

  • Why is it important to understand the concept of continuous compounding in finance?

    -Continuous compounding is important in finance because it provides a more accurate representation of how interest grows over time, especially for very short periods or when dealing with very high compounding frequencies.

  • How does the video script connect the concept of compound interest to the broader mathematical concept of e?

    -The script connects compound interest to e by demonstrating that as the frequency of compounding increases (approaching infinity), the formula for compound interest simplifies to e raised to the power of the interest rate times the principal.

  • What is the practical implication of understanding continuous compounding in financial calculations?

    -Understanding continuous compounding is crucial for accurate financial calculations, as it allows for precise determination of the future value of investments or loans when interest is compounded an infinite number of times per year.

Outlines
00:00
πŸ“ˆ Introduction to Compound Interest and e

The video script begins with an introduction to the mathematical constant e, which is naturally introduced through the concept of compound interest. The speaker uses Excel to demonstrate how compounding periods converge to the value of e, which is approximately 2.7183. They explain the formula used in Excel, which involves raising (1 + 1/n) to the power of n, and show how increasing the number of compounding periods results in the value approaching e. The script also touches on the ubiquity of e and pi in various fields, suggesting these numbers are fundamental to the universe. The speaker ends this section by transitioning back to the topic of compound interest, setting the stage for a deeper exploration in subsequent videos.

05:02
πŸ” Generalizing Compound Interest with Variable Rates

In the second paragraph, the script delves into the generalization of compound interest by introducing a variable interest rate 'r'. The speaker clarifies that 'r' represents the interest rate as a percentage, which is converted to a decimal for calculations. They explain the formula for calculating the amount owed after one compounding period, which involves the initial principal 'p', the annual interest rate 'r', and the number of compounding periods 'n'. Using an example with a 10% interest rate compounded semi-annually, the speaker illustrates how the formula works over time. The paragraph culminates in a discussion about continuous compounding, where 'n' approaches infinity, and introduces a substitution to find the limit of the compound interest formula as 'n' becomes very large, which leads to the use of the constant e raised to the power of 'r'.

Mindmap
Keywords
πŸ’‘e (Euler's number)
Euler's number, commonly denoted as 'e', is an important mathematical constant that is the base of the natural logarithm. It is approximately equal to 2.7183 and is often associated with growth processes in nature and finance. In the video, 'e' is introduced as the value to which the expression (1 + 1/n)^n converges as n approaches infinity, illustrating its fundamental role in the concept of compound interest.
πŸ’‘Compound Interest
Compound interest is the interest on a loan or deposit calculated based on both the initial principal and the accumulated interest from previous periods. It is a key concept in finance and mathematics, as it represents the exponential growth of money over time. The video script discusses compound interest as a way to introduce the mathematical constant 'e', using it to explain how interest accumulates in finance.
πŸ’‘Excel
Excel is a widely used spreadsheet program that allows for data organization, analysis, and calculations. In the context of the video, the speaker mentions using Excel to demonstrate the convergence of the compound interest formula to the value of 'e'. This shows how technology can be utilized to visualize and understand complex mathematical concepts.
πŸ’‘Google as a Calculator
The script mentions that Google can be used as a calculator, which is a feature that allows users to search for mathematical expressions and receive direct answers. This is an example of how technology integrates with everyday life, making complex calculations easily accessible and highlighting the ubiquity of mathematical constants like 'e'.
πŸ’‘Pi (Ο€)
Pi, represented by the Greek letter 'Ο€', is another well-known mathematical constant that is the ratio of a circle's circumference to its diameter, approximately equal to 3.14159. While not the main focus of the video, pi is mentioned alongside 'e' as an example of mathematical constants that appear in various contexts, suggesting a deeper, interconnected nature of mathematical concepts.
πŸ’‘Imaginary Numbers
Imaginary numbers are a concept in mathematics where numbers are defined as multiples of the square root of -1, denoted as 'i'. They extend the real number system and are essential in complex analysis. The video script hints at the mysterious and pervasive nature of mathematical constants like 'e' and pi, including imaginary numbers, which are fundamental in various mathematical and physical theories.
πŸ’‘Continuous Compounding
Continuous compounding refers to the process where interest is calculated an infinite number of times at every instant. It is a concept that leads to the formula for 'e', as the limit of (1 + r/n)^n as n approaches infinity. The video explains how continuous compounding simplifies to the formula e^r * p, where 'p' is the principal amount, illustrating the direct relationship between 'e' and compound interest.
πŸ’‘Interest Rate
The interest rate is the percentage at which interest is paid by borrowers to lenders, typically expressed as an annual percentage rate (APR). In the video, different scenarios of compounding interest are explored by varying the interest rate 'r', which affects the final amount owed after compounding periods.
πŸ’‘Principal
The principal is the initial amount of money that is borrowed or invested. In the context of the video, the principal 'p' is used in the formula for compound interest to calculate the final amount after interest has been applied. The script uses the principal to demonstrate how the final amount owed grows with compound interest.
πŸ’‘Limit
In mathematics, a limit is the value that a function or sequence approaches as the input approaches some value. The video discusses the concept of limits in the context of continuous compounding, where the limit as n approaches infinity of (1 + r/n)^n is used to derive the value of 'e'.
πŸ’‘Loan Shark
A loan shark is an illegal lender who offers loans at extremely high interest rates, often engaging in predatory lending practices. In the video, the term is used humorously to illustrate a scenario where compound interest could be applied, although the ethical implications of such practices are not the focus of the video.
Highlights

The video discusses the concept of compound interest as a natural way to introduce the mathematical constant e.

Demonstrates the convergence of the formula (1 + 1/n)^n to e using Excel as n increases.

Shows that the number e is approximately 2.7183 and is recognized by Google as a calculator input.

Mentions the ability to find e to arbitrary decimal places on certain websites.

Highlights the occurrence of numbers like pi and e in various places in the universe, suggesting a deep underlying connection.

Introduces the idea that e and pi are almost magically related and appear in different areas of mathematics.

Discusses the motivation behind exploring these numbers for those interested in mathematics or starting a 'new cult'.

Explains the generalization of compound interest to different interest rates, not just 100%.

Clarifies that the interest rate r is expressed as a decimal in the formula for compound interest.

Provides a formula for calculating the amount owed after one compounding period with an annual interest rate r and compounding frequency n.

Uses an example with an interest rate of 10% compounded twice a year on a $50.00 loan to illustrate the formula.

Explains the concept of continuous compounding as n approaches infinity.

Substitutes r/n with 1/x to find the limit as n approaches infinity, relating it to x also approaching infinity.

Derives the formula for continuous compounding as e^r, where e is the base of the natural logarithm.

Applies the concept to an interest rate of 10% to show how much would be owed after one year of continuous compounding.

Notes the presence of the principal amount p as a scaling factor throughout the compounding process.

Promises to explore the implications of continuously compounding interest for multiple years in the next video.

Transcripts

Browse More Related Video

Compound interest and e (part 2) | Exponential and logarithmic functions | Algebra II | Khan Academy

Review of Compound Interest (Precalculus - College Algebra 65)

What is e and ln(x)? (Euler's Constant and The Natural Logarithm)

Compound Interest Formula Explained, Investment, Monthly & Continuously, Word Problems, Algebra

Deriving Functions with e!

e to the pi i for dummies

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsFinanceCompound InterestNatural LogExcele ConstantInterest RateCalculusFinancial PlanningEducational