Compound interest and e (part 2) | Exponential and logarithmic functions | Algebra II | Khan Academy

Khan Academy
22 Apr 200810:14
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the concept of compound interest, demonstrating how the amount owed grows as the compounding period decreases. The presenter uses a 100% interest rate example, starting with annual compounding and progressively moving to more frequent compounding periods, such as monthly, daily, and hourly. Through this process, the script reveals that as compounding intervals shrink, the growth rate approaches a constant known as 'e' (approximately 2.71), which is a fundamental number in mathematics, appearing in various fields including finance and natural sciences. The script concludes with the idea of continuous compounding and the intriguing mathematical properties of 'e'.

Takeaways
  • 🧮 The script discusses the concept of compound interest, starting with a simple 100% annual interest rate and then exploring different compounding periods.
  • 📈 The video explains that with daily compounding at a 2.7% daily rate, the formula becomes 1.027 to the power of 365, which is approximately 2.7148 times the principal after one year.
  • 🤔 The presenter makes a mistake with Excel and corrects it by calculating the daily interest rate as 0.274% instead of 2%.
  • 🕒 The concept of compounding interest every hour is introduced, with the rate calculated as 0.0114% per hour, leading to a compounded amount of approximately 2.71443 times the principal after a year.
  • 🔢 As compounding periods decrease, the total amount owed approaches a constant value, which is hinted to be a significant mathematical constant.
  • 🌟 The video reveals that the constant approached is 'e', an important mathematical constant approximately equal to 2.71.
  • 🔬 The script illustrates that as the number of compounding periods increases (approaching infinity), the compounded amount converges to 'e'.
  • 📚 The presenter mentions that 'e' is a transcendental number like pi and appears in various mathematical fields such as combinatorics and complex analysis.
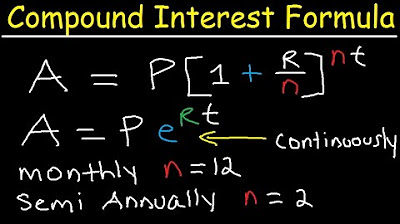
  • 🎯 The general formula for compound interest is presented as P(1 + r/n)^n, where P is the principal, r is the interest rate, and n is the number of compounding periods.
  • 🧐 The video script suggests an experiment to see how many digits of 'e' one can calculate by using increasingly large numbers for n.
  • 🔜 The presenter teases the next video, where they will discuss the concept of continuous compounding, which is compounding an infinite number of times per second.
Q & A

  • What is the initial interest rate mentioned in the script?

    -The initial interest rate mentioned in the script is 100%.

  • What does the script discuss regarding compounding interest?

    -The script discusses how compounding interest works when it is applied at different intervals, such as every six months, every month, every day, and even every hour, and how it approaches the mathematical constant e as the compounding intervals become smaller.

  • What is the daily interest rate if it is compounded daily?

    -The daily interest rate if compounded daily is 0.274%, which is derived from dividing 100% by 365 days.

  • What is the hourly interest rate if interest is compounded hourly?

    -The hourly interest rate if compounded hourly is 0.0114%, which is 100% divided by the number of hours in a year (8760).

  • What is the mathematical constant e in the context of this script?

    -In the context of this script, e is the value that the compounded interest rate approaches as the compounding intervals become smaller and more frequent, eventually tending towards continuous compounding.

  • What is the final amount owed after one year with daily compounding at a daily rate of 0.274%?

    -The final amount owed after one year with daily compounding at a daily rate of 0.274% is approximately $2.7148.

  • How many times does the script mention compounding interest over different periods?

    -The script mentions compounding interest over different periods three times: every six months, every month, and then every day and every hour.

  • What is the significance of the number 365 in the script?

    -The number 365 is significant in the script as it represents the number of days in a year, which is used to calculate the daily compounding interest rate.

  • What is the total amount owed after one year with hourly compounding at an hourly rate of 0.0114%?

    -The total amount owed after one year with hourly compounding at an hourly rate of 0.0114% is approximately $2.71443.

  • What is the script's main point about the relationship between compounding interest and the number e?

    -The script's main point is that as the frequency of compounding interest increases (compounding more times per year), the total amount owed at the end of the year approaches the mathematical constant e, which is approximately 2.71828.

  • What is the script's final conclusion about the concept of continuous compounding?

    -The script concludes that in the limit as the number of compounding periods approaches infinity (continuous compounding), the formula for the amount owed becomes the principal times e raised to the power of the interest rate.

Outlines
00:00
📈 Understanding Compound Interest

The first paragraph explains the concept of compound interest with varying compounding periods. The speaker starts by discussing a simple 100% interest rate for one year and then explores the effects of halving the rate but compounding it every six months. The conversation moves to compounding on a monthly and daily basis, using a daily rate of 2.7% and demonstrating the calculation process with Excel. The speaker corrects a mistake in the Excel formula and provides the correct daily interest rate of 0.274%. The paragraph concludes with the calculation of how much would be owed after one year with daily compounding and then extends the concept to hourly compounding, revealing the owed amount after a year with hourly compounding at a rate of 0.0114%.

05:03
🔍 Approaching the Mathematical Constant e

The second paragraph delves into the mathematical constant e, which is approached as the compounding periods become smaller and more frequent. The speaker illustrates how the amount owed after one year increases as the compounding frequency increases, from yearly to daily and then to hourly. It is observed that despite the increase in the number of compounding periods, the final amount owed seems to gravitate towards a constant value, which is identified as e. The paragraph formalizes the compounding formula using variables and demonstrates how as the number of compounding periods (n) increases, the result approaches the value of e. The speaker emphasizes the significance of e as a transcendental number that appears in various mathematical fields and invites viewers to experiment with large values of n to approximate e more closely.

10:05
🕰️ Continuous Compounding and the Next Steps

The third and final paragraph briefly touches on the concept of continuous compounding, which is the limit as the number of compounding periods approaches infinity. The speaker expresses amazement at the ability to calculate interest rates for continuous compounding, compounding every fraction of a second. The paragraph ends with a teaser for the next video, where the speaker promises to explain how to calculate continuously compounded interest.

Mindmap
Keywords
💡Compound Interest
Compound interest is the interest on a loan or deposit calculated based on both the initial principal and the accumulated interest from previous periods. In the video, the concept is explored by varying the frequency of compounding, showing how the total owed increases with more frequent compounding intervals, ultimately approaching the mathematical constant e.
💡Interest Rate
The interest rate is the percentage at which interest accrues on the initial amount of money invested or borrowed, known as the principal. In the script, the speaker uses a 100% interest rate as a base to demonstrate how different compounding periods affect the final amount owed.
💡Principal
The principal refers to the original amount of money that is invested, borrowed, or deposited. In the context of the video, the principal is the initial amount of money that accrues interest over time, and it's the basis for calculating compound interest.
💡Excel
Excel is a spreadsheet program used for organizing data, performing calculations, and creating charts. In the script, the speaker mentions using Excel to calculate the compounded interest and to illustrate the mathematical process of compounding over different periods.
💡Daily Compounding
Daily compounding refers to the process where interest is calculated and added to the principal on a daily basis. The video script discusses the impact of charging 2.7% daily and compounding it for 365 days, leading to a significant increase in the amount owed.
💡Hourly Compounding
Hourly compounding is a more frequent compounding method where interest is calculated and added every hour. The script explores the idea of compounding interest hourly, showing that even with 8,760 compounding periods in a year, the final amount owed is still remarkably close to the result of daily compounding.
💡e (Euler's number)
Euler's number, commonly represented as 'e', is an important mathematical constant approximately equal to 2.71828. It is the base of the natural logarithm and appears in various mathematical contexts, including compound interest. The video demonstrates that as the frequency of compounding increases towards infinity, the compounded amount approaches 'e'.
💡Transcendental Numbers
Transcendental numbers are numbers that are not the root of any non-zero polynomial equation with rational coefficients. The script mentions 'e' and 'pi' as examples of transcendental numbers, highlighting their unique properties and their occurrence in various mathematical fields, including the calculation of compound interest.
💡Limit
In mathematics, a limit is the value that a function or sequence approaches as the input or index approaches some value. The video discusses the concept of limits in the context of compounding interest, specifically how as the number of compounding periods (n) approaches infinity, the compounded amount approaches 'e'.
💡Continuous Compounding
Continuous compounding is a method of calculating interest where the interest is compounded an infinite number of times per unit time. The script hints at the concept of continuous compounding as the logical extension of increasing the frequency of compounding, suggesting that it can be calculated and results in an amount very close to 'e'.
Highlights

Introduction to compound interest and its effect on loan repayments over time.

Explanation of daily compounding interest at a rate of 2.7% per day, calculated using Excel.

Mistake made in calculating daily compound interest and correction to the formula.

Clarification on the daily interest rate charged, which is 0.274%.

Demonstration of how compounding interest increases over time with daily compounding.

Introduction of the concept of hourly compounding interest.

Calculation of the number of hours in a year for hourly compounding.

Explanation of the hourly interest rate charged, which is 0.0114%.

Illustration of how compounding interest accumulates with hourly compounding.

Observation that compounding interest gravitates towards a mystical number, e.

Introduction of the mathematical constant e and its significance in compounding interest.

Discussion on the uniqueness of e as a transcendental number similar to pi.

Explanation of the mathematical formula for compound interest as n approaches infinity.

Demonstration of how compounding interest converges to e as the number of compounding periods increases.

Introduction to the concept of continuous compounding and its relation to e.

Teaser for the next video on calculating interest rates with continuous compounding.

Transcripts

Browse More Related Video

Compound interest and e (part 3) | Exponential and logarithmic functions | Algebra II | Khan Academy

Review of Compound Interest (Precalculus - College Algebra 65)

Introduction to compound interest and e | Algebra II | Khan Academy

Deriving Functions with e!

Compound Interest Formula Explained, Investment, Monthly & Continuously, Word Problems, Algebra

2.2 - Derivatives of Exponential Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Compound InterestMathematicsFinancial EducationInterest RateExcel CalculationTranscendental NumbersE ConvergenceEducational ContentInvestment StrategyMathematical Analysis