Partial Fraction Decomposion
TLDRThis video tutorial introduces the concept of partial fraction decomposition, a mathematical technique used to simplify complex rational expressions. The instructor demonstrates how to break down a single fraction into smaller, more manageable fractions by finding common denominators and factoring the denominator. Through step-by-step examples, the video shows how to set up equations to solve for unknown constants, using either a system of linear equations or by strategically plugging in values. The process is illustrated with two problems, guiding viewers through the factorization, setting up of equations, and solving for constants to achieve the decomposed form of the rational function.
Takeaways
- 📚 Partial fraction decomposition is a method used to break down a single complex fraction into simpler fractions.
- 🔍 The process involves finding a common denominator to combine fractions and then decomposing them into smaller parts.
- 📐 The video provides an example of combining 2/(3x) + 4/(5y) into a single fraction with a common denominator of 15xy.
- 📝 The method is the reverse of combining fractions; it starts with a single fraction and breaks it into multiple, simpler fractions.
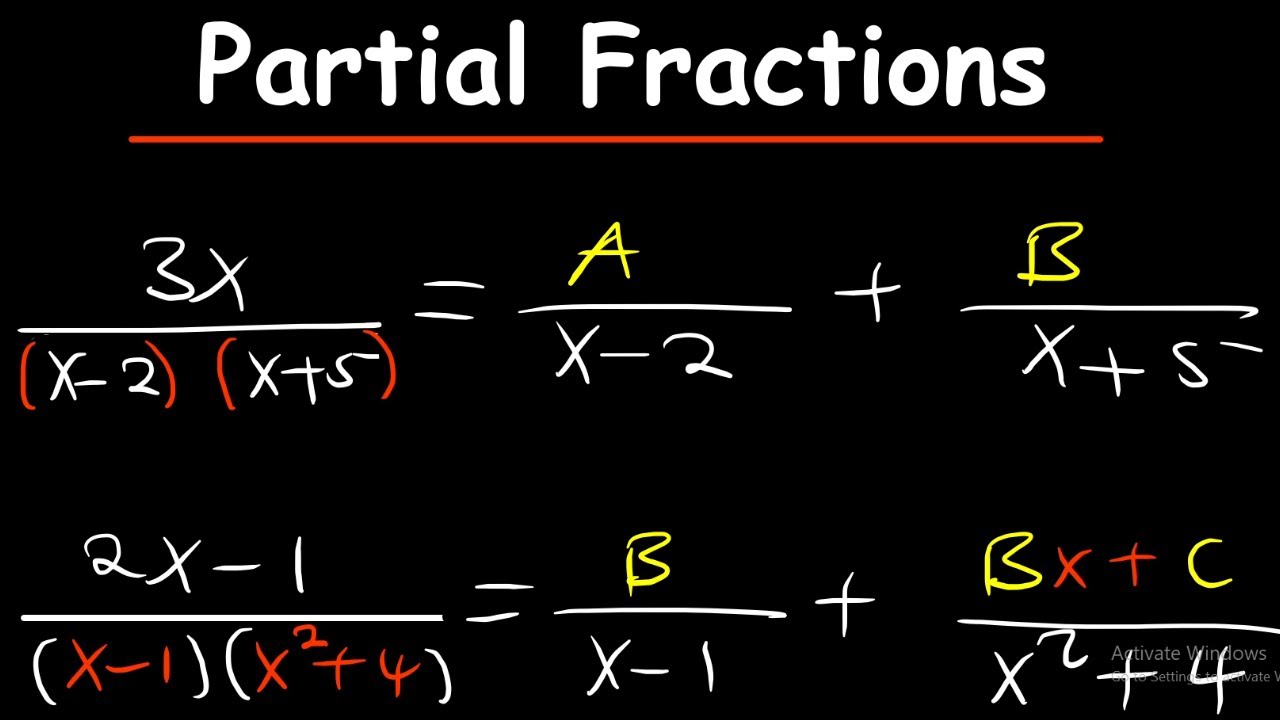
- 🔑 To decompose a fraction, the denominator must be fully factored, including linear and quadratic factors, and repeated factors if necessary.
- 📈 The script illustrates the decomposition of the rational function (7x - 23) / (x^2 - 7x + 10) into simpler fractions.
- 🧩 The numerator of the decomposed fractions is determined by constants (a and b in the example) that are solved for using substitution or system of equations.
- 🔍 The video demonstrates solving for constants by plugging in specific x values that simplify the equation and isolate the constants.
- 📉 The example shows that after finding constants a and b, the original fraction can be expressed as a sum of simpler fractions: 3/(x - 2) + 4/(x - 5).
- 🔄 To verify the decomposition, the simpler fractions can be multiplied back together to check if they yield the original complex fraction.
- 📚 Another example is given with the fraction (29 - 3x) / (x^2 - x - 6), which is decomposed into 4/(x - 3) - 7/(x + 2) after finding the constants.
Q & A
What is partial fraction decomposition?
-Partial fraction decomposition is a method used to break down a single complex fraction into simpler fractions. It is the reverse process of combining fractions with a common denominator into a single fraction.
Why do we need to find common denominators before performing partial fraction decomposition?
-Finding common denominators is necessary because it allows us to combine fractions into a single fraction, which is the starting point for partial fraction decomposition.
Can you give an example of how to combine fractions into a single fraction?
-Sure, if we have 2/(3x) + 4/(5y), we can multiply the second fraction by (3x)/(3x) and the first one by (5y)/(5y) to get a common denominator of 15xy, resulting in (10y + 12x)/15xy.
What is the first step in performing partial fraction decomposition on a rational function?
-The first step is to factor the denominator completely, if possible, into linear and/or quadratic factors.
What are linear factors and how can they be identified?
-Linear factors are expressions of the form x + a or ax + b, where a and b are constants. They can be identified by their linear (first-degree) nature in the variable x.
How do you determine the constants in a partial fraction decomposition?
-The constants can be determined by multiplying both sides of the equation by the denominator and then solving for the constants using methods such as plugging in specific x values or setting up a system of linear equations.
What is a repeated linear factor and how does it affect the decomposition?
-A repeated linear factor is a factor that appears more than once in the denominator, like (x - a)^2. It affects the decomposition by requiring a term with a variable coefficient (not just a constant) in the corresponding fraction.
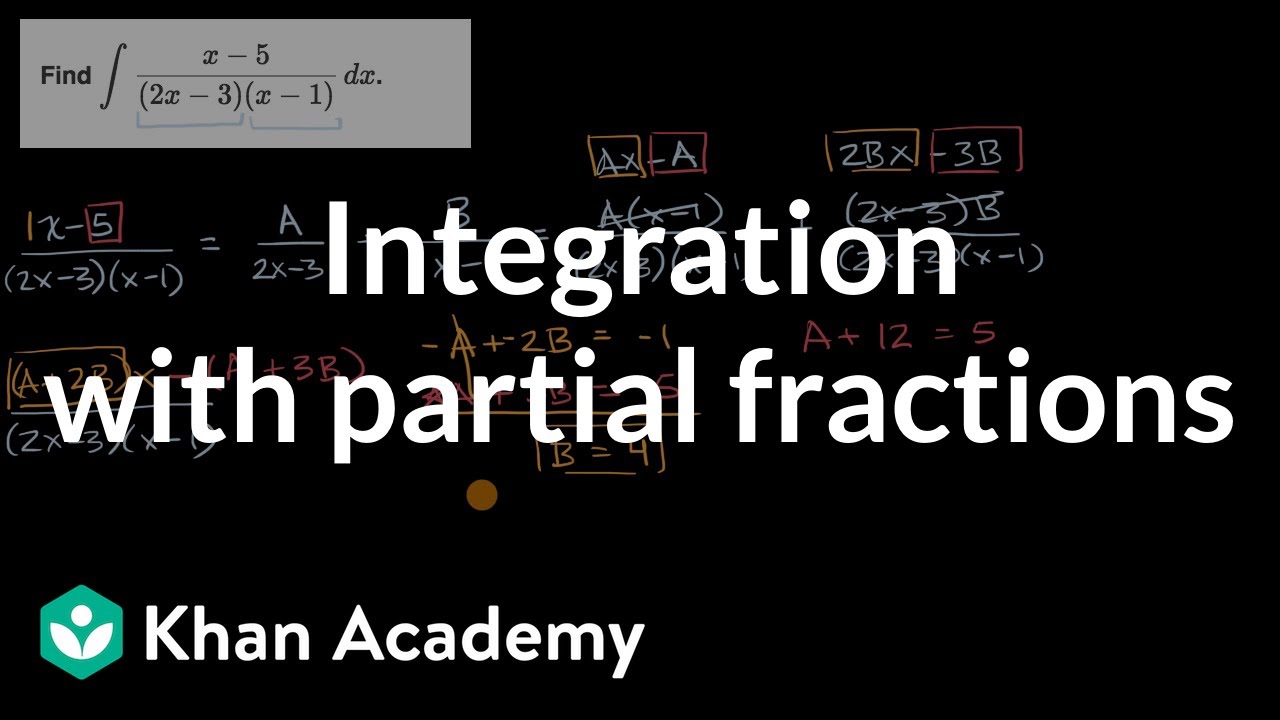
Can you provide a step-by-step guide on how to decompose the fraction (7x - 23)/(x^2 - 7x + 10)?
-First, factor the denominator to (x - 2)(x - 5). Then, set up the decomposition as A/(x - 2) + B/(x - 5). Multiply through by the denominator to find A and B by plugging in convenient x values, such as the roots of the denominator.
How do you check if your partial fraction decomposition is correct?
-To check, combine the decomposed fractions back into a single fraction and see if it simplifies to the original complex fraction.
What is the purpose of plugging in specific x values when determining constants in partial fraction decomposition?
-Plugging in specific x values simplifies the equation by eliminating one of the constants, making it easier to solve for the other constant.
Can you provide an example of a quadratic factor and how it might be used in partial fraction decomposition?
-A quadratic factor might look like x^2 + 8x + 16, which is factorable into (x + 4)^2. In partial fraction decomposition, if a quadratic factor is irreducible, it would be used as is in the decomposition without further factoring.
Outlines
📚 Introduction to Partial Fraction Decomposition
This paragraph introduces the concept of partial fraction decomposition, a mathematical technique used to simplify complex rational functions. The speaker illustrates the method by first showing how to combine two fractions into a single fraction with a common denominator. The example given is simplifying 2/(3x+4) + 4/(5y) into a single fraction with a common denominator of 15xy. The process involves multiplying each fraction by the missing part of the common denominator. The concept is then reversed to demonstrate how a single fraction can be decomposed into smaller fractions, which is the essence of partial fraction decomposition. The speaker then sets up an example problem involving the rational function (7x - 23) / (x^2 - 7x + 10) and explains the importance of factoring the denominator completely before proceeding with the decomposition.
🔍 Factoring and Decomposing a Rational Function
In this paragraph, the speaker continues the explanation of partial fraction decomposition by focusing on the factorization of the denominator of the given rational function. The function (7x - 23) / (x^2 - 7x + 10) is factored into (x - 2)(x - 5). The speaker emphasizes the need to express the denominator in terms of linear and quadratic factors and introduces the concept of repeated linear and quadratic factors. The rational function is then set to be decomposed into two fractions with linear factors in the denominator, each having an unknown constant (a and b) to be determined. The speaker outlines the process of multiplying both sides of the equation by the denominator to isolate these constants and suggests using specific x values to solve for them.
🔢 Solving for Constants in Partial Fractions
The speaker demonstrates how to solve for the constants a and b in the partial fraction decomposition of the rational function (7x - 23) / ((x - 2)(x - 5)). By strategically choosing x values that simplify the equation, the speaker plugs in x = 5 to eliminate the term involving a, and then solves for b, finding that b = 4. The process is repeated with x = 2 to solve for a, yielding a = 3. The speaker then rewrites the original problem with the determined values of a and b, showing that the decomposed form of the rational function is 3/(x - 2) + 4/(x - 5). To verify the result, the speaker combines the two fractions and confirms that they yield the original rational function.
📘 Applying Partial Fraction Decomposition to Another Problem
In this final paragraph, the speaker applies the partial fraction decomposition technique to another problem, decomposing the rational function (29 - 3x) / (x^2 - x - 6). The denominator is factored into (x - 3)(x + 2), and the function is set equal to a fraction with two unknown constants, a and b. The speaker again uses the method of multiplying both sides by the denominator and plugging in specific x values to solve for the constants. By setting x = 3 and x = -2, the speaker finds that a = 4 and b = -7, respectively. The final decomposed form of the rational function is given as 4/(x - 3) - 7/(x + 2), and the speaker concludes the explanation by reiterating the steps and the result.
Mindmap
Keywords
💡Partial Fraction Decomposition
💡Common Denominator
💡Rational Function
💡Factoring
💡Linear Factors
💡Quadratic Factors
💡Repeated Linear Factor
💡System of Linear Equations
💡Plugging in x Values
💡Combining Fractions
Highlights
Introduction to partial fraction decomposition and its purpose.
Illustration of combining fractions with a common denominator.
Explanation of breaking down a single fraction into smaller fractions as the reverse of combining fractions.
Demonstration of factoring a trinomial in the denominator for partial fraction decomposition.
Description of linear and quadratic factors in the context of decomposition.
Introduction of repeated linear and quadratic factors and their importance in decomposition.
Setting up the equation for partial fraction decomposition with constants a and b.
Strategy for determining the values of constants a and b using multiplication by the denominator.
Method of solving for constants by plugging in specific x values.
Example calculation of constants a and b for a given rational function.
Verification of the partial fraction decomposition by recombining the fractions.
Application of the method to a second problem with a different rational function.
Factoring the denominator of the second problem to set up for partial fraction decomposition.
Solving for the constants in the second problem using the same strategy as before.
Final answer presentation for the second problem with constants a and b.
Emphasis on the importance of understanding the terms and process of partial fraction decomposition.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: