how to setup partial fractions (all cases)

bprp calculus basics
24 Apr 202209:08
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script delves into the concept of partial fractions, a technique used in calculus for integrating rational functions. It clarifies why, when dealing with a fraction that has a repeating factor in the denominator, it's necessary to include all powers of that factor, starting from the first. The script outlines the setup for partial fractions, emphasizing the importance of ensuring the degree of the numerator is less than the degree of the denominator. It also explains how to handle irreducible quadratics and provides a strategy for setting up and solving for constants in partial fraction decomposition. The video aims to demystify the 'biggest why' in partial fractions, using substitution to simplify complex expressions and offering a clear, step-by-step approach to mastering the topic.

Takeaways
  • 📚 Partial fractions are used to decompose complex rational expressions into simpler fractions.
  • 🔍 The degree of the numerator must always be less than the degree of the denominator in a partial fraction setup.
  • 📉 When dealing with a repeating linear factor, such as (x + 2)^2, both the first power and the squared power need to be included in the setup.
  • 📝 For irreducible quadratic factors, the setup involves a linear term on the numerator and a constant on the top for the corresponding fraction.
  • 🔑 The general form for a partial fraction with a quadratic factor includes a linear term on the numerator (bx + c).
  • 📈 The 'build up the power' method is used to systematically set up the numerators for repeated factors, starting from the first power and incrementing by one.
  • 🧩 It's possible to split fractions with single-term denominators into separate fractions for easier decomposition.
  • 🔄 The process of substitution, such as setting t = x + 2, can simplify complex expressions and help in setting up partial fractions.
  • 🔍 The top of the fraction in a partial fraction decomposition can be constants or linear terms, depending on the degree of the denominator.
  • 📐 The method of partial fractions is not just about the numbers but also about the form of the expression, which is crucial for the setup.
  • 🔑 To solve for the constants in a partial fraction decomposition, techniques like the 'kapha method' can be used, as mentioned for a future video.
Q & A

  • What is the main topic of the video script?

    -The main topic of the video script is explaining the concept of partial fractions, particularly when dealing with repeating factors in the denominator.

  • Why is the degree of the numerator less than the degree of the denominator when setting up partial fractions?

    -The degree of the numerator must be less than the degree of the denominator to ensure that the fraction can be decomposed into simpler fractions that can be easily integrated or simplified.

  • What does the term 'repeating factor' refer to in the context of partial fractions?

    -A 'repeating factor' refers to a factor in the denominator that appears more than once, such as (x + 2)^2, which requires a different approach in setting up the partial fractions.

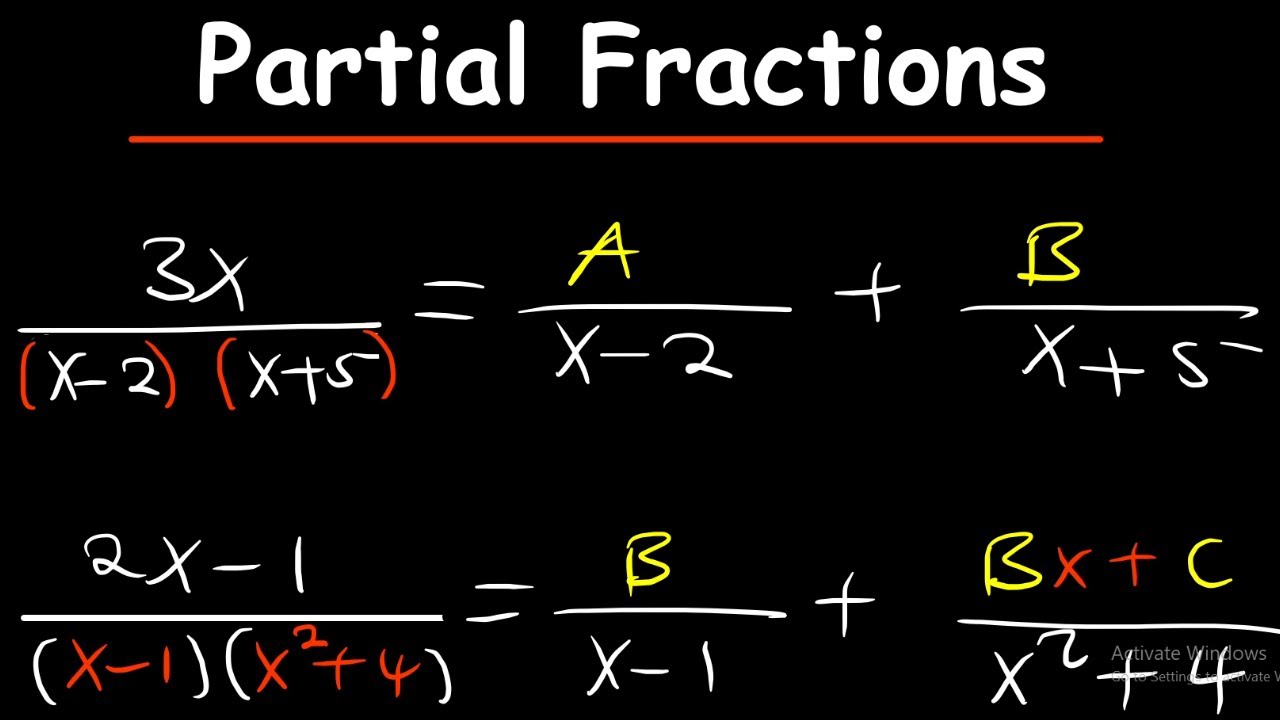
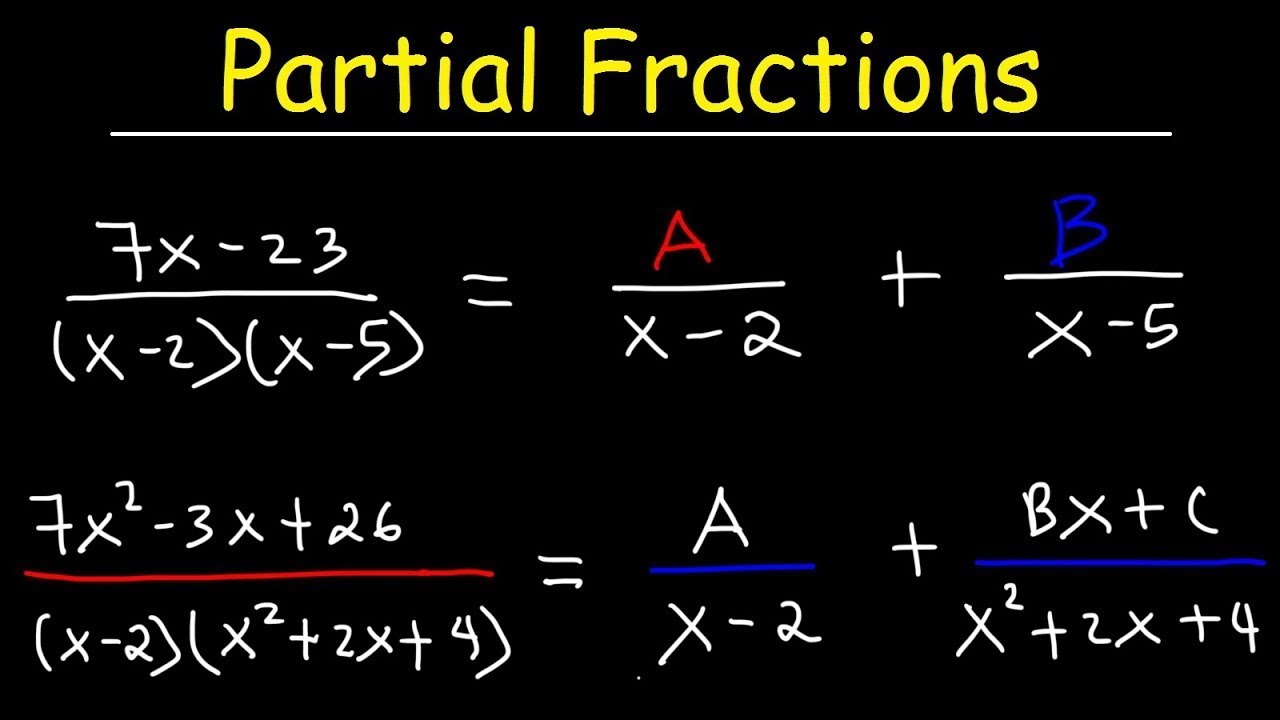
  • What is the general form of the setup for partial fractions when dealing with linear factors?

    -The general form for partial fractions with linear factors is to have constants (A and B) as the numerators over each linear factor in the denominator, ensuring the degree of the numerator is one less than the degree of the denominator.

  • How does the setup change when there is an irreducible quadratic in the denominator?

    -When there is an irreducible quadratic in the denominator, the setup involves a constant numerator over the quadratic factor for the first fraction, and a linear term (Bx + C) over the quadratic for the second fraction.

  • What is the 'build up the power' method mentioned in the script?

    -The 'build up the power' method refers to starting with the lowest power of x in the denominator and incrementally increasing the power by one for each subsequent term in the partial fraction decomposition, until reaching the highest power present in the original denominator.

  • Why is it necessary to include both x and x^2 in the setup when dealing with a quadratic denominator like x^2?

    -Including both x and x^2 is necessary because x^2 can be seen as x multiplied by itself (x*x), which means that when setting up partial fractions, we need to account for each power of x separately, starting from the first power and building up.

  • How can we determine the constants A, B, and C in the partial fraction setup?

    -The constants A, B, and C can be determined by using methods such as equating coefficients or the Heaviside cover-up method, which involves substituting specific values to solve for these constants.

  • What is the purpose of the substitution t = x + 2 in the script?

    -The substitution t = x + 2 simplifies the expression by transforming it into a form that is easier to work with when setting up partial fractions, especially when dealing with repeating factors.

  • Can the method of partial fractions be applied to any rational function?

    -Partial fractions can be applied to any rational function where the degree of the numerator is less than the degree of the denominator. It is particularly useful for integration and simplification purposes.

Outlines
00:00
📚 Understanding Partial Fractions Decomposition

This paragraph delves into the concept of partial fractions, particularly when dealing with a complex fraction that includes a repeating factor in the denominator. The speaker explains the importance of setting up the equation correctly, ensuring the degree of the numerator is less than that of the denominator. The example provided involves a fraction with a quadratic factor in the denominator, which is irreducible and requires a different approach than linear factors. The speaker also introduces the general form for setting up partial fractions, emphasizing the need for constants in the numerator when the denominator is quadratic and explaining how to handle different cases, including irreducible quadratics and linear factors.

05:05
🔍 Deep Dive into Repeating Factors and Building Up Powers

In this paragraph, the focus shifts to the intricacies of handling repeating factors in partial fraction decomposition. The speaker illustrates the process of 'building up the power', which involves starting with the first power of x and incrementally increasing the power until it matches the highest power in the denominator. The paragraph provides a step-by-step guide on setting up the fraction, including the substitution of variables to simplify the expression. The use of substitution (e.g., setting t = x + 2) is highlighted as a method to transform the fraction into a more manageable form, allowing for easier decomposition. The speaker concludes by reiterating the importance of correctly identifying and setting up the terms in the numerator to match the powers in the denominator.

Mindmap
Keywords
💡Partial Fractions
Partial fractions is a technique in calculus used to decompose a complex rational function into simpler fractions. In the video, the concept is introduced to handle integration of rational functions where the numerator has a lower degree than the denominator. The script discusses the setup for partial fractions, emphasizing the importance of the degree of the numerator being less than the degree of the denominator.
💡Repeating Factor
A repeating factor in algebra refers to a factor that appears more than once in the denominator of a fraction. In the context of the video, when dealing with a quadratic factor that cannot be factored further with real numbers, it is treated as a repeating factor. The script explains that when a repeating factor is present, the setup for partial fractions involves powers of the factor on the outside of the parentheses.
💡Degree
In mathematics, the degree of a polynomial is the highest power of the variable. The video script mentions that the degree of the numerator must be less than the degree of the denominator for partial fractions to be applicable. This is a crucial condition for setting up the partial fraction decomposition correctly.
💡Irreducible Quadratic
An irreducible quadratic is a quadratic polynomial that cannot be factored over the real numbers. In the script, the concept is used to describe a quadratic term in the denominator that does not have real roots, hence it must be dealt with as it is during the partial fraction decomposition process.
💡Setup
The setup in the context of partial fractions refers to the initial arrangement of the equation before applying the decomposition method. The video script outlines different cases for setting up partial fractions, such as when dealing with linear factors, irreducible quadratics, and repeating factors, ensuring that the top degree is always less than the bottom degree.
💡Kapha Method
Although not explicitly defined in the script, the 'kapha method' seems to refer to a technique for finding the coefficients (a, b, c, etc.) in the partial fraction decomposition. The script mentions that this method will be discussed in another video, indicating it as a method to solve for the unknowns in the setup.
💡General Form
The general form in the context of the video refers to the standard template used for setting up partial fractions. For instance, when dealing with a quadratic term, the general form might include a linear term (bx + c) on the top and the quadratic term on the bottom. The script emphasizes the importance of using the general form to correctly set up the partial fractions.
💡Build Up the Power
This phrase from the script describes a strategy for constructing the terms in the numerator when dealing with repeating factors in the denominator. It involves starting with the lowest power of x and incrementally increasing the power by one for each term in the sequence, until reaching the power that matches the highest power in the denominator.
💡Substitution
Substitution is a mathematical technique where one variable is replaced with another to simplify expressions or to make them more recognizable. In the script, the concept is used to transform the expression involving x into one involving a new variable t, which simplifies the process of setting up partial fractions.
💡Integration
Although not explicitly mentioned in the script, the overarching theme of the video is likely related to integration, as partial fractions are commonly used to simplify the integration of rational functions. The script's focus on breaking down complex fractions suggests that the ultimate goal is to make integration more manageable.
Highlights

Introduction to partial fractions and the importance of degree on the numerator being less than the denominator.

Setup for partial fractions when dealing with linear factors.

Explanation of why the degree on the top must be one less than the degree on the bottom.

Strategy for setting up partial fractions with irreducible quadratic factors.

Dealing with repeating factors in partial fraction decomposition.

The concept of 'build up the power' in setting up partial fractions for higher degree polynomials.

How to handle a quadratic term in the denominator with a linear numerator.

The method of splitting the fraction when the denominator has a single term.

Cancellation technique in partial fractions to simplify the expression.

The necessity of including terms up to the power of the highest degree in the denominator.

The process of substitution to simplify complex partial fraction expressions.

Transformation of the original expression into a simpler form using substitution.

Re-establishing the original variable to match the simplified expression.

The significance of the form over the numbers in setting up partial fractions.

Final expression setup for partial fractions with quadratic and linear terms.

The reason for including each term in the partial fraction setup, even if it seems redundant.

Conclusion and summary of the key points in partial fraction decomposition.

Transcripts

Browse More Related Video

Partial Fractions Decomposition simplified

AP Calculus BC Lesson 6.12

7.3.6 Partial Fraction Decomposition

Partial Fraction Decomposion

Integration By Partial Fractions

Lesson 7 - Integration By Partial Fractions (Calculus 2 Tutor)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Partial FractionsRepeating FactorsQuadratic EquationsMathematics TutorialDegree ComparisonIrreducible QuadraticsAlgebraic TechniquesFraction DecompositionEducational ContentMath Strategy