Integration By Partial Fractions
TLDRThis video tutorial explains the process of integrating rational functions using partial fraction decomposition. It begins by discussing the importance of completely factoring the denominator and distinguishing between linear and quadratic factors. The video then illustrates the technique by breaking down complex fractions into simpler ones and demonstrates how to calculate the constants involved. It proceeds with examples, showing how to integrate expressions involving linear and quadratic factors, and concludes with a more complex example that includes a repeated linear factor. The explanation is detailed, emphasizing the step-by-step approach and the use of logarithmic functions in integration.
Takeaways
- 📚 The video discusses the method of integrating rational functions using partial fraction decomposition.
- 🔍 Before applying partial fractions, ensure the integral has a completely factored bottom part, especially looking for differences of perfect squares.
- 🌟 Linear factors are terms like x, ax, bx + c, while quadratic factors include x², ax², bx² + cx + d.
- 📝 The process of partial fraction decomposition involves breaking down a complex fraction into simpler fractions.
- 🎯 To find the values of constants a and b in the decomposition, set up and solve a system of equations by multiplying through by the denominators and plugging in specific x-values.
- 🌐 The anti-derivative of 1/x is ln|x|, and for more complex rational functions, apply the natural logarithm after integrating each term.
- 📌 When integrating rational functions, use the properties of logarithms to combine multiple log terms into a single term if possible.
- 🔧 For repeated linear factors, such as (x - p)², the decomposition will include an additional term with the factor squared, like c/(x - p)².
- 🧩 The video provides a step-by-step walkthrough of several examples, demonstrating how to apply partial fraction decomposition and integrate the resulting simpler fractions.
- 📐 The video also touches on alternative integration methods, such as u-substitution and trigonometric substitution, for certain types of rational functions.
- 🔑 Remember to include the constant of integration, C, at the end of the integration process.
Q & A
What is the main topic of the video?
-The main topic of the video is how to integrate rational functions using partial fraction decomposition.
What is the first step in integrating rational functions?
-The first step is to ensure that the integral is completely factored, particularly the denominator, which may involve factoring a difference of perfect squares.
What is the difference between a linear factor and a quadratic factor in this context?
-A linear factor includes terms like x, 3x, x plus 4, 4x minus 5, while a quadratic factor typically has an x squared term, such as x squared, x squared plus 4, x squared plus 3x, or x squared plus 2x plus 7.
How does partial fraction decomposition work?
-Partial fraction decomposition involves breaking up a single complex fraction into two or more simpler fractions. It is used to decompose an expression into parts that can be more easily integrated.
What is the shortcut technique for calculating the values of a and b in partial fraction decomposition?
-The shortcut technique involves plugging in certain x values that make the denominators of some fractions zero, allowing for the simplification and solution of the constants a and b.
How can the anti-derivative of one over x be expressed?
-The anti-derivative of one over x is the natural logarithm of the absolute value of x, denoted as ln|x|, because the natural log function cannot have a negative value inside it.
What is the method used to integrate expressions of the form 1/(x^2 + a^2)?
-The method used is substitution, where x is replaced with a trigonometric function, typically tangent, and the integral is evaluated using the properties of trigonometric functions and their derivatives.
How does the video demonstrate the process of partial fraction decomposition?
-The video demonstrates the process by first setting up the decomposition, then solving for the constants using substitution, and finally integrating the simpler fractions to find the anti-derivative of the original expression.
What is the significance of the constant of integration, C, in the context of anti-derivatives?
-The constant of integration, C, is included in the anti-derivative to account for the arbitrary constant that can arise when evaluating definite integrals or when the initial value of the function is not specified.
How does the video handle repeated linear factors in partial fraction decomposition?
-For repeated linear factors, the video shows that additional fractions are introduced in the decomposition, with each fraction having a power of the repeated factor in the denominator, and constants are solved for each of these fractions.
What is the final expression for the integral of 1/(x^2 - 1)(x^2 + 4)?
-The final expression for the integral is ln|x + 1| - 1/2 arctan(x/2) + C, where C is the constant of integration.
Outlines
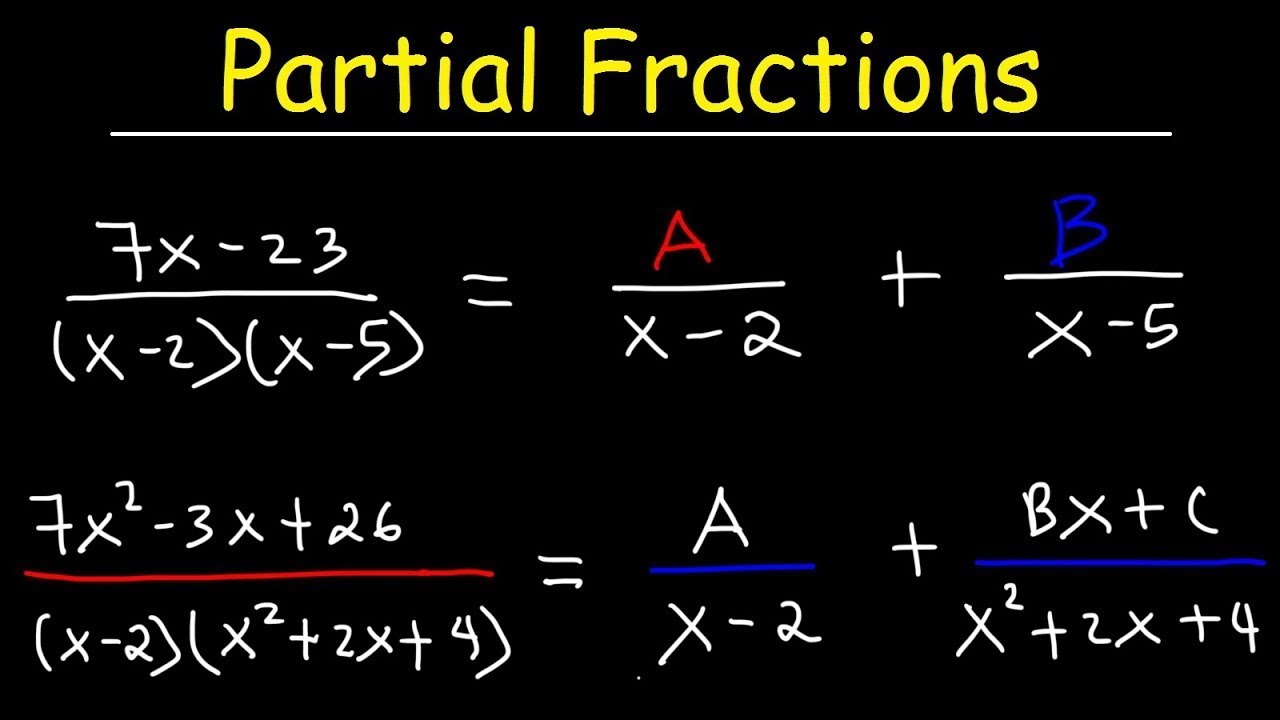
📚 Introduction to Partial Fraction Decomposition
This paragraph introduces the concept of partial fraction decomposition, a technique used to integrate rational functions. It explains the importance of having the integral completely factored, particularly focusing on the factorization of a difference of perfect squares. The explanation includes the distinction between linear and quadratic factors and sets up the foundation for breaking down complex fractions into simpler ones. The paragraph also provides an example of how to decompose a fraction into simpler fractions, akin to the process of finding a common denominator.
🔢 Calculating Values of a and b in Partial Fractions
The paragraph delves into the method of determining the values of constants a and b in a partial fraction decomposition. It describes a shortcut technique involving the selection of specific x values that simplify the equation to solve for these constants. The process is illustrated with a worked example, where the values of a and b are calculated, and then used to find the indefinite integral of the given expressions. The explanation transitions into discussing the anti-derivative of rational functions and how to integrate them into natural logarithmic functions.
🌟 Anti-Derivatives of Rational Functions
This section focuses on finding the anti-derivatives of rational functions, particularly those involving the natural logarithm. It explains the process of integrating functions of the form 1/x and provides the general anti-derivative for such functions. The paragraph then applies this knowledge to the previously calculated values of a and b to find the final anti-derivative of the given expression, including the constant of integration. The explanation is clear and methodical, ensuring a solid understanding of the integration process.
📈 Factoring and Solving a Trinomial
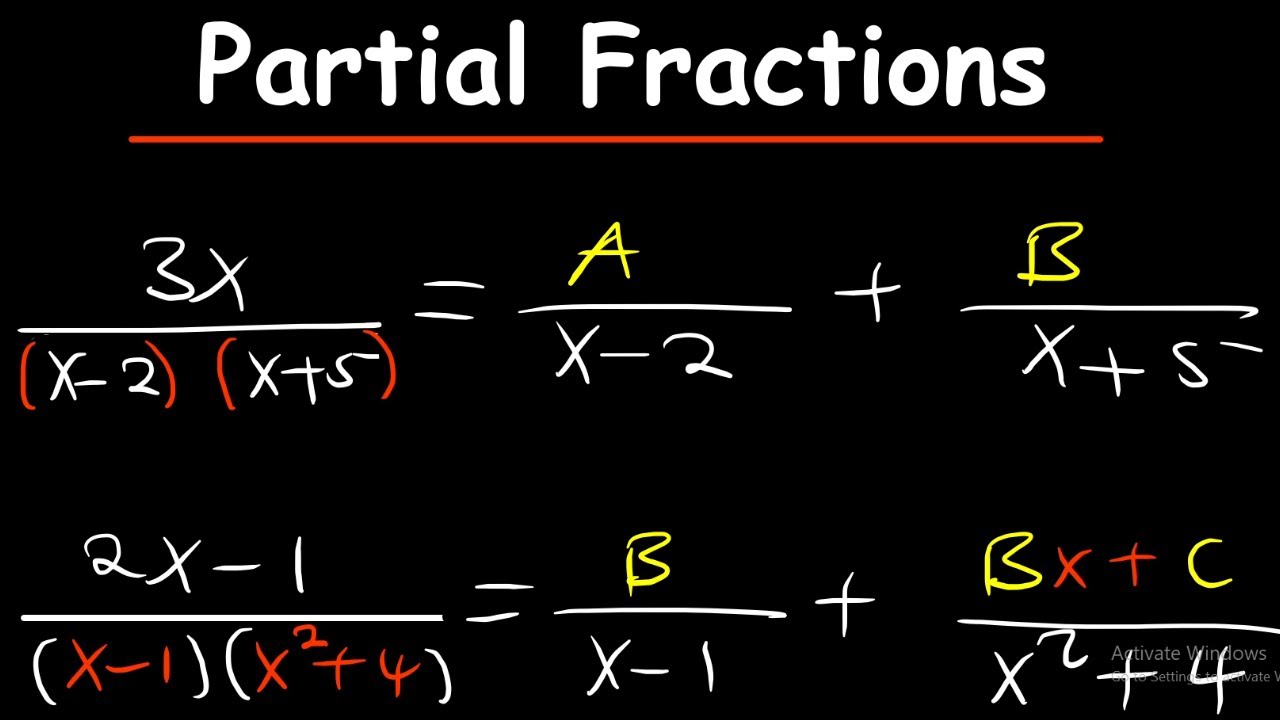
The paragraph presents a step-by-step approach to factoring a trinomial and solving for the constants a and b in a rational function. It begins with identifying the correct factors for the trinomial x^2 + 2x - 15 and proceeds to set up the partial fraction decomposition. The method of plugging in x values to find a and b is reiterated, with a new example provided for practice. The paragraph emphasizes the importance of careful calculation and the use of linear factors to simplify the equation.
🔄 Dealing with Repeated Linear Factors
This section discusses the approach to handling rational functions with repeated linear factors. It explains the setup for the integral involving three fractions due to the presence of a repeated linear factor (x - 2)^2. The paragraph illustrates the process of rewriting the expression without integral symbols and solving for the constants a, b, and c. The explanation includes the method of plugging in values and the use of properties of logarithms to simplify the final expression.
🧠 Solving a Complex Partial Fraction Decomposition
The paragraph tackles a complex problem involving a linear factor and a repeated linear factor. It outlines the process of setting up the problem with three fractions and solving for the constants a, b, and c. The explanation includes the method of plugging in values to find the constants and the use of u-substitution for integration. The paragraph concludes with the integration of the given expression using the power rule and the properties of logarithms.
📊 Factoring a Quadratic Expression and Solving for Constants
This section focuses on factoring a quadratic expression and solving for the constants a, b, c, and d in a rational function. It explains the process of factoring x^2 - 1 and setting up the partial fraction decomposition with a quadratic factor. The paragraph details the method of plugging in x values to find the constants and the importance of accuracy in these calculations. The explanation includes the integration of the quadratic factor using a known formula and the use of trigonometric substitution if necessary.
🎓 Combining Logs and Finalizing the Integral
The final paragraph combines the previously found constants and anti-derivatives to present the complete solution to the integral problem. It explains how to combine the natural logarithm terms and the use of the arc tangent function to integrate the quadratic factor. The paragraph provides a clear and concise final answer, demonstrating the application of the concepts discussed throughout the video script.
Mindmap
Keywords
💡Partial Fraction Decomposition
💡Rational Functions
💡Integration
💡Linear Factors
💡Quadratic Factors
💡Difference of Perfect Squares
💡Anti-Derivative
💡Natural Logarithm
💡Integration by Substitution
💡Trinomial
💡Arctangent
Highlights
The video discusses the method of integrating rational functions using partial fraction decomposition.
A key step is to ensure the integral has a completely factored bottom part, particularly looking for differences of perfect squares.
Linear factors include terms like x, 3x, x plus 4, 4x minus 5, while quadratic factors include x squared, x squared plus 4, x squared plus 3x, and x squared plus 2x plus 7.
Partial fraction decomposition involves breaking a complex fraction into simpler fractions.
An example is provided to illustrate how to decompose 9/20 into simpler fractions 1/4 and 1/5.
The process of determining the values of constants a and b in the decomposition is explained through algebraic manipulation.
The video demonstrates how to integrate rational functions into natural logarithmic functions, with the anti-derivative of 1/x being ln|x|.
Integration by substitution and the use of properties of logarithms are also discussed for more complex integrals.
A method for dealing with repeated linear factors is introduced, such as integrating 1/(x-2)^2 by introducing an additional fraction c/(x-2)^2.
The video provides a step-by-step solution for integrating x-4/(x^2+2x-15) by factoring the denominator and setting up the partial fraction decomposition.
The process of finding the constants a, b, c, and d for a more complex rational function is detailed, including the use of plugging in values to solve for these constants.
The video concludes with a comprehensive example of integrating x^2+9/(x^2-1)(x^2+4) and the use of trigonometric substitution for certain integrals.
A formula for integrating dx/(x^2+a^2) is mentioned, which can simplify the process when applicable.
The final answer for the example involving x^2+9/(x^2-1)(x^2+4) is provided in both log and arc tangent forms, showcasing flexibility in representing the solution.
The video emphasizes the importance of careful calculation when dealing with partial fraction decomposition and integration of rational functions.
The method of partial fraction decomposition is highlighted as a powerful tool for integrating complex rational functions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: