7.3.6 Partial Fraction Decomposition

Justin Backeberg
14 Apr 202011:37
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers a comprehensive guide on partial fraction decomposition, a technique used in mathematics to simplify complex rational expressions. The process involves factoring the denominator, setting up a system of equations with unknown coefficients, and solving for these coefficients. The video provides a step-by-step breakdown of two examples, demonstrating how to decompose rational expressions into simpler fractions, ultimately enhancing the viewer's understanding of this mathematical concept.

Takeaways
  • 📚 Partial fraction decomposition is a technique used to break down complex rational expressions into simpler fractions.
  • 🔍 The process involves starting with a larger fraction and working backwards to find the partial fractions that make up the original expression.
  • 🌟 Factoring the denominator is the first step in the decomposition process, looking for factors that can be used as denominators for the partial fractions.
  • 🎯 Setting up the partial fractions with unknown coefficients (a and b) is crucial, which will later be solved for using a system of equations.
  • 📐 Multiplying both sides of the equation by the factors of the denominator helps to eliminate fractions and simplify the equation.
  • 🔄 Canceling out common factors in the numerator and denominator is essential to isolating the original numerator and setting up the system of equations.
  • 📑 Solving the system of equations for the coefficients a and b can be done using various methods such as substitution, elimination, or matrices.
  • 🔢 Once the values of a and b are found, they are substituted back into the partial fraction decomposition to obtain the final simplified form.
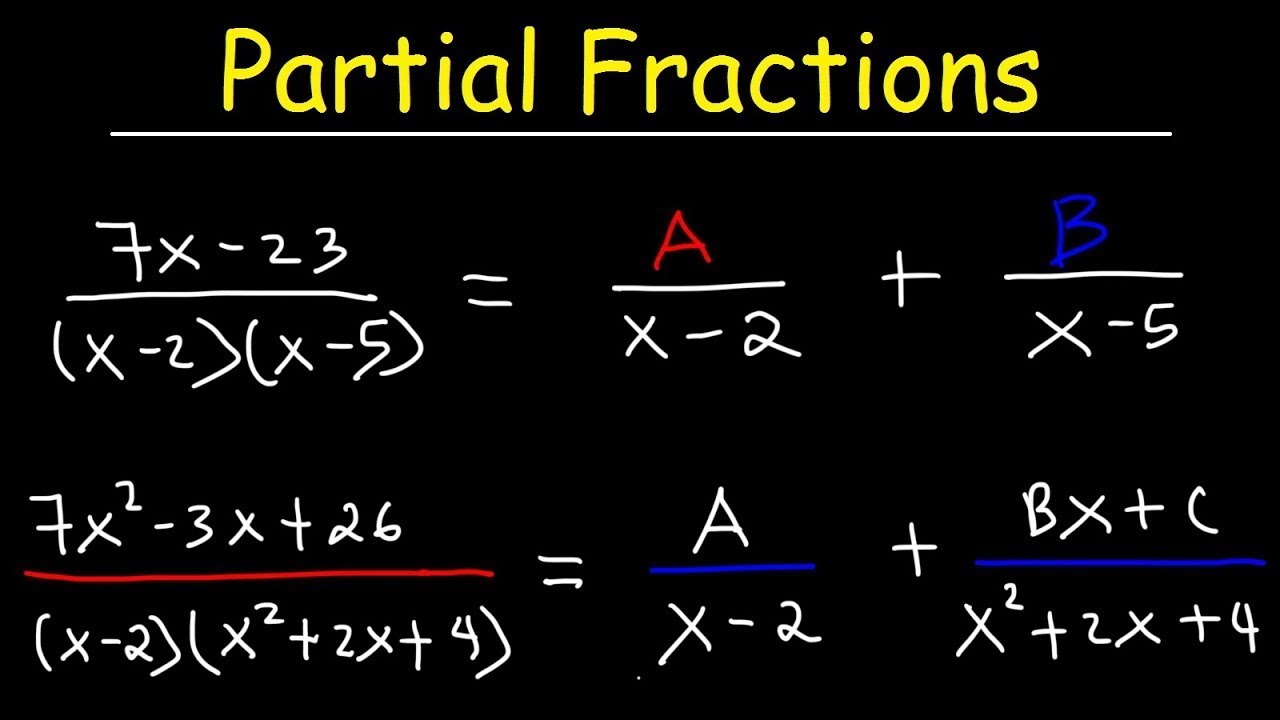
  • 📈 The video provides a step-by-step example of decomposing the rational expression 5x - 1 / (x² - 2x - 15) into partial fractions 3 / (x - 5) + 2 / (x + 3).
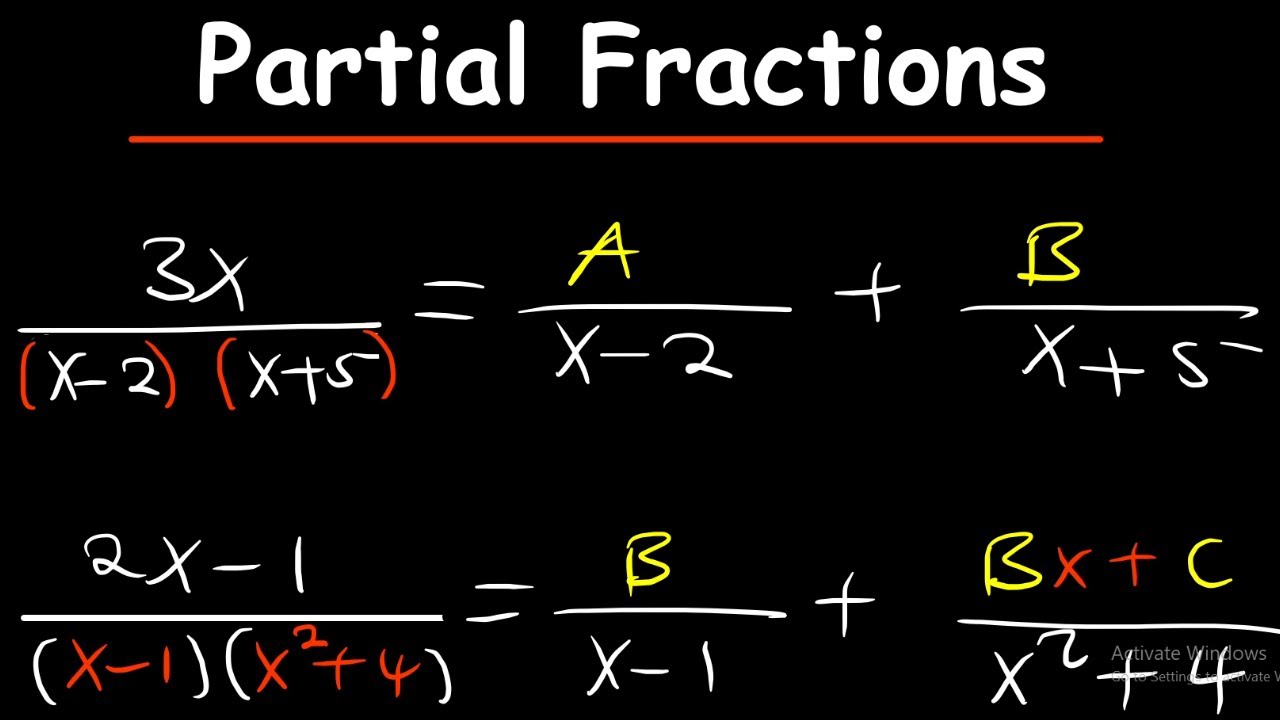
  • 📌 Another example given is decomposing 3x - 4 / (x² - 2x) into partial fractions A / x + B / (x - 2), with the solution being A = 2 and B = 1.
  • 👍 The video emphasizes the importance of practice and understanding the steps involved in partial fraction decomposition for mastering this mathematical technique.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is partial fraction decomposition, a technique used in mathematics to break down complex rational expressions into simpler fractions.

  • Why is it necessary to have a common denominator when dealing with fractions?

    -Having a common denominator is necessary when dealing with fractions to combine them into a single fraction. This process, known as finding a common denominator, allows for easier addition and subtraction of fractions.

  • What is the general process of partial fraction decomposition?

    -The general process of partial fraction decomposition involves starting with a larger, more complicated rational expression and breaking it down into smaller, easier-to-work-with fractions. This is done by factoring the denominator and expressing the rational expression as a sum or difference of simpler fractions with the factors as denominators.

  • How does the video demonstrate the factoring of the denominator in the given example?

    -The video demonstrates the factoring of the denominator by looking at the quadratic expression x^2 - 2x - 15 and factoring it into (x - 5)(x + 3). The factors are then used as the denominators for the partial fractions.

  • What are the general variables used in the numerators of the partial fractions in the example?

    -In the example provided, the general variables used in the numerators of the partial fractions are 'a' for the fraction with (x - 5) in the denominator and 'b' for the fraction with (x + 3) in the denominator.

  • How does the video approach solving for the values of 'a' and 'b' in the partial fractions?

    -The video approaches solving for 'a' and 'b' by setting up a system of equations based on the numerators of the partial fractions after canceling out the denominators. The equations are then solved using elimination method to find the values of 'a' and 'b'.

  • What is the significance of canceling out the terms in the numerator and denominator during the decomposition process?

    -Canceling out terms in the numerator and denominator during the decomposition process simplifies the rational expression, making it easier to solve for the unknown variables 'a' and 'b'. This also helps in obtaining the final form of the partial fraction decomposition.

  • How does the video handle the second example involving the rational expression 3x - 4 over x^2 - 2x?

    -For the second example, the video first factors the denominator x^2 - 2x into (x)(x - 2). It then sets up partial fractions with 'a' over (x) and 'b' over (x - 2). After multiplying both sides of the equation by the factors and distributing, the video solves the resulting system of equations to find that 'a' equals 2 and 'b' equals 1. The final partial fraction decomposition is then 2 over x plus 1 over (x - 2).

  • What is the importance of partial fraction decomposition in mathematics?

    -Partial fraction decomposition is important in mathematics as it simplifies complex rational expressions, making them easier to integrate, analyze, and manipulate. This technique is particularly useful in various fields of engineering, physics, and other scientific disciplines where such mathematical operations are common.

  • What are some alternative methods to solve the system of equations for 'a' and 'b'?

    -Aside from the elimination method used in the video, alternative methods to solve for 'a' and 'b' include substitution and matrix methods. The choice of method often depends on the preference of the individual and the specific characteristics of the system of equations.

Outlines
00:00
📚 Introduction to Partial Fraction Decomposition

This paragraph introduces the concept of partial fraction decomposition, a technique used in mathematics to break down complex rational expressions into simpler fractions. The video begins by discussing the importance of working with fractions and the need for a common denominator when adding or subtracting them. The speaker then presents an example of a rational expression and explains the process of decomposing it into partial fractions. The key points include identifying the factors of the denominator to form the partial fractions and the importance of these factors in the decomposition process. The speaker also emphasizes the goal of transforming a complicated rational expression into a sum or difference of smaller, more manageable fractions.

05:00
🔢 Solving for A and B in Partial Fractions

In this paragraph, the speaker focuses on the process of solving for the unknown coefficients, denoted as 'a' and 'b', in the partial fractions. The method involves setting up a system of equations based on the original rational expression and the decomposed partial fractions. The speaker demonstrates how to eliminate variables and solve for 'a' and 'b' using the method of elimination. The summary highlights the steps of dividing by 'x' to simplify the equations, building the system of equations, and solving for 'a' and 'b'. The speaker also mentions alternative methods such as substitution and matrices but chooses elimination for this example. The goal is to find the values of 'a' and 'b' that will correctly represent the original rational expression as a sum of partial fractions.

10:02
📝 Finalizing the Partial Fraction Decomposition

The final paragraph discusses the completion of the partial fraction decomposition process. The speaker has already found the values for 'a' and 'b', and now substitutes these values back into the partial fraction form to obtain the decomposed rational expression. The speaker emphasizes the importance of checking the work against the original problem to ensure accuracy. The summary outlines the steps of substituting 'a' and 'b' into the partial fractions, simplifying the expression, and verifying the result. The speaker also presents another example to demonstrate the process and reinforces the concept of partial fraction decomposition. The goal is to show that the sum of the partial fractions with the determined coefficients equals the original rational expression, thereby completing the decomposition process.

Mindmap
Keywords
💡Partial Fraction Decomposition
Partial Fraction Decomposition is a mathematical technique used to break down a complex rational expression into simpler fractions. In the context of the video, it involves taking a larger, more complicated fraction and splitting it into a sum or difference of smaller, more manageable fractions. This process is demonstrated by starting with a rational expression like 5x - 1 over x^2 - 2x - 15 and breaking it down into partial fractions with simpler denominators, such as (a/(x-5)) + (b/(x+3)).
💡Rational Expression
A rational expression is a mathematical term that refers to the quotient or ratio of two polynomials. In the video, the initial rational expression is 5x - 1 over x^2 - 2x - 15, which is a fraction where the numerator is a linear term (5x - 1) and the denominator is a quadratic polynomial (x^2 - 2x - 15). Rational expressions are the focus of the video as they are the main objects being decomposed into partial fractions.
💡Denominator
The denominator of a fraction is the bottom part, indicating into how many parts the whole is divided. In the context of the video, the denominator of the initial rational expression is a quadratic polynomial (x^2 - 2x - 15), which is factored to find the partial fractions. The process of partial fraction decomposition involves identifying factors of the denominator to create the simpler fractions that make up the decomposition.
💡Factoring
Factoring is the process of breaking down a polynomial into its constituent factors, which are smaller polynomials that multiply together to give the original polynomial. In the video, factoring is crucial for partial fraction decomposition, as it allows the quadratic denominator to be expressed as a product of linear factors, which then correspond to the denominators of the partial fractions.
💡Common Denominators
Common denominators refer to the same denominator that is shared by multiple fractions. When adding or subtracting fractions, if they have different denominators, it is often necessary to find a common denominator to combine them into a single fraction. However, in the context of partial fraction decomposition, the goal is to work backwards from a single, complex fraction to multiple simpler fractions with different denominators, which were originally derived from the factors of the original denominator.
💡Numerator
The numerator of a fraction is the top part, representing the number of parts being considered from the whole. In the process of partial fraction decomposition, the numerator of the original complex fraction is broken down into the sum of the numerators of the simpler fractions. These numerators are initially represented by variables, such as 'a' and 'b', which are determined through the decomposition process.
💡Variables
In mathematics, variables are symbols that represent unknown quantities or values that can change. In the context of the video, variables such as 'a' and 'b' are used to represent the unknown coefficients in the numerators of the partial fractions during the decomposition process. These variables are later determined by setting up and solving a system of equations based on the decomposed rational expression.
💡System of Equations
A system of equations is a set of mathematical equations that are solved simultaneously. In the video, after decomposing the rational expression into partial fractions with unknown coefficients, a system of equations is formed by equating the decomposed expression to the original expression. This system is then solved to find the values of the variables 'a' and 'b' that complete the decomposition process.
💡Equations
An equation is a mathematical statement that asserts the equality of two expressions. In the context of the video, equations are used to represent the relationship between the original rational expression and its decomposed form as partial fractions. They are also used to set up a system for solving the unknown coefficients in the partial fractions. Solving these equations yields the values of the variables that complete the decomposition process.
💡Canceling
Canceling in mathematics refers to the process of simplifying expressions by eliminating common factors in the numerator and denominator of a fraction. In the video, canceling occurs when multiplying both sides of the equation by the factors of the denominator, which helps to clear the fractions and make the coefficients of the partial fractions visible.
💡Distributing
Distributing is a mathematical operation that involves multiplying a term outside of parentheses to each term inside the parentheses. In the video, distributing is used when multiplying the factors of the denominator across the numerators of the partial fractions. This step is crucial for setting up the system of equations needed to solve for the unknown coefficients in the partial fractions.
Highlights

Introduction to partial fraction decomposition in math, a technique for breaking down complex rational expressions into simpler fractions. (Start time: 0s)

The necessity of finding a common denominator when adding or subtracting fractions, which is reversed during partial fraction decomposition. (Start time: 10s)

Example given: decomposing the rational expression 5x - 1 over x^2 - 2x - 15 into partial fractions. (Start time: 20s)

Step-by-step explanation of factoring the quadratic in the denominator, using x^2 - 2x as an example, and identifying factors that multiply to the constant term and add up to the coefficient of the linear term. (Start time: 30s)

The process of setting up partial fractions using the identified factors as denominators and introducing general variables (a and B) for the numerators. (Start time: 40s)

Clear explanation of multiplying both sides of the equation by the factors to eliminate fractions and the cancellation process that follows. (Start time: 50s)

Formation of a system of linear equations from the decomposed rational expression to solve for the values of a and B. (Start time: 1m)

Demonstration of solving the system of equations using the elimination method to find the values of a and B. (Start time: 1m 30s)

Reconstruction of the original rational expression using the found values of a and B, showcasing the completed partial fraction decomposition. (Start time: 2m)

Second example provided: decomposing 3x - 4 over x^2 - 2x, with a walkthrough of the factoring process and setting up of partial fractions. (Start time: 2m 30s)

Explanation of how to distribute and simplify the equations after multiplying by the factors from the denominator. (Start time: 3m)

Solving for the values of a and B using algebraic manipulation, including the division and substitution methods. (Start time: 3m 30s)

Final step of substituting the found values of a and B back into the partial fraction decomposition to verify the result. (Start time: 4m)

Conclusion summarizing the video's content and expressing gratitude to viewers for watching. (Start time: 4m 30s)

The video's practical application in math, specifically in the field of algebra and its usefulness in simplifying complex rational expressions. (Start time: Throughout)

Innovative method of teaching math concepts through step-by-step video tutorials, which can be beneficial for visual learners. (Start time: Throughout)

Theoretical contribution to the understanding of algebraic fractions and the mathematical community's approach to problem-solving. (Start time: Throughout)

Transcripts

Browse More Related Video

Partial Fraction Decomposion

Partial Fractions Decomposition simplified

AP Calculus BC Lesson 6.12

Ch. 10.7 Partial Fractions

how to setup partial fractions (all cases)

Factoring Cubic Polynomials- Algebra 2 & Precalculus

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsFractionsDecompositionRational ExpressionsAlgebraEducationOnline LearningMath TutorialQuadratic EquationsMathematics Education