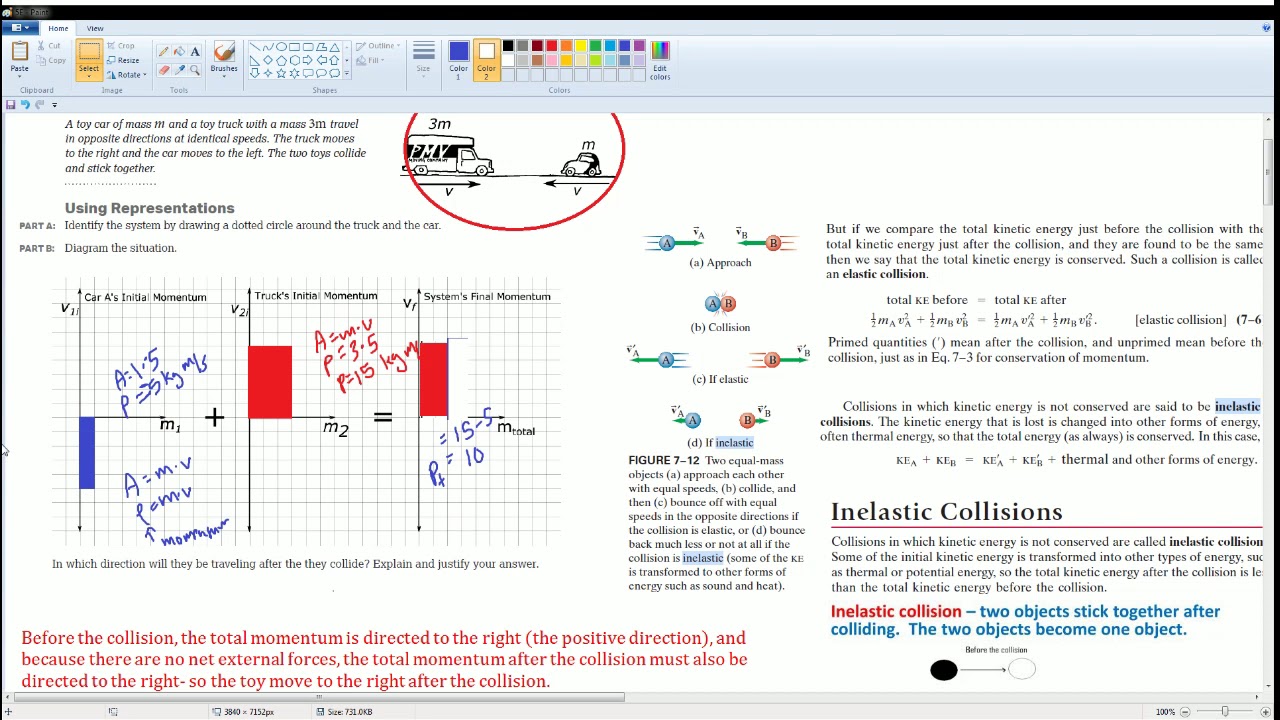
AP PHYSICS 1: Unit 5 FRQ 2 (AP Physics Classroom)

The video begins with Mr. Heinrich introducing himself and mentioning that the focus is on a free-response question (FRQ) from Unit 5 of an AP Physics course. He encourages viewers to subscribe for more content covering up to Unit 7, which is relevant for the upcoming AP tests. The problem at hand involves a ball bouncing at an angle theta naught, and the task is to analyze the change in momentum in two dimensions. Mr. Heinrich explains that momentum is a vector quantity with both magnitude and direction, and he proceeds to break down the problem into its horizontal (x) and vertical (y) components. He emphasizes that the change in momentum is calculated by subtracting the initial momentum from the final momentum for each component.

In this paragraph, Mr. Heinrich delves deeper into the specifics of the momentum problem. He explains that since the ball bounces off at the same angle it came in, there is no change in the horizontal momentum component. This means that the initial and final horizontal momentums are equal, resulting in a change of zero in the x-axis. However, for the vertical component, there is a change because the ball loses some of its momentum upon bouncing. Mr. Heinrich uses the conservation of momentum principle to show that the change in momentum in the y-axis is twice the final vertical momentum (2P2y), which is a result of the ball losing kinetic energy during the bounce. He then relates this to the actual grid diagram that was asked to be filled in the question, indicating that the horizontal component has no change, while the vertical component is represented by six grid boxes, signifying the change in momentum.

Mr. Heinrich transitions to discussing the conservation of energy and momentum in the context of the ball bouncing. He notes that while the question does not explicitly mention air resistance, it is usually not considered in such problems unless stated. He explains that the potential energy of the ball-Earth system is converted into kinetic energy as the ball falls. Upon bouncing, the ball does not reach the same height it started from, indicating a loss of kinetic energy, which is mostly converted into thermal energy due to the inelastic nature of the collision. He also addresses the momentum aspect, explaining that the loss of momentum by the ball is transferred to the ground, causing the molecules to speed up and gain kinetic energy. Mr. Heinrich concludes by stating that this loss of kinetic energy and momentum results in the ball not bouncing back to its original height, thus providing a complete explanation for the observed phenomenon.

In the final paragraph, Mr. Heinrich wraps up the explanation by reiterating the key points. He emphasizes that due to the loss of kinetic energy and momentum during the bounce, the ball will not ascend to the same height it initially fell from (h1), resulting in a smaller height (h2) upon its second bounce. He concludes the video by expressing hope that the explanation was helpful and wishes the viewers a great day, promising to continue the discussion in future videos.