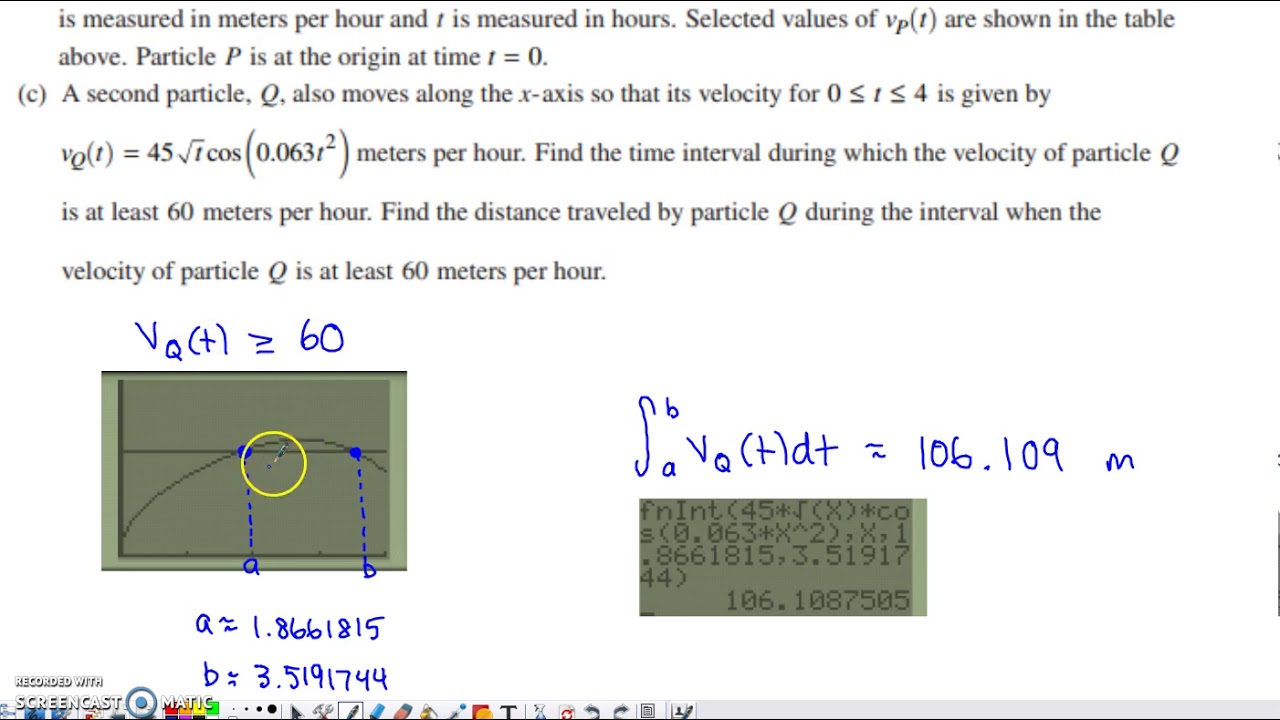
2022 AP Calculus AB Exam FRQ #6
TLDRThe video script presents a detailed walkthrough of a particle motion problem from the 2022 AP Calculus AB exam. The problem involves two particles, P and Q, moving along the x and y axes respectively. Particle P's position is given by a function of time, and its velocity is derived through differentiation. Particle Q's velocity is expressed as a function of time, and its acceleration is found by differentiating the velocity. The script explores when the speed of particle Q is decreasing, which is identified to be for all time greater than zero due to the opposite signs of velocity and acceleration. The position function for particle Q is determined using the fundamental theorem of calculus. Finally, the script examines which particle will be farther from the origin as time approaches infinity, concluding that particle P will be further away since its position limit is 6 units from the origin, compared to particle Q's limit of 3 units.
Takeaways
- 📐 The video discusses problem number six from the 2022 Calculus AB AP exam, which is a particle motion problem involving two particles moving along different axes.
- 🚀 Particle P moves along the x-axis, and its position is given by the formula x_p(t) = 6 - 4e^{-t} for time t > 0.
- 🔄 Particle Q moves along the y-axis with a velocity v_q(t) = 1/t^2 for time t > 0, and its position at t = 1 is y_q(1) = 2.
- 🎯 For part A, the velocity of particle P at time t is found by differentiating its position function, resulting in v_p(t) = 4e^{-t}.
- ⏱️ To find the acceleration of particle Q (a_q(t)), the derivative of its velocity function is taken, yielding a_q(t) = -2/t^3.
- ↕️ The speed of particle Q is always decreasing for t > 0 because its velocity and acceleration are always in opposite directions.
- 🧮 Using the fundamental theorem of calculus, the position of particle Q at time t is calculated as y_q(t) = 3 - 1/t.
- 🌌 As t approaches infinity, the position of particle P tends towards a constant value of 6 units from the origin.
- 📈 Similarly, as t approaches infinity, the position of particle Q tends towards 3 units from the origin along the y-axis.
- 🏁 Particle P will eventually be farther from the origin than particle Q, as 6 is greater than 3, indicating the horizontal movement of P exceeds the vertical movement of Q over time.
- ✅ The video provides a step-by-step solution to the problem, emphasizing the importance of clearly stating relationships between velocity, acceleration, and position.
Q & A
What is the problem discussed in the video?
-The video discusses a particle motion problem from the 2022 calculus AP exam, involving two particles moving along the x and y axes.
How is the position of particle P described in the video?
-The position of particle P is given by the function x_p(t) = 6 - 4e^(-t) for time t greater than zero.
What is the velocity function of particle P?
-The velocity function of particle P, v_p(t), is the derivative of its position function, which is v_p(t) = 4e^(-t).
How is the velocity of particle Q described?
-The velocity of particle Q is given by v_q(t) = 1/t^2 for time t greater than zero.
What is the acceleration function of particle Q?
-The acceleration function of particle Q, a_q(t), is the derivative of its velocity function, which is a_q(t) = -2/t^3.
When is the speed of particle Q decreasing?
-The speed of particle Q is decreasing for all times t greater than zero, as the velocity and acceleration have opposite signs.
What is the position function of particle Q?
-The position function of particle Q, y_q(t), is derived from the integral of its velocity function and is given by y_q(t) = 3 - 1/t.
As time approaches infinity, which particle will be farther from the origin?
-As time approaches infinity, particle P will be farther from the origin since its position limit is 6 units away, compared to particle Q's limit of 3 units.
What is the significance of the subscript notation in the video?
-The subscript notation is used to denote the specific variables for each particle, such as x_p(t) for particle P's position and v_q(t) for particle Q's velocity.
Why does the video mention the Fundamental Theorem of Calculus?
-The Fundamental Theorem of Calculus is mentioned because it is used to find the position function of particle Q by integrating its velocity function over time.
What is the final conclusion about which particle will be farther from the origin?
-The final conclusion is that particle P will eventually be farther from the origin as time approaches infinity, based on the limits of their respective position functions.
What is the reason for the negative sign in the acceleration function of particle Q?
-The negative sign in the acceleration function of particle Q indicates that the acceleration is in the opposite direction to the velocity, which means the particle is slowing down.
Outlines
📐 Calculus AP Exam Problem: Particle Motion Analysis
The video begins with an introduction to a problem from the 2022 Calculus AP exam, specifically number six, which involves particle motion. The problem features two particles, one moving along the x-axis and the other along the y-axis. The position of particle P is given by a formula involving an exponential function, while particle Q's velocity is given as a function of time. The video proceeds to solve for the velocity of particle P (v_p of t) by differentiating its position function. It also finds the acceleration of particle Q (a_q of t) and determines the times when particle Q's speed is decreasing, which is shown to be for all time greater than zero due to the opposite signs of velocity and acceleration. Finally, the video solves for the position of particle Q (y_q of t) using integration and the fundamental theorem of calculus.
🔍 Limit Analysis: Which Particle is Farther from the Origin?
The second paragraph of the video script deals with the limit of particle positions as time approaches infinity to determine which particle will be farther from the origin. The presenter calculates the limit for particle P's position (x_p of t), which simplifies to a constant value of 6, indicating that particle P will be 6 units away from the origin. Similarly, the limit for particle Q's position (y_q of t) is found, which results in 3, meaning particle Q will be 3 units away from the origin along the y-axis. By comparing these values, the presenter concludes that particle P will eventually be farther from the origin since 6 is greater than 3.
Mindmap
Keywords
💡Particle motion
💡Velocity
💡Acceleration
💡Derivative
💡Integral
💡Fundamental Theorem of Calculus
💡Speed
💡Limit
💡Position function
💡Exponential function
💡Power rule
Highlights
The video discusses problem number six from the 2022 AP Calculus exam, focusing on particle motion.
Particle P moves along the x-axis with its position given by the function x_p(t) = 6 - 4e^(-t) for t > 0.
Particle Q moves along the y-axis with its velocity v_q(t) = 1/t^2 for t > 0.
The position of Particle Q at time t=1 is y_q(1) = 2.
The velocity of Particle P, v_p(t), is derived as the derivative of x_p(t), resulting in v_p(t) = 4e^(-t).
The acceleration of Particle Q, a_q(t), is found by differentiating v_q(t), yielding a_q(t) = -2/t^3.
The speed of Particle Q is always decreasing for t > 0, as velocity and acceleration have opposite signs.
The position function for Particle Q, y_q(t), is determined using the fundamental theorem of calculus.
The integral of v_q(t) from 1 to t is solved to find y_q(t), resulting in y_q(t) = 3 - 1/t.
As t approaches infinity, the limit of x_p(t) is 6, indicating Particle P will be 6 units from the origin.
The limit of y_q(t) as t approaches infinity is 3, meaning Particle Q will be 3 units from the origin on the y-axis.
Particle P will eventually be farther from the origin compared to Particle Q, as 6 is greater than 3.
The video provides a step-by-step solution to a calculus problem involving particle motion and calculus concepts.
The use of calculus to model real-world scenarios, such as the motion of particles, is demonstrated.
The importance of clearly stating the relationship between velocity and position is emphasized.
The video also stresses the need to understand the signs of velocity and acceleration to determine the behavior of speed.
The application of the fundamental theorem of calculus to find the position function of a particle is showcased.
The video concludes with a practical application of limits to determine the final position of particles as time approaches infinity.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: